What is the result of −7+3?
-10
-4
4
10
The world of integers, the building blocks of arithmetic. This guide is meticulously designed to demystify integers for both educators and students, presenting concepts in clear, simple English. From understanding basic definitions to exploring complex operations, our examples illuminate the path to mastering integers. Ideal for classroom instruction, this resource aids in breaking down mathematical barriers, fostering a supportive learning environment where students can thrive in their numerical literacy journey.
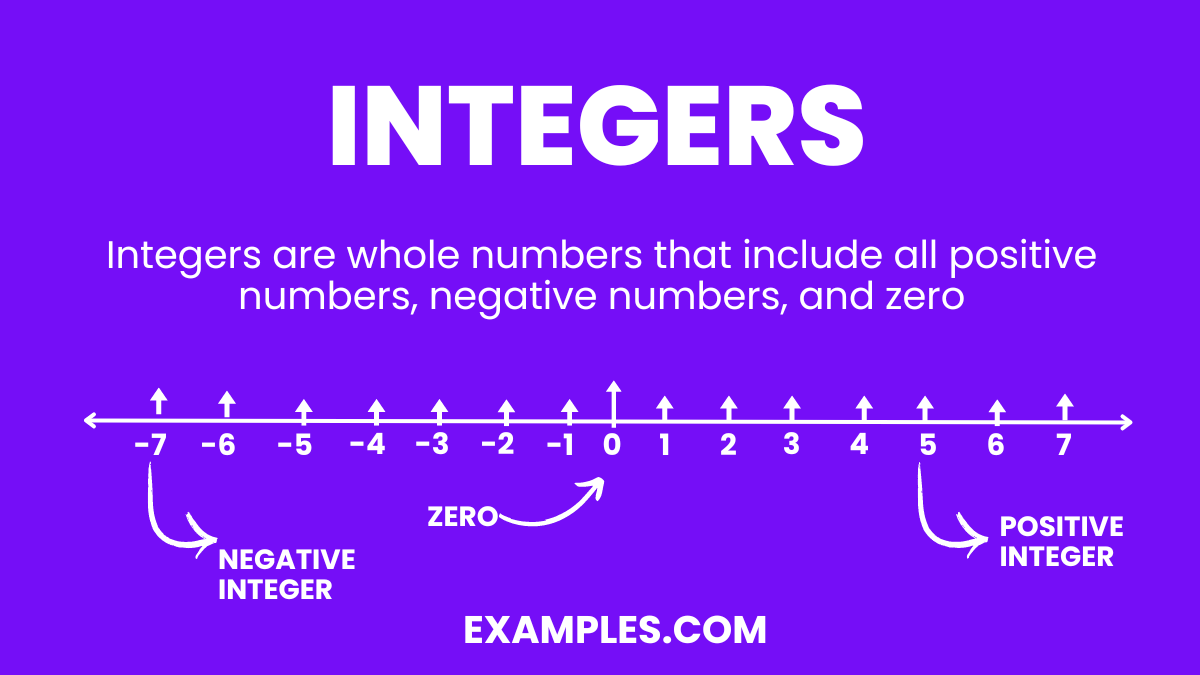
Integers are whole numbers that include all positive numbers, negative numbers, and zero. This set does not include fractions or decimals. Integers are fundamental in mathematics, allowing us to perform various arithmetic operations and understand concepts like positive and negative values.
A standout example of integers in real life is temperature, especially when it dips below zero. Representing temperatures as integers, such as -10°C for a chilly winter day, illustrates how these numbers can be used to describe conditions in the environment, showcasing the practical application of negative values.
Integers encompass the set of whole numbers including positive numbers, negative numbers, and zero. This range makes integers indispensable for various mathematical calculations and real-life applications. Understanding the different types of integers equips students with the tools needed for arithmetic operations, algebra, and beyond, enhancing their mathematical literacy and problem-solving skills.
The classification of integers is a fundamental concept in mathematics that organizes integers into distinct categories based on their properties. This classification helps in simplifying mathematical operations and understanding the underlying patterns within the number system. It is crucial for students to grasp these categories to navigate through more complex mathematical concepts effectively.
The set of integers is a complete collection of whole numbers comprising positive numbers, negative numbers, and zero. This set is symbolized by the letter Z and forms the basis for arithmetic and algebra. Understanding the set of integers is crucial for students to develop a comprehensive mathematical foundation, enabling accurate computation and logical reasoning in everyday scenarios.
Placing integers on a number line is a visual method to understand their value and relationship with each other. This approach demystifies the concept of positive and negative numbers, offering a clear, intuitive understanding of their sequence and spacing. Ideal for educational settings, it aids students in grasping the basics of number theory, enhancing their numerical communication skills with practical, visual examples.
Graphing integers on a number line is an effective tool for visualizing and comparing numbers. It simplifies complex concepts, such as ordering and absolute values, into straightforward, easily digestible visual information. This technique is particularly valuable in teaching scenarios, enabling students to better conceptualize mathematical principles and apply them in various contexts.
Integer operations include addition, subtraction, multiplication, and division, each following specific rules that govern the arithmetic of whole numbers. Understanding these operations is crucial for students, as they form the foundation of mathematical problem-solving and logical reasoning. This knowledge equips learners with the skills to tackle more complex equations and real-life mathematical scenarios.

Mastering the addition of integers is essential for navigating through mathematical concepts, from basic arithmetic to complex algebra. This guide introduces the foundational steps and rules, making it easier for students to understand how positive and negative numbers combine, enriching their arithmetic skills and confidence.
The addition of integers follows simple rules that ensure accuracy in calculation. Understanding these rules helps students correctly assess the sum of positive and negative numbers, enhancing their problem-solving skills.
Subtraction of integers involves specific rules that help in determining the result of combining positive and negative numbers. Understanding these rules is crucial for accurately performing mathematical operations and solving problems. Here’s how these rules apply to the subtraction of integers:
Subtracting a smaller positive integer from a larger positive integer results in a positive difference, as the overall value decreases but remains positive. For example, 8−3=5. However, if the smaller number is subtracted from the larger one, the result is negative, such as 3−8=−5, reflecting a decrease beyond zero.
Subtracting a negative integer from another negative integer where the minuend is less negative than the subtrahend results in a positive difference, such as (−3)−(−7)=4, since removing a negative is akin to adding a positive. If the minuend is more negative, the result is negative, like (−7)−(−3)=−4, as the value decreases further.
Subtracting a negative integer from a positive integer increases the overall value, resulting in a positive difference. This is because subtracting a negative is the same as adding its absolute value, such as 7−(−2)=9.
When a positive integer is subtracted from a negative integer, the overall value decreases, resulting in a larger negative difference. This reflects further movement away from zero on the number line, such as (−3)−4=−7.
Subtracting several integers involves applying the rules sequentially, taking into account the sign of each integer and its effect on the total value. For example, (−2)−3−(−5)−4=−4, demonstrates the combined effect of subtracting both positive and negative integers from an initial value.
These rules for the subtraction of integers simplify the process of dealing with positive and negative numbers, allowing for straightforward calculations and problem-solving in mathematics.
Multiplication of integers extends the concept of addition by focusing on repeated addition. It’s vital for students to grasp these rules to effectively engage with various mathematical operations and real-life applications.
Understanding the rules of integer multiplication allows students to navigate through calculations with ease, fostering a deeper comprehension of mathematical operations.
Division of integers is akin to multiplication but involves distributing a total into equal parts. It’s crucial for understanding fractions, ratios, and proportional reasoning.
The division of integers follows specific rules that mirror those of multiplication, guiding students through calculations involving division by positive and negative numbers.
Integers embody a rich tapestry of mathematical properties that facilitate various operations. These include the commutative, associative, and distributive properties for addition and multiplication, along with the existence of additive inverses and identity elements. Understanding these properties is crucial for grasping the fundamental concepts of arithmetic and algebra, making integers a pivotal topic in math education.
Representing integers on a number line is a visual method to illustrate the position of integers in relation to each other. This approach helps in understanding the concept of magnitude, the difference between positive and negative integers, and their relative distances from zero. It’s a foundational skill in mathematics, aiding students in visualizing and comprehending integers’ behavior and relationships.
Integers find extensive applications in various fields, reflecting their importance beyond the classroom. From representing real-world quantities to encoding information in computer science, integers form the backbone of numerous practical and theoretical domains, underscoring their ubiquity and utility in everyday life and advanced scientific research.
In conclusion, integers encapsulate a fundamental aspect of mathematics, bridging simple counting with the complex realms of algebra and beyond. Through exploring their properties, representations, and wide-ranging applications, this guide illuminates the integral role integers play in both educational settings and real-world scenarios. Tailored for educators and students, it aims to demystify integers, fostering a deeper understanding and appreciation of this essential mathematical concept.

The world of integers, the building blocks of arithmetic. This guide is meticulously designed to demystify integers for both educators and students, presenting concepts in clear, simple English. From understanding basic definitions to exploring complex operations, our examples illuminate the path to mastering integers. Ideal for classroom instruction, this resource aids in breaking down mathematical barriers, fostering a supportive learning environment where students can thrive in their numerical literacy journey.
Integers are whole numbers that include all positive numbers, negative numbers, and zero. This set does not include fractions or decimals. Integers are fundamental in mathematics, allowing us to perform various arithmetic operations and understand concepts like positive and negative values.
A standout example of integers in real life is temperature, especially when it dips below zero. Representing temperatures as integers, such as -10°C for a chilly winter day, illustrates how these numbers can be used to describe conditions in the environment, showcasing the practical application of negative values.
Integers encompass the set of whole numbers including positive numbers, negative numbers, and zero. This range makes integers indispensable for various mathematical calculations and real-life applications. Understanding the different types of integers equips students with the tools needed for arithmetic operations, algebra, and beyond, enhancing their mathematical literacy and problem-solving skills.
Positive Integers (+1, +2, +3,…):
Represent quantities or amounts above zero. For instance, saving $5 is represented as +5 in banking.
Negative Integers (-1, -2, -3,…):
Used to denote debts or below-zero temperatures. A debt of $5 is represented as -5.
Zero (0):
Acts as a neutral element in addition and subtraction. For example, a balance of zero in an account.
Even Integers (0, ±2, ±4, ±6,…):
Divisible by 2 with no remainder. For instance, 2 apples, making it an even distribution.
Odd Integers (±1, ±3, ±5,…):
Not evenly divisible by 2. For example, 3 cookies shared among two people.
The classification of integers is a fundamental concept in mathematics that organizes integers into distinct categories based on their properties. This classification helps in simplifying mathematical operations and understanding the underlying patterns within the number system. It is crucial for students to grasp these categories to navigate through more complex mathematical concepts effectively.
Prime Integers (2, 3, 5, 7,…):
Numbers greater than 1, divisible only by 1 and themselves. For example, 2 is prime because its only divisors are 1 and 2.
Composite Integers (4, 6, 8,…):
Numbers that have divisors other than 1 and themselves. For instance, 4 can be divided by 1, 2, and 4.
Non-negative Integers (0, 1, 2,…):
All positive integers including zero. For example, the number of students in a class.
Non-positive Integers (0, -1, -2,…):
All negative integers including zero. For example, temperatures below zero.
Absolute Value Integers (|−5| = 5, |3| = 3):
Represents the distance of a number from zero. For instance, both -5 and 5 have an absolute value of 5.
The set of integers is a complete collection of whole numbers comprising positive numbers, negative numbers, and zero. This set is symbolized by the letter Z and forms the basis for arithmetic and algebra. Understanding the set of integers is crucial for students to develop a comprehensive mathematical foundation, enabling accurate computation and logical reasoning in everyday scenarios.
The Counting Numbers {1, 2, 3,…}:
Also known as natural numbers, used for counting objects. For example, counting 3 books.
Whole Numbers {0, 1, 2, 3,…}:
Natural numbers plus zero. For instance, indicating no error messages as 0.
Negative of Natural Numbers {-1, -2, -3,…}:
Used in financial contexts to represent debts or withdrawals. For example, withdrawing $10 is -10.
Zero {0}:
Represents nothingness or a neutral state. For example, a game starting score.
All Integers {…, -3, -2, -1, 0, 1, 2, 3,…}:
The complete set used for various mathematical and real-life applications. For instance, elevation levels above and below sea level.
Placing integers on a number line is a visual method to understand their value and relationship with each other. This approach demystifies the concept of positive and negative numbers, offering a clear, intuitive understanding of their sequence and spacing. Ideal for educational settings, it aids students in grasping the basics of number theory, enhancing their numerical communication skills with practical, visual examples.
Zero as the Origin
Zero serves as the central point, dividing the line into positive and negative integers, illustrating the balance of numbers.
Positive Numbers to the Right
Placing positive integers to the right of zero shows ascending values, aiding in visualizing growth and progression.
Negative Numbers to the Left
Negative integers are placed to the left of zero, representing values less than zero, clarifying the concept of debts or below zero temperatures.
Equal Spacing
Each integer is equally spaced, emphasizing that the difference between consecutive integers is always the same, highlighting uniformity in value change.
Marking Integers
Marking specific integers, like -5 or 5, helps students quickly locate and understand their positions relative to zero, enhancing spatial numerical understanding.
Graphing integers on a number line is an effective tool for visualizing and comparing numbers. It simplifies complex concepts, such as ordering and absolute values, into straightforward, easily digestible visual information. This technique is particularly valuable in teaching scenarios, enabling students to better conceptualize mathematical principles and apply them in various contexts.
Comparing Values
By graphing -3 and 3, students can visually comprehend the concept of absolute value and the symmetry of the number line.
Sequencing Activities
Plotting a series of integers, like -2, 1, and 4, on the number line helps students understand the order and distance between numbers.
Distance from Zero
Graphing integers shows their distance from zero, clarifying the notion of magnitude and how far a number is from the origin.
Intervals
Highlighting intervals between two integers, such as -1 to 3, visually represents the concept of range and inclusivity on the number line.
Negative and Positive Sides
Graphing integers on both sides of zero aids in distinguishing between positive and negative realms, fostering an understanding of directional value change.
Integer operations include addition, subtraction, multiplication, and division, each following specific rules that govern the arithmetic of whole numbers. Understanding these operations is crucial for students, as they form the foundation of mathematical problem-solving and logical reasoning. This knowledge equips learners with the skills to tackle more complex equations and real-life mathematical scenarios.
Addition with Positive Integers
Adding two positive integers, like 2 + 3, results in a positive sum, illustrating basic accumulation.
Subtraction Leading to Negative Result
Subtracting a larger integer from a smaller one, such as 3 – 5, demonstrates how operations can cross the zero point, resulting in a negative outcome.
Multiplication with Mixed Signs
Multiplying integers with different signs, like -4 * 2, shows that the product of a negative and a positive integer is negative, illustrating sign rules.
Division Resulting in Negative Quotient
Dividing a negative integer by a positive one, such as -8 / 2, yields a negative quotient, reinforcing the concept of sign influence in division.
Combining Operations
Performing multiple operations, like (-3 + 2) * 4, helps students understand the order of operations and the cumulative effect on integer values.

Mastering the addition of integers is essential for navigating through mathematical concepts, from basic arithmetic to complex algebra. This guide introduces the foundational steps and rules, making it easier for students to understand how positive and negative numbers combine, enriching their arithmetic skills and confidence.
Positive + Positive = Positive
Adding two positive integers, like 5 + 3 = 8, results in a positive sum, reflecting increased value.
Negative + Negative = Negative
Combining two negative integers, such as -4 + (-6) = -10, intensifies the decrease in value.
Positive + Negative
Adding a positive and a negative integer, like 7 + (-2) = 5, depends on the numbers’ magnitudes.
Negative + Positive
When a negative integer is added to a positive one, like -3 + 4 = 1, the outcome is determined by the larger absolute value.
Multiple Integers
Combining several integers, like -2 + 3 + (-5) + 4 = 0, showcases how different signs interact in addition.
The addition of integers follows simple rules that ensure accuracy in calculation. Understanding these rules helps students correctly assess the sum of positive and negative numbers, enhancing their problem-solving skills.
Same Signs
Integers with the same sign, when added, keep the sign and sum the absolute values.
Different Signs
Integers with different signs, when added, take the sign of the larger absolute value and subtract the smaller from the larger.
Zero
Adding zero to any integer does not change its value, demonstrating the identity property of addition.
Sequential Addition
When adding more than two integers, group them by sign for easier calculation.
Using a Number Line
Visualizing integer addition on a number line can simplify understanding, especially with opposite signs.
Subtraction of integers involves specific rules that help in determining the result of combining positive and negative numbers. Understanding these rules is crucial for accurately performing mathematical operations and solving problems. Here’s how these rules apply to the subtraction of integers:
Subtracting a smaller positive integer from a larger positive integer results in a positive difference, as the overall value decreases but remains positive. For example, 8−3=5. However, if the smaller number is subtracted from the larger one, the result is negative, such as 3−8=−5, reflecting a decrease beyond zero.
Subtracting a negative integer from another negative integer where the minuend is less negative than the subtrahend results in a positive difference, such as (−3)−(−7)=4, since removing a negative is akin to adding a positive. If the minuend is more negative, the result is negative, like (−7)−(−3)=−4, as the value decreases further.
Subtracting a negative integer from a positive integer increases the overall value, resulting in a positive difference. This is because subtracting a negative is the same as adding its absolute value, such as 7−(−2)=9.
When a positive integer is subtracted from a negative integer, the overall value decreases, resulting in a larger negative difference. This reflects further movement away from zero on the number line, such as (−3)−4=−7.
Subtracting several integers involves applying the rules sequentially, taking into account the sign of each integer and its effect on the total value. For example, (−2)−3−(−5)−4=−4, demonstrates the combined effect of subtracting both positive and negative integers from an initial value.
These rules for the subtraction of integers simplify the process of dealing with positive and negative numbers, allowing for straightforward calculations and problem-solving in mathematics.

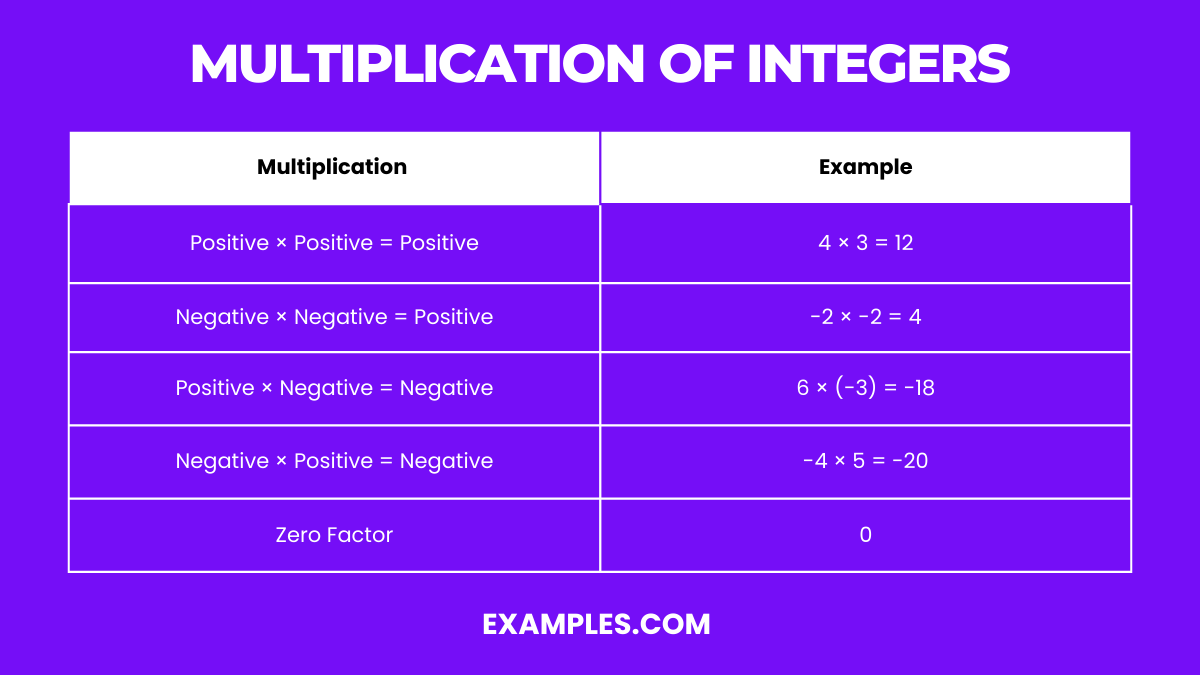
Multiplication of integers extends the concept of addition by focusing on repeated addition. It’s vital for students to grasp these rules to effectively engage with various mathematical operations and real-life applications.
Positive × Positive = Positive
Multiplying two positive integers, like 4 × 3 = 12, results in a positive product.
Negative × Negative = Positive
When two negative integers are multiplied, such as -2 × -2 = 4, the product is positive.
Positive × Negative = Negative
A positive and a negative integer, like 6 × (-3) = -18, result in a negative product.
Negative × Positive = Negative
A negative integer multiplied by a positive one, like -4 × 5 = -20, yields a negative product.
Zero Factor
Multiplying any integer by zero results in zero, emphasizing the zero property of multiplication.
Understanding the rules of integer multiplication allows students to navigate through calculations with ease, fostering a deeper comprehension of mathematical operations.
Product Sign
The sign of the product depends on the signs of the factors: two negatives make a positive, while a positive and a negative give a negative.
Commutative Property
The order of multiplication does not affect the product, ensuring flexibility in calculation.
Associative Property
Grouping factors in multiplication does not change the outcome, aiding in complex computations.
Distributive Property
Multiplying a sum by an integer distributes over addition, simplifying expressions.
Multiplicative Identity
Multiplying any integer by one leaves it unchanged, demonstrating the identity property of multiplication.
Division of integers is akin to multiplication but involves distributing a total into equal parts. It’s crucial for understanding fractions, ratios, and proportional reasoning.
Positive ÷ Positive = Positive
Dividing two positive integers, like 8 ÷ 2 = 4, results in a positive quotient.
Negative ÷ Negative = Positive
A negative integer divided by another negative, such as -10 ÷ -2 = 5, yields a positive quotient.
Positive ÷ Negative = Negative
Dividing a positive by a negative integer, like 12 ÷ (-3) = -4, results in a negative quotient.
Negative ÷ Positive = Negative
A negative integer divided by a positive one, like -15 ÷ 3 = -5, produces a negative quotient.
Division by Zero
Division by zero is undefined, highlighting a fundamental rule in mathematics.
The division of integers follows specific rules that mirror those of multiplication, guiding students through calculations involving division by positive and negative numbers.
Quotient Sign
The sign of the quotient depends on the signs of the dividend and divisor, with like signs resulting in a positive quotient and unlike signs in a negative quotient.
Dividing by One
Dividing any integer by one yields the integer itself, reflecting the identity property of division.
Zero Divided by an Integer
Zero divided by any non-zero integer results in zero, emphasizing the zero property of division.
Undefined Division
Division by zero is undefined, a critical rule ensuring the coherence of mathematical operations.
Reciprocal Multiplication
Dividing by an integer is equivalent to multiplying by its reciprocal, facilitating the understanding of division operations.
Integers embody a rich tapestry of mathematical properties that facilitate various operations. These include the commutative, associative, and distributive properties for addition and multiplication, along with the existence of additive inverses and identity elements. Understanding these properties is crucial for grasping the fundamental concepts of arithmetic and algebra, making integers a pivotal topic in math education.
Commutative Property of Addition
For any integers a and b, a + b = b + a. This property illustrates that the order in which two numbers are added does not affect the sum.
Associative Property of Multiplication
Given three integers a, b, and c, (a * b) * c = a * (b * c). This demonstrates how grouping does not change the product.
Distributive Property
For any integers a, b, and c, a * (b + c) = ab + ac. It shows how multiplication is distributed over addition.
Additive Inverse
For any integer a, there exists an integer -a such that a + (-a) = 0. This property highlights the concept of negation in integers.
Identity Element of Addition
For any integer a, a + 0 = a. Zero acts as the additive identity since adding it to any integer returns the original integer.
Representing integers on a number line is a visual method to illustrate the position of integers in relation to each other. This approach helps in understanding the concept of magnitude, the difference between positive and negative integers, and their relative distances from zero. It’s a foundational skill in mathematics, aiding students in visualizing and comprehending integers’ behavior and relationships.
Marking Positive Integers
Positive integers are placed to the right of zero, each unit step representing an increase by one.
Marking Negative Integers
Negative integers appear to the left of zero, each unit step indicating a decrease by one, demonstrating their position in relation to zero.
Zero as the Origin
Zero is centrally located on the number line, serving as a reference point for distinguishing positive and negative integers.
Equal Distance
Integers equidistant from zero on opposite sides illustrate the concept of absolute value and opposites.
Interval between Integers
The space between any two consecutive integers represents a unit step, crucial for understanding integer addition and subtraction.
Integers find extensive applications in various fields, reflecting their importance beyond the classroom. From representing real-world quantities to encoding information in computer science, integers form the backbone of numerous practical and theoretical domains, underscoring their ubiquity and utility in everyday life and advanced scientific research.
Financial Transactions
Positive integers represent earnings or deposits, while negative integers denote expenses or withdrawals, facilitating clear financial records.
Temperature Measurements
Temperatures above and below zero are represented by positive and negative integers, respectively, essential for weather forecasting and scientific research.
Elevation Levels
Above sea level elevations are indicated by positive integers, and below sea level depths by negative integers, vital for geographical and environmental studies.
Sports Scoring
Scores in games can be added or subtracted using integers, making the tracking of points straightforward and efficient.
Computer Science
Integers are used in programming for counting, indexing, and operations, foundational for algorithms and data processing.
In conclusion, integers encapsulate a fundamental aspect of mathematics, bridging simple counting with the complex realms of algebra and beyond. Through exploring their properties, representations, and wide-ranging applications, this guide illuminates the integral role integers play in both educational settings and real-world scenarios. Tailored for educators and students, it aims to demystify integers, fostering a deeper understanding and appreciation of this essential mathematical concept.
Text prompt
Add Tone
Types of Integers
Classification of Integers
What is the result of −7+3?
-10
-4
4
10
What is −8 × 6?
-48
-14
14
48
Which of the following is the additive inverse of 9?
-9
0
9
18
What is the result of 12−(−5)?
7
-7
17
-17
What is −15 ÷ 3?
-5
-3
3
5
Which number is not an integer?
-4
0
2.5
10
What is the result of 7 + (−9)?
-16
-2
2
16
What is (−6) × (−4)?
-24
-10
10
24
What is the result of −9 + 9?
-18
-9
0
18
What is 20 ÷ (−4)?
-5
-4
4
5
Before you leave, take our quick quiz to enhance your learning!

