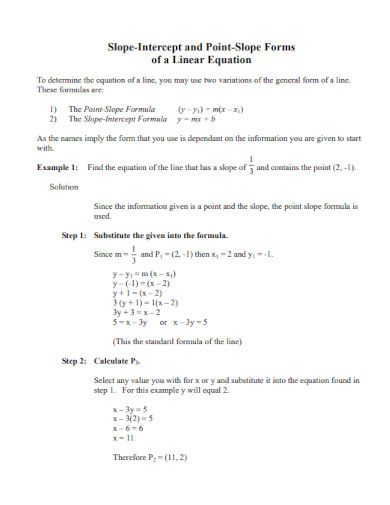
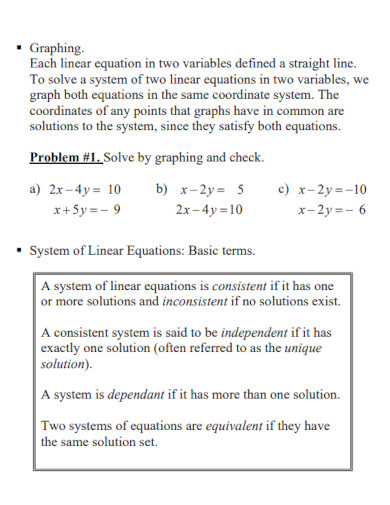
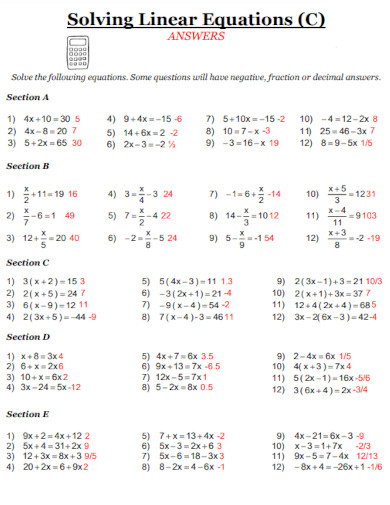
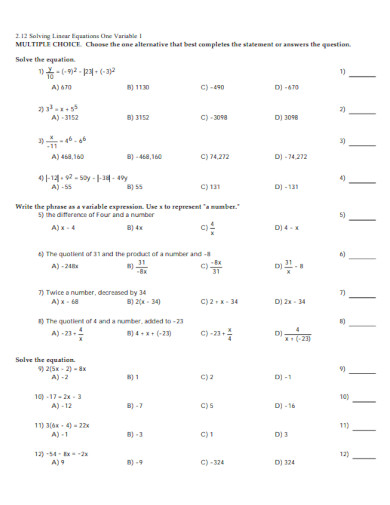
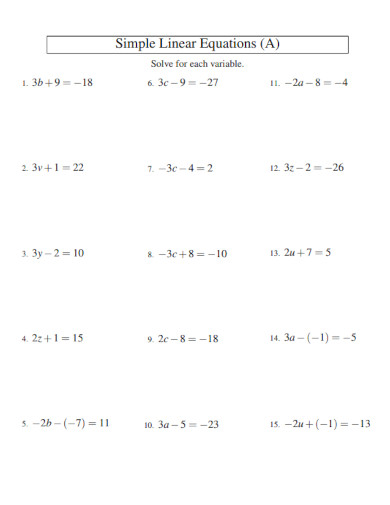
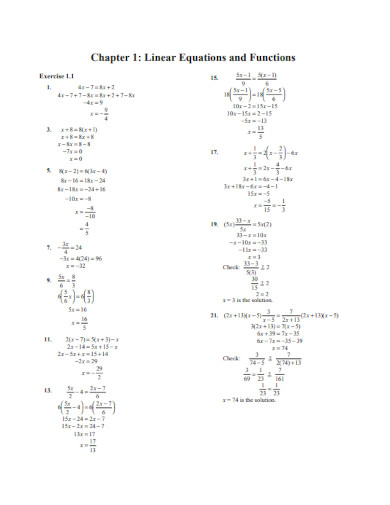
Solve for x: 2x + 3 = 11
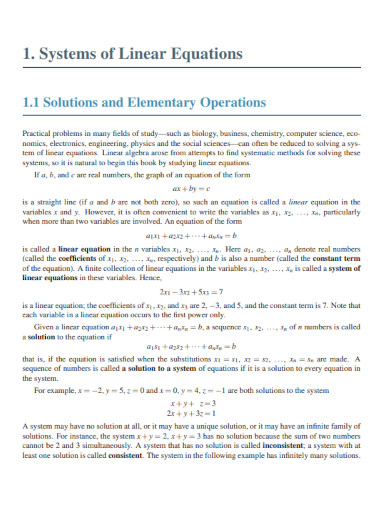
2
3
4
5

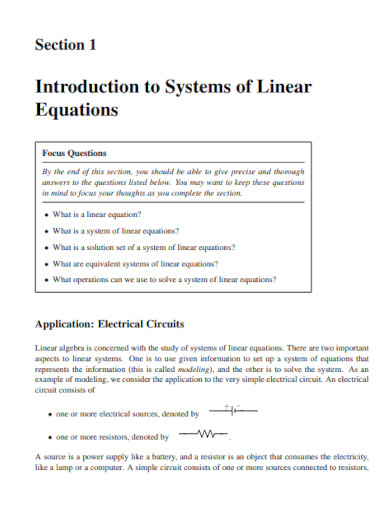
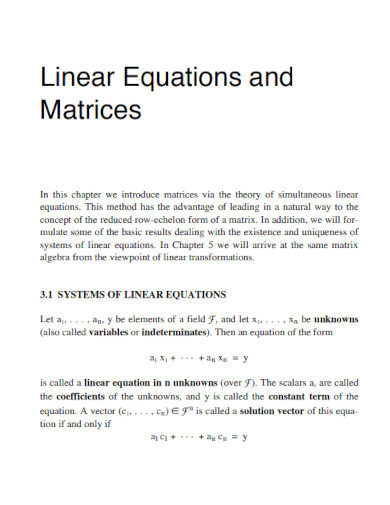
Lines are illustrations of the relationship between two points in a given plane. This means that the make and the direction are directly affected by the location of the two points. You can easily illustrate this relationship through linear equations and lines.
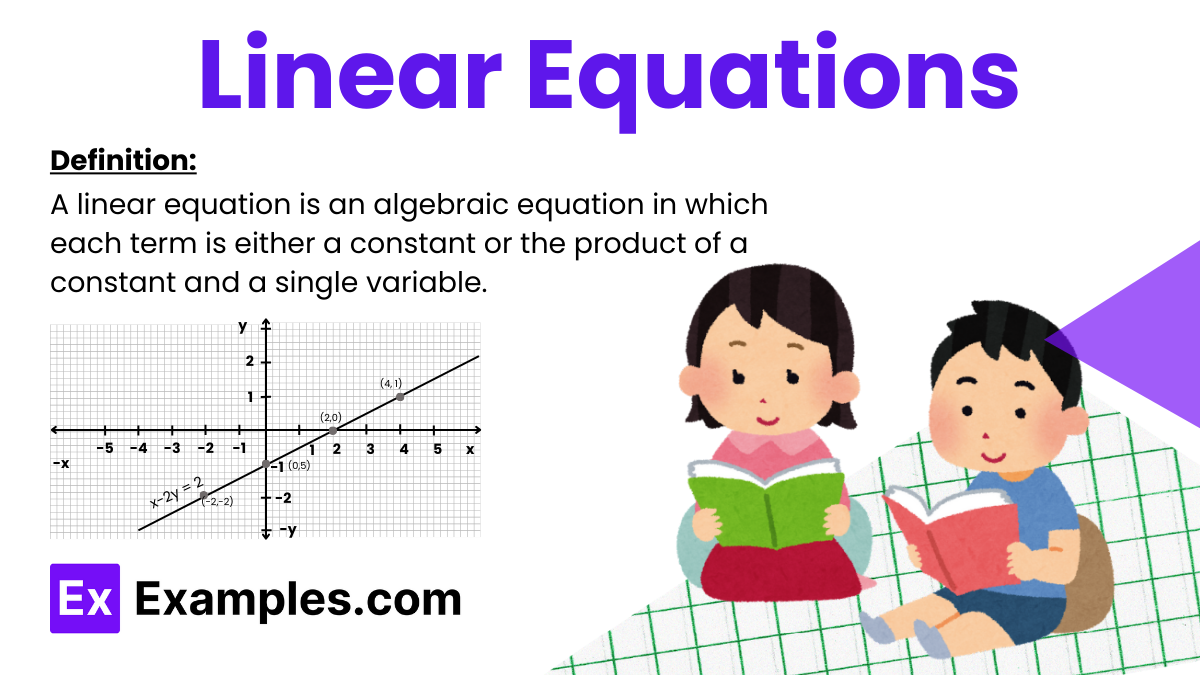
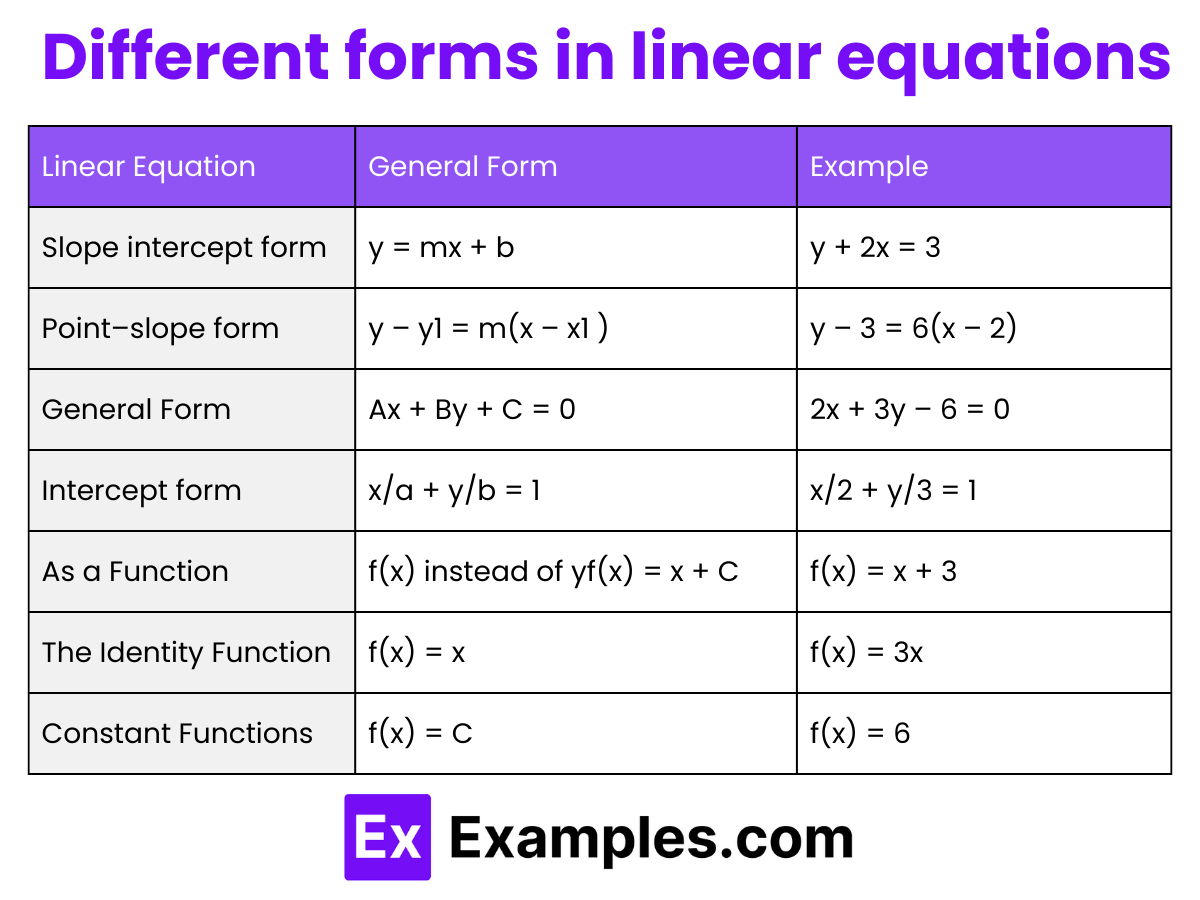
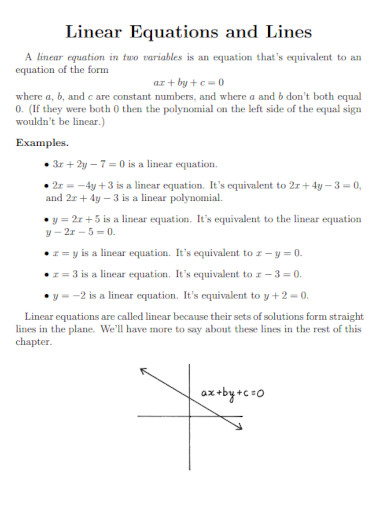
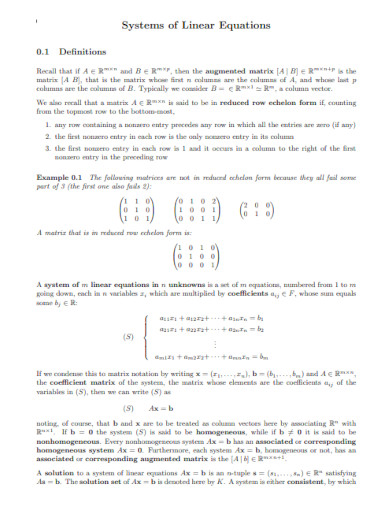
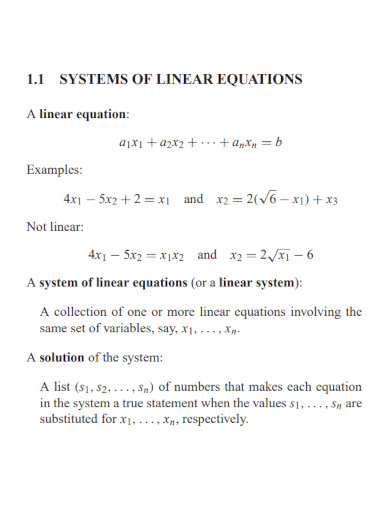
A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and a single variable. Linear equations can be represented in various forms, but the most common is the standard form: ax+by=c
| Equations | Linear or Non-Linear |
|---|---|
| y = 8x – 10 | Linear |
| y = x² – 22 | Non-Linear, the power of the variable x is 2 |
| √y + x = 4 | Non-Linear, the power of the variable y is 1/2 |
| y + 8x – 1 = 0 | Linear |
| y² – x = 7 | Non-Linear, the power of the variable y is 2 |
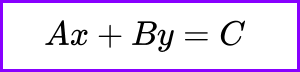
In this form, A, B, and C are constants. The equation describes a line in two-dimensional space, and neither variable is isolated on one side of the equation.

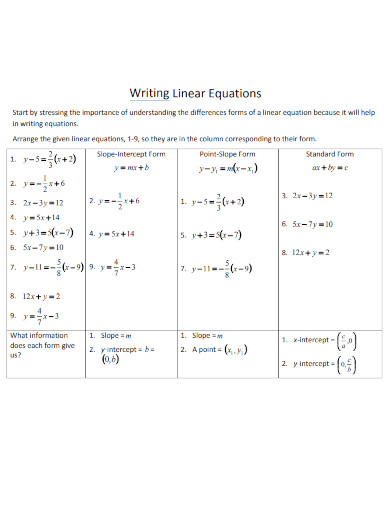
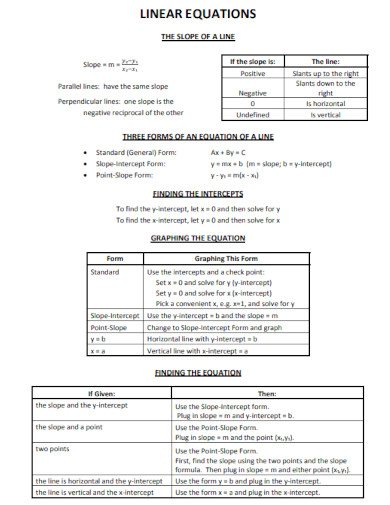
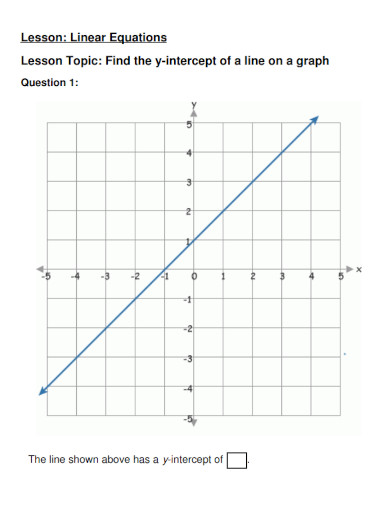
This is perhaps the most frequently used form when dealing with linear equations in basic algebra. Here, m represents the slope of the line, and b represents the y-intercept, which is the point where the line crosses the y-axis. This form makes it easy to graph the equation and to understand how changes in x affect y.
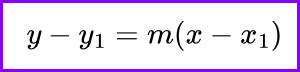
This form is useful when you know a point on the line (x₁,y₁) and the slope m. It’s particularly handy for writing the equation of a line when given a point and the slope without having to calculate the y-intercept.

The graph of a linear equation involving one variable, such as x, forms a vertical line that is parallel to the y-axis. Conversely, if the equation involves only the variable y, the graph will be a horizontal line parallel to the x-axis. On the other hand, the graph of a linear equation involving two variables, x and y, forms a straight line. This allows us to visually interpret the relationship between the variables. Let’s explore how to graph a linear equation with two variables through the following example:
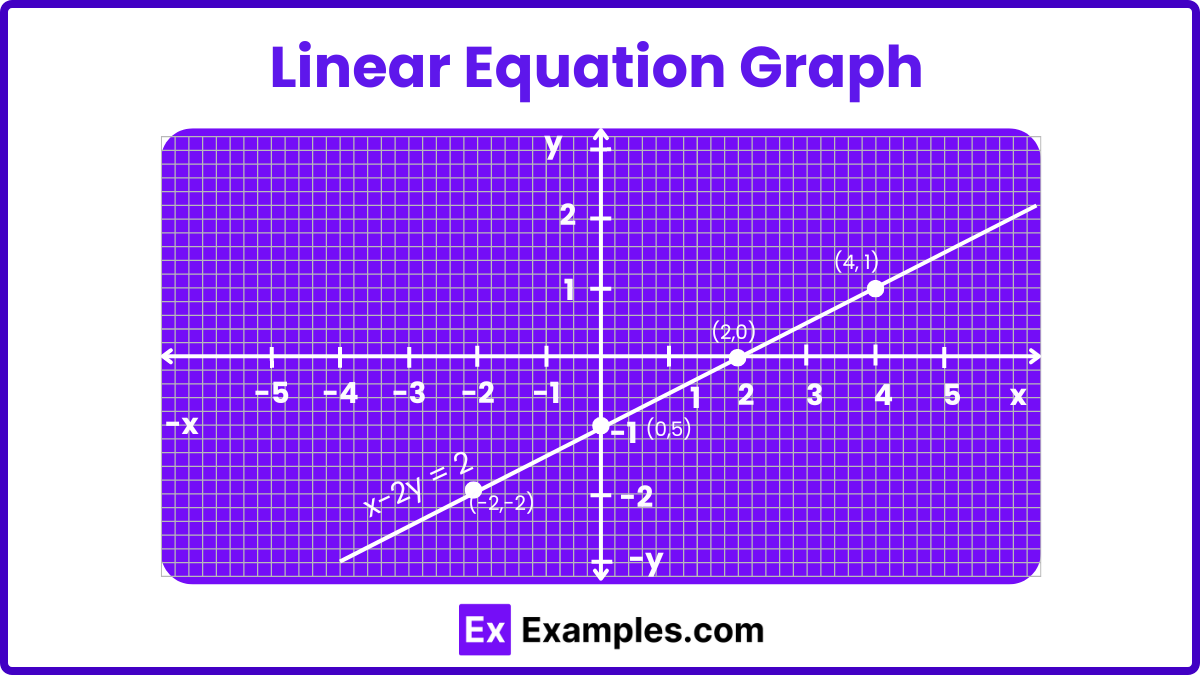
Example: Graphing the Linear Equation x−2y=2
To plot the graph of the linear equation in two variables, follow these steps:
Step 1: Identify the equation. The given equation is x−2y=2
Step 2: Convert the equation to slope-intercept form (y = mx + b). Rearrange x−2y=2 to express y in terms of x: y=(x/2)−1
This puts the equation in the form where m (slope) is 1/2 and b (y-intercept) is −1.
Step 3: Calculate coordinates by substituting different values of x.
Step 4: Plot these points and draw the line. List the coordinates and plot them on a Cartesian plane:
| x | 0 | 2 | 4 | -2 |
|---|---|---|---|---|
| y | -1 | 0 | 1 | -2 |
Connect these points with a straight line to visualize the relationship defined by the equation y=x/2−1. Each pair of (x, y) values satisfies the equation, illustrating the linear relationship between x and y.
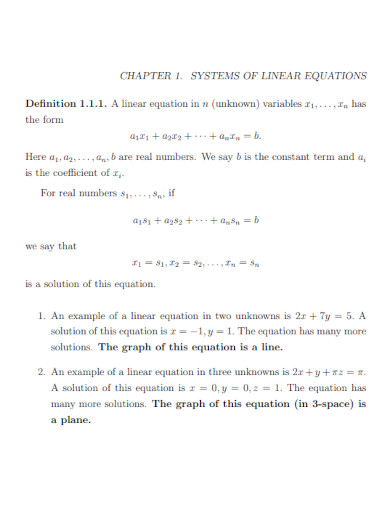
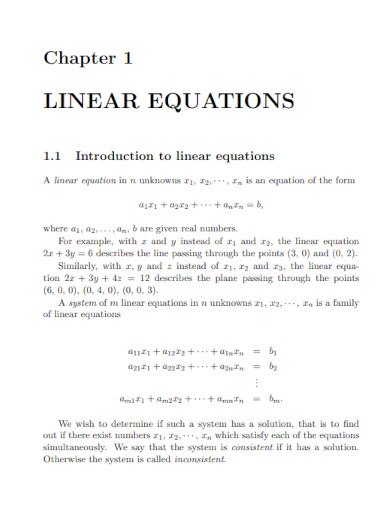
Definition: A linear equation in one variable is an equation that can be written in the form ax+b=0, where aaa and bbb are constants and x is the variable. The goal is to find the value of x that makes the equation true.
Standard Form: ax+b=0
Steps to Solve:
Example: Solve 3x+5=11
Applications:
Definition: A linear equation in two variables is an equation that can be written in the form ax+by+c=0, where a, b, and c are constants, and x and y are variables. This type of equation represents a straight line when graphed on a coordinate plane.
Standard Form: ax+by+c=0
General Form: y=mx+b Where m is the slope and b is the y-intercept.
Methods to Solve:
Steps to Solve:
Methods to Solve:
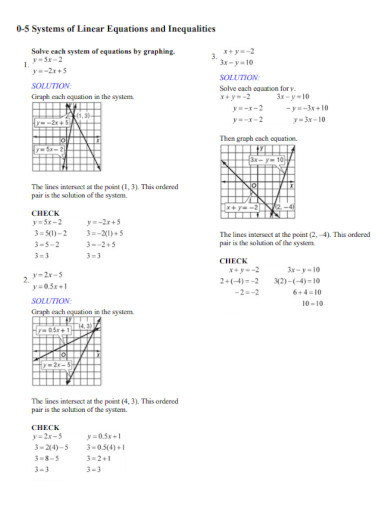
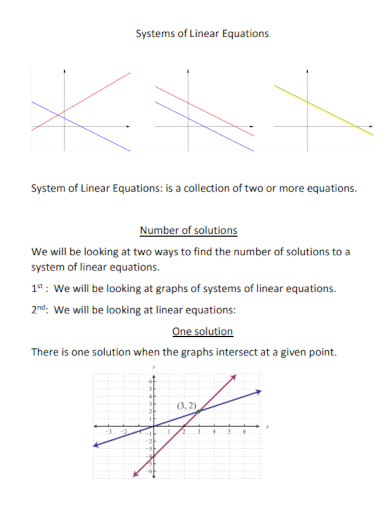
Linear equations are equations that illustrate the proportional relationship between two variables and points. Simple linear equations are equations with a single variable, while there are linear equations with two variables, and even linear equations with three variables. All of which have their systems of linear equations and ways of solving said linear equations.
A can come in many different forms. The standard form of a linear equation with two variables is Ax + By = C where x is the X coordinate, y is the Y coordinate, and C is the constant number. One variable linear equation will come in the form of Ax + B = C.
Begin by writing down the equation in a physical note, or digital note-taking software. This will help you visualize the equation without needing to backread the question.
When you have finished writing down the equation, check whether the equation has one point or two points. You will be able to know this by the presence of X and Y coordinates in the equation. If the equation follows the Ax + By + C = 0 formats, it will require specific X and Y values that you can obtain from a different formula. Only use this how-to with equations that use the standard form of the linear equation or the standard one-variable linear equation.
When you have finished discerning the equation, you must simplify the equation to its simplest form. This means if there is a common denominator between the three variables, then you must divide them by said common denominator.
After you have simplified the equation, you must isolate and move one of the variables to the equals sign. The equation would either come in the form of x = C – B, Ax = C – By, or By = C – Ax.
After isolating one of the points, you must now substitute the isolated variable with specific points. For example, if 2x = 2 – Y is the linear equation, we can substitute the x value to be x = 0. The equation will then become 2(0) = 2 – Y, which will equal to Y = 2.
Repeat these steps until you have up to four sets of X and Y coordinates, note that each set will be a pair. Following the example above, the set of X and Y coordinates will be (0,2). Once you have four sets of these coordinates, graph the linear equation into the cartesian plane using these coordinates as your reference points. Afterward, you will connect all the reference points to create a straight line.
To solve linear equations, isolate the variable by performing inverse operations. Simplify the equation step-by-step, combining like terms and balancing both sides until the variable is isolated.
A linear equation has variables with an exponent of 1 and graphs as a straight line. It typically appears in the form ax+b=c Where a, b, and c are constants.
Linear equations represent relationships where the change between variables is constant. They are expressed in the form ax+by=c, producing straight-line graphs.
A linear equation is an algebraic equation with a constant rate of change between variables, represented as a straight line. Examples: y= 4x+2, 2x+3y = 6
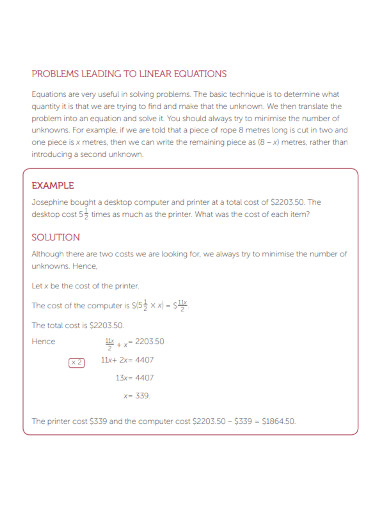
A real-life example of a linear equation is calculating the total cost (C) of items given a fixed price per item (p) and quantity (q): C=pq.
A linear equation is like a simple rule that tells you how to get from one number to another using addition, subtraction, multiplication, or division. For example, if you have 2x+3=7, you find out what number makes it true.
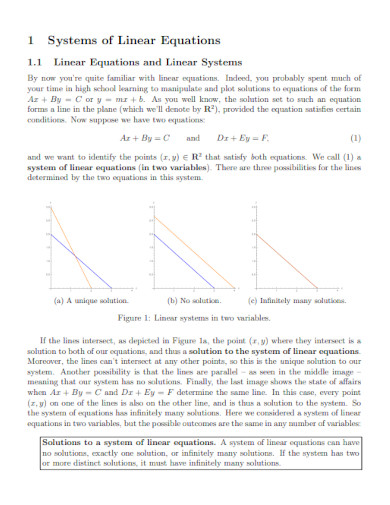
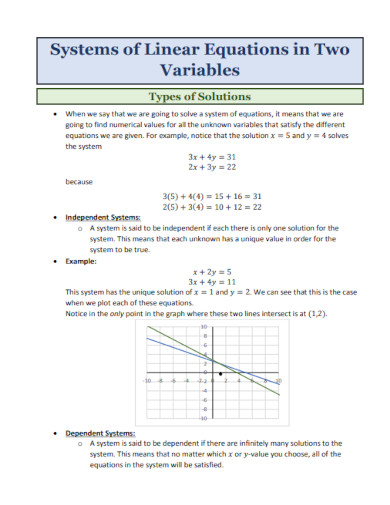
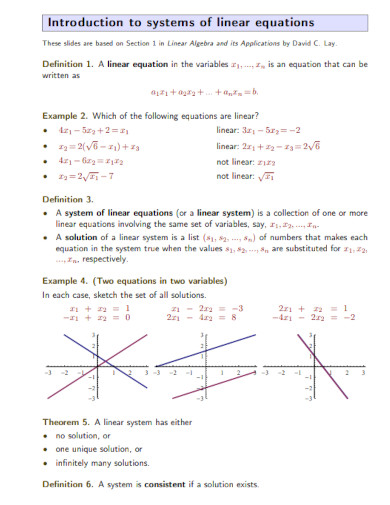
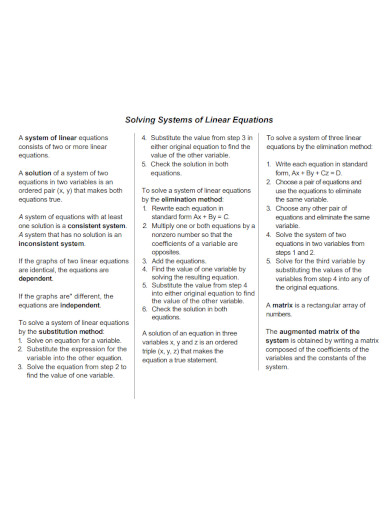
Two linear equations form a system that can be solved together to find the values of variables that satisfy both equations. This can be done through methods like substitution or elimination.
Linear equations are equations and solutions that describe the direct relationship between two variables or values. These two values are often represented by the letters X and Y, which can be solved to obtain four points that will create a straight line.

Lines are illustrations of the relationship between two points in a given plane. This means that the make and the direction are directly affected by the location of the two points. You can easily illustrate this relationship through linear equations and lines.
A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and a single variable. Linear equations can be represented in various forms, but the most common is the standard form: ax+by=c
Equations | Linear or Non-Linear |
|---|---|
y = 8x – 10 | Linear |
y = x² – 22 | Non-Linear, the power of the variable x is 2 |
√y + x = 4 | Non-Linear, the power of the variable y is 1/2 |
y + 8x – 1 = 0 | Linear |
y² – x = 7 | Non-Linear, the power of the variable y is 2 |
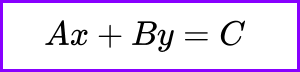
In this form, A, B, and C are constants. The equation describes a line in two-dimensional space, and neither variable is isolated on one side of the equation.

This is perhaps the most frequently used form when dealing with linear equations in basic algebra. Here, m represents the slope of the line, and b represents the y-intercept, which is the point where the line crosses the y-axis. This form makes it easy to graph the equation and to understand how changes in x affect y.
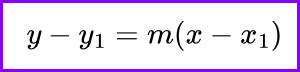
This form is useful when you know a point on the line (x₁,y₁) and the slope m. It’s particularly handy for writing the equation of a line when given a point and the slope without having to calculate the y-intercept.
A, B, and C are real numbers, and A and B are not both zero.
x and y are variables representing the coordinates on a Cartesian plane.
Coefficients are Integers: Typically, the coefficients A, B, and C are written as integers. If they are not integers, they can usually be converted to integers by multiplying the entire equation by an appropriate factor.
Coefficient of x is Non-negative: It’s common practice, though not mandatory, to write the equation with A≥0 . If A is negative, the entire equation can be multiplied by -1 to make A positive.
Intercepts: This form is particularly useful for easily finding the intercepts of the line with the x-axis and y-axis. To find the x-intercept, set y=0 and solve for x. To find the y-intercept, set x=0 and solve for y.

The graph of a linear equation involving one variable, such as x, forms a vertical line that is parallel to the y-axis. Conversely, if the equation involves only the variable y, the graph will be a horizontal line parallel to the x-axis. On the other hand, the graph of a linear equation involving two variables, x and y, forms a straight line. This allows us to visually interpret the relationship between the variables. Let’s explore how to graph a linear equation with two variables through the following example:

Example: Graphing the Linear Equation x−2y=2
To plot the graph of the linear equation in two variables, follow these steps:
Step 1: Identify the equation. The given equation is x−2y=2
Step 2: Convert the equation to slope-intercept form (y = mx + b). Rearrange x−2y=2 to express y in terms of x: y=(x/2)−1
This puts the equation in the form where m (slope) is 1/2 and b (y-intercept) is −1.
Step 3: Calculate coordinates by substituting different values of x.
For x=0:
y=2/0−1=−1
This gives the point (0, -1).
For x=2:
y=2/2−1=0
This gives the point (2, 0).
For x=4:
y=4/2 -1 = 1
This gives the point (4, 1).
For x=−2:
y=-2/2−1=−2
This gives the point (-2, -2).
Step 4: Plot these points and draw the line. List the coordinates and plot them on a Cartesian plane:
(0, -1)
(2, 0)
(4, 1)
(-2, -2)
x | 0 | 2 | 4 | -2 |
|---|---|---|---|---|
y | -1 | 0 | 1 | -2 |
Connect these points with a straight line to visualize the relationship defined by the equation y=x/2−1. Each pair of (x, y) values satisfies the equation, illustrating the linear relationship between x and y.
Definition: A linear equation in one variable is an equation that can be written in the form ax+b=0, where aaa and bbb are constants and x is the variable. The goal is to find the value of x that makes the equation true.
Standard Form: ax+b=0
Steps to Solve:
Isolate the variable: Move all terms involving x to one side of the equation and constants to the other side.
Simplify the equation: Combine like terms if necessary.
Solve for the variable: Divide or multiply to get x by itself.
Example: Solve 3x+5=11
Subtract 5 from both sides: 3x=6
Divide by 3: x=2
Applications:
Solving problems related to distance, speed, and time.
Finding unknown quantities in financial problems.
Definition: A linear equation in two variables is an equation that can be written in the form ax+by+c=0, where a, b, and c are constants, and x and y are variables. This type of equation represents a straight line when graphed on a coordinate plane.
Standard Form: ax+by+c=0
General Form: y=mx+b Where m is the slope and b is the y-intercept.
Methods to Solve:
Graphing:
Plot the equation on a coordinate plane and identify the points where the line intersects the axes.
Substitution Method:
Solve one of the equations for one variable and substitute this value into the other equation.
Elimination Method:
Add or subtract equations to eliminate one variable, making it easier to solve for the remaining variable.

Steps to Solve:
Simplify Both Sides: If necessary, use the distributive property and combine like terms on each side of the equation.
Isolate the Variable: Move all terms involving the variable to one side of the equation and constant terms to the other side.
Solve for the Variable: Divide or multiply to isolate the variable and solve the equation.
Methods to Solve:
Rewrite Equations: Put each equation in the slope-intercept form y=mx+b
Graph Both Equations: Plot the lines on the same coordinate plane.
Find Intersection: The point where the lines intersect is the solution
Solve One Equation for One Variable: Express one variable in terms of the other.
Substitute: Plug this expression into the other equation.
Solve the Resulting Equation: Solve for the remaining variable.
Back-Substitute: Use this value to find the other variable.
Align Equations: Write both equations in standard form.
Eliminate One Variable: Add or subtract equations to cancel out one variable.
Solve for Remaining Variable: Solve the resulting single-variable equation.
Back-Substitute: Use the found value to solve for the other variable.

palmbeachstate.edu

mathcentre.ac.uk
mercury.educ.kent.edu
alamo.edu
math.utah.edu

math.utah.edu

alamo.edu

math.berkeley.edu
math.ucla.ed

map.mathshell.org

people.tamu.edu

map.mathshell.org
mandal.ku.edu
users.math.msu.edu

web.auburn.edu
math.colorado.edu
precalculus-assessment.weebly.com

people.ucsc.edu
mathworks.com
nctm.org

rit.edu

scholarworks.lib.csusb.edu

static.bigideasmath.com

sccollege.edu

wallace.ccfaculty.org
Details
File Format
Size: 32 KB

mgccc.edu
doe.virginia.gov

cpschools.org

bergen.edu
Details
File Format
Size: 49 KB
ucdavis.edu

nctm.org
mayfieldschools.org
Details
File Format
Size: 160 KB

tamiu.edu

math.usm.edu

sites.millersville.edu

globaljournals.org

math.hkust.edu.hk
math.emory.edu
amsi.org.au

lboro.ac.uk
regent.edu

lavc.edu
cazoommaths.com

web.math.princeton.edu

humbleisd.net

courses.washington.edu
cseweb.ucsd.edu
numbertheory.org

digitalcommons.ursinus.edu

math.okstate.edu
sharpschool.com

northmasonschools.org
clarku.edu

sites.math.rutgers.edu
valenciacollege.edu

personal.umd.umich.edu

whatcom.edu

cs.franklin.edu

math.bu.edu

courses.byui.edu
neshaminy.org
arminstraub.com
wccusd.net

faculty.math.illinois.edu

uah.edu

csun.edu

tojsat.net
college.cengage.com
sradai.tripod.com
richlandone.org
images.pcmac.org

nios.ac.in

montgomeryschoolsmd.org

ssag.sk

maths.tcd.ie

cimt.org.uk

mathematicsmagazine.com

in.ixl.com

hetwebsite.net

jmap.org
math.gmu.edu

web.northeastern.edu
hancockcollege.edu

ucl.ac.uk
wiki.math.ntnu.no

mymathclasses.com

bvasd.net

bobprior.com

people.math.harvard.edu

goldenrams.com
esperanzano.org
mfhs.bie.edu

open.lib.umn.edu

faculty.nps.edu
faculty.tru.ca

glendale.edu

cbsd.org
pghschools.org

kutasoftware.com

casdonline.org
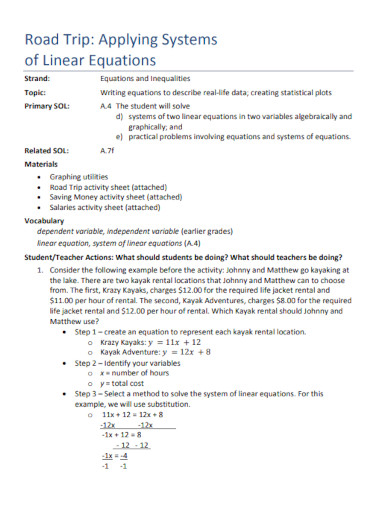
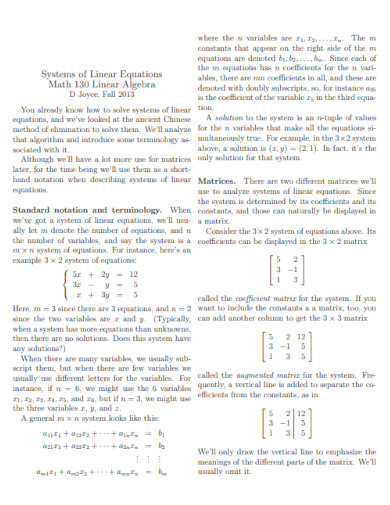
Linear equations are equations that illustrate the proportional relationship between two variables and points. Simple linear equations are equations with a single variable, while there are linear equations with two variables, and even linear equations with three variables. All of which have their systems of linear equations and ways of solving said linear equations.
A can come in many different forms. The standard form of a linear equation with two variables is Ax + By = C where x is the X coordinate, y is the Y coordinate, and C is the constant number. One variable linear equation will come in the form of Ax + B = C.
Begin by writing down the equation in a physical note, or digital note-taking software. This will help you visualize the equation without needing to backread the question.
When you have finished writing down the equation, check whether the equation has one point or two points. You will be able to know this by the presence of X and Y coordinates in the equation. If the equation follows the Ax + By + C = 0 formats, it will require specific X and Y values that you can obtain from a different formula. Only use this how-to with equations that use the standard form of the linear equation or the standard one-variable linear equation.
When you have finished discerning the equation, you must simplify the equation to its simplest form. This means if there is a common denominator between the three variables, then you must divide them by said common denominator.
After you have simplified the equation, you must isolate and move one of the variables to the equals sign. The equation would either come in the form of x = C – B, Ax = C – By, or By = C – Ax.
After isolating one of the points, you must now substitute the isolated variable with specific points. For example, if 2x = 2 – Y is the linear equation, we can substitute the x value to be x = 0. The equation will then become 2(0) = 2 – Y, which will equal to Y = 2.
Repeat these steps until you have up to four sets of X and Y coordinates, note that each set will be a pair. Following the example above, the set of X and Y coordinates will be (0,2). Once you have four sets of these coordinates, graph the linear equation into the cartesian plane using these coordinates as your reference points. Afterward, you will connect all the reference points to create a straight line.
To solve linear equations, isolate the variable by performing inverse operations. Simplify the equation step-by-step, combining like terms and balancing both sides until the variable is isolated.
A linear equation has variables with an exponent of 1 and graphs as a straight line. It typically appears in the form ax+b=c Where a, b, and c are constants.
Linear equations represent relationships where the change between variables is constant. They are expressed in the form ax+by=c, producing straight-line graphs.
A linear equation is an algebraic equation with a constant rate of change between variables, represented as a straight line. Examples: y= 4x+2, 2x+3y = 6
A real-life example of a linear equation is calculating the total cost (C) of items given a fixed price per item (p) and quantity (q): C=pq.
A linear equation is like a simple rule that tells you how to get from one number to another using addition, subtraction, multiplication, or division. For example, if you have 2x+3=7, you find out what number makes it true.
Two linear equations form a system that can be solved together to find the values of variables that satisfy both equations. This can be done through methods like substitution or elimination.
Linear equations are equations and solutions that describe the direct relationship between two variables or values. These two values are often represented by the letters X and Y, which can be solved to obtain four points that will create a straight line.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Solve for x: 2x + 3 = 11
2
3
4
5
Solve for y: 2y − 7 = 132
10
11
12
13
Find x: 4x + 9 = 3x + 15
5
6
7
8
Solve for y: 6y + 5 = 4y + 15
4
5
6
7
Solve for x: 9x − 2 = 4x + 13
1
2
3
4
Find x: 7x + 1 = 3x + 9
1
2
3
4
Solve for x: 6x + 2 = 3x + 11
3
4
5
6
Find y: 4y + 8 = 3y + 12
2
3
4
5
Solve for y: 8y − 3 = 3y + 17
1
2
3
4
Solve for y: 5y + 3 = 2y + 18
2
4
6
8
Before you leave, take our quick quiz to enhance your learning!

