Which of the following best describes mechanical energy?
The energy stored in chemical bonds
The energy associated with the motion and position of an object
The energy stored in atomic nuclei
The energy from electromagnetic waves

Mechanical energy, a cornerstone concept in physics, stands as a critical element in understanding the dynamics of motion and forces. It’s an amalgamation of kinetic and potential energies, seamlessly interplaying within objects in motion or at rest. This guide delves into the essence of mechanical energy, providing clear, comprehensible examples and definitions. Ideal for educators and learners alike, it breaks down complex physics into easily digestible information, fostering a deeper comprehension of this fundamental principle.
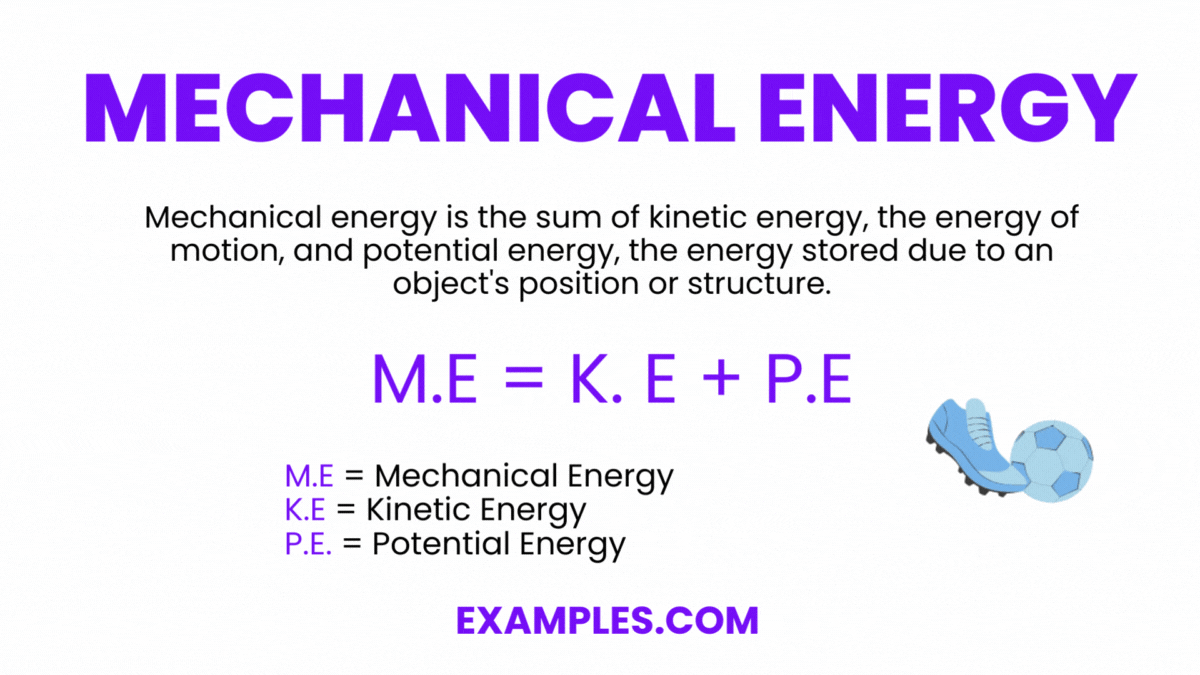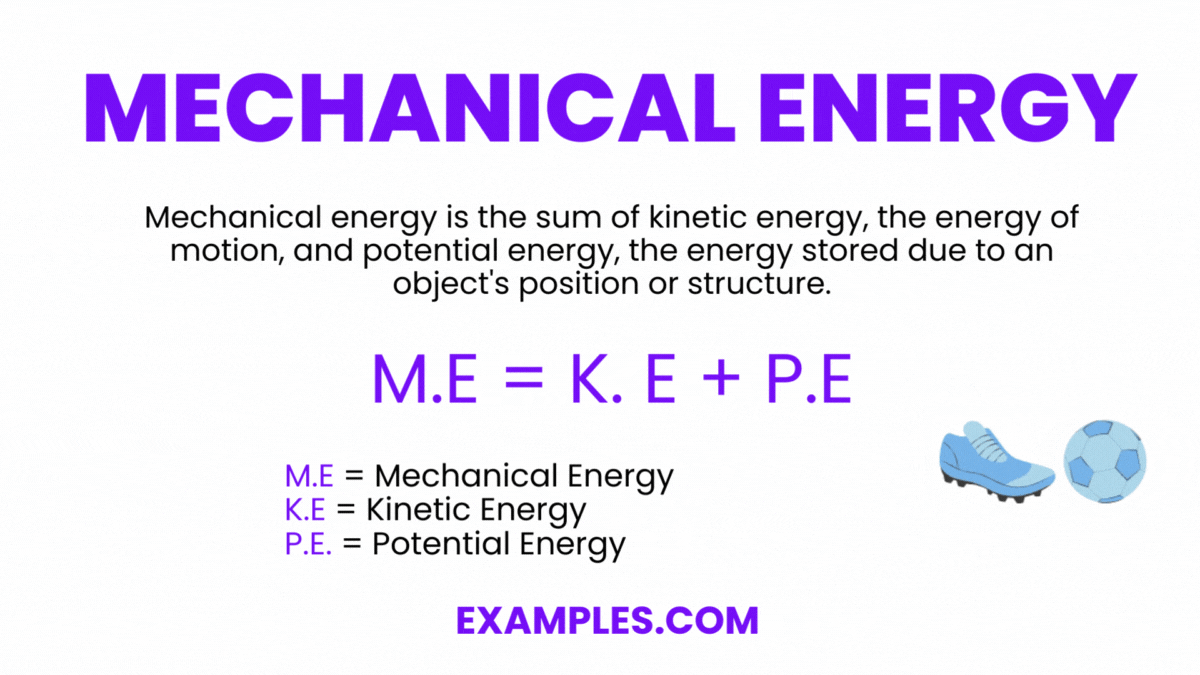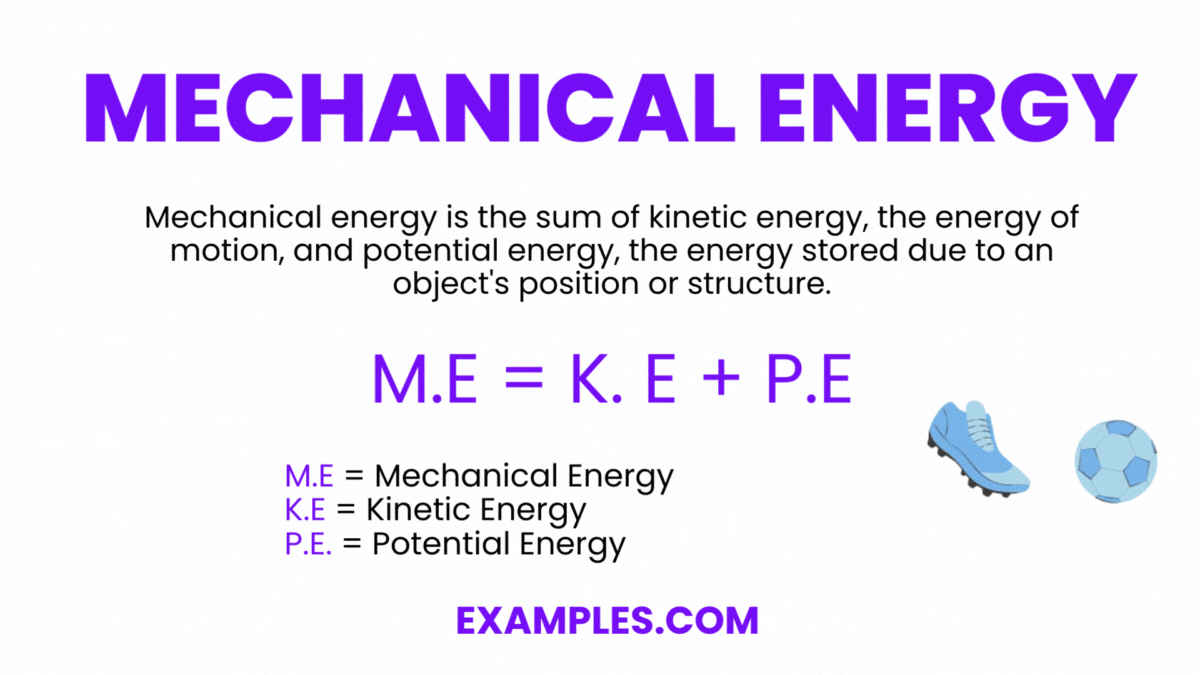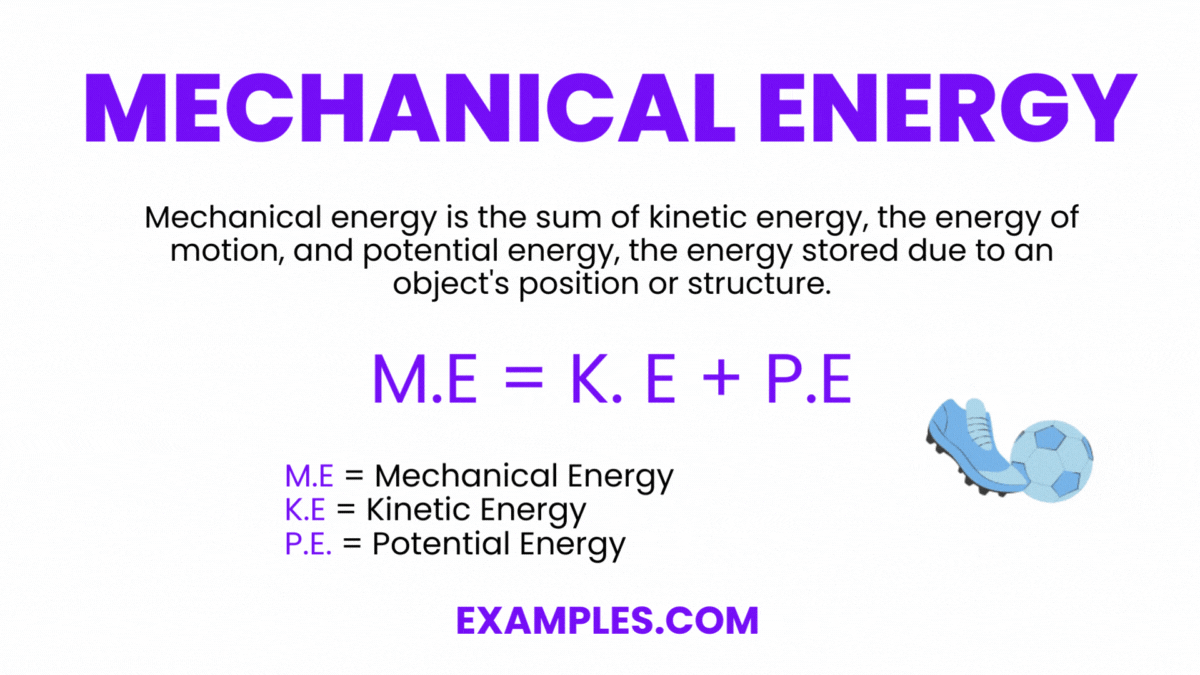
Mechanical energy is the sum of kinetic energy, the energy of motion, and potential energy, the energy stored due to an object’s position or structure. It’s a key concept in physics, illustrating how energy is conserved and transferred between objects and forms. This energy plays a pivotal role in everything from simple daily tasks to complex mechanical systems, making it an essential topic in physics education.
One of the best examples of mechanical energy in action is a roller coaster. As the coaster climbs to the top of a hill, it accumulates potential energy. This energy is due to its elevated position and the gravitational pull. Once the coaster begins its descent, this potential energy converts into kinetic energy, the energy of motion, propelling it along the tracks. This transformation and conservation of energy, from potential to kinetic and vice versa, perfectly illustrate the concept of mechanical energy in a tangible, relatable way.
Mechanical energy, a fundamental concept in physics, can be described as the sum of kinetic energy and potential energy. In simpler terms, it’s the energy associated with motion and position. The formula to calculate mechanical energy is:
M.E = K. E + P.E
{ Mechanical Energy = Kinetic Energy + Potential Energy }
K.E. = 1/2×m×v2
And
P.E. =m×g×h
Hence,
M.E. = 1/2×m×v2+m×g×h
| M.E. | Mechanical Energy |
| K.E. | Kinetic Energy |
| P.E. | Potential Energy |
| m | Mass of the object |
| g | Acceleration due to gravity |
| h | Height position |
| v | The velocity of the object |
Delve into the world of mechanical energy with these 22 unique and vivid examples, each illustrating the remarkable ways in which mechanical energy shapes our world. From everyday occurrences to sophisticated machinery, this collection showcases the versatility and omnipresence of mechanical energy. It serves as an excellent educational resource for teachers aiming to elucidate this fundamental physics concept to students. Through clear, concise explanations, these examples are tailored to enhance understanding and spark curiosity in the realm of physics.
The sources of mechanical energy are diverse and integral to various applications in daily life and industrial processes. They range from natural forces to engineered systems, each playing a crucial role in converting different forms of energy into mechanical energy.
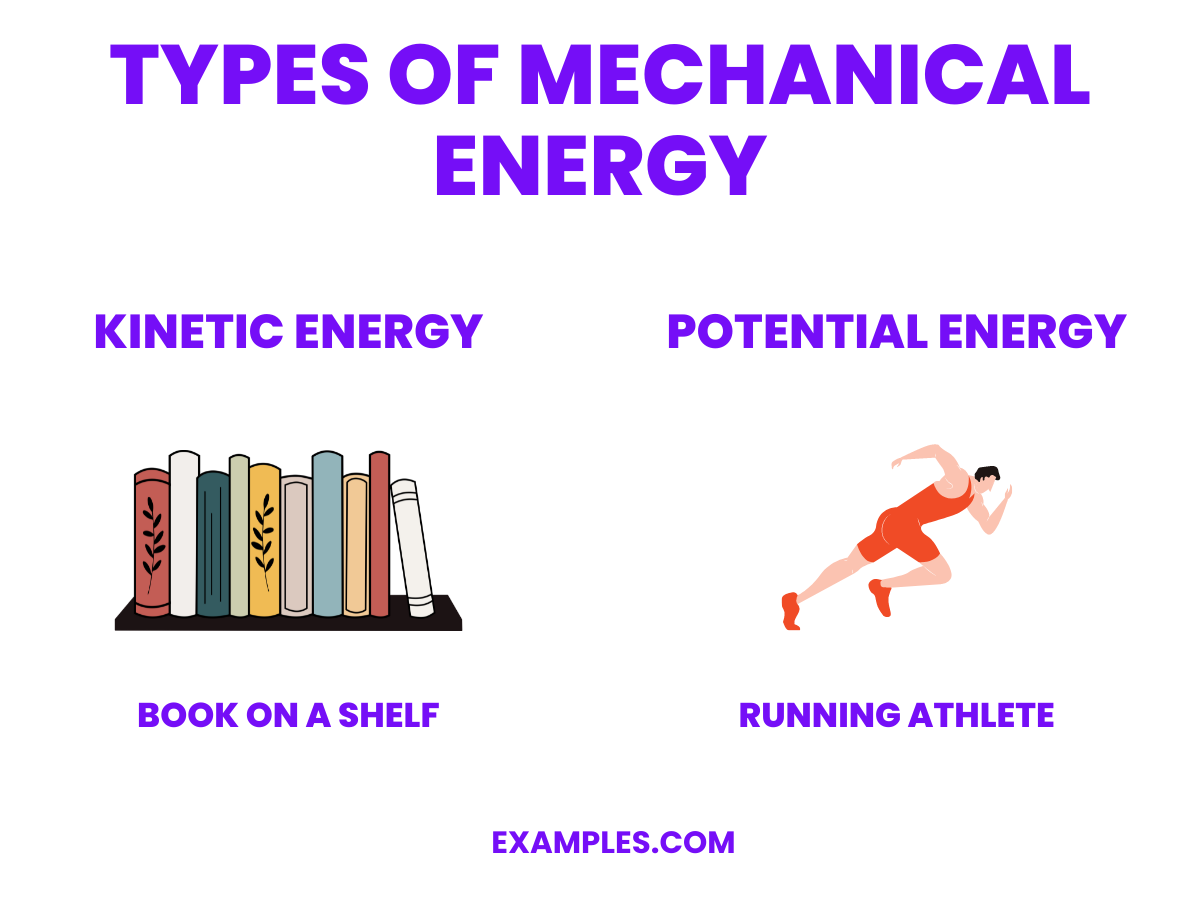
Mechanical energy, pivotal in physics, is classified into two primary types: kinetic energy and Potential Energy. Kinetic Energy is the energy of motion, evident in moving objects, while potential energy is stored energy, present due to an object’s position or structure. This distinction is crucial for understanding various physical phenomena and energy transformations. Here, we explore examples demonstrating each type, offering a practical understanding of these fundamental energy forms.
Kinetic energy is a captivating concept in physics, illustrating energy in motion. It’s essential for teachers and students to grasp this idea through real-world examples. Our list provides a diverse range of instances where kinetic energy is at play, making the concept tangible and easier to understand.
Discover the hidden mechanics of everyday life with these mechanical energy examples. From household chores to recreational activities, mechanical energy is omnipresent, subtly powering our daily routines. This exploration offers insight into the practical application of physics principles, making the abstract concept of mechanical energy tangible and relatable. It’s an invaluable resource for teachers and students, simplifying complex ideas into everyday occurrences.
Engaging and educational, these mechanical energy examples are perfect for young minds. Each example is a playful yet informative demonstration of mechanical energy in action, encouraging kids to observe and understand the physics in their surroundings. This section is crafted to spark curiosity and foster a love for learning among children.
Mechanical energy seamlessly integrates into our daily lives, often unnoticed. This section unveils its presence in routine activities, demonstrating how fundamental physics principles are to our everyday experiences. These examples are carefully chosen to resonate with both teachers and students, simplifying the concept of mechanical energy.
Converting mechanical energy to electrical energy is a process central to many modern technologies. This conversion typically involves using mechanical force to turn a generator, which then produces electricity. This principle underpins much of our power generation infrastructure, from wind turbines to hydroelectric dams.
Producing power from mechanical energy involves a series of steps, each crucial to the transformation of energy from one form to another. This process is fundamental in power generation and mechanical systems.
Mechanical energy follows a very simple formula of (M.E.) = ((1/2)mv^2) + (m × g × h). Where M.E. is mechanical energy (J), m is the mass of the object (kg), v is the velocity of the object (ms^-1), g is the acceleration due to gravity (constant 9.81 m/s^2), and h is the height of the object (m).
Begin by writing down the formula for mechanical energy, so that you have a visual outline of the final form of the equation. The formula should be (M.E.) = ((1/2)mv^2) + (m × g × h).
After you have written the formula for mechanical energy, you will need to list down all the variables given in the problem. Doing this will help you reduce instances of confusion when substituting the values. Not only that but you have to ensure each variable has the correct applicable measurement. If any of the variables are the wrong measurement, convert them accordingly.
You must substitute each variable to the correct value in the mechanical energy equation. After doing that, you will be presented with a missing variable which the equation will try to answer.
When you have solved the equation, you must ensure that the value you answered is the correct measurement value. If the variable you have obtained from the mechanical energy equation is not the asked measurement, convert it accordingly.
People have defined kinetic energy as the energy exerted by an object in motion, which is the juxtaposition of potential energy. An object exerts kinetic energy through actions that involve work and movement. Mechanical energy, on the other hand, is the overarching energy that kinetic and potential are categorized into. This energy refers to both the potential energy and possible kinetic energy a single object can create and manipulate. Mechanical energy is the system that takes into account the potential energy of an object in place and the kinetic energy of an object put to work. In conclusion, kinetic energy deals with a specific part of work, whilst mechanical energy takes into account the whole system.
Following Newton’s third law of motion, wherein Newton states that every object has an equal and opposite reaction, the object exerted upon will move or stay still depending on the exerted mechanical force and energy. This means that an object at work will either push or pull an object of lesser resistance to the direction the moving object is directed at. If the inertia is stronger than the force of the working object, then said object will not move or displace the other object it has impacted.
Mechanical energy is one of the most important and abundant forms of energy that one can easily observe in nature. This makes it very easy for people to utilize and harvest electrical energy through the usage of specialized tools and instruments. The reason why people can do this is because of the law of conservation of energy, which states that energy can never be created nor destroyed, only converted from one form to another. Using this principle, humans have been able to convert mechanical energy into electrical energy through the use of hydropower plants and wind turbines.
Mechanical energy is a type of energy that deals with the motion of an object through the concept of work. This type of energy is very abundant and can be converted into heat or electrical energy, which is used in both exercise and electrical generation. Therefore, it is very important to understand the concept of mechanical energy, even a simple grasp of this concept allows one to know how this energy works and affects our everyday lives.

Mechanical energy, a cornerstone concept in physics, stands as a critical element in understanding the dynamics of motion and forces. It’s an amalgamation of kinetic and potential energies, seamlessly interplaying within objects in motion or at rest. This guide delves into the essence of mechanical energy, providing clear, comprehensible examples and definitions. Ideal for educators and learners alike, it breaks down complex physics into easily digestible information, fostering a deeper comprehension of this fundamental principle.
Mechanical energy is the sum of kinetic energy, the energy of motion, and potential energy, the energy stored due to an object’s position or structure. It’s a key concept in physics, illustrating how energy is conserved and transferred between objects and forms. This energy plays a pivotal role in everything from simple daily tasks to complex mechanical systems, making it an essential topic in physics education.
One of the best examples of mechanical energy in action is a roller coaster. As the coaster climbs to the top of a hill, it accumulates potential energy. This energy is due to its elevated position and the gravitational pull. Once the coaster begins its descent, this potential energy converts into kinetic energy, the energy of motion, propelling it along the tracks. This transformation and conservation of energy, from potential to kinetic and vice versa, perfectly illustrate the concept of mechanical energy in a tangible, relatable way.
Mechanical energy, a fundamental concept in physics, can be described as the sum of kinetic energy and potential energy. In simpler terms, it’s the energy associated with motion and position. The formula to calculate mechanical energy is:
M.E = K. E + P.E
{ Mechanical Energy = Kinetic Energy + Potential Energy }
K.E. = 1/2×m×v2
And
P.E. =m×g×h
Hence,
M.E. = 1/2×m×v2+m×g×h
M.E. | Mechanical Energy |
K.E. | Kinetic Energy |
P.E. | Potential Energy |
m | Mass of the object |
g | Acceleration due to gravity |
h | Height position |
v | The velocity of the object |
Delve into the world of mechanical energy with these 22 unique and vivid examples, each illustrating the remarkable ways in which mechanical energy shapes our world. From everyday occurrences to sophisticated machinery, this collection showcases the versatility and omnipresence of mechanical energy. It serves as an excellent educational resource for teachers aiming to elucidate this fundamental physics concept to students. Through clear, concise explanations, these examples are tailored to enhance understanding and spark curiosity in the realm of physics.
Windmills: Converting wind kinetic energy into mechanical energy for grinding grains or generating electricity.
Bow and Arrow: Storing potential energy in the bent bow, transforming into kinetic energy when released.
Riding a Bicycle: Pedaling creates kinetic energy, moving the bike forward.
Clocks (Pendulum): Potential energy at the highest swing point converts to kinetic energy as it descends.
Hydropower Plants: Water flow’s kinetic energy turns turbines, generating electricity.
Trampolines: Jumping converts kinetic energy to potential energy at peak height.
Cars: Combustion engines convert chemical energy to mechanical energy for movement.
Rubber Bands: Stretching stores potential energy, released as kinetic energy when let go.
Elevators: Electrical energy converts to mechanical energy to move between floors.
Roller Coasters: Potential energy at the top of tracks turns into kinetic energy during descent.
Hammering a Nail: The hammer’s kinetic energy drives the nail into wood.
Basketball Bouncing: The ball’s descent transforms potential energy to kinetic, causing it to bounce.
Springs: Compressing a spring stores potential energy, released as kinetic upon release.
Water Wheels: Flowing water’s kinetic energy rotates the wheel, often used in mills.
Rowing a Boat: Human kinetic energy propels the boat through water.
Skateboarding: Pushing off the ground transfers kinetic energy to the skateboard.
Cranes: Lifting objects converts electrical energy to mechanical for construction tasks.
Golf Swing: Potential energy in the raised club converts to kinetic energy when striking the ball.
Pulley Systems: Used to lift heavy objects by converting kinetic energy of pulling into mechanical lifting force.
Archery: Drawing the bowstring stores potential energy, released as kinetic when the arrow is shot.
Seesaws: Converts kinetic energy of one side to lift the other.
Airplanes: Jet engines convert chemical energy to mechanical to propel forward.
The sources of mechanical energy are diverse and integral to various applications in daily life and industrial processes. They range from natural forces to engineered systems, each playing a crucial role in converting different forms of energy into mechanical energy.
Muscular Effort: Human or animal physical effort producing movement or lifting objects.
Gravity: Waterfalls or downhill slopes converting gravitational potential energy.
Elasticity: Elastic materials like springs and rubber bands storing and releasing energy.
Combustion Engines: Converting chemical energy from fuel into mechanical motion.
Electric Motors: Transforming electrical energy into mechanical rotation.
Wind: Wind turbines converting wind’s kinetic energy into mechanical form.

Mechanical energy, pivotal in physics, is classified into two primary types: kinetic energy and Potential Energy. Kinetic Energy is the energy of motion, evident in moving objects, while potential energy is stored energy, present due to an object’s position or structure. This distinction is crucial for understanding various physical phenomena and energy transformations. Here, we explore examples demonstrating each type, offering a practical understanding of these fundamental energy forms.
A drawn bow: Energy stored due to its stretched position.
A raised hammer: Energy stored when held high, ready to strike.
Water in a reservoir: Elevated water has gravitational potential energy.
A parked car on a hill: Energy due to its elevated position.
A spring in a toy: Compressed spring stores elastic potential energy.
A rock on a mountain edge: Positioned high, it has gravitational potential energy.
A ripe fruit hanging on a tree: Stored energy due to its position.
A book on a shelf: Elevated position contributes to its gravitational potential energy.
A diver on a diving board: Height gives gravitational potential energy.
A stretched rubber band: Contains elastic potential energy.
Kinetic energy is a captivating concept in physics, illustrating energy in motion. It’s essential for teachers and students to grasp this idea through real-world examples. Our list provides a diverse range of instances where kinetic energy is at play, making the concept tangible and easier to understand.
Throwing a Ball: Energy transferred to the ball during the throw.
Riding a Bicycle: The cyclist’s movement generates kinetic energy.
Flowing River: Water moving downstream embodies this energy.
Running Athlete: Energy is evident in the athlete’s motion.
Flying Airplane: The aircraft in flight is a classic example.
Rolling Bowling Ball: Demonstrates energy in a bowling alley.
Moving Car: Shows how engines convert fuel into motion.
Spinning Wind Turbine: Converts wind’s kinetic energy into electricity.
Falling Rocks: Energy increases as they accelerate downward.
Jumping Kangaroo: A lively illustration of muscular energy in motion.
Discover the hidden mechanics of everyday life with these mechanical energy examples. From household chores to recreational activities, mechanical energy is omnipresent, subtly powering our daily routines. This exploration offers insight into the practical application of physics principles, making the abstract concept of mechanical energy tangible and relatable. It’s an invaluable resource for teachers and students, simplifying complex ideas into everyday occurrences.
Opening a Door: The energy used to push or pull a door comes from converting your kinetic energy.
Using Scissors: Hand movement creates kinetic energy, enabling the scissors to cut through materials.
Mopping the Floor: The mop converts your kinetic energy into mechanical energy to clean surfaces.
Jumping on a Bed: Jumping converts your kinetic energy to potential energy at the peak of the jump.
Playing on a Swing: Swinging involves a continuous conversion between kinetic and potential energy.
Pushing a Shopping Cart: Exerting force to push the cart converts kinetic energy into mechanical, facilitating movement.
Using a Can Opener: The hand’s rotational movement transfers kinetic energy to the opener, cutting the can.
Sweeping the Floor: The broom transforms the kinetic energy of your arms into mechanical energy to sweep debris.
Flipping Pancakes: Lifting and turning the pancake involves converting kinetic energy into mechanical to cook evenly.
Pumping Air into a Bicycle Tire: The hand pump converts kinetic energy into mechanical energy, inflating the tire
Engaging and educational, these mechanical energy examples are perfect for young minds. Each example is a playful yet informative demonstration of mechanical energy in action, encouraging kids to observe and understand the physics in their surroundings. This section is crafted to spark curiosity and foster a love for learning among children.
Spinning a Top: Twisting and releasing the top transforms kinetic energy into mechanical, making it spin.
Jumping Rope: Swinging the rope and jumping converts kinetic energy to mechanical, keeping the rope in motion.
Playing on a See-Saw: The up and down movement on a see-saw is a perfect example of mechanical energy transfer.
Using a Slinky: A slinky demonstrates mechanical energy as it stretches and recoils, moving down steps.
Building with Blocks: Stacking blocks involves transferring kinetic energy from hands to create structures.
Riding a Scooter: Pushing off the ground transfers kinetic energy to the scooter, propelling it forward.
Bouncing a Ball: The ball’s descent transforms gravitational potential energy into kinetic energy, causing it to bounce.
Using a Slide: Sliding down converts potential energy (due to height) into kinetic energy.
Flying a Kite: The kite in the air has potential energy, which varies with its height and wind strength.
Playing with Toy Cars: Pushing the car converts your kinetic energy to the car’s mechanical energy.
Mechanical energy seamlessly integrates into our daily lives, often unnoticed. This section unveils its presence in routine activities, demonstrating how fundamental physics principles are to our everyday experiences. These examples are carefully chosen to resonate with both teachers and students, simplifying the concept of mechanical energy.
Walking or Running: Your body converts metabolic energy into mechanical energy for movement.
Using a Blender: Electrical energy is transformed into mechanical energy to rotate the blades.
Washing Hands: Moving your hands creates kinetic energy, aiding in cleaning.
Climbing Stairs: Ascending converts kinetic energy into potential energy due to increased height.
Typing on a Keyboard: Pressing keys is a transfer of kinetic energy from fingers to the computer.
Opening and Closing Curtains: Drawing curtains utilizes mechanical energy to control light and privacy.
Stirring a Pot of Soup: The stirring motion transfers kinetic energy to the spoon, mixing the soup.
Winding a Watch: Turning the watch’s knob stores mechanical energy in its springs, powering its mechanism.
Shaking a Snow Globe: Shaking it transfers kinetic energy, creating the snowfall effect.
Squeezing a Stress Ball: Compressing the ball stores mechanical energy, which is released when the grip is loosened.
Converting mechanical energy to electrical energy is a process central to many modern technologies. This conversion typically involves using mechanical force to turn a generator, which then produces electricity. This principle underpins much of our power generation infrastructure, from wind turbines to hydroelectric dams.
Producing power from mechanical energy involves a series of steps, each crucial to the transformation of energy from one form to another. This process is fundamental in power generation and mechanical systems.
Generation of Mechanical Energy: This could be through natural forces like wind or water flow, or through artificial means like engines.
Transmission of Mechanical Force: The mechanical energy is then transmitted to a generator or a similar device.
Conversion to Electrical Energy: Inside the generator, the mechanical energy turns a turbine, which then drives a generator to produce electricity.
Regulation and Distribution: The generated electricity is regulated to ensure a consistent power output and then distributed through the power grid.
Utilization: Finally, this electricity is used to power various devices and systems, completing the transformation from mechanical to electrical energy.
Mechanical energy follows a very simple formula of (M.E.) = ((1/2)mv^2) + (m × g × h). Where M.E. is mechanical energy (J), m is the mass of the object (kg), v is the velocity of the object (ms^-1), g is the acceleration due to gravity (constant 9.81 m/s^2), and h is the height of the object (m).
Begin by writing down the formula for mechanical energy, so that you have a visual outline of the final form of the equation. The formula should be (M.E.) = ((1/2)mv^2) + (m × g × h).
After you have written the formula for mechanical energy, you will need to list down all the variables given in the problem. Doing this will help you reduce instances of confusion when substituting the values. Not only that but you have to ensure each variable has the correct applicable measurement. If any of the variables are the wrong measurement, convert them accordingly.
You must substitute each variable to the correct value in the mechanical energy equation. After doing that, you will be presented with a missing variable which the equation will try to answer.
When you have solved the equation, you must ensure that the value you answered is the correct measurement value. If the variable you have obtained from the mechanical energy equation is not the asked measurement, convert it accordingly.
People have defined kinetic energy as the energy exerted by an object in motion, which is the juxtaposition of potential energy. An object exerts kinetic energy through actions that involve work and movement. Mechanical energy, on the other hand, is the overarching energy that kinetic and potential are categorized into. This energy refers to both the potential energy and possible kinetic energy a single object can create and manipulate. Mechanical energy is the system that takes into account the potential energy of an object in place and the kinetic energy of an object put to work. In conclusion, kinetic energy deals with a specific part of work, whilst mechanical energy takes into account the whole system.
Following Newton’s third law of motion, wherein Newton states that every object has an equal and opposite reaction, the object exerted upon will move or stay still depending on the exerted mechanical force and energy. This means that an object at work will either push or pull an object of lesser resistance to the direction the moving object is directed at. If the inertia is stronger than the force of the working object, then said object will not move or displace the other object it has impacted.
Mechanical energy is one of the most important and abundant forms of energy that one can easily observe in nature. This makes it very easy for people to utilize and harvest electrical energy through the usage of specialized tools and instruments. The reason why people can do this is because of the law of conservation of energy, which states that energy can never be created nor destroyed, only converted from one form to another. Using this principle, humans have been able to convert mechanical energy into electrical energy through the use of hydropower plants and wind turbines.
Mechanical energy is a type of energy that deals with the motion of an object through the concept of work. This type of energy is very abundant and can be converted into heat or electrical energy, which is used in both exercise and electrical generation. Therefore, it is very important to understand the concept of mechanical energy, even a simple grasp of this concept allows one to know how this energy works and affects our everyday lives.
Text prompt
Add Tone
Mechanical Energy Formula
22 Mechanical Energy Examples
Which of the following best describes mechanical energy?
The energy stored in chemical bonds
The energy associated with the motion and position of an object
The energy stored in atomic nuclei
The energy from electromagnetic waves
What is the kinetic energy of a 2 kg object moving at a speed of 3 m/s?
3 J
6 J
9 J
18 J
Which of the following is NOT a type of potential energy?
Gravitational potential energy
Elastic potential energy
Chemical potential energy
Thermal potential energy
An object is moving with a constant speed in a circular path. Which type of energy remains constant?
Kinetic energy
Potential energy
Total mechanical energy
Both A and C
Which of the following is an example of converting potential energy into kinetic energy?
A car accelerating on a flat road
A ball rolling up a hill
A rock falling off a cliff
A person sitting still
The work done on an object is equal to which of the following?
The change in its potential energy
The change in its kinetic energy
The change in its mechanical energy
All of the above
A pendulum has maximum potential energy at which point in its swing?
At the lowest point
At the highest point
At the midpoint
At the equilibrium point
What happens to the kinetic energy of a moving object if its mass is doubled while its velocity remains constant?
It remains the same
It is halved
It is doubled
It is quadrupled
A 0.5 kg ball is thrown vertically upwards with an initial velocity of 10 m/s. What is its kinetic energy at the moment of release?
5 J
25 J
50 J
100 J
What is the relationship between work and mechanical energy?
Work is equal to the change in mechanical energy
Work is the potential energy of an object
Work is always greater than mechanical energy
Work is unrelated to mechanical energy
Before you leave, take our quick quiz to enhance your learning!

