What is the cube root of 27?
3
6
9
12
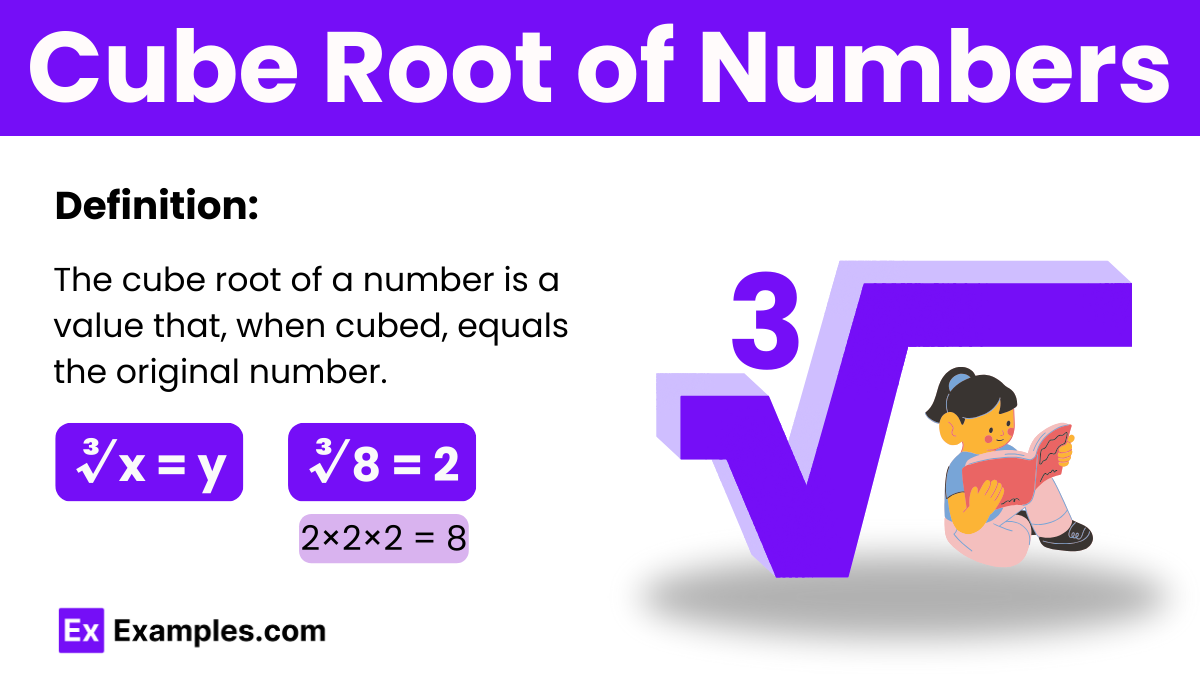
The cube root of a number is a value that, when multiplied by itself two more times, yields the original number. For instance, the cube root of 27, expressed as 3√27, is 3 because multiplying 3 by itself twice more (3 x 3 x 3) equals 27, or 3³. This process reveals the original value that was cubed, indicating that 27 is a perfect cube. The term “cube root” itself suggests the root cause of the cube. To determine the cube root of perfect cubes, the prime factorization method is typically employed, illustrating the concept’s significance akin to that of the square root.
The cube root symbol is denoted as ³√. This notation is used to represent the cube root of a number, which is placed inside the bracket. For example, ³√27 signifies the cube root of 27.
The cube root formula is a way to find out which number was multiplied by itself three times to get a certain number, and it’s written with a special symbol, ³√. To find the cube root, you can start by breaking down the number into its prime factors. Then, use the cube root formula. If we have a number x, and x=y×y×y, then the cube root of x is simply ³√x=y. This means y is the cube root of x. If y turns out to be a whole number, then x is called a perfect cube.
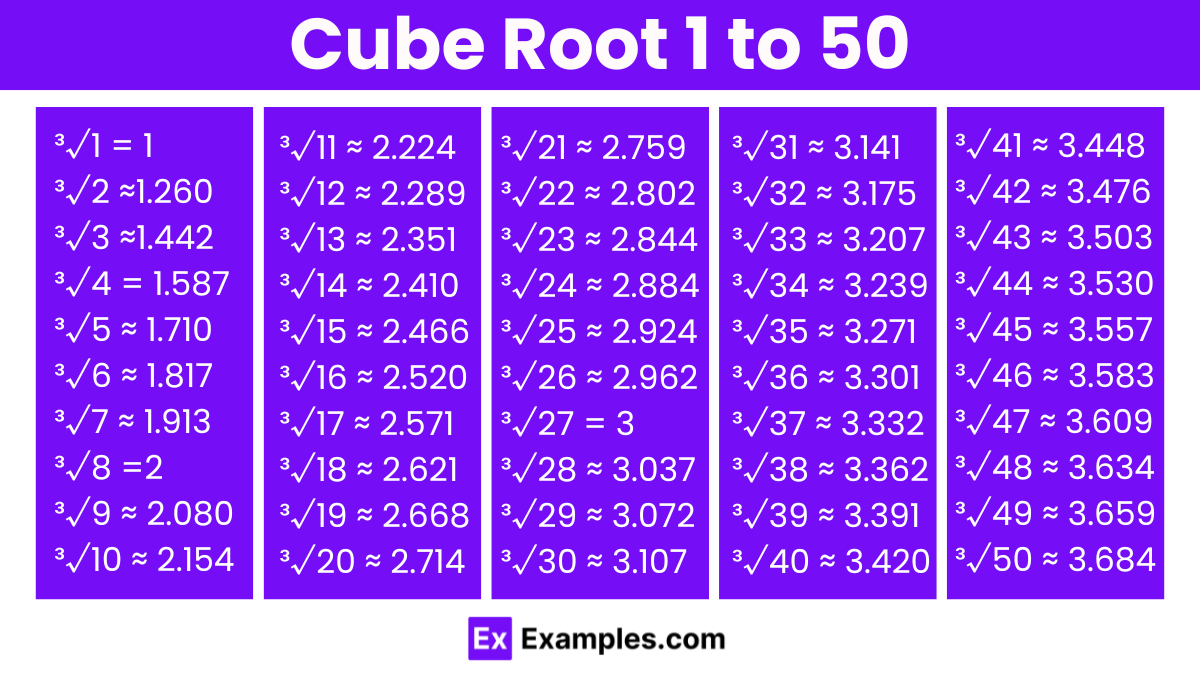
| Number | Cube Root | Number | Cube Root | Number | Cube Root |
|---|---|---|---|---|---|
| ³√1 | 1.000 | ³√18 | 2.621 | ³√35 | 3.271 |
| ³√2 | 1.260 | ³√19 | 2.668 | ³√36 | 3.302 |
| ³√3 | 1.442 | ³√20 | 2.714 | ³√37 | 3.332 |
| ³√4 | 1.587 | ³√21 | 2.759 | ³√38 | 3.362 |
| ³√5 | 1.710 | ³√22 | 2.802 | ³√39 | 3.391 |
| ³√6 | 1.817 | ³√23 | 2.844 | ³√40 | 3.420 |
| ³√7 | 1.913 | ³√24 | 2.884 | ³√41 | 3.448 |
| ³√8 | 2.000 | ³√25 | 2.924 | ³√42 | 3.476 |
| ³√9 | 2.080 | ³√26 | 2.962 | ³√43 | 3.503 |
| ³√10 | 2.154 | ³√27 | 3.000 | ³√44 | 3.530 |
| ³√11 | 2.224 | ³√28 | 3.037 | ³√45 | 3.557 |
| ³√12 | 2.289 | ³√29 | 3.072 | ³√46 | 3.583 |
| ³√13 | 2.351 | ³√30 | 3.107 | ³√47 | 3.609 |
| ³√14 | 2.410 | ³√31 | 3.141 | ³√48 | 3.634 |
| ³√15 | 2.466 | ³√32 | 3.175 | ³√49 | 3.659 |
| ³√16 | 2.520 | ³√33 | 3.207 | ³√50 | 3.684 |
| ³√17 | 2.571 | ³√34 | 3.239 |
To find the cube root of 27:
The cube root of 64 is 4. This is because multiplying 4 by itself three times (4x4x4) equals 64.
The cube root of 27 is 3. This is determined by the fact that 3 multiplied by itself three times (3x3x3) equals 27.

The cube root of a number is a value that, when multiplied by itself two more times, yields the original number. For instance, the cube root of 27, expressed as 3√27, is 3 because multiplying 3 by itself twice more (3 x 3 x 3) equals 27, or 3³. This process reveals the original value that was cubed, indicating that 27 is a perfect cube. The term “cube root” itself suggests the root cause of the cube. To determine the cube root of perfect cubes, the prime factorization method is typically employed, illustrating the concept’s significance akin to that of the square root.
The cube root of a number is the value that, when multiplied by itself three times (or cubed), results in the original number. It answers the question, “Which number, when cubed, gives the current value?” For example, the cube root of 8, denoted as 8³, is 2, because 2×2×2=8. This concept is crucial in mathematics for finding the original base value of a cube number. Identifying the cube root is especially relevant for working with volumes in geometry, where understanding the dimensions of cubic shapes is necessary. The cube root symbol is represented as 3³, with the number whose cube root is being sought placed inside the bracket.
The cube root symbol is denoted as ³√. This notation is used to represent the cube root of a number, which is placed inside the bracket. For example, ³√27 signifies the cube root of 27.
The cube root formula is a way to find out which number was multiplied by itself three times to get a certain number, and it’s written with a special symbol, ³√. To find the cube root, you can start by breaking down the number into its prime factors. Then, use the cube root formula. If we have a number x, and x=y×y×y, then the cube root of x is simply ³√x=y. This means y is the cube root of x. If y turns out to be a whole number, then x is called a perfect cube.
Cuberoot of x= ³√x= ³√(y×y×y) = y
Number | Cube Root | Number | Cube Root | Number | Cube Root |
|---|---|---|---|---|---|
³√1 | 1.000 | ³√18 | 2.621 | ³√35 | 3.271 |
³√2 | 1.260 | ³√19 | 2.668 | ³√36 | 3.302 |
³√3 | 1.442 | ³√20 | 2.714 | ³√37 | 3.332 |
³√4 | 1.587 | ³√21 | 2.759 | ³√38 | 3.362 |
³√5 | 1.710 | ³√22 | 2.802 | ³√39 | 3.391 |
³√6 | 1.817 | ³√23 | 2.844 | ³√40 | 3.420 |
³√7 | 1.913 | ³√24 | 2.884 | ³√41 | 3.448 |
³√8 | 2.000 | ³√25 | 2.924 | ³√42 | 3.476 |
³√9 | 2.080 | ³√26 | 2.962 | ³√43 | 3.503 |
³√10 | 2.154 | ³√27 | 3.000 | ³√44 | 3.530 |
³√11 | 2.224 | ³√28 | 3.037 | ³√45 | 3.557 |
³√12 | 2.289 | ³√29 | 3.072 | ³√46 | 3.583 |
³√13 | 2.351 | ³√30 | 3.107 | ³√47 | 3.609 |
³√14 | 2.410 | ³√31 | 3.141 | ³√48 | 3.634 |
³√15 | 2.466 | ³√32 | 3.175 | ³√49 | 3.659 |
³√16 | 2.520 | ³√33 | 3.207 | ³√50 | 3.684 |
³√17 | 2.571 | ³√34 | 3.239 |
Break Down into Prime Factors: Start by breaking the number down into its prime factors. Prime factorization involves dividing the number into its basic building blocks—prime numbers that, when multiplied together, give the original number.
Group the Factors: Group these prime factors into sets of three identical numbers. Each set represents the cubed factor.
Multiply One from Each Group: Take one number from each group and multiply them together. This product is the cube root of the original number.
Apply the Cube Root Symbol: For simpler calculations or with the help of a calculator, you can directly apply the cube root symbol (³√x) to the number. This directly gives you the cube root without the need for factorization.
To find the cube root of 27:
Prime Factorize 27: 27=3×3×3
Group the Factors: There is one group of three 3s.
Take the Cube Root: The cube root of 27 is 3, because 3×3×3=27.
Estimate: For numbers that are not perfect cubes or when you’re without a calculator, estimate by finding the two closest perfect cube numbers it falls between. The cube root of your number will be between the cube roots of these two perfect cubes.
Perfect cubes are numbers like 8, 27, 64, etc., where the cube roots are whole numbers (2, 3, 4, respectively).
For non-perfect cubes, the result may be a decimal or irrational number, which often requires a calculator for precise calculation.
The cube root of 64 is 4. This is because multiplying 4 by itself three times (4x4x4) equals 64.
The cube root of 27 is 3. This is determined by the fact that 3 multiplied by itself three times (3x3x3) equals 27.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the cube root of 27?
3
6
9
12
Find the cube root of 64.
2
4
6
8
What is the cube root of 125?
5
10
15
20
Find the cube root of 216.
5
6
7
8
What is the cube root of 343?
7
14
21
29
Find the cube root of 512.
8
16
24
32
What is the cube root of 729?
7
8
9
10
Find the cube root of 1000.
10
20
30
40
What is the cube root of 1331?
11
22
33
44
Find the cube root of 1728.
12
24
32
48
Before you leave, take our quick quiz to enhance your learning!

