What are the factors of 19?
1 and 19
1, 19, and 2
1, 19, and 3
1, 19, and 5
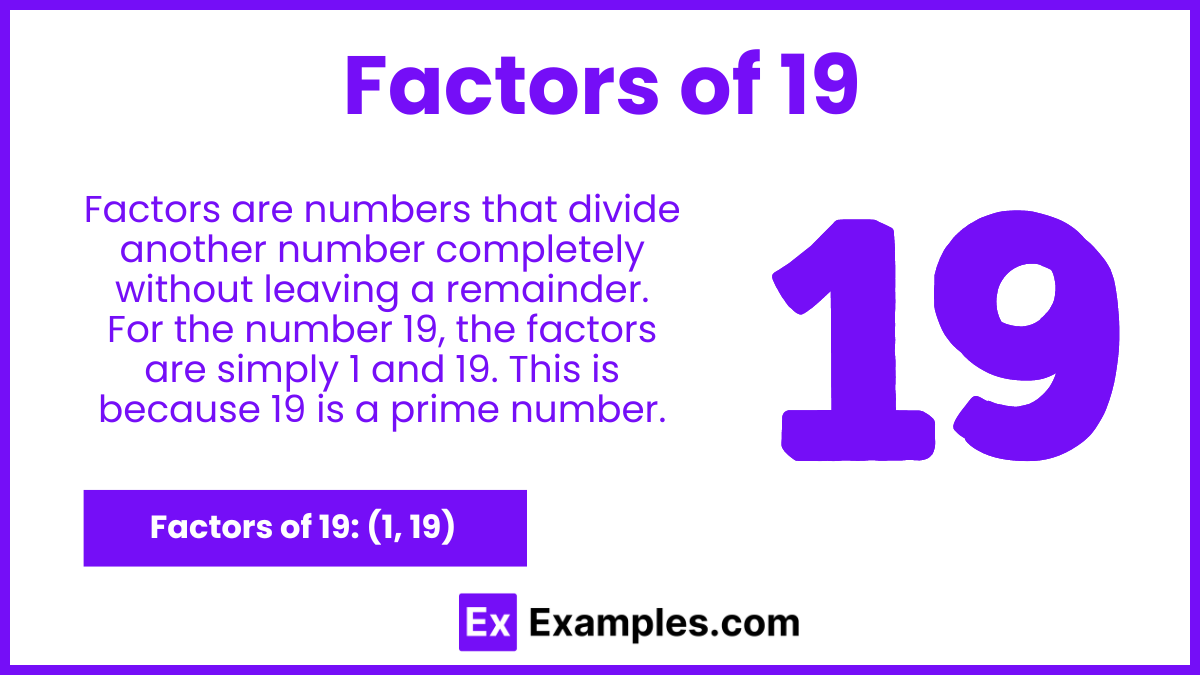
The factors of a number is fundamental in mathematics. For the number 19, this task is straightforward yet interesting due to its prime nature. A prime number is defined as a number greater than 1 that has no positive divisors other than 1 and itself. Hence, the factors of 19 are simply 1 and 19. This unique property makes 19 an essential example when studying prime numbers and their characteristics. Whether you’re a student or just curious about mathematics, knowing the factors of 19 helps in grasping the broader concept of number theory and prime numbers.

The factors of 19 are quite straightforward due to its classification as a prime number. A prime number is one that can only be divided by 1 and itself without leaving a remainder. Therefore, the only factors of 19 are 1 and 19. This unique property distinguishes prime numbers from composite numbers, which have more than two factors. Understanding the factors of 19 not only highlights its prime nature but also reinforces the fundamental principles of number theory.
Calculating the prime factors of a number involves determining the prime numbers that multiply together to give the original number. Prime numbers are those that have no divisors other than 1 and themselves. Since 19 is a prime number, its prime factorization is straightforward. Here’s how you can calculate the prime factors of 19.
The factors of a number is essential in mathematics, especially when dealing with prime numbers like 19. Factors are numbers that divide another number exactly without leaving a remainder. Since 19 is a prime number, it has unique properties that simplify its factorization. Here are some tips to help you understand and work with the factors of 19.
The prime factorization of 19 is simply 19, as it is a prime number.
The sum of the factors of 19 is 1 + 19 = 20.
The greatest common factor of 19 and 38 is 19, as 19 is a factor of 38.
Yes, the factors of 19 are positive numbers. In standard factorization, only positive factors are considered.
Yes, 19 can be a common factor in composite numbers if it is one of their prime factors. For example, 38 is a composite number with 19 as a factor.
The positive pair factors of 19 are (1, 19). The negative pair factors are (-1, -19). These pairs multiply to give 19, reflecting its prime nature.

The factors of a number is fundamental in mathematics. For the number 19, this task is straightforward yet interesting due to its prime nature. A prime number is defined as a number greater than 1 that has no positive divisors other than 1 and itself. Hence, the factors of 19 are simply 1 and 19. This unique property makes 19 an essential example when studying prime numbers and their characteristics. Whether you’re a student or just curious about mathematics, knowing the factors of 19 helps in grasping the broader concept of number theory and prime numbers.

The factors of 19 are quite straightforward due to its classification as a prime number. A prime number is one that can only be divided by 1 and itself without leaving a remainder. Therefore, the only factors of 19 are 1 and 19. This unique property distinguishes prime numbers from composite numbers, which have more than two factors. Understanding the factors of 19 not only highlights its prime nature but also reinforces the fundamental principles of number theory.
The factor pairs of 19 are: (1, 19)
As 19 is a prime number, it has only one pair of factors.
These pairs represent the two numbers that multiply together to equal 19.
Factor pairs help in understanding the divisibility and properties of numbers.
Calculating the prime factors of a number involves determining the prime numbers that multiply together to give the original number. Prime numbers are those that have no divisors other than 1 and themselves. Since 19 is a prime number, its prime factorization is straightforward. Here’s how you can calculate the prime factors of 19.
Recognize that a prime factor is a prime number that divides another number exactly, without leaving a remainder.
Start with the smallest prime number, which is 2. Since 19 is an odd number, it cannot be divided by 2.
Move to the next prime numbers: 3, 5, 7, 11, 13, and so on.
Check if 19 is divisible by any of these numbers.
Divide 19 by each of these prime numbers. Since none of them divide 19 exactly, it confirms that 19 is not divisible by any other number except 1 and itself.
Since 19 cannot be divided by any prime numbers other than itself, conclude that 19 is a prime number.
Therefore, the only prime factor of 19 is 19 itself.
Problem: Determine the factors of 19.
Solution: The factors of 19 are the numbers that divide 19 exactly without leaving a remainder. These numbers are 1 and 19.
Answer: 1, 19
Problem: Find the sum of all factors of 19.
Solution: The factors of 19 are 1 and 19. Add these factors together: 1 + 19 = 20.
Answer: 20
Problem: Calculate the product of all factors of 19.
Solution: The factors of 19 are 1 and 19. Multiply these factors: 1 × 19 = 19.
Answer: 19
Problem: Perform the prime factorization of 19.
Solution: Since 19 is a prime number, it has no other factors besides 1 and itself. The prime factorization of 19 is simply 19.
Answer: 19
Problem: Verify if 19 is divisible by any number other than 1 and itself.
Solution: To check divisibility, divide 19 by other prime numbers less than 19 (e.g., 2, 3, 5, 7, 11, 13, 17). Since 19 is not divisible by any of these numbers, it confirms that 19 is a prime number.
Answer: 19 is only divisible by 1 and 19.
The factors of a number is essential in mathematics, especially when dealing with prime numbers like 19. Factors are numbers that divide another number exactly without leaving a remainder. Since 19 is a prime number, it has unique properties that simplify its factorization. Here are some tips to help you understand and work with the factors of 19.
Recognize that 19 is a prime number, meaning it has exactly two distinct factors: 1 and 19.
Since 19 is prime, you don’t need to check for multiple factors; just remember its only factors are 1 and 19.
The prime factorization of 19 is simple: it’s just 19, with no other prime numbers involved.
To confirm a number is prime, check if it’s divisible by any smaller prime numbers (e.g., 2, 3, 5, 7, etc.). If none divide it without a remainder, the number is prime.
Use the knowledge of 19’s factors in mathematical problems, such as simplifying fractions, finding common denominators, and solving equations where 19 might be a factor.
Knowing the factors of 19 can help in understanding its role in prime factorization problems and number theory.
Recognize that 19, being a prime number, cannot be broken down into smaller factors, simplifying many mathematical operations.
Apply the concept of prime factors in real-world problems, such as cryptography, where prime numbers play a crucial role in algorithms and encryption techniques.
The prime factorization of 19 is simply 19, as it is a prime number.
The sum of the factors of 19 is 1 + 19 = 20.
The greatest common factor of 19 and 38 is 19, as 19 is a factor of 38.
Yes, the factors of 19 are positive numbers. In standard factorization, only positive factors are considered.
Yes, 19 can be a common factor in composite numbers if it is one of their prime factors. For example, 38 is a composite number with 19 as a factor.
The positive pair factors of 19 are (1, 19). The negative pair factors are (-1, -19). These pairs multiply to give 19, reflecting its prime nature.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What are the factors of 19?
1 and 19
1, 19, and 2
1, 19, and 3
1, 19, and 5
Which of the following numbers is a factor of 19?
2
5
19
30
Is 1 a factor of 19?
Yes
No
Only in specific cases
Not sure
Identify the prime factors of 19.
1 and 19
Only 19
2, 3, and 19
1, 2, and 19
How many factors does 19 have?
1
2
3
4
Which of the following statements is true about the factors of 19?
19 has more than two factors.
19 has only one factor.
19 has exactly two factors.
19 has three factors.
Can 19 be divided exactly by any number other than 1 and 19?
Yes
No
Sometimes
Only by even numbers
Is 19 a composite number?
Yes
No
Sometimes
Only in certain cases
Which pair of numbers are the factors of 19?
1 and 9
1 and 19
2 and 19
1 and 3
What makes 19 a prime number?
It has more than two factors.
It has only one factor.
It has exactly two factors.
It is an odd number.
Before you leave, take our quick quiz to enhance your learning!

