Pre-Algebra

Last Updated: August 23, 2024

Notes

Pre-algebra serves as the foundational stepping stone between basic arithmetic and the more complex world of algebra. It introduces students to the fundamental concepts and skills that are necessary for success in algebra and subsequent higher-level mathematics courses. In pre-algebra, students develop an understanding of numbers, their properties, and the operations that can be performed on them. This course typically covers topics such as integers, fractions, decimals, ratios, proportions, percentages, and basic equations. By mastering these topics, students gain the confidence and competence required to tackle more advanced mathematical challenges.

Learning Objectives

The learning objectives for pre-algebra encompass a broad spectrum of mathematical skills and concepts that are essential for success in higher-level math courses. Students will learn to understand and perform operations with integers, fractions, and decimals; solve basic equations and inequalities; interpret and analyze ratios, proportions, and percentages; and apply these concepts to real-world problems. Additionally, students will develop critical thinking and problem-solving skills, enabling them to approach mathematical challenges with confidence and competence. Mastery of pre-algebra lays the groundwork for future success in algebra, geometry, and beyond, fostering a deep appreciation for the logical structure and beauty of mathematics.

Pre-Algebra is a crucial foundation for the ACT Math section. This guide covers essential concepts, definitions, and examples to help you master pre-algebra and achieve a high score.

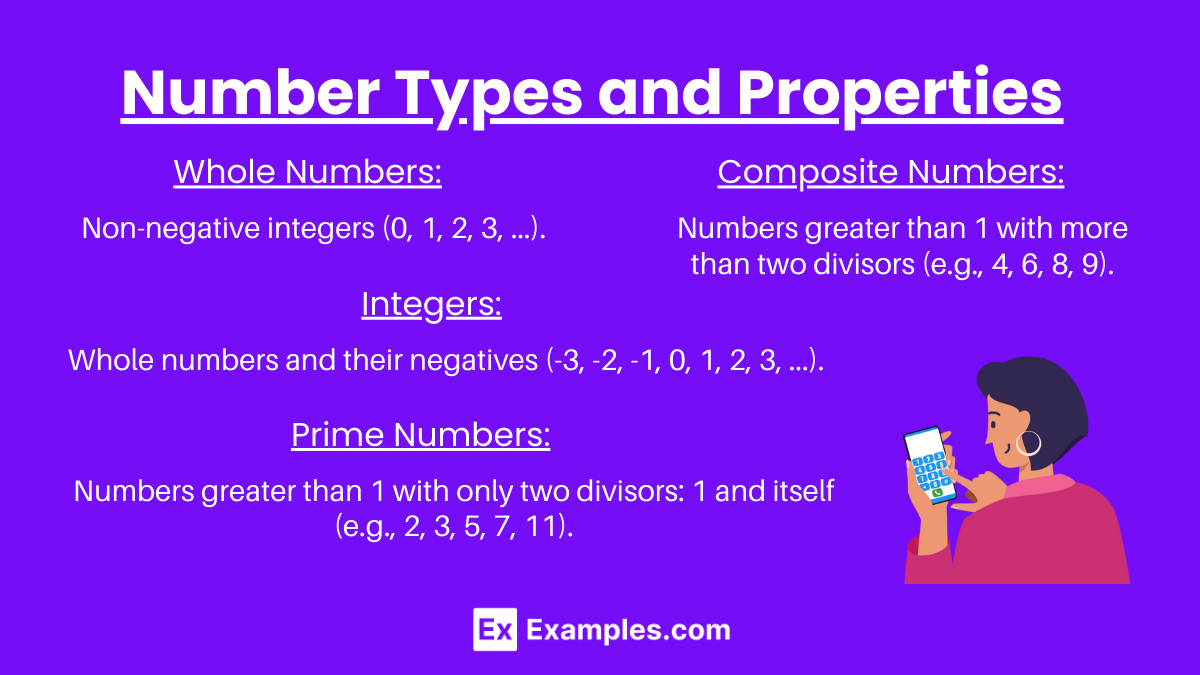
Number Types and Properties

Whole Numbers: Non-negative integers (0, 1, 2, 3, …).

Integers: Whole numbers and their negatives (-3, -2, -1, 0, 1, 2, 3, …).

Rational Numbers: Numbers that can be expressed as a fraction of two integers (e.g.,

$\left(\frac{1}{2}, \frac{3}{4}\right)$

Irrational Numbers: Numbers that cannot be expressed as fractions (e.g.,

$\left(\pi, \sqrt{2}\right)$

Prime Numbers: Numbers greater than 1 with only two divisors: 1 and itself (e.g., 2, 3, 5, 7, 11).

Composite Numbers: Numbers greater than 1 with more than two divisors (e.g., 4, 6, 8, 9).

Operations and Properties

Addition and Subtraction: Basic arithmetic operations.

Commutative Property: a+b = b+a
Associative Property: a+(b+c) = (a+b)+c
Identity Property: a+0 = a

Multiplication and Division: Basic arithmetic operations.

Commutative Property: a×b = b×a
Associative Property: a×(b×c) = (a×b)×c
Identity Property: a×1 = a
Distributive Property: a(b+c) = ab+ac

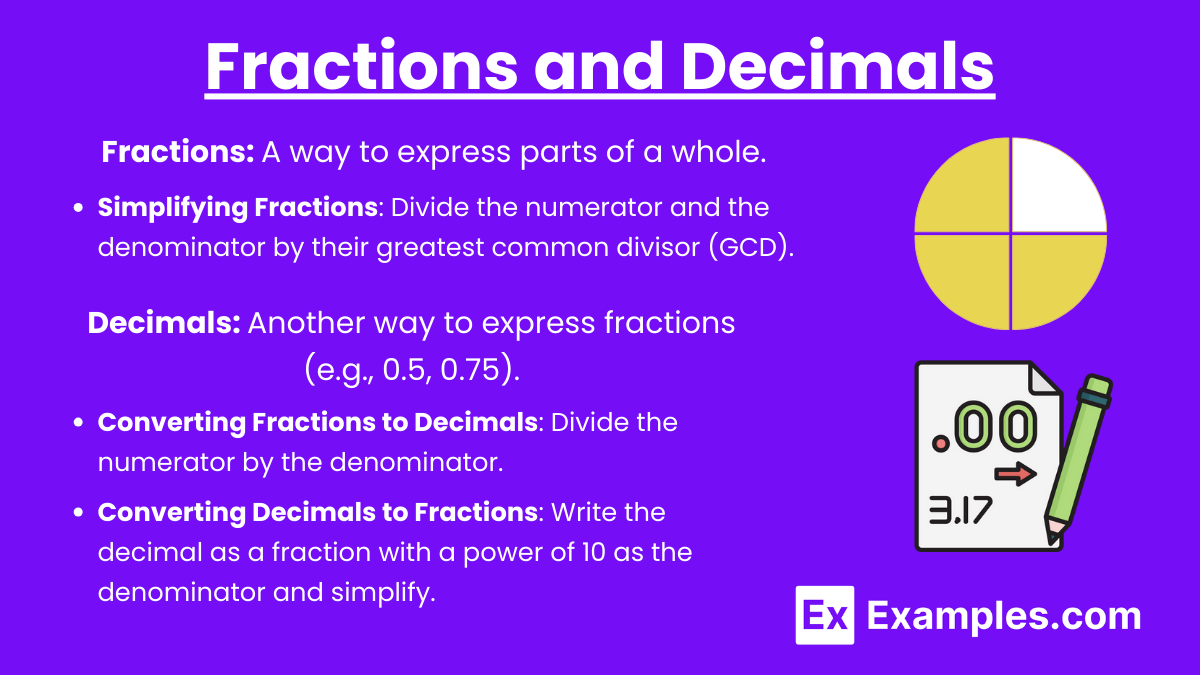
Fractions and Decimals

Fractions: A way to express parts of a whole.

Simplifying Fractions: Divide the numerator and the denominator by their greatest common divisor (GCD).

Decimals: Another way to express fractions (e.g., 0.5, 0.75).

Converting Fractions to Decimals: Divide the numerator by the denominator.
Converting Decimals to Fractions: Write the decimal as a fraction with a power of 10 as the denominator and simplify.

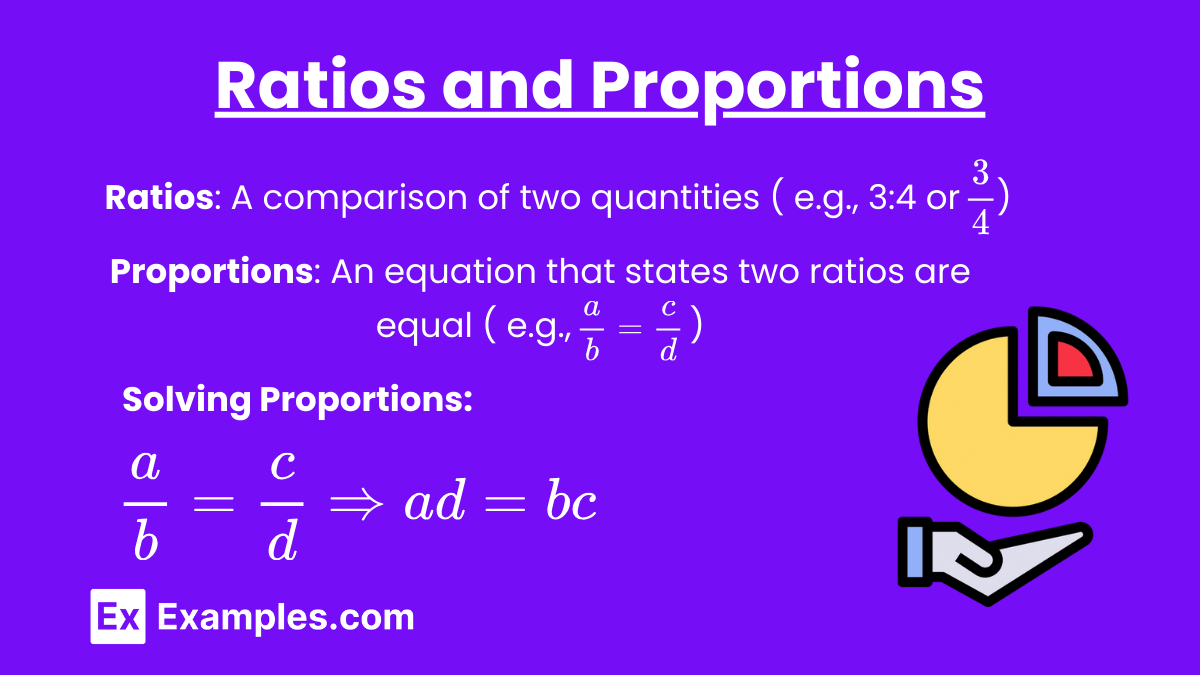
Ratios and Proportions

Ratios: A comparison of two quantities

Proportions: An equation that states two ratios are equal

Solving Proportions:

$\frac{a}{b} = \frac{c}{d} \Rightarrow ad = bc$

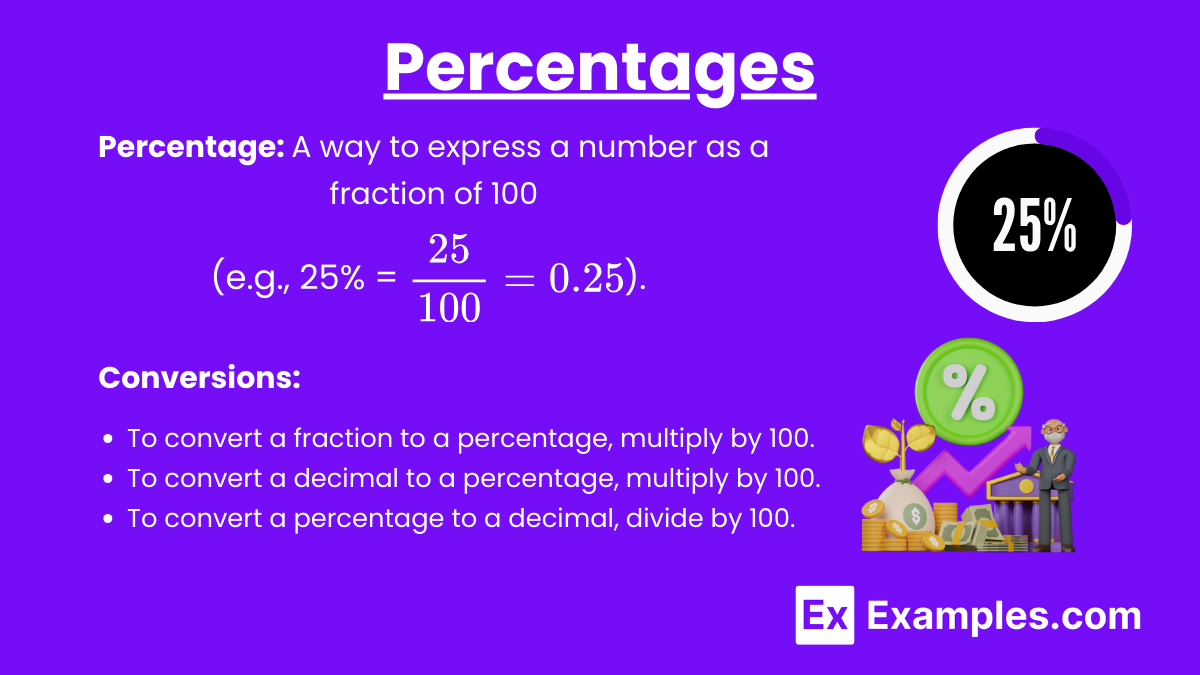
Percentages

Percentage: A way to express a number as a fraction of 100

Conversions:

To convert a fraction to a percentage, multiply by 100.
To convert a decimal to a percentage, multiply by 100.
To convert a percentage to a decimal, divide by 100.

Basic Geometry

Shapes and Properties:

Triangles: Sum of interior angles is 180⁰.
Quadrilaterals: Sum of interior angles is 360°.
Circles: C = 2πrC , A = πr²

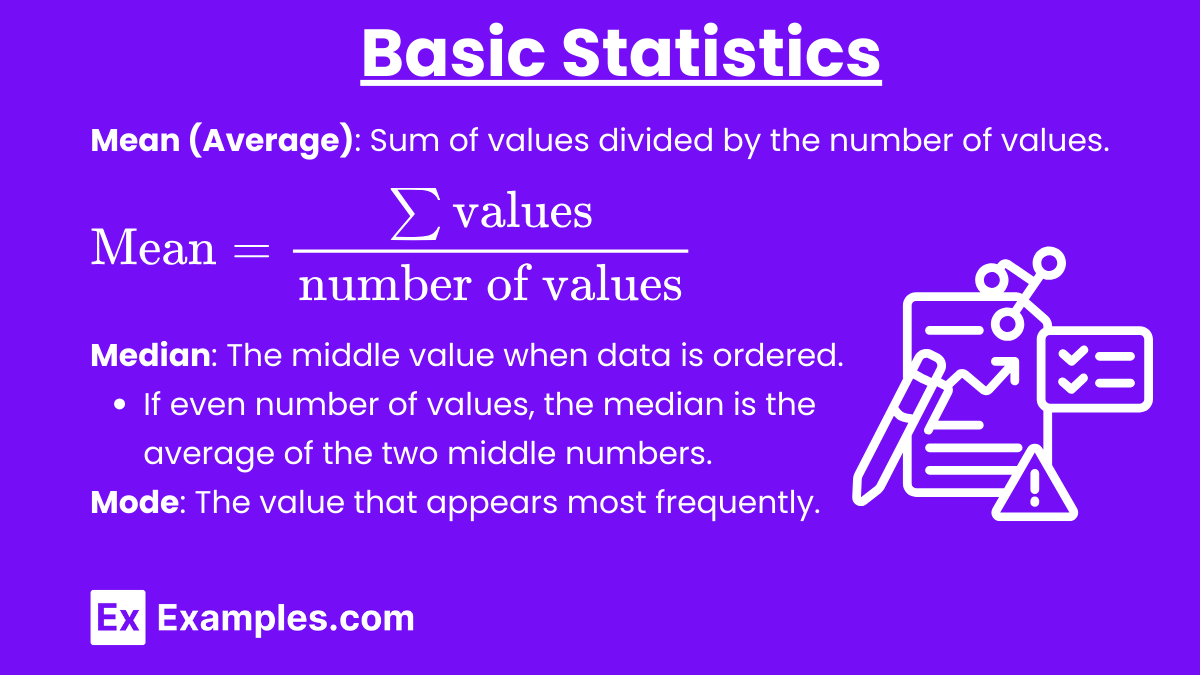
Basic Statistics

Mean (Average): Sum of values divided by the number of values.

$\text{Mean} = \frac{\sum \text{values}}{\text{number of values}}$

Median: The middle value when data is ordered.

If even number of values, the median is the average of the two middle numbers.

Mode: The value that appears most frequently.

Simple Equations and Inequalities

Equations: Mathematical statements that assert the equality of two expressions.

Solving Simple Equations: Isolate the variable using inverse operations.

Inequalities: Expressions that compare two values.

Solving Simple Inequalities: Similar to solving equations but remember to reverse the inequality sign when multiplying or dividing by a negative number.

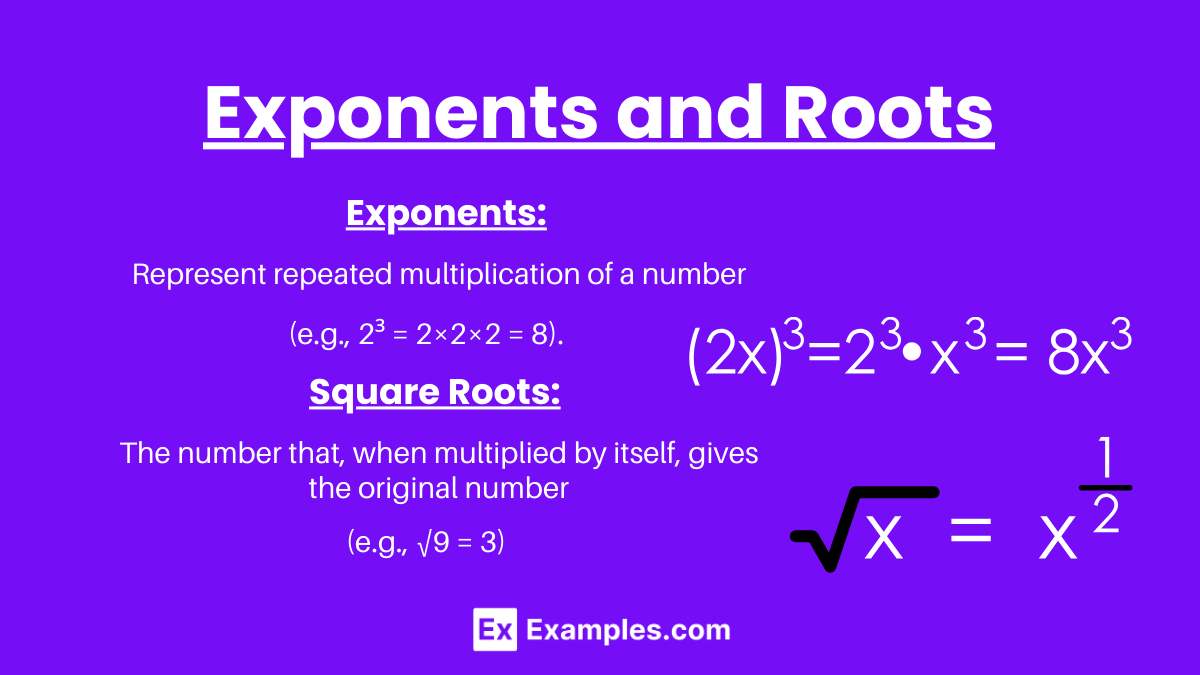
Exponents and Roots

Exponents: Represent repeated multiplication of a number (e.g., 2³ = 2×2×2 = 8).

Square Roots: The number that, when multiplied by itself, gives the original number (e.g., √9 = 3)

Pre-Algebra Practice Multiple Choice Questions

Question 1:

Solve for x in the equation: 2x+5 = 17.

A. x = 5
B. x = 6
C. x = 7
D. x = 8

Answer: B. x = 6

Explanation:

To solve the equation 2x+5 = 17, follow these steps:

Subtract 5 from both sides: 2x+5−5 = 17−5
2x = 12
Divide both sides by 2:
$\frac{2x}{2} = \frac{12}{2}$

x = 6

So, the correct answer is B. x = 6.

Question 2:

What is the value of the expression 3(a+4)−2a when a = 5?

A. 15
B. 17
C. 23
D. 29

Answer: C. 23

Explanation:

First, substitute a = 5 into the expression 3(a+4)−2a:

Substitute a = 5: 3(5+4)−2(5)
Simplify inside the parentheses: 3(9)−2(5)
Multiply: 27−10
Subtract: 27−10 = 17

So, the correct answer is C. 23.

Question 3:

If the perimeter of a rectangle is 36 units and the length is 10 units, what is the width?

A. 6 units
B. 8 units
C. 9 units
D. 12 units

Answer: A. 6 units

Explanation:

The formula for the perimeter of a rectangle is P = 2l+2w, where l is the length and w is the width. Given P = 36 and l = 10:

Substitute the known values into the perimeter formula: 36 = 2(10)+2w
Simplify: 36 = 20+2w
Subtract 20 from both sides: 36−20 = 2w
16 = 2w
Divide both sides by 2:
$\frac{16}{2} = \frac{2w}{2}$

w = 8

So, the correct answer is A. 6 units.