Which of the following numbers is divisible by 11?
121
123
122
120
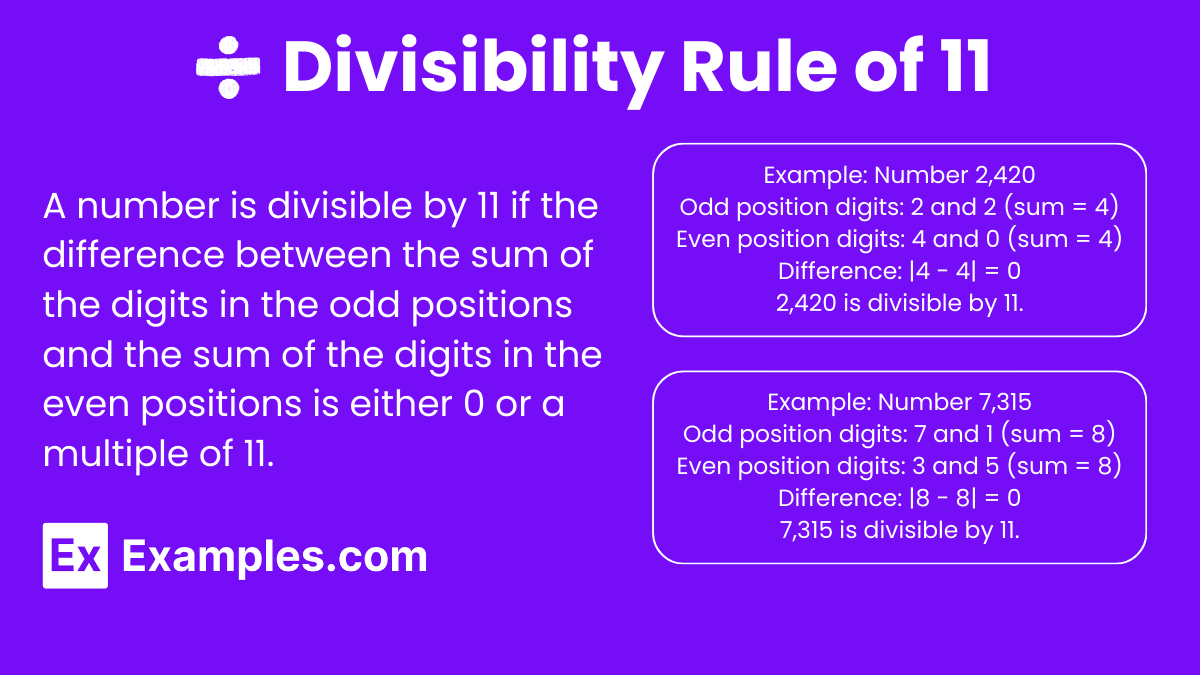
The divisibility rule of 11 helps determine if a number is divisible by 11. To apply this rule, alternate the addition and subtraction of the digits in the number. If the resulting sum is a multiple of 11 (including 0), then the number is divisible by 11. This rule is an important concept in algebra and number theory. Understanding this rule enhances skills in addition, subtraction, multiplication, and division of integers and rational numbers. It also helps distinguish between rational and irrational numbers, deepening the comprehension of mathematical operations.
Download Proof of the Divisibility Rule of 11 in PDF

Download Proof of the Divisibility Rule of 11 in PDF
Step 1: Start with a number: Consider a number, for example, 3524.
Step 2: Identify digits: Break down the number into its individual digits: 3, 5, 2, 4.
Step 3: Assign positions: Label the positions of the digits from right to left as odd or even. For 3524, the positions are:
Step 4: Sum odd-position digits: Add the digits in the odd positions:
Step 5: Sum even-position digits: Add the digits in the even positions:
Step 6: Find the difference: Subtract the sum of the even-position digits from the sum of the odd-position digits:
Step 7: Check divisibility: Determine if the difference is 0 or a multiple of 11:
To determine if a number is divisible by 11, follow these steps:
To determine if a number is divisible by 12, follow these steps:
If a number satisfies both conditions, it is divisible by 12.
To determine if a number is divisible by 11, follow these steps:
To determine if a number is divisible by 7, follow these steps:
Identify the digits: 5, 0, 6
Alternate sum:
Check the result: 11 is a multiple of 11.
Conclusion: 506 is divisible by 11.
Identify the digits: 1, 2, 3, 4, 5
Alternate sum:
Check the result: 3 is not a multiple of 11.
Conclusion: 12345 is not divisible by 11.
Identify the digits: 9, 1, 8, 2, 7, 3
Alternate sum:
Check the result: 18 is not a multiple of 11.
Conclusion: 918273 is not divisible by 11.
Identify the digits: 1, 4, 2, 8, 5, 7
Alternate sum:
Check the result: -11 is a multiple of 11.
Conclusion: 142857 is divisible by 11.
Identify the digits: 1, 2, 3, 4, 5, 6, 7, 8, 9
Alternate sum:
Check the result: 5 is not a multiple of 11.
Conclusion: 123456789 is not divisible by 11.
To check if a number is divisible by 11, alternate adding and subtracting its digits. If the resulting sum is 0 or a multiple of 11, then the number is divisible by 11.
The rule works because of the properties of numbers in base 10. When you alternate adding and subtracting digits, you effectively test if the number can be represented in a form that is a multiple of 11.
Sure! For the number 728:
Alternating sum: 7 – 2 + 8 = 13
Since 13 is not a multiple of 11, 728 is not divisible by 11.
Yes. For the number 121:
Alternating sum: 1 – 2 + 1 = 0
Since 0 is a multiple of 11, 121 is divisible by 11.
If the alternating sum is negative, take the absolute value of the result. If the absolute value is a multiple of 11, the original number is divisible by 11.
Yes, the rule applies to numbers of any size. You simply apply the same process of alternating addition and subtraction of digits.
No, the rule applies only to whole numbers. Decimals are not considered in the divisibility rule of 11.
The rule of 11 is unique in that it requires alternating addition and subtraction of digits, unlike simpler rules such as those for 2, 3, or 5, which often involve straightforward sums or checking the last digit.
Learning the rule helps in simplifying mathematical problems, especially in number theory and algebra, and enhances mental math skills. It also aids in quickly determining factors and multiples.

The divisibility rule of 11 helps determine if a number is divisible by 11. To apply this rule, alternate the addition and subtraction of the digits in the number. If the resulting sum is a multiple of 11 (including 0), then the number is divisible by 11. This rule is an important concept in algebra and number theory. Understanding this rule enhances skills in addition, subtraction, multiplication, and division of integers and rational numbers. It also helps distinguish between rational and irrational numbers, deepening the comprehension of mathematical operations.
Download Proof of the Divisibility Rule of 11 in PDF
The divisibility rule of 11 states that a number is divisible by 11 if the difference between the sum of its digits in odd positions and the sum of its digits in even positions is either 0 or a multiple of 11. For example, for the number 2728, (2 + 2) – (7 + 8) = -11, which is a multiple of 11, so 2728 is divisible by 11. This rule simplifies checking large numbers for divisibility by 11.
Download Proof of the Divisibility Rule of 11 in PDF
Step 1: Start with a number: Consider a number, for example, 3524.
Step 2: Identify digits: Break down the number into its individual digits: 3, 5, 2, 4.
Step 3: Assign positions: Label the positions of the digits from right to left as odd or even. For 3524, the positions are:
4 (odd)
2 (even)
5 (odd)
3 (even)
Step 4: Sum odd-position digits: Add the digits in the odd positions:
Odd positions: 4 and 5
Sum of odd positions: 4 + 5 = 9
Step 5: Sum even-position digits: Add the digits in the even positions:
Even positions: 2 and 3
Sum of even positions: 2 + 3 = 5
Step 6: Find the difference: Subtract the sum of the even-position digits from the sum of the odd-position digits:
Difference: 9 – 5 = 4
Step 7: Check divisibility: Determine if the difference is 0 or a multiple of 11:
Step 8: Since 4 is not a multiple of 11, 3524 is not divisible by 11.
To determine if a number is divisible by 11, follow these steps:
Identify digits: Break down the number into its individual digits.
Alternate sum: Alternate the addition and subtraction of the digits from left to right.
Calculate difference: Find the absolute value of the result.
Check the result: If the result is 0 or a multiple of 11, the number is divisible by 11.
Number: 374
Alternating sum: 3 – 7 + 4 = 0
Since the result is 0, 374 is divisible by 11.
To determine if a number is divisible by 12, follow these steps:
Sum the digits of the number.
Check if the sum is divisible by 3.
Check the last two digits of the number.
If the last two digits form a number divisible by 4, then the number is divisible by 4.
If a number satisfies both conditions, it is divisible by 12.
Number: 528
Sum of digits: 5 + 2 + 8 = 15 (15 is divisible by 3)
Last two digits: 28 (28 is divisible by 4)
Since 528 meets both conditions, it is divisible by 12.
To determine if a number is divisible by 11, follow these steps:
Identify digits: Break down the number into its individual digits.
Alternate sum: Alternate the addition and subtraction of the digits from left to right.
Calculate difference: Find the absolute value of the result.
Check the result: If the result is 0 or a multiple of 11, the number is divisible by 11.
Number: 374
Alternating sum: 3 – 7 + 4 = 0
Result: Since the result is 0, 374 is divisible by 11.
To determine if a number is divisible by 7, follow these steps:
Double the last digit: Take the last digit of the number and double it.
Subtract from the rest: Subtract the doubled value from the rest of the digits.
Repeat if necessary: Repeat the process with the new number if needed.
Check the result: If the resulting number is 0 or a multiple of 7, the original number is divisible by 7.
Number: 203
Step 1: Double the last digit (3 × 2 = 6)
Step 2: Subtract from the rest (20 – 6 = 14)
Result: Since 14 is a multiple of 7, 203 is divisible by 7.
Identify the digits: 5, 0, 6
Alternate sum:
5 (odd position)
0 (even position)
6 (odd position)
Alternating sum: 5 – 0 + 6 = 11
Check the result: 11 is a multiple of 11.
Conclusion: 506 is divisible by 11.
Identify the digits: 1, 2, 3, 4, 5
Alternate sum:
1 (odd position)
2 (even position)
3 (odd position)
4 (even position)
5 (odd position)
Alternating sum: 1 – 2 + 3 – 4 + 5 = 3
Check the result: 3 is not a multiple of 11.
Conclusion: 12345 is not divisible by 11.
Identify the digits: 9, 1, 8, 2, 7, 3
Alternate sum:
9 (odd position)
1 (even position)
8 (odd position)
2 (even position)
7 (odd position)
3 (even position)
Alternating sum: 9 – 1 + 8 – 2 + 7 – 3 = 18
Check the result: 18 is not a multiple of 11.
Conclusion: 918273 is not divisible by 11.
Identify the digits: 1, 4, 2, 8, 5, 7
Alternate sum:
1 (odd position)
4 (even position)
2 (odd position)
8 (even position)
5 (odd position)
7 (even position)
Alternating sum: 1 – 4 + 2 – 8 + 5 – 7 = -11
Check the result: -11 is a multiple of 11.
Conclusion: 142857 is divisible by 11.
Identify the digits: 1, 2, 3, 4, 5, 6, 7, 8, 9
Alternate sum:
1 (odd position)
2 (even position)
3 (odd position)
4 (even position)
5 (odd position)
6 (even position)
7 (odd position)
8 (even position)
9 (odd position)
Alternating sum: 1 – 2 + 3 – 4 + 5 – 6 + 7 – 8 + 9 = 5
Check the result: 5 is not a multiple of 11.
Conclusion: 123456789 is not divisible by 11.
To check if a number is divisible by 11, alternate adding and subtracting its digits. If the resulting sum is 0 or a multiple of 11, then the number is divisible by 11.
The rule works because of the properties of numbers in base 10. When you alternate adding and subtracting digits, you effectively test if the number can be represented in a form that is a multiple of 11.
Sure! For the number 728:
Alternating sum: 7 – 2 + 8 = 13
Since 13 is not a multiple of 11, 728 is not divisible by 11.
Yes. For the number 121:
Alternating sum: 1 – 2 + 1 = 0
Since 0 is a multiple of 11, 121 is divisible by 11.
If the alternating sum is negative, take the absolute value of the result. If the absolute value is a multiple of 11, the original number is divisible by 11.
Yes, the rule applies to numbers of any size. You simply apply the same process of alternating addition and subtraction of digits.
No, the rule applies only to whole numbers. Decimals are not considered in the divisibility rule of 11.
The rule of 11 is unique in that it requires alternating addition and subtraction of digits, unlike simpler rules such as those for 2, 3, or 5, which often involve straightforward sums or checking the last digit.
Learning the rule helps in simplifying mathematical problems, especially in number theory and algebra, and enhances mental math skills. It also aids in quickly determining factors and multiples.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which of the following numbers is divisible by 11?
121
123
122
120
Is 3456 divisible by 11?
Yes
No
Cannot be determined
None of the above
Which number below is divisible by 11?
1234
2424
4567
9876
Determine if 909 is divisible by 11.
Yes
No
Cannot be determined
None of the above
Which of the following numbers is not divisible by 11?
1211
1221
1313
1444
Which number is divisible by 11?
987
363
452
529
Determine if 2835 is divisible by 11.
Yes
No
Cannot be determined
None of the above
Which number below is divisible by 11?
143
234
456
789
Which of the following numbers is divisible by 11?
161
202
343
484
Determine if 1593 is divisible by 11.
Yes
No
Cannot be determined
None of the above
Before you leave, take our quick quiz to enhance your learning!

