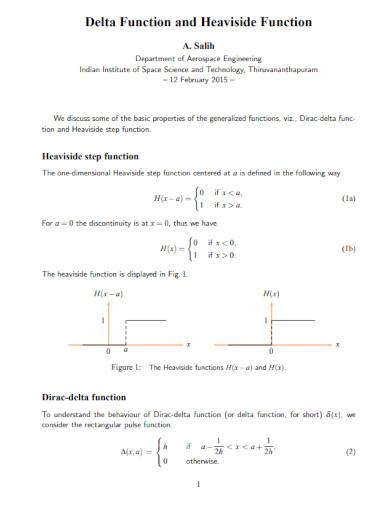
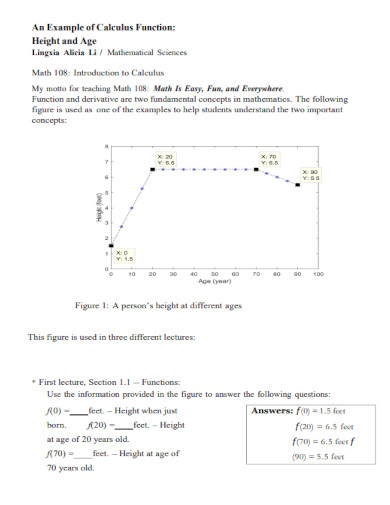
Which of the following describes a function?
A relation where each input has multiple outputs.
A relation where each input has exactly one output.
A relation where each output has multiple inputs.
A relation with no specific input-output pairing.


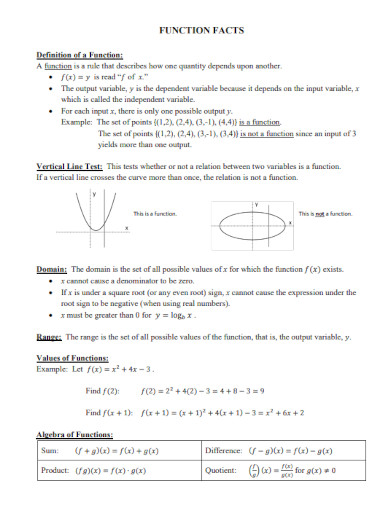
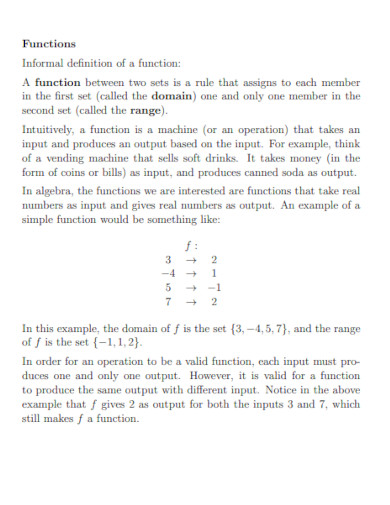
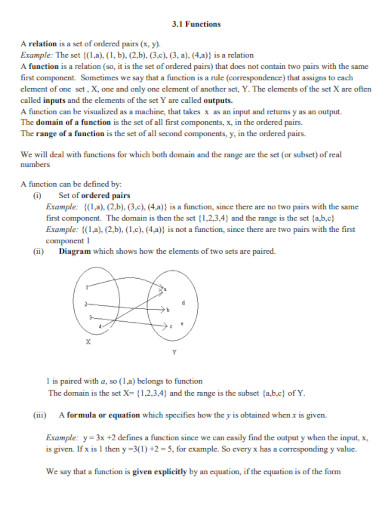
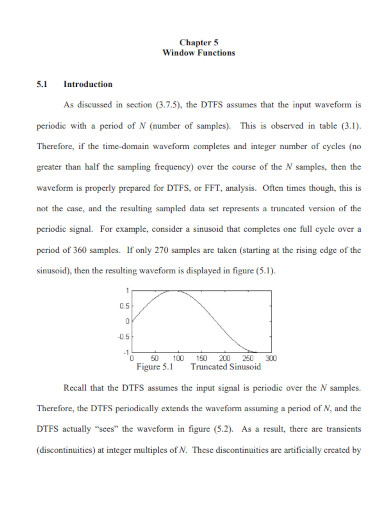
In mathematics, there are many relationships that people can investigate and deal with, like quadratic equations, summations, and permutations. A unique quantity or variable can have a relationship with another individual variable, which people call a function.
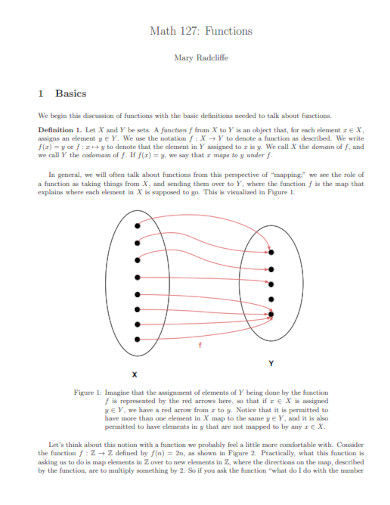
A function is a fundamental rule or law that relates one variable to another variable usually in the form of an independent variable and a dependent variable. This is a very important concept as it will allow people to create formulas and solutions to solve problems or relate mathematical concepts to physics and other practical contexts, themes, and tones.
A person can graph a function in a cartesian plane due to its specific unique nature. It is very easy to identify a function because of its specific elements and characteristics that set it apart from other types of mathematical relationships.
People can illustrate functions in variable pairs, which will not have recurring numbers in other pairs. The outline format or outline of the function will look like (X1, Y1), where X is the independent variable and Y is the dependent variable.
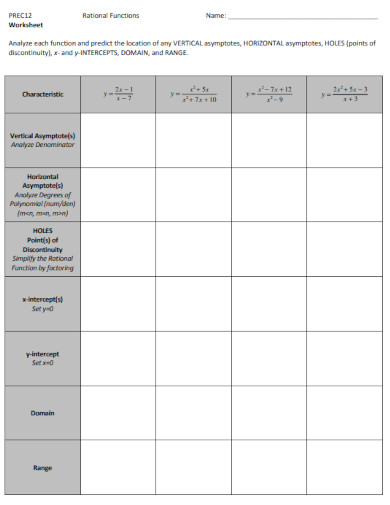
If the variable set is in the form of a quadratic function, you must obtain both the variables pairs that are present in the whole function. Often a quadratic function will create two sets of x and y pairs.
Functions only occur when the variables in the pairs do not repeat in subsequent pairs. To determine if the variable set illustrates a function, then you must check if the variables repeat in the sequence. If it does, then the variable set is not a function.
Functions can be graphed by using the Xs and Ys of the different variables to graph a specific shape or curve. If the graph repeats a specific X or Y point, then the variable set is not a function.
Functions have many uses due to the basic element of having a variable be related to another one. For example, a person can buy a specific product for a certain price, this whole process is made efficient due to the use of functions. Various machines and algorithms use this concept to allow specific inputs to have a specific output.
Functions have a practical bearing that allows people to associate specific things with other things like price, requirements, and needs. For example, drivers can easily estimate the amount of distance and time they can travel based on the number of fuel present in their vehicle. This can be done by associating the specific amount of fuel with an estimated amount of distance
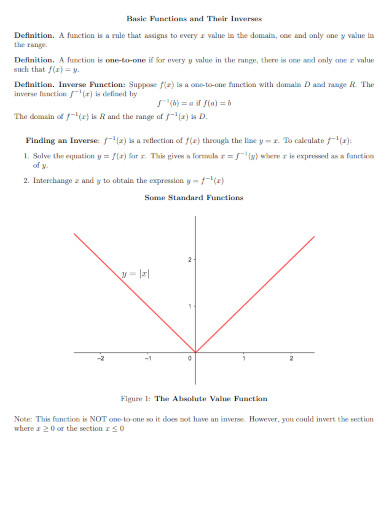
A vertical line test is a tool people can use to determine if the set of variables forms a function. This test will determine if the line will hit unique and specific X and Y-coordinates.
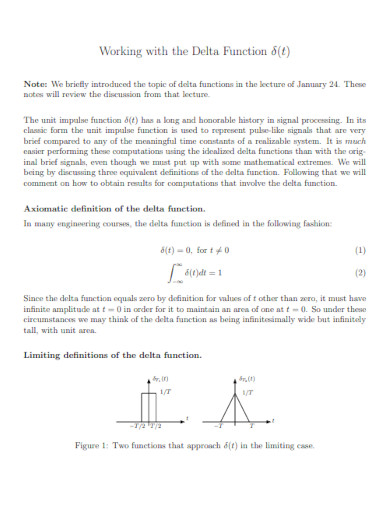
A function is a specific set of variables that will not have any repeats in the set. A function often connects one variable to another variable, which people usually denote as independent and dependent variables.

In mathematics, there are many relationships that people can investigate and deal with, like quadratic equations, summations, and permutations. A unique quantity or variable can have a relationship with another individual variable, which people call a function.
hancockcollege.edu
Details
File Format
Size: 126 KB
berkeleycitycollege.edu
Details
File Format
Size: 97 KB

shsu.edu
Details
File Format
Size: 551 KB
bates.edu
Details
File Format
Size: 445 KB

cerritos.edu
Details
File Format
Size: 341 KB
math.cmu.edu
Details
File Format
Size: 693 KB

math.rutgers.edu
Details
File Format
Size: 94 KB
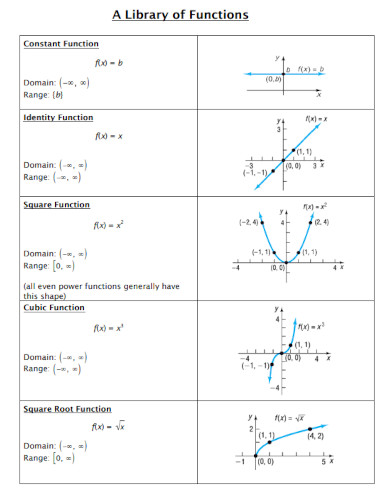
losmedanos.edu
Details
File Format
Size: 633 KB

mathcentre.ac.uk
Details
File Format
Size: 118 KB
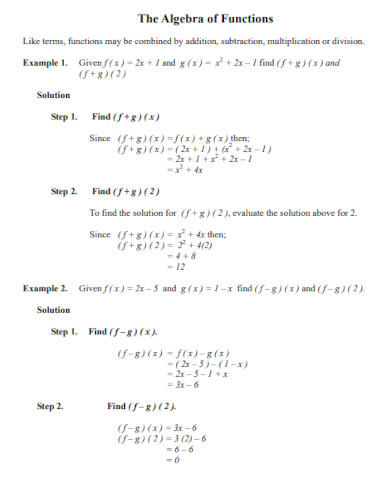
alamo.edu
Details
File Format
Size: 59 KB

alamo.edu
Details
File Format
Size: 31 KB

math.hawaii.edu
Details
File Format
Size: 86 KB

citeseerx.ist.psu.edu
Details
File Format
Size: 98 KB
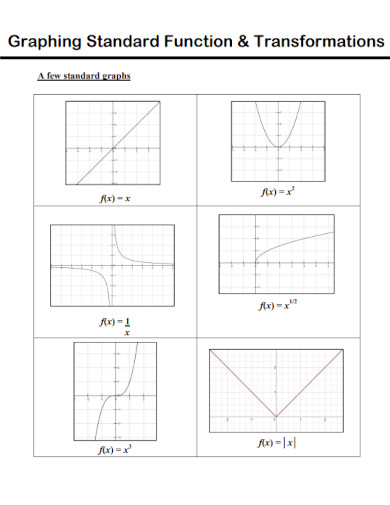
tutoring.asu.edu
Details
File Format
Size: 459 KB
math.mit.edu
Details
File Format
Size: 184 KB

resources.saylor.org
Details
File Format
Size: 118 KB

whatcom.edu
Details
File Format
Size: 359 KB

scholarlyoa.com
Details
File Format
Size: 266 KB
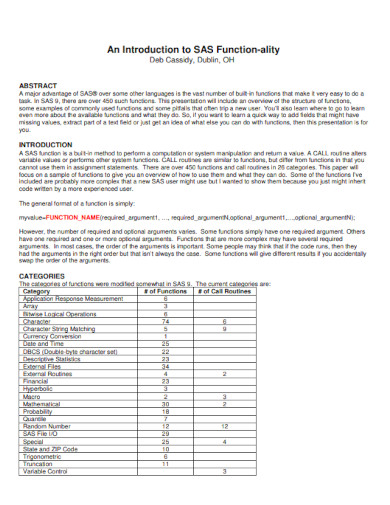
support.sas.com
Details
File Format
Size: 96 KB

fema.gov
Details
File Format
Size: 129 KB

harvard.edu
Details
File Format
Size: 44 KB

suffolk.edu
Details
File Format
Size: 85 KB
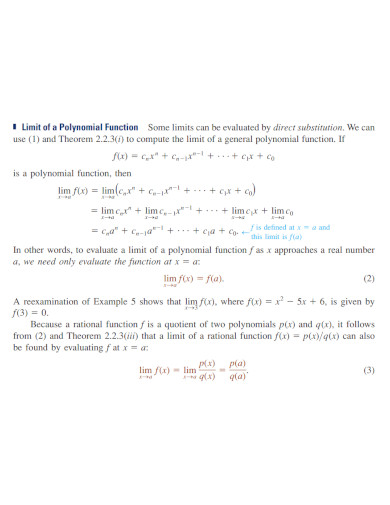
samples.jbpub.com
Details
File Format
Size: 4 MB

fitelson.org
Details
File Format
Size: 8000 KB

college.cengage.com
Details
File Format
Size: 20 KB
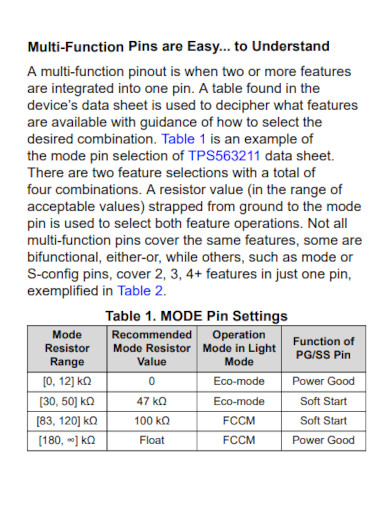
ti.com
Details
File Format
Size: 372 KB

fhwa.dot.gov
Details
File Format
Size: 194 KB

mathweb.ucsd.edu
Details
File Format
Size: 2 MB

media.news.health.ufl.edu
Details
File Format
Size: 15 KB
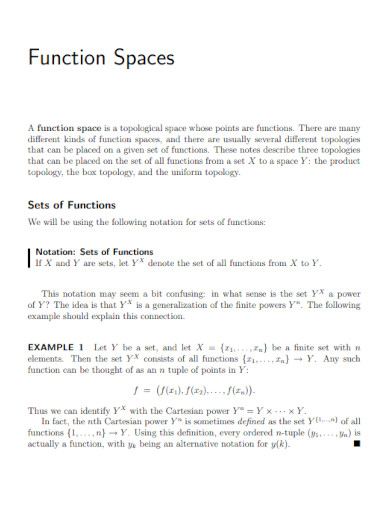
e.math.cornell.edu
Details
File Format
Size: 142 KB

math.uh.edu
Details
File Format
Size: 51 KB
ucl.ac.uk
Details
File Format
Size: 65 KB

caps.unm.edu
Details
File Format
Size: 92 KB
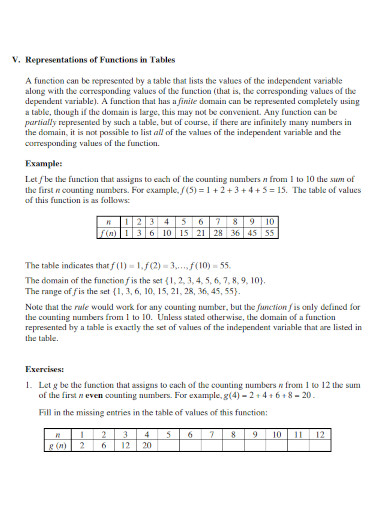
math.kent.edu
Details
File Format
Size: 186 KB

des.nh.gov
Details
File Format
Size: 410 KB

people.math.wisc.edu
Details
File Format
Size: 443 KB

heil.math.gatech.edu
Details
File Format
Size: 76 KB

engr.mun.ca
Details
File Format
Size: 61 KB
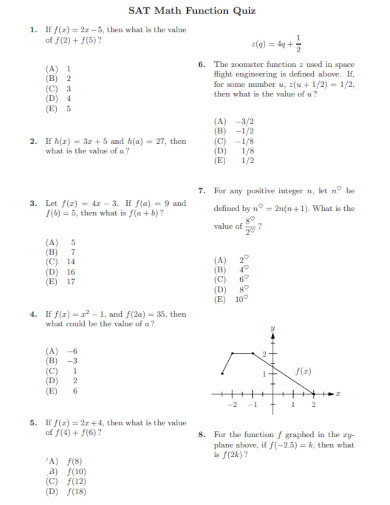
erikthered.com
Details
File Format
Size: 34 KB

doe.virginia.gov
Details
File Format
Size: 280 KB
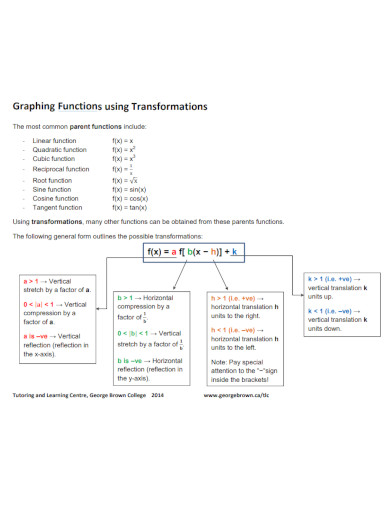
georgebrown.ca
Details
File Format
Size: 601 KB

math.leidenuniv.nl
Details
File Format
Size: 254 KB

cs.toronto.edu
Details
File Format
Size: 442 KB

sites.math.northwestern.edu
Details
File Format
Size: 58 KB
hr.nv.gov
Details
File Format
Size: 199 KB
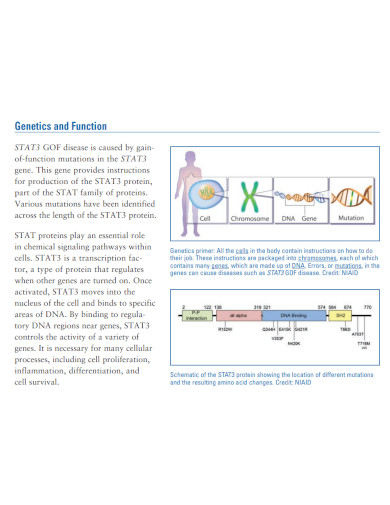
niaid.nih.gov
Details
File Format
Size: 271 KB
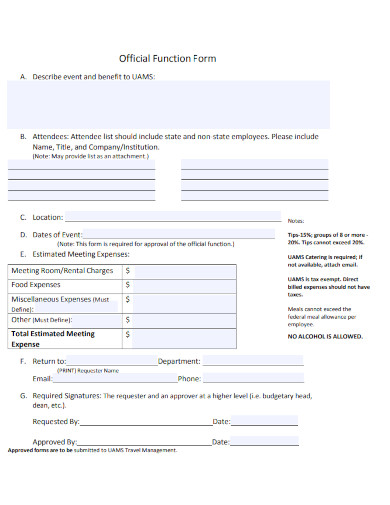
finance.uams.edu
Details
File Format
Size: 323 KB

web.mnstate.ed
Details
File Format
Size: 24 KB

terrytao.files.wordpress.com
Details
File Format
Size: 129 KB
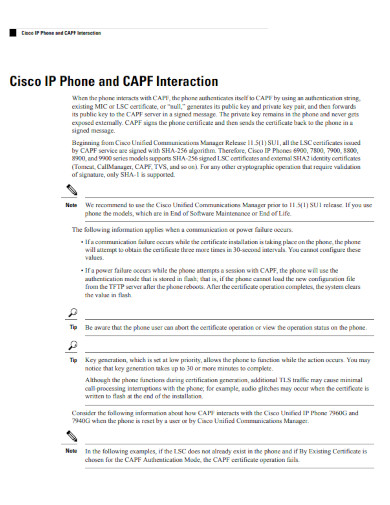
cisco.com
Details
File Format
Size: 1 MB

watermark.silverchair.com
Details
File Format
Size: 777 KB
faculty.fiu.edu
Details
File Format
Size: 320 KB

isabelle.in.tum.de
Details
File Format
Size: 465 KB

sgp.fas.org
Details
File Format
Size: 332 KB

virtuallearningacademy.net
Details
File Format
Size: 104 KB

web.uvic.ca
Details
File Format
Size: 90 KB
iist.ac.in
Details
File Format
Size: 57 KB
plaza.ufl.edu
Details
File Format
Size: 182 KB

mat.uab.cat
Details
File Format
Size: 445 KB

faculty.math.illinois.edu
Details
File Format
Size: 168 KB

mae.ufl.edu
Details
File Format
Size: 106 KB
mrsk.ca
Details
File Format
Size: 361 KB

math.vanderbilt.edu
Details
File Format
Size: 166 KB

reed.edu
Details
File Format
Size: 116 KB

jmap.org
Details
File Format
Size: 92 KB

cchem.berkeley.edu
Details
File Format
Size: 68 KB
course.ece.cmu.edu
Details
File Format
Size: 279 KB

undergroundmathematics.org
Details
File Format
Size: 21 KB

arxiv.org
Details
File Format
Size: 304 KB

axon.cs.byu.edu
Details
File Format
Size: 331 KB
aemc.fr
Details
File Format
Size: 1 MB

johnstonmd.files.wordpress.com
Details
File Format
Size: 106 KB
m-media.resosir.com
Details
File Format
Size: 179 KB

ore.ac.uk
Details
File Format
Size: 96 KB

eprint.iacr.org
Details
File Format
Size: 950 KB

web.gccaz.edu
Details
File Format
Size: 363 KB

math.columbia.edu
Details
File Format
Size: 211 KB
siers.weebly.com
Details
File Format
Size: 343 KB

archives-ouvertes.fr
Details
File Format
Size: 127 KB
allegion.com
Details
File Format
Size: 69 KB

web.gccaz.edu
Details
File Format
Size: 433 KB

webpages.uidaho.edu
Details
File Format
Size: 212 KB
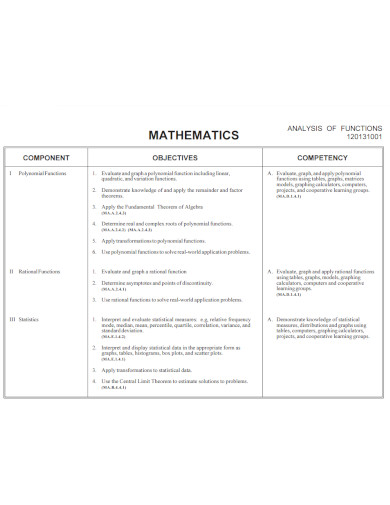
dadeschools.net
Details
File Format
Size: 382 KB
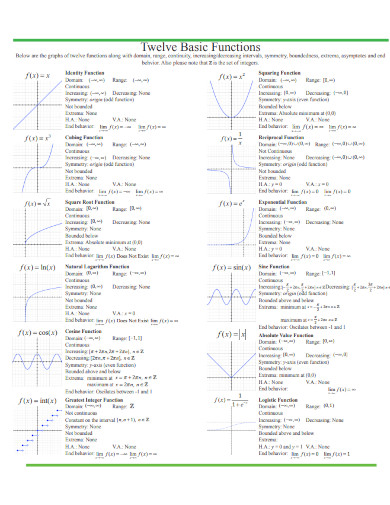
learntutoring.com
Details
File Format
Size: 532 KB
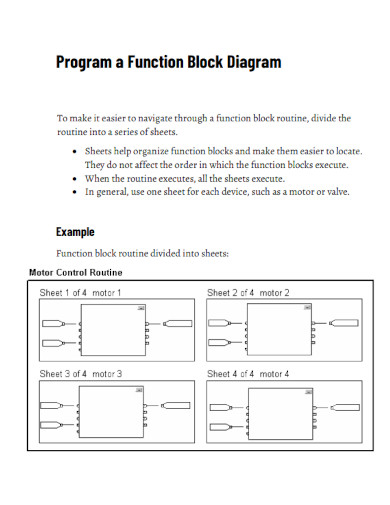
literature.rockwellautomation.com
Details
File Format
Size: 2 MB

math.ucdavis.edu
Details
File Format
Size: 241 KB

austincc.edu
Details
File Format
Size: 284 KB

page.mi.fu-berlin.de
Details
File Format
Size: 173 KB
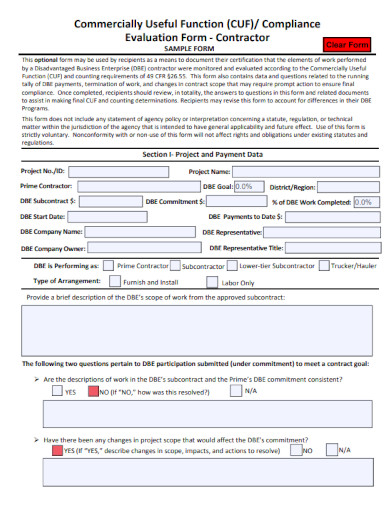
transportation.gov
Details
File Format
Size: 1 MB
stearnscenter.gmu.edu
Details
File Format
Size: 631 KB

amherst.edu
Details
File Format
Size: 32 KB
toomey.org
Details
File Format
Size: 525 KB

monkey.org
Details
File Format
Size: 159 KB
people.vcu.edu
Details
File Format
Size: 254 KB
oracle.com
Details
File Format
Size: 216 KB
anthonyschool.org
Details
File Format
Size: 331 KB
sac.edu
Details
File Format
Size: 73 KB
washoeschools.net
Details
File Format
Size: 354 KB
math.uiowa.edu
Details
File Format
Size: 173 KB

cdn.kutasoftware.com
Details
File Format
Size: 34 KB
A function is a fundamental rule or law that relates one variable to another variable usually in the form of an independent variable and a dependent variable. This is a very important concept as it will allow people to create formulas and solutions to solve problems or relate mathematical concepts to physics and other practical contexts, themes, and tones.
A person can graph a function in a cartesian plane due to its specific unique nature. It is very easy to identify a function because of its specific elements and characteristics that set it apart from other types of mathematical relationships.
People can illustrate functions in variable pairs, which will not have recurring numbers in other pairs. The outline format or outline of the function will look like (X1, Y1), where X is the independent variable and Y is the dependent variable.
If the variable set is in the form of a quadratic function, you must obtain both the variables pairs that are present in the whole function. Often a quadratic function will create two sets of x and y pairs.
Functions only occur when the variables in the pairs do not repeat in subsequent pairs. To determine if the variable set illustrates a function, then you must check if the variables repeat in the sequence. If it does, then the variable set is not a function.
Functions can be graphed by using the Xs and Ys of the different variables to graph a specific shape or curve. If the graph repeats a specific X or Y point, then the variable set is not a function.
Functions have many uses due to the basic element of having a variable be related to another one. For example, a person can buy a specific product for a certain price, this whole process is made efficient due to the use of functions. Various machines and algorithms use this concept to allow specific inputs to have a specific output.
Functions have a practical bearing that allows people to associate specific things with other things like price, requirements, and needs. For example, drivers can easily estimate the amount of distance and time they can travel based on the number of fuel present in their vehicle. This can be done by associating the specific amount of fuel with an estimated amount of distance
A vertical line test is a tool people can use to determine if the set of variables forms a function. This test will determine if the line will hit unique and specific X and Y-coordinates.
A function is a specific set of variables that will not have any repeats in the set. A function often connects one variable to another variable, which people usually denote as independent and dependent variables.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which of the following describes a function?
A relation where each input has multiple outputs.
A relation where each input has exactly one output.
A relation where each output has multiple inputs.
A relation with no specific input-output pairing.
What is the domain of a function?
The set of all possible inputs.
The set of all possible outputs.
The set of all possible pairs.
The set of all possible values that a function cannot take.
In the function f(x) = 2x + 3 , what is the output when x = 5 ?
11
12
13
14
If f(x) = x^2 - 4 , what is f(3) ?
3
4
5
7
Which of the following represents a function?
{ (1, 2), (2, 3), (1, 4) }
{ (1, 2), (2, 3), (3, 4) }
{ (1, 2), (2, 2), (2, 3) }
{ (2, 2), (2, 3), (3, 2) }
What is the range of a function?
The set of all possible inputs.
The set of all possible pairs.
The set of all possible outputs.
The set of all possible values that a function cannot take.
For the function f(x) = 3x - 7 , what is f(0) ?
7
0
-7
6
If f(x) = x + 4 and g(x) = 2x - 1 , what is (f \circ g)(2) ?
7
8
9
10
What is the inverse of the function f(x) = 2x + 5 ?
f^{-1}(x) = \frac{x - 5}{2}
f^{-1}(x) = \frac{x + 5}{2}
f^{-1}(x) = 2x - 5
f^{-1}(x) = \frac{x}{2} - 5
What is the domain of the function f(x) = \frac{1}{x - 2} ?
x \neq 2
x \geq 2
x \leq 2
All real numbers
Before you leave, take our quick quiz to enhance your learning!

