What is the value of ?
-1
1
0
-i

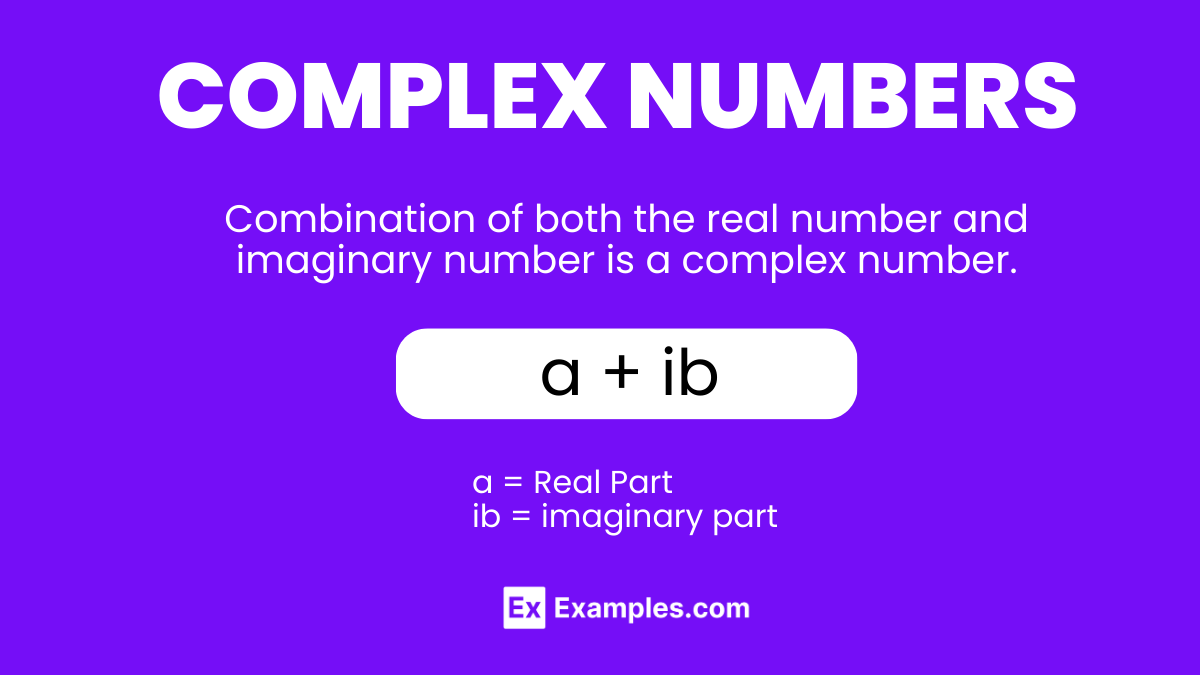
Complex Numbers a cornerstone concept in advanced mathematics. This guide offers a lucid introduction, tailored for educators and students, showcasing how complex numbers combine real and imaginary units to solve equations that real numbers alone cannot. Through practical examples and straightforward explanations, we aim to demystify complex numbers, making them accessible and captivating. Whether for academic curiosity or classroom instruction, this guide serves as an invaluable resource for exploring the intriguing world of complex mathematics.
Complex numbers are a sophisticated class of numbers that extend the traditional number line into a two-dimensional plane by incorporating both a real part and an imaginary part. This unique structure allows for the representation and solution of equations that no real number can solve. Essential in various fields of science and engineering, understanding complex numbers enriches one’s mathematical toolkit, opening doors to advanced topics in algebra, physics, and beyond.
A quintessential example of a complex number is the solution to the equation X2 = -1, which is X = √-1, , or i, where i is the imaginary unit. This forms the basis for complex numbers like a + bi where a and b are real numbers. Complex numbers such as 3 + 4i illustrate how they enable calculations and representations not possible with real numbers alone, showcasing their utility in mathematical and practical applications.
The formula for complex numbers, a + bi, combines a real number a and an imaginary number b multiplied by i, (where i2 = -1). This elegant formula lays the foundation for complex arithmetic, enabling operations like addition, subtraction, multiplication, and division in the complex plane. It’s a gateway to understanding the dynamic interactions between real and imaginary components, crucial for advanced mathematics, physics, and engineering.
The unit of complex numbers, known as the imaginary unit i, is the cornerstone of complex number theory. Defined by the property that i2=-1,it facilitates the expression of square roots of negative numbers, a concept not possible in the realm of real numbers. This unit extends our mathematical horizons, allowing for the exploration of phenomena in quantum mechanics, electrical engineering, and signal processing with unparalleled depth and clarity.
Real numbers encompass the entire spectrum of numbers used in mathematics, including all the rational and irrational numbers. They can be found on the number line, stretching infinitely in both positive and negative directions. This set includes everyday numbers used for counting, measuring, and ordering, making it fundamental in both abstract mathematical theories and real-world applications. Understanding real numbers is essential for grasping the full scope of mathematics, from basic arithmetic to complex analysis.
Notation in mathematics serves as a standardized language, allowing mathematicians and students to communicate complex ideas succinctly and precisely. This includes symbols for operations, functions, sets, and numbers. Effective notation is critical for understanding and conveying mathematical concepts, facilitating learning, problem-solving, and research. It forms the backbone of mathematical clarity and efficiency, from elementary education to advanced theoretical work.
Imaginary numbers are a class of numbers that are defined as multiples of the square root of -1, denoted as i. They cannot be found on the traditional number line and are crucial for solving equations that have no solution within the realm of real numbers. Imaginary numbers expand the scope of solutions available for quadratic equations and beyond, playing a key role in advanced mathematics, physics, and engineering.
To clearly understand the distinctions between complex numbers, real numbers, and imaginary numbers, let’s look at their definitions and characteristics in a structured table format:
| Feature | Complex Numbers | Real Numbers | Imaginary Numbers |
|---|---|---|---|
| Definition | Numbers that combine real and imaginary parts. | Numbers that can be found on the number line. | Numbers that are multiples of the imaginary unit i, where i2=-1. |
| Example | 3 + 4i | 5 | 2i |
| Representation | Expressed as a+bi where a is the real part and b is the imaginary part. | Directly represented as a point on the number line. | Expressed as bi where b is a real number. |
| Operational Base | Can be used in all forms of mathematical operations, extending the possibilities of calculations. | Limited to operations within the real number system. | Primarily used in operations involving square roots of negative numbers. |
| Practical Usage | Essential in fields requiring multidimensional analysis, like electrical engineering and quantum physics. | Used in everyday counting, measuring, and ordering. | Integral in complex number theory and certain aspects of wave mechanics. |
Yes, 0 is considered a complex number. It can be represented as 0+0i, where both the real and imaginary parts are zero. This highlights the inclusivity of the complex number system, encapsulating real numbers and providing a foundation for broader mathematical concepts.
Graphical representations bring abstract concepts to life. Here are five distinct examples related to the graphical representation of numbers:
These examples underscore the diverse ways in which numbers can be graphically represented, each offering unique insights into their properties and relationships.
The absolute value of a number represents its distance from zero on the number line, irrespective of direction. This concept is fundamental in mathematics, providing the groundwork for understanding magnitude and comparison among numbers. It’s crucial in various fields, including engineering and physics, where the size of a quantity matters more than its direction.
Examples:
Algebraic operations on complex numbers expand the realm of mathematics by enabling calculations involving both real and imaginary parts. These operations include addition, subtraction, multiplication, and division, essential for solving complex equations in advanced mathematics, physics, and engineering.
Examples:
Roots of complex numbers involve finding numbers that, when raised to a certain power, yield the original complex number. This concept is a cornerstone of complex analysis and plays a vital role in understanding the geometry of complex numbers and solving polynomial equations.
Examples:
Complex number formulas facilitate operations involving complex numbers, each embodying a mixture of real and imaginary parts. These formulas are pivotal in extending our ability to perform addition, subtraction, multiplication, and division beyond the realm of real numbers, enabling intricate calculations in fields like engineering, physics, and applied mathematics.
Examples:
The power of iota (i) is fundamental in complex numbers, cycling through four key values : i0 = 1, i1 = i, i2= -1, i3= -1, and i4 = -1 then repeating. This cyclic nature simplifies calculations involving powers of i by reducing them to one of these four outcomes.
Explanation: The first power of i is itself, serving as the basis for defining complex numbers.
Explanation: This fundamental identity underpins the square root of a negative number, defining the imaginary unit.
Explanation: Following the cycle, i3 returns the negative of the imaginary unit, further showcasing the cyclic nature.
Explanation: Completing the cycle, i4 resets back to 1, illustrating the periodicity in powers of i.
Explanation: Powers of i repeat every 4 cycles. Since 17 mod 4 = 1, i17= -1 simplifies to i.
Identities of complex numbers provide foundational truths that facilitate their manipulation and understanding. These identities are instrumental in solving complex equations and performing advanced operations in mathematics and physics.
Examples:
These examples and descriptions showcase the vast and intricate landscape of complex numbers, revealing their beauty and utility in mathematical explorations.
Complex numbers possess unique properties that set them apart in the mathematical world, facilitating their manipulation and application across various fields. These properties include their addition, subtraction, multiplication, and division rules, which maintain the integrity of complex numbers and enable complex equations’ solutions. Understanding these properties is essential for anyone delving into higher mathematics, physics, or engineering, as they lay the groundwork for advanced concepts in these disciplines.
The modulus and conjugate are fundamental concepts in complex number theory, providing insight into the geometric and algebraic properties of complex numbers. The modulus measures the distance of a complex number from the origin on the complex plane, while the conjugate reflects the number across the real axis, changing the sign of the imaginary part. These concepts are crucial for operations involving complex numbers, including simplification and finding solutions to complex equations.
Understanding complex numbers can dramatically enhance students’ mathematical abilities, providing them with the tools to tackle a wide range of problems. These examples demonstrate the application of complex numbers in various scenarios, reinforcing their importance and versatility in mathematical contexts.
Question: Add the complex numbers 3+2i and 1+4i.
Solution:
Question: Find the conjugate of the complex number 5−3i.
Solution:
Question: Multiply 2+3i by 4−i.
Solution:
Question: Divide 10+6i by 2+4i.
Solution:
Question: Find the magnitude of 3+4i.
Solution:
Complex numbers, bridging real and imaginary realms, are pivotal for advancing students’ mathematical understanding. This article has unveiled their intricacies, from basic operations to profound concepts like magnitude and conjugation. Empowering educators with practical examples, it aims to demystify complex numbers, making them accessible and fascinating for learners. Embracing these concepts can significantly enhance problem-solving skills and mathematical fluency among students.

Complex Numbers a cornerstone concept in advanced mathematics. This guide offers a lucid introduction, tailored for educators and students, showcasing how complex numbers combine real and imaginary units to solve equations that real numbers alone cannot. Through practical examples and straightforward explanations, we aim to demystify complex numbers, making them accessible and captivating. Whether for academic curiosity or classroom instruction, this guide serves as an invaluable resource for exploring the intriguing world of complex mathematics.
Complex numbers are a sophisticated class of numbers that extend the traditional number line into a two-dimensional plane by incorporating both a real part and an imaginary part. This unique structure allows for the representation and solution of equations that no real number can solve. Essential in various fields of science and engineering, understanding complex numbers enriches one’s mathematical toolkit, opening doors to advanced topics in algebra, physics, and beyond.
A quintessential example of a complex number is the solution to the equation X2 = -1, which is X = √-1, , or i, where i is the imaginary unit. This forms the basis for complex numbers like a + bi where a and b are real numbers. Complex numbers such as 3 + 4i illustrate how they enable calculations and representations not possible with real numbers alone, showcasing their utility in mathematical and practical applications.
The formula for complex numbers, a + bi, combines a real number a and an imaginary number b multiplied by i, (where i2 = -1). This elegant formula lays the foundation for complex arithmetic, enabling operations like addition, subtraction, multiplication, and division in the complex plane. It’s a gateway to understanding the dynamic interactions between real and imaginary components, crucial for advanced mathematics, physics, and engineering.
The unit of complex numbers, known as the imaginary unit i, is the cornerstone of complex number theory. Defined by the property that i2=-1,it facilitates the expression of square roots of negative numbers, a concept not possible in the realm of real numbers. This unit extends our mathematical horizons, allowing for the exploration of phenomena in quantum mechanics, electrical engineering, and signal processing with unparalleled depth and clarity.
Real numbers encompass the entire spectrum of numbers used in mathematics, including all the rational and irrational numbers. They can be found on the number line, stretching infinitely in both positive and negative directions. This set includes everyday numbers used for counting, measuring, and ordering, making it fundamental in both abstract mathematical theories and real-world applications. Understanding real numbers is essential for grasping the full scope of mathematics, from basic arithmetic to complex analysis.
Notation in mathematics serves as a standardized language, allowing mathematicians and students to communicate complex ideas succinctly and precisely. This includes symbols for operations, functions, sets, and numbers. Effective notation is critical for understanding and conveying mathematical concepts, facilitating learning, problem-solving, and research. It forms the backbone of mathematical clarity and efficiency, from elementary education to advanced theoretical work.
Imaginary numbers are a class of numbers that are defined as multiples of the square root of -1, denoted as i. They cannot be found on the traditional number line and are crucial for solving equations that have no solution within the realm of real numbers. Imaginary numbers expand the scope of solutions available for quadratic equations and beyond, playing a key role in advanced mathematics, physics, and engineering.
To clearly understand the distinctions between complex numbers, real numbers, and imaginary numbers, let’s look at their definitions and characteristics in a structured table format:
Feature | Complex Numbers | Real Numbers | Imaginary Numbers |
|---|---|---|---|
Definition | Numbers that combine real and imaginary parts. | Numbers that can be found on the number line. | Numbers that are multiples of the imaginary unit i, where i2=-1. |
Example | 3 + 4i | 5 | 2i |
Representation | Expressed as a+bi where a is the real part and b is the imaginary part. | Directly represented as a point on the number line. | Expressed as bi where b is a real number. |
Operational Base | Can be used in all forms of mathematical operations, extending the possibilities of calculations. | Limited to operations within the real number system. | Primarily used in operations involving square roots of negative numbers. |
Practical Usage | Essential in fields requiring multidimensional analysis, like electrical engineering and quantum physics. | Used in everyday counting, measuring, and ordering. | Integral in complex number theory and certain aspects of wave mechanics. |
Yes, 0 is considered a complex number. It can be represented as 0+0i, where both the real and imaginary parts are zero. This highlights the inclusivity of the complex number system, encapsulating real numbers and providing a foundation for broader mathematical concepts.
Graphical representations bring abstract concepts to life. Here are five distinct examples related to the graphical representation of numbers:
Complex Plane:
Explanation: Complex numbers are graphically represented on the complex plane, with the x-axis representing the real part and the y-axis representing the imaginary part.
Example: The complex number 3+4i is plotted 3 units along the x-axis (real axis) and 4 units along the y-axis (imaginary axis).
Number Line:
Explanation: Real numbers are represented on a one-dimensional number line, showing a linear sequence of numbers.
Example: The number 5 is located five units to the right of the origin on the number line.
Imaginary Axis:
Explanation: Imaginary numbers are represented along the imaginary axis (y-axis) in the complex plane.
Example: The imaginary number 2i is plotted 2 units up along the imaginary axis.
Origin Representation:
Explanation: The number 0, as a complex number (0+0i), is represented at the origin of the complex plane, where the real and imaginary axes intersect.
Example: This signifies the point of intersection, indicating the absence of both real and imaginary components.
Vectors in Complex Plane:
Explanation: Complex numbers can also be represented as vectors from the origin to the point (a,b) in the complex plane.
Example: The complex number 3+4i forms a vector from the origin to the point (3, 4), illustrating its magnitude and direction in the complex plane.
These examples underscore the diverse ways in which numbers can be graphically represented, each offering unique insights into their properties and relationships.
The absolute value of a number represents its distance from zero on the number line, irrespective of direction. This concept is fundamental in mathematics, providing the groundwork for understanding magnitude and comparison among numbers. It’s crucial in various fields, including engineering and physics, where the size of a quantity matters more than its direction.
Examples:
Absolute Value of -5:
Explanation: The absolute value of -5 is 5, demonstrating how negative numbers are converted to positive, reflecting distance from zero.
Absolute Value of 3:
Explanation: The absolute value of 3 is 3, illustrating that positive numbers remain unchanged, as they already denote distance from zero positively.
Absolute Value of 0:
Explanation: The absolute value of 0 is 0, showing that zero is neutral, having no distance from itself.
Absolute Value in Equations:
Explanation: x−4∣=2 leads to two possible solutions, x=6 or x=2, showcasing the absolute value’s role in solving equations.
Absolute Value Function:
Explanation: The graph of y=∣x∣ is a V shape, indicating that all output values are positive or zero, visualizing the concept of absolute magnitude.
Algebraic operations on complex numbers expand the realm of mathematics by enabling calculations involving both real and imaginary parts. These operations include addition, subtraction, multiplication, and division, essential for solving complex equations in advanced mathematics, physics, and engineering.
Examples:
Addition: (3 + 4i) + (5 + 2i):
Explanation: Results in 8 + 6i, showing how real parts are added together and imaginary parts are added together.
Subtraction: (6 + 3i) – (2 + 5i):
Explanation: Results in 4 – 2i, demonstrating subtraction of real and imaginary components individually.
Multiplication: (2 + 3i) * (1 + 4i):
Explanation: Yields -10 + 11i, illustrating the distributive property and the fact that i2=−1.
Division: 1+1/1−i:
Explanation: Simplifies to i, showcasing the use of conjugates to divide complex numbers.
Complex Conjugation:
Explanation: The conjugate of 3+4i is 3−4i, useful in various operations including division.
Roots of complex numbers involve finding numbers that, when raised to a certain power, yield the original complex number. This concept is a cornerstone of complex analysis and plays a vital role in understanding the geometry of complex numbers and solving polynomial equations.
Examples:
Square Root of -1:
Explanation: Defined as i, which forms the basis for all complex numbers, demonstrating how roots extend beyond real numbers.
Cube Roots of 8:
Explanation: Include 22, showing real root, and two complex roots, highlighting the multifaceted nature of complex roots.
Fourth Roots of 16:
Explanation: Comprise 2, −2, 2i, and −2i, illustrating that complex numbers can have both real and imaginary roots.
Roots of Unity:
Explanation: For nth roots, such as e2πi, showcase the cyclical nature of complex roots on the complex plane.
De Moivre’s Theorem:
Explanation: Useful for finding nth roots of complex numbers, showing how trigonometric identities apply in the complex domain.
Complex number formulas facilitate operations involving complex numbers, each embodying a mixture of real and imaginary parts. These formulas are pivotal in extending our ability to perform addition, subtraction, multiplication, and division beyond the realm of real numbers, enabling intricate calculations in fields like engineering, physics, and applied mathematics.
Examples:
Addition (a + bi) + (c + di):
Explanation: To add two complex numbers, add their real parts and imaginary parts separately. For example, (3 + 2i) + (1 + 4i) = 4 + 6i.
Subtraction (a + bi) – (c + di):
Explanation: Similar to addition, subtract the real and imaginary parts separately. For instance, (5 + 3i) – (2 + i) = 3 + 2i.
Multiplication (a + bi)(c + di):
Explanation: Multiply the complex numbers using distribution, remembering that i2 = -1 For example, (3 + 2i)(1 + 4i) = -5 + 14i.
Division a+bi/c+di:
Explanation: Multiply numerator and denominator by the conjugate of the denominator to rationalize. E.g., 3+2i/1+4i = 11/17 – 10/17i
Conjugate (a + bi) and (a – bi):
Explanation: The conjugate is used in division to simplify complex numbers. For (3 + 4i), its conjugate is (3 – 4i).
The power of iota (i) is fundamental in complex numbers, cycling through four key values : i0 = 1, i1 = i, i2= -1, i3= -1, and i4 = -1 then repeating. This cyclic nature simplifies calculations involving powers of i by reducing them to one of these four outcomes.
Explanation: The first power of i is itself, serving as the basis for defining complex numbers.
Explanation: This fundamental identity underpins the square root of a negative number, defining the imaginary unit.
Explanation: Following the cycle, i3 returns the negative of the imaginary unit, further showcasing the cyclic nature.
Explanation: Completing the cycle, i4 resets back to 1, illustrating the periodicity in powers of i.
Explanation: Powers of i repeat every 4 cycles. Since 17 mod 4 = 1, i17= -1 simplifies to i.
Identities of complex numbers provide foundational truths that facilitate their manipulation and understanding. These identities are instrumental in solving complex equations and performing advanced operations in mathematics and physics.
Examples:
Complex Conjugate Identity:
Explanation: For any complex number a+bi, its conjugate a−bi multiplied together gives a2+b2, a real number.
Modulus of a Complex Number:
Explanation: The modulus ∣a+bi∣ equals a2+b2, representing the distance from the origin in the complex plane.
Additive Identity:
Explanation: Adding 0 to any complex number a+bi leaves it unchanged, demonstrating the additive identity: a+bi+0=a+bi.
Multiplicative Identity:
Explanation: Multiplying any complex number by 1 maintains its value, showcasing the multiplicative identity: (a+bi)⋅1=a+bi.
These examples and descriptions showcase the vast and intricate landscape of complex numbers, revealing their beauty and utility in mathematical explorations.
Complex numbers possess unique properties that set them apart in the mathematical world, facilitating their manipulation and application across various fields. These properties include their addition, subtraction, multiplication, and division rules, which maintain the integrity of complex numbers and enable complex equations’ solutions. Understanding these properties is essential for anyone delving into higher mathematics, physics, or engineering, as they lay the groundwork for advanced concepts in these disciplines.
Addition Property:
Explanation: When adding complex numbers, add their real parts and imaginary parts separately. For instance, (3 + 2i) + (1 + 4i) = 4 + 6i.
Multiplication Property:
Explanation: Multiplying complex numbers involves distributing each part of the first number by each part of the second. For example, (2 + 3i)(1 + 2i) = 2 + 7i + 6i² = 2 + 7i – 6.
Conjugate Property:
Explanation: The conjugate of a complex number reverses the sign of the imaginary part, which is useful in division. If z = a + bi, then the conjugate, ˉzˉ, is a – bi.
Modulus Property:
Explanation: The modulus of a complex number represents its distance from the origin in the complex plane, calculated as 2∣z∣=a2+b2 for z = a + bi.
Distributive Property:
Explanation: Complex numbers follow the distributive law, allowing for the multiplication of a complex number by a sum of complex numbers, akin to real numbers.
The modulus and conjugate are fundamental concepts in complex number theory, providing insight into the geometric and algebraic properties of complex numbers. The modulus measures the distance of a complex number from the origin on the complex plane, while the conjugate reflects the number across the real axis, changing the sign of the imaginary part. These concepts are crucial for operations involving complex numbers, including simplification and finding solutions to complex equations.
Modulus of 3 + 4i:
Explanation: The modulus is √32+42=5, representing the distance from the origin to the point (3,4) on the complex plane.
Conjugate of 3 + 4i:
Explanation: The conjugate is 3 – 4i, which mirrors the complex number across the real axis on the complex plane.
Modulus in Polar Form:
Explanation: The modulus serves as the radius in polar coordinates, directly influencing the complex number’s representation in polar form.
Conjugate for Division:
Explanation: Using the conjugate in division helps eliminate the imaginary part in the denominator, simplifying the expression.
Physical Applications:
Explanation: In physics, the modulus represents magnitude, and the conjugate plays a role in wave functions and signal processing.
Understanding complex numbers can dramatically enhance students’ mathematical abilities, providing them with the tools to tackle a wide range of problems. These examples demonstrate the application of complex numbers in various scenarios, reinforcing their importance and versatility in mathematical contexts.
Question: Add the complex numbers 3+2i and 1+4i.
Solution:
Step 1: Add the real parts: 3+1=4.
Step 2: Add the imaginary parts: 2i+4i=6i.
Result: The sum is 4+6i.
Question: Find the conjugate of the complex number 5−3i.
Solution:
Step: The conjugate of a complex number changes the sign of the imaginary part.
Result: The conjugate is 5+3i.
Question: Multiply 2+3i by 4−i.
Solution:
Step 1: Apply the distributive property: 2∗4+2∗(−i)+3i∗4+3i∗(−i).
Step 2: Simplify: 8−2i+12i−3.
Result: The product is 5+10i.
Question: Divide 10+6i by 2+4i.
Solution:
Step 1: Multiply numerator and denominator by the conjugate of the denominator: (10+6i)∗(2−4i)/(2+4i)∗(2−4i).
Step 2: Simplify and calculate: 20−40i+12i+24/4+16.
Result: The quotient is 11−7i.
Question: Find the magnitude of 3+4i.
Solution:
Step: Use the formula a2+b2 where a = 3 and b = 4
Result: The magnitude is 5.
Complex numbers, bridging real and imaginary realms, are pivotal for advancing students’ mathematical understanding. This article has unveiled their intricacies, from basic operations to profound concepts like magnitude and conjugation. Empowering educators with practical examples, it aims to demystify complex numbers, making them accessible and fascinating for learners. Embracing these concepts can significantly enhance problem-solving skills and mathematical fluency among students.
Text prompt
Add Tone
Complex Number Formulas
Algebraic Operations on Complex Numbers
What is the value of i2?
-1
1
0
-i
Which of the following is the complex conjugate of 3+4i?
3 - 4i
-3 + 4i
3 + 4i
-3 - 4i
What is the result of (2+3i)+(4−i)?
8 + 2i
6 + 4i
6 + 2i
8 + 4i
Compute (1+2i)⋅(3−4i).
11 + 2i
11 - 2i
11 + 10i
-5 - 10i
What is the magnitude of the complex number 3−4i?
3
4
5
6
Which complex number is purely imaginary?
2 + 5i
4
-3
0 + 7i
What is the result of dividing 6+2i by 3−i?
2 + 2i
2 + i
3 + i
4 + 2i
Find the argument of the complex number −1+i.
3π4
π2
π
π4
What is the result of (5+2i)−(3−4i)?
8 - 2i
2 - 6i
2 + 6i
8 + 6i
What is i4?
1
-1
i
-i
Before you leave, take our quick quiz to enhance your learning!

