Divisibility Rule of 7 – Examples, Proof, Methods, What is Divisibility Rule of 7

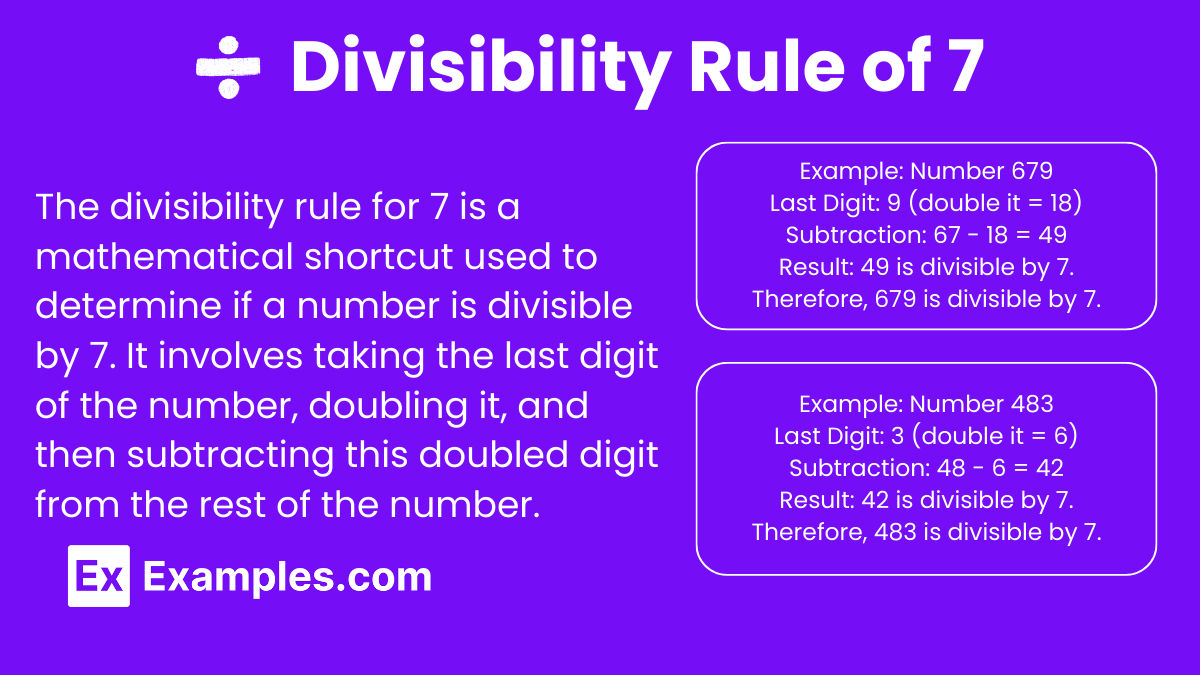
The divisibility rule for 7, while less straightforward than rules for smaller numbers, is a useful mathematical tool for identifying divisibility within the integer subset of rational number. This rule involves taking the last digit of the number, doubling it, and then subtracting this product from the remaining leading truncated number. Repeating this process as needed can indicate divisibility by 7 when the resulting smaller number is known to be divisible by 7. In algebra and arithmetic, mastering this rule enhances one’s ability to simplify and factorize expressions and solve equations efficiently. The rule applies specifically to integers, aiding significantly in tasks involving addition, subtraction, multiplication, and division.

Download Proof of Divisibility Rule of 7 in PDF

What is Divisibility Rule of 7?

Proof of Divisibility Rule of 7

Download Proof of Divisibility Rule of 7 in PDF

To prove the divisibility rule for 7 using a specific number, let’s use the number 352. Here’s the step-by-step explanation of how the rule applies to this number:

Step 1: Consider the number 352.

Step 2: Apply the rule for divisibility by 7:

To use the rule, take the last digit of the number, double it, and subtract this product from the rest of the number.

Step 3: Separate the last digit from the rest:

352→35 and 2

Step 4: Double the last digit:

2×2 = 4

Step 5: Subtract the doubled digit from the remaining number:

35−4 = 31

Step 6: Check the result:

Now, determine if 31 is divisible by 7. Since 31 is not divisible by 7, we repeat the process if necessary or directly assess the smaller number.

Step 7: Apply the rule again if unclear (not needed in this example):

For simplicity, we can state that since 31 is not divisible by 7, it is clear from this first step. Alternatively, further application could be made by repeating steps with smaller results from further operations, but it’s evident here.

Step 8: Conclusion:

Since the result, 31, is not divisible by 7, the original number 352 is also not divisible by 7.

Divisibility Rule of 7 and 11

Divisibility Rule for 7

• Take the last digit of the number, double it.
• Subtract the doubled digit from the rest of the number.
• If the result is 0 or divisible by 7, then the original number is divisible by 7.
• If the result is still large or unclear, repeat the process with the new number.

Example:

For the number 203:

• Last digit is 3, doubled is 6.
• Subtract 6 from 20, which gives 14.
• Since 14 is divisible by 7, 203 is also divisible by 7.

Divisibility Rule for 11

• Add and subtract the digits of the number in an alternating pattern.
• Start from the rightmost digit: add, then subtract the next, and so on.
• If the result is 0 or divisible by 11, then the original number is divisible by 11.

Example:

For the number 2728:

• Calculation: 8 – 2 + 7 – 2 = 11
• Since 11 is divisible by 11, 2728 is also divisible by 11.

Divisibility Rule of 7 and 13

Divisibility Rule for 7:

• Take the last digit of the number, double it.
• Subtract the doubled digit from the rest of the number.
• If the resulting number is 0 or divisible by 7, then the original number is divisible by 7.
• If the result is still large, repeat the process with the new number until you can easily determine its divisibility.

Example:

Consider the number 161:

• Last digit is 1, doubled is 2.
• Subtract 2 from 16, which gives 14.
• Since 14 is divisible by 7, 161 is also divisible by 7.

Divisibility Rule for 13:

• Take the last digit of the number, multiply it by 4.
• Add this product to the rest of the number.
• If the resulting number is 0 or divisible by 13, then the original number is divisible by 13.
• If the result is still large, repeat the process with the new number until you can easily determine its divisibility.

Example:

Consider the number 455:

• Last digit is 5, multiplied by 4 is 20.
• Add 20 to 45, which gives 65.
• Since 65 is divisible by 13 (65 ÷ 13 = 5), 455 is also divisible by 13.

Divisibility Rule of 7 Examples

Number 203

• Step 1: Take the last digit (3) and double it (3 x 2 = 6).
• Step 2: Subtract the doubled digit (6) from the rest of the number (20 – 6 = 14).
• Step 3: Since 14 is divisible by 7, the original number (203) is also divisible by 7.

Number 352

• Step 1: Take the last digit (2) and double it (2 x 2 = 4).
• Step 2: Subtract the doubled digit (4) from the rest of the number (35 – 4 = 31).
• Step 3: Since 31 is not divisible by 7, the number 352 is not divisible by 7.

Number 1617

• Step 1: Take the last digit (7) and double it (7 x 2 = 14).
• Step 2: Subtract the doubled digit (14) from the rest of the number (161 – 14 = 147).
• Step 3: Check if the result (147) is divisible by 7. It is, since 147 ÷ 7 = 21.
• Step 4: Since 147 is divisible by 7, the original number (1617) is also divisible by 7.

Number 994

• Step 1: Take the last digit (4) and double it (4 x 2 = 8).
• Step 2: Subtract the doubled digit (8) from the rest of the number (99 – 8 = 91).
• Step 3: Check if the result (91) is divisible by 7. It is, since 91 ÷ 7 = 13.
• Step 4: Since 91 is divisible by 7, the original number (994) is also divisible by 7.

FAQs