What is the main characteristic of particles that obey Bose-Einstein statistics?
They are fermions.
They obey the Pauli exclusion principle.
They are bosons.
They cannot occupy the same quantum state.

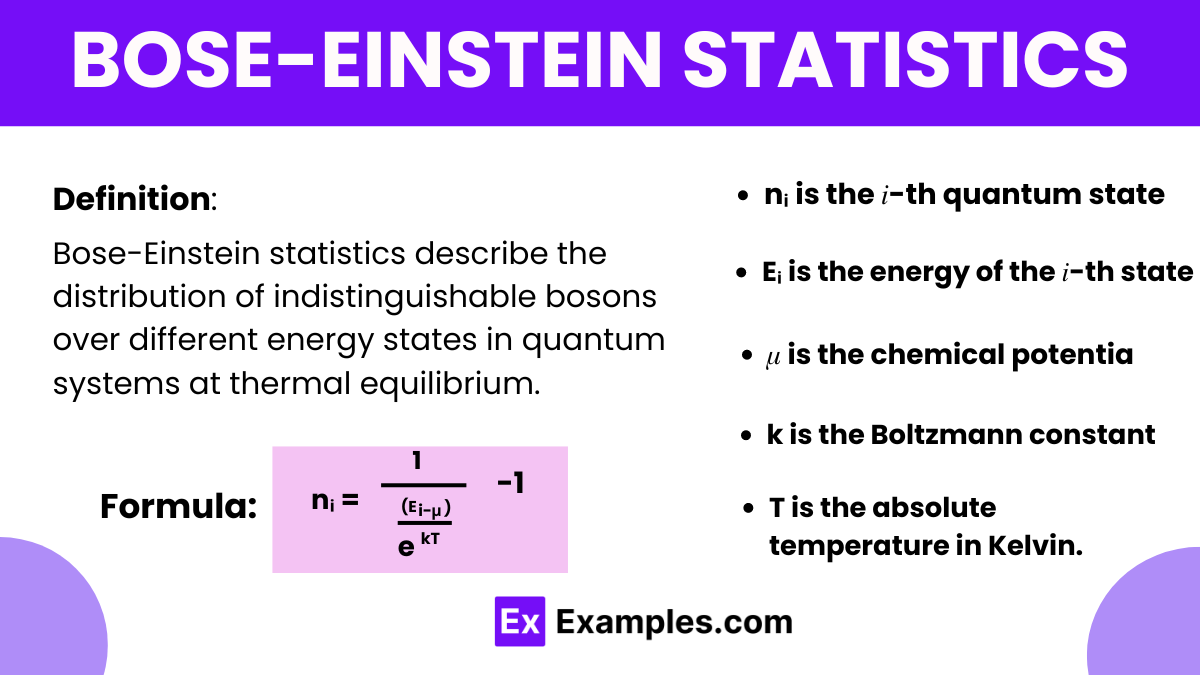
Bose-Einstein statistics describe the distribution of identical particles with integer spin, known as bosons, under quantum mechanics. This statistical model, developed by Satyendra Nath Bose and Albert Einstein, predicts how bosons congregate in the same quantum state, particularly at low temperatures. Unlike particles described by Fermi-Dirac statistics, bosons do not adhere to the Pauli exclusion principle, allowing phenomena like superfluidity and superconductivity. This framework is a cornerstone in the broader field of physics, integrating fundamental laws of mechanics and quantum principles to explain complex material behaviors and quantum phenomena.
Scenario: Consider a blackbody radiation scenario where photons (which are bosons) are emitted by an object at a temperature 𝑇.
Application: The Bose-Einstein distribution is used to model the distribution of photon energies in the cavity. Since photons do not have a chemical potential (𝜇=0).
This formula predicts the number of photons at each energy level, which is essential for understanding the spectral distribution of the blackbody radiation.
Scenario: Helium-4 atoms at temperatures near absolute zero behave as bosons and can undergo Bose-Einstein condensation, leading to superfluidity.
Application: For Helium-4, the Bose-Einstein statistics can be used to predict the fraction of atoms that condense into the ground state as the temperature approaches zero. At very low temperatures, a significant number of atoms accumulate in the lowest available energy state, leading to the phenomenon of superfluidity.
Scenario: In laboratory conditions, atoms like rubidium or sodium are cooled to temperatures just a few nanokelvin above absolute zero under high-vacuum conditions.
Application: Experimental physicists use the Bose-Einstein formula to estimate and observe the formation of a Bose-Einstein condensate. As the temperature drops, more atoms fall into the lowest energy state, observable as a peak in the spatial density distribution of the atoms.
Bosons, the particles governed by Bose-Einstein statistics, are characterized by their integer spin values. This quantum mechanical property allows multiple bosons to occupy the same quantum state, unlike fermions which are subject to the Pauli exclusion principle due to their half-integer spins.
In quantum mechanics, particles like bosons are considered indistinguishable from one another when they are in the same quantum state. This indistinguishability is a key aspect that influences the statistical distribution of bosons. In Bose-Einstein statistics, the probability of a boson occupying a particular energy state is not influenced by the presence of other bosons in that state.
A significant quantum phenomenon described by Bose-Einstein statistics is the Bose-Einstein condensate (BEC). This state of matter occurs when bosons are cooled to temperatures near absolute zero, causing a large fraction of the bosons to collapse into the lowest available energy state, forming a single quantum state. This condensate is a macroscopic quantum state where quantum effects like coherence and superfluidity can be observed at a larger scale.
The properties of Bose-Einstein condensates demonstrate several macroscopic quantum phenomena:
The development of Bose-Einstein statistics and the subsequent discovery of Bose-Einstein condensates have had profound implications for theoretical physics, providing deep insights into quantum field theory, particle physics, and the behavior of quantum fluids. These studies also bridge some aspects of condensed matter physics and quantum mechanics, highlighting the versatility and foundational nature of quantum theory in modern physics.
Overall, the “quantum” in Bose-Einstein statistics is not just about small scales but is fundamentally about how quantum mechanics dictates the behavior of particles at very low temperatures, leading to new states of matter and exotic physical phenomena.
Bosons are unique because they do not obey the Pauli Exclusion Principle, which states that no two fermions (particles with half-integer spins) can occupy the same quantum state simultaneously. Bosons, however, can occupy the same quantum state in large numbers, leading to phenomena such as Bose-Einstein condensation.
Bose-Einstein condensation was first predicted theoretically by Albert Einstein in 1924 based on Satyendra Nath Bose’s work on the statistical mechanics of photons. The first experimental observation of BEC occurred in 1995 with ultracold rubidium and sodium atoms, a discovery that was awarded the Nobel Prize in Physics in 2001.
Bose-Einstein statistics are fundamental to understanding various quantum phenomena that play significant roles in theoretical physics, including the behaviors of particles at extremely low temperatures and the quantum nature of large-scale phenomena like superconductivity and superfluidity.

Bose-Einstein statistics describe the distribution of identical particles with integer spin, known as bosons, under quantum mechanics. This statistical model, developed by Satyendra Nath Bose and Albert Einstein, predicts how bosons congregate in the same quantum state, particularly at low temperatures. Unlike particles described by Fermi-Dirac statistics, bosons do not adhere to the Pauli exclusion principle, allowing phenomena like superfluidity and superconductivity. This framework is a cornerstone in the broader field of physics, integrating fundamental laws of mechanics and quantum principles to explain complex material behaviors and quantum phenomena.
Bose-Einstein statistics is a branch of quantum statistics that applies to particles known as bosons, which are particles with integer spin values (like 0, 1, 2, etc.). This statistical model describes how these particles distribute themselves among various energy states at thermal equilibrium. A key characteristic of bosons, according to Bose-Einstein statistics, is that unlike fermions (which follow Fermi-Dirac statistics and are subject to the Pauli exclusion principle), multiple bosons can occupy the same quantum state. This ability leads to unique quantum phenomena such as Bose-Einstein condensation, where bosons at low temperatures and high densities occupy the lowest available energy state, resulting in macroscopic quantum phenomena.
Scenario: Consider a blackbody radiation scenario where photons (which are bosons) are emitted by an object at a temperature 𝑇.
Application: The Bose-Einstein distribution is used to model the distribution of photon energies in the cavity. Since photons do not have a chemical potential (𝜇=0).
This formula predicts the number of photons at each energy level, which is essential for understanding the spectral distribution of the blackbody radiation.
Scenario: Helium-4 atoms at temperatures near absolute zero behave as bosons and can undergo Bose-Einstein condensation, leading to superfluidity.
Application: For Helium-4, the Bose-Einstein statistics can be used to predict the fraction of atoms that condense into the ground state as the temperature approaches zero. At very low temperatures, a significant number of atoms accumulate in the lowest available energy state, leading to the phenomenon of superfluidity.
Scenario: In laboratory conditions, atoms like rubidium or sodium are cooled to temperatures just a few nanokelvin above absolute zero under high-vacuum conditions.
Application: Experimental physicists use the Bose-Einstein formula to estimate and observe the formation of a Bose-Einstein condensate. As the temperature drops, more atoms fall into the lowest energy state, observable as a peak in the spatial density distribution of the atoms.
Bosons, the particles governed by Bose-Einstein statistics, are characterized by their integer spin values. This quantum mechanical property allows multiple bosons to occupy the same quantum state, unlike fermions which are subject to the Pauli exclusion principle due to their half-integer spins.
In quantum mechanics, particles like bosons are considered indistinguishable from one another when they are in the same quantum state. This indistinguishability is a key aspect that influences the statistical distribution of bosons. In Bose-Einstein statistics, the probability of a boson occupying a particular energy state is not influenced by the presence of other bosons in that state.
A significant quantum phenomenon described by Bose-Einstein statistics is the Bose-Einstein condensate (BEC). This state of matter occurs when bosons are cooled to temperatures near absolute zero, causing a large fraction of the bosons to collapse into the lowest available energy state, forming a single quantum state. This condensate is a macroscopic quantum state where quantum effects like coherence and superfluidity can be observed at a larger scale.
The properties of Bose-Einstein condensates demonstrate several macroscopic quantum phenomena:
Superfluidity: The ability of the fluid to flow without viscosity, which can be observed in liquid helium.
Coherence: The particles in a BEC exhibit a high degree of quantum mechanical wave function overlap, leading to phenomena similar to those observed in laser light, such as interference.
The development of Bose-Einstein statistics and the subsequent discovery of Bose-Einstein condensates have had profound implications for theoretical physics, providing deep insights into quantum field theory, particle physics, and the behavior of quantum fluids. These studies also bridge some aspects of condensed matter physics and quantum mechanics, highlighting the versatility and foundational nature of quantum theory in modern physics.
Overall, the “quantum” in Bose-Einstein statistics is not just about small scales but is fundamentally about how quantum mechanics dictates the behavior of particles at very low temperatures, leading to new states of matter and exotic physical phenomena.
Superconductivity: Bose-Einstein statistics help explain the phenomenon of superconductivity, where certain materials can conduct electricity without resistance at very low temperatures. The theory suggests that pairs of electrons (known as Cooper pairs) can act as bosons and condense into a lower energy state that allows for resistance-free current flow.
Superfluidity: The study of liquid helium at temperatures close to absolute zero has demonstrated superfluid behavior, where the liquid flows with zero viscosity. This behavior is explained by Bose-Einstein condensation of helium-4 atoms, providing insights into fluid dynamics at quantum levels.
Quantum Computing: Bose-Einstein condensates are being explored as potential resources for quantum computing. The ability of these condensates to demonstrate macroscopic quantum phenomena makes them suitable for developing qubits and implementing quantum logic operations, which are fundamental for quantum computing.
Precision Measurement and Metrology: Instruments based on properties of Bose-Einstein condensates, such as atom interferometers, are highly sensitive to gravitational and magnetic fields, making them useful for precision measurements. These instruments are used in applications ranging from gravitational wave detection to earth science measurements and navigation.
Photonic Technologies: The principles of Bose-Einstein statistics are applicable in the development of photonic technologies, such as low-threshold lasers and other light sources based on Bose-Einstein condensation of exciton-polaritons in semiconductors. These technologies are vital for advancing optical systems and improving communication technologies.
Thermal Management: Understanding Bose-Einstein statistics has implications in thermal management technologies, particularly in designing systems that exploit superfluid properties for efficient heat transfer and management in electronic and photonic devices.
Fundamental Physics Research: Bose-Einstein condensates provide a controlled environment for studying quantum mechanical properties on macroscopic scales. This has applications in testing fundamental theories of physics, studying matter under extreme conditions, and exploring the intersection of quantum mechanics and general relativity.
Bosons are unique because they do not obey the Pauli Exclusion Principle, which states that no two fermions (particles with half-integer spins) can occupy the same quantum state simultaneously. Bosons, however, can occupy the same quantum state in large numbers, leading to phenomena such as Bose-Einstein condensation.
Bose-Einstein condensation was first predicted theoretically by Albert Einstein in 1924 based on Satyendra Nath Bose’s work on the statistical mechanics of photons. The first experimental observation of BEC occurred in 1995 with ultracold rubidium and sodium atoms, a discovery that was awarded the Nobel Prize in Physics in 2001.
Bose-Einstein statistics are fundamental to understanding various quantum phenomena that play significant roles in theoretical physics, including the behaviors of particles at extremely low temperatures and the quantum nature of large-scale phenomena like superconductivity and superfluidity.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the main characteristic of particles that obey Bose-Einstein statistics?
They are fermions.
They obey the Pauli exclusion principle.
They are bosons.
They cannot occupy the same quantum state.
Which of the following particles is an example of a boson?
Electron
Proton
Neutron
Photon
What phenomenon is explained by Bose-Einstein statistics?
Superconductivity
Blackbody radiation
Bose-Einstein condensation
Fermi gas behavior
At what temperature do Bose-Einstein condensates typically form?
Room temperature
Absolute zero
Below a critical temperature near absolute zero
At high temperatures
Which scientist, along with Albert Einstein, developed the theory of Bose-Einstein statistics?
Niels Bohr
Satyendra Nath Bose
Wolfgang Pauli
Erwin Schrodinger
What is the statistical distribution function for bosons called?
Fermi-Dirac distribution
Maxwell-Boltzmann distribution
Bose-Einstein distribution
Planck distribution
What is a key difference between Bose-Einstein and Fermi-Dirac statistics?
Only Bose-Einstein statistics apply to particles with half-integer spins.
Bose-Einstein statistics allow multiple particles to occupy the same quantum state.
Fermi-Dirac statistics apply to bosons.
Fermi-Dirac statistics allow multiple particles to occupy the same quantum state.
What happens to the chemical potential μ of a Bose gas as the temperature approaches absolute zero?
It increases indefinitely.
It decreases indefinitely.
It approaches zero.
It becomes negative infinity.
In the context of Bose-Einstein statistics, what is degeneracy pressure?
The pressure exerted by particles that obey Fermi-Dirac statistics.
The pressure exerted by particles that obey Maxwell-Boltzmann statistics.
The pressure exerted by bosons in a Bose-Einstein condensate.
The pressure due to the Pauli exclusion principle.
Which physical property of a particle determines whether it obeys Bose-Einstein or Fermi-Dirac statistics?
Mass
Charge
Spin
Energy
Before you leave, take our quick quiz to enhance your learning!

