Which of the following is a scalar quantity?
Velocity
Force
Temperature
Displacement

Scalars and vectors are fundamental concepts in physics that describe different types of quantities. Scalars are quantities that are fully described by a magnitude (or numerical value) alone, such as temperature, mass, speed, and time. They have no direction associated with them. In contrast, vectors are quantities that have both a magnitude and a direction. Examples of vectors include displacement, velocity, acceleration, and force. Understanding the distinction between scalars and vectors is crucial for solving problems in physics, including vector algebra and determining the units of momentum and units of velocity, as vectors require consideration of both their magnitude and direction for accurate representation and calculation.
Scalars and vectors are fundamental concepts in physics that describe different types of quantities. Scalars are quantities that have only magnitude, such as temperature, mass, and speed. They are completely described by a single numerical value and a unit. Vectors, however, have both magnitude and direction, making them more complex. Examples of vectors include velocity, force, and displacement.
Scalars are quantities that only have magnitude and no direction. They are described by a single value with units.
Speed is the rate at which an object covers distance. Here, ddd represents the total distance traveled, and ttt is the time taken. Speed is a scalar because it does not include any directional information.
In Einstein’s mass-energy equivalence formula, EEE represents energy, mmm is mass, and ccc is the speed of light in a vacuum (approximately 3×1083 \times 10^83×108 meters per second). This formula shows that mass can be converted into energy. Energy is a scalar quantity.
Vectors have both magnitude and direction. They are represented by arrows in diagrams, where the length of the arrow indicates the magnitude and the direction of the arrow shows the direction of the vector.
Displacement is the vector that shows the change in position of an object. 𝑟⃗1 is the initial position vector, 𝑟⃗2and is the final position vector. Displacement considers direction, making it a vector.
Velocity is the rate of change of displacement with time. Unlike speed, velocity is a vector because it includes direction. Here,𝑑⃗ is displacement and t is time.
According to Newton’s second law of motion, force is the product of mass and acceleration. Here, m is mass and 𝑎⃗ is acceleration. Force is a vector because it causes an object to move in a specific direction.

Scalar quantities are physical quantities that have only magnitude and no direction. They are completely described by a single numerical value and a unit. Common examples of scalar quantities include temperature, which can be measured in degrees Celsius or Fahrenheit; mass, measured in kilograms or grams; and speed, measured in meters per second or miles per hour. Other scalar quantities include time, energy, distance, volume, and density. Scalars are straightforward because they do not involve any directional component, making them easier to handle in calculations and everyday measurements.
Vector quantities, on the other hand, have both magnitude and direction, adding a layer of complexity to their description. Vectors are represented by arrows, where the length of the arrow indicates the magnitude and the arrowhead points in the direction. Examples of vector quantities include velocity, which specifies the speed of an object and the direction it is moving; force, which describes the push or pull on an object in a specific direction; and displacement, which indicates the change in position of an object from one point to another. Other vector quantities include acceleration, momentum, electric field, magnetic field, and weight. Understanding vector quantities is essential in physics, as they provide a more comprehensive description of many physical phenomena, requiring vector addition and subtraction for accurate analysis.
| Aspect | Scalar | Vector |
|---|---|---|
| Definition | A quantity with only magnitude. | A quantity with both magnitude and direction. |
| Examples | Temperature, mass, speed, time, energy | Velocity, force, displacement, acceleration |
| Representation | Represented by a single number and unit. | Represented by an arrow with length (magnitude) and direction. |
| Addition | Added using simple arithmetic. | Added using vector addition rules (parallelogram law or triangle method). |
| Subtraction | Subtracted using simple arithmetic. | Subtracted using vector subtraction rules. |
| Multiplication | Multiplied or divided by other scalars directly. | Multiplied by scalars to change magnitude but not direction. |
| Examples in Physics | Energy (Joules), distance (meters) | Force (Newtons), velocity (meters per second) |
Scalars and vectors each have distinct properties that are essential for understanding and solving physics problems.
A vector is represented by an arrow. The length represents the magnitude, and the arrow points in the direction.
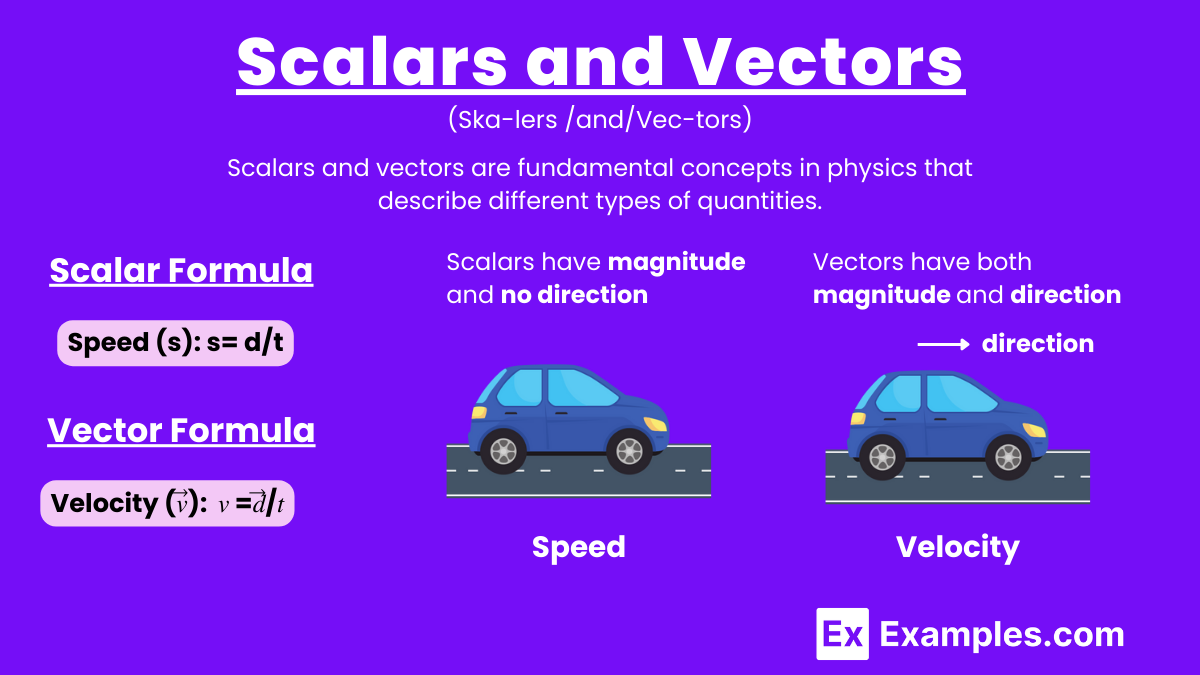
Speed is a scalar quantity, indicating how fast an object moves. Velocity is a vector, indicating speed with direction.
Vectors are added using the head-to-tail method or by breaking them into components and adding corresponding components.
The result is a vector whose magnitude is scaled by the scalar and direction remains unchanged.
A unit vector has a magnitude of one and indicates direction only. It is used to specify direction in vector operations.
No, a vector cannot have a negative magnitude, but its direction can be reversed.
The magnitude of a vector is found using the Pythagorean theorem for its components.
Distance is a scalar quantity, measuring the path length. Displacement is a vector, measuring the straight-line distance between initial and final points.
Vectors are used to represent quantities like force, acceleration, and velocity, which have both magnitude and direction.
Yes, vector subtraction is performed by adding the negative of the vector to be subtracted, using the head-to-tail method.

Scalars and vectors are fundamental concepts in physics that describe different types of quantities. Scalars are quantities that are fully described by a magnitude (or numerical value) alone, such as temperature, mass, speed, and time. They have no direction associated with them. In contrast, vectors are quantities that have both a magnitude and a direction. Examples of vectors include displacement, velocity, acceleration, and force. Understanding the distinction between scalars and vectors is crucial for solving problems in physics, including vector algebra and determining the units of momentum and units of velocity, as vectors require consideration of both their magnitude and direction for accurate representation and calculation.
Scalars and vectors are fundamental concepts in physics that describe different types of quantities. Scalars are quantities that have only magnitude, such as temperature, mass, and speed. They are completely described by a single numerical value and a unit. Vectors, however, have both magnitude and direction, making them more complex. Examples of vectors include velocity, force, and displacement.
Scalars are quantities that only have magnitude and no direction. They are described by a single value with units.
Speed (s): s= d/t
Speed is the rate at which an object covers distance. Here, ddd represents the total distance traveled, and ttt is the time taken. Speed is a scalar because it does not include any directional information.
Energy (E): E=mc2
In Einstein’s mass-energy equivalence formula, EEE represents energy, mmm is mass, and ccc is the speed of light in a vacuum (approximately 3×1083 \times 10^83×108 meters per second). This formula shows that mass can be converted into energy. Energy is a scalar quantity.
Vectors have both magnitude and direction. They are represented by arrows in diagrams, where the length of the arrow indicates the magnitude and the direction of the arrow shows the direction of the vector.
Displacement (𝑑⃗): 𝑑⃗ = 𝑟⃗2−𝑟⃗1
Displacement is the vector that shows the change in position of an object. 𝑟⃗1 is the initial position vector, 𝑟⃗2and is the final position vector. Displacement considers direction, making it a vector.
Velocity (𝑣⃗): 𝑣 =𝑑⃗/𝑡
Velocity is the rate of change of displacement with time. Unlike speed, velocity is a vector because it includes direction. Here,𝑑⃗ is displacement and t is time.
Force (𝐹⃗): 𝐹 = 𝑚𝑎⃗
According to Newton’s second law of motion, force is the product of mass and acceleration. Here, m is mass and 𝑎⃗ is acceleration. Force is a vector because it causes an object to move in a specific direction.

Temperature: Temperature is a scalar quantity that measures the average kinetic energy of particles in a substance. It is fully described by its magnitude, 25°C, without any directional component.
Mass : Mass represents the amount of matter in an object. It is a scalar quantity described by a single value, 10 kilograms, and does not depend on direction.
Speed : Speed is the rate at which an object covers distance. As a scalar, it only has magnitude, such as 60 km/h, and no directional information.
Distance : Distance is a scalar that measures the total path length traveled by an object. For example, 5 meters indicates how far an object has moved, without specifying direction.
Energy : Energy quantifies the capacity to do work. It is a scalar quantity; 100 Joules of energy can be transferred or transformed without a direction.
Time : Time is a scalar representing the duration of an event. Three seconds indicate the length of time elapsed, independent of direction.
Volume : Volume measures the amount of space occupied by an object. It is a scalar quantity, such as 2 liters, with only magnitude.
Density : Density is the mass per unit volume of a substance. As a scalar, it is described by its magnitude, 1.2 g/cm³, without direction.
Pressure : Pressure is the force exerted per unit area. It is a scalar quantity; 101.3 kPa indicates the magnitude of pressure applied uniformly in all directions.
Work : Work measures the energy transferred by a force over a distance. It is a scalar quantity, with 500 Joules representing the magnitude of work done.
Power : Power is the rate at which work is done or energy is transferred. As a scalar, 60 Watts quantifies the magnitude of power without direction.
Charge : Charge represents the quantity of electricity. It is a scalar quantity, such as 1.6 x 10⁻¹⁹ Coulombs, with only magnitude.
Luminous Intensity : Luminous intensity measures the brightness of a light source. It is a scalar quantity, with 100 Candela indicating its magnitude.
Electric Potential : Electric potential represents the electric potential energy per unit charge. As a scalar, 12 Volts describes its magnitude without direction.
Frequency : Frequency measures the number of cycles per second in a periodic event. It is a scalar quantity; 50 Hertz indicates its magnitude.
Velocity : Velocity is a vector quantity that includes both speed and direction. For example, 50 km/h north specifies the magnitude of speed and the direction of motion.
Force : Force is a vector quantity characterized by its magnitude and direction. Twenty Newtons upwards indicates the strength and direction of the force applied.
Displacement : Displacement measures the change in position of an object. It is a vector quantity, with 10 meters east specifying both the distance and direction from the starting point.
Acceleration : Acceleration is a vector that describes the rate of change of velocity. For example, 9.8 m/s² downwards indicates both the magnitude and the direction of acceleration due to gravity.
Momentum : Momentum is a vector quantity that combines mass and velocity. Fifteen kg·m/s at 30° to the horizontal indicates its magnitude and direction.
Electric Field : The electric field is a vector that represents the force per unit charge. For instance, 100 N/C to the right specifies both the strength and direction of the electric field.
Magnetic Field : The magnetic field is a vector quantity that describes the magnetic influence. For example, 0.5 Tesla upwards indicates the magnitude and direction of the field.
Torque : Torque is a vector quantity representing the rotational force. Thirty Newton-meters clockwise specifies its magnitude and rotational direction.
Gravitational Field : The gravitational field is a vector that measures the gravitational force per unit mass. For example, 9.8 N/kg downwards indicates the strength and direction of gravity.
Impulse : Impulse is a vector quantity representing the change in momentum. Two hundred N·s north specifies both the magnitude and direction of the applied force.
Current Density : Current density is a vector that describes the electric current per unit area. For example, 3 A/m² to the right indicates both the magnitude and direction of the current flow.
Angular Momentum : Angular momentum is a vector quantity describing rotational motion. Five kg·m²/s counterclockwise specifies its magnitude and rotational direction.
Linear Momentum : Linear momentum is a vector that combines mass and velocity. For instance, 25 kg·m/s west indicates its magnitude and direction.
Stress : Stress is a vector quantity representing force per unit area within materials. Forty N/m² upwards indicates the magnitude and direction of the internal force.
Position : Position is a vector that specifies the location of an object. For example, 3 meters southeast indicates both the distance and direction from a reference point.
Scalar quantities are physical quantities that have only magnitude and no direction. They are completely described by a single numerical value and a unit. Common examples of scalar quantities include temperature, which can be measured in degrees Celsius or Fahrenheit; mass, measured in kilograms or grams; and speed, measured in meters per second or miles per hour. Other scalar quantities include time, energy, distance, volume, and density. Scalars are straightforward because they do not involve any directional component, making them easier to handle in calculations and everyday measurements.
Vector quantities, on the other hand, have both magnitude and direction, adding a layer of complexity to their description. Vectors are represented by arrows, where the length of the arrow indicates the magnitude and the arrowhead points in the direction. Examples of vector quantities include velocity, which specifies the speed of an object and the direction it is moving; force, which describes the push or pull on an object in a specific direction; and displacement, which indicates the change in position of an object from one point to another. Other vector quantities include acceleration, momentum, electric field, magnetic field, and weight. Understanding vector quantities is essential in physics, as they provide a more comprehensive description of many physical phenomena, requiring vector addition and subtraction for accurate analysis.
Aspect | Scalar | Vector |
|---|---|---|
Definition | A quantity with only magnitude. | A quantity with both magnitude and direction. |
Examples | Temperature, mass, speed, time, energy | Velocity, force, displacement, acceleration |
Representation | Represented by a single number and unit. | Represented by an arrow with length (magnitude) and direction. |
Addition | Added using simple arithmetic. | Added using vector addition rules (parallelogram law or triangle method). |
Subtraction | Subtracted using simple arithmetic. | Subtracted using vector subtraction rules. |
Multiplication | Multiplied or divided by other scalars directly. | Multiplied by scalars to change magnitude but not direction. |
Examples in Physics | Energy (Joules), distance (meters) | Force (Newtons), velocity (meters per second) |
Scalars and vectors each have distinct properties that are essential for understanding and solving physics problems.
Magnitude Only: Scalars are described by a single numerical value, representing the magnitude.
No Direction: Scalars do not have any directional component.
Examples: Common scalar quantities include mass, temperature, speed, and time.
Addition/Subtraction: Scalars can be added or subtracted using simple arithmetic.
Units: Scalars have units that correspond to their physical quantity, such as kilograms for mass or seconds for time.
Magnitude and Direction: Vectors are described by both a magnitude and a direction.
Representation: Vectors are often represented graphically by arrows, where the length represents the magnitude and the arrowhead indicates the direction.
Examples: Common vector quantities include displacement, velocity, acceleration, and force.
Vector Algebra: Vectors follow specific rules for addition, subtraction, and multiplication, including the use of vector components and unit vectors.
Units of Momentum and Velocity: Vectors like momentum and velocity have specific units, such as kg·m/s for momentum and m/s for velocity.
Operations: Vector operations include the dot product and cross product, which have applications in physics to calculate work, torque, and other quantities.
A vector is represented by an arrow. The length represents the magnitude, and the arrow points in the direction.
Speed is a scalar quantity, indicating how fast an object moves. Velocity is a vector, indicating speed with direction.
Vectors are added using the head-to-tail method or by breaking them into components and adding corresponding components.
The result is a vector whose magnitude is scaled by the scalar and direction remains unchanged.
A unit vector has a magnitude of one and indicates direction only. It is used to specify direction in vector operations.
No, a vector cannot have a negative magnitude, but its direction can be reversed.
The magnitude of a vector is found using the Pythagorean theorem for its components.
Distance is a scalar quantity, measuring the path length. Displacement is a vector, measuring the straight-line distance between initial and final points.
Vectors are used to represent quantities like force, acceleration, and velocity, which have both magnitude and direction.
Yes, vector subtraction is performed by adding the negative of the vector to be subtracted, using the head-to-tail method.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which of the following is a scalar quantity?
Velocity
Force
Temperature
Displacement
What is a vector quantity?
A quantity with magnitude only
A quantity with magnitude and direction
A quantity that is always positive
A quantity that cannot be measured
Which of the following represents a vector quantity?
Mass
Speed
Distance
Acceleration
When two vectors are added together, what is the resulting vector called?
The sum vector
The resultant vector
The difference vector
The component vector
Which of the following operations is not valid for vectors?
Addition
Subtraction
Multiplication by a scalar
Division by a vector
In vector addition, what method can be used to find the resultant vector if two vectors are perpendicular to each other?
Triangle method
Parallelogram method
Pythagorean theorem
Dot product
If a vector A has a magnitude of 5 units and is directed 30 degrees above the horizontal, what is the horizontal component of the vector?
5 units
4.33 units
2.5 units
5√3 units
Which of the following statements about scalar and vector quantities is true?
Scalars can be added only to other scalars
Vectors cannot be multiplied by scalars
Vectors are always positive
Scalars can be subtracted from other scalars
Which operation can be performed on vectors to determine the angle between them?
Scalar multiplication
Dot product
Cross product
Scalar addition
What is the result of the vector subtraction A - B if A and B are vectors of equal magnitude and opposite directions?
Zero vector
Vector A
Vector B
A vector with double the magnitude of A or B
Before you leave, take our quick quiz to enhance your learning!

