Which of the following is Gauss's Law for electricity?
∇·E = ρ/ε₀
∇×E = -∂B/∂t
∇·B = 0
∇×B = μ₀J + μ₀ε₀∂E/∂t

The first equation, known as Gauss’s Law for Electricity, asserts that the electric flux through a closed surface is proportional to the charge enclosed by that surface. This equation helps explain the behavior of electric fields as they emanate from or converge upon electric charges. The second, Gauss’s Law for Electromagnetism, states that the magnetic flux through a closed surface is zero, implying that magnetic poles always occur in pairs—north and south—and that isolated magnetic poles, or monopoles, do not exist in nature.
The third, Faraday’s Law of Electromagnetic Induction, describes how a changing magnetic field through a loop induces an electromotive force or voltage in the loop, forming the basis for electric generators and transformers. The final equation, Ampere’s Law with Maxwell’s addition, relates magnetic fields to the electric currents and the change in electric fields that produce them. This last addition by Maxwell introduced the concept of displacement current, which allowed him to predict that light is an electromagnetic wave. Maxwell’s Equations thus not only explain existing phenomena but also predict new, fundamental properties of light and electromagnetic radiation.
Maxwell’s Equations are fundamental to the theory of electromagnetism, linking electric and magnetic fields with their sources, electric charge and current. These equations form the basis for understanding classical electrodynamics, optics, and electrical circuits. Below, we explore each of Maxwell’s equations and outline their basic derivations.
Gauss’s Law for Electricity is a fundamental principle in electromagnetism that describes the distribution of electric field emanating from charges. The law is elegantly expressed in both integral and differential forms and provides a profound link between electric charge and the resulting electric field.
Derivation: Gauss’s Law for electricity states that the electric flux through a closed surface is proportional to the charge enclosed. Mathematically, it is derived from the inverse square law of Coulomb’s law generalized over a surface. The integral form can be converted to a differential form using the divergence theorem, leading to the equation where ∇ . E is the divergence of the electric field, 𝜌 is the charge density, and ϵ₀ is the permittivity of free space.
Gauss’s Law for Magnetism is one of Maxwell’s four fundamental equations of electromagnetism. It expresses a fundamental property of magnetic fields, particularly focusing on the nature of magnetic monopoles.
Derivation : This law implies that magnetic field lines neither start nor end but form continuous loops. It is derived based on the experimental observation that magnetic monopoles do not exist. The differential form ∇ . B = 0. irectly states that there are no sources (or sinks) of magnetic field lines, reflecting the fact that every magnetic field line that enters a volume must also exit it, effectively ensuring that the net magnetic flux is zero.
Faraday’s Law of Electromagnetic Induction is a fundamental principle that describes how electric currents and electromotive force (EMF) are generated by changing magnetic fields. This law, formulated by Michael Faraday in 1831, is one of the four Maxwell’s Equations and plays a crucial role in the operation of electrical generators, transformers, and many types of electrical machinery.
Derivation: Faraday’s Law states that a changing magnetic field creates an electric field. This is derived by applying the principle of conservation of energy to a loop moving through a magnetic field. The line integral of the electric field around a closed loop is equal to the negative rate of change of the magnetic flux through any surface spanning the loop. Using Stokes’ Theorem, the integral form of Faraday’s Law is converted into a differential form, showing that the curl of E equals the negative rate of change of the magnetic field 𝐵.
Ampere’s Law, initially formulated by André-Marie Ampère, relates magnetic fields to the electric currents that generate them. The law states that the magnetic field created in space around an electric current is proportional to the current. However, James Clerk Maxwell later realized that the original formulation of Ampere’s Law was incomplete and added a crucial term to account for situations where electric fields change over time.
Derivation: Originally, Ampere’s Law related magnetic fields to the currents that produce them. Maxwell added the displacement current term ∂E/∂t to account for the changing electric fields acting like currents and producing magnetic fields. The full law can be derived by considering the magnetic effects of both conduction current and displacement current. Using Stokes’ Theorem, the loop integral around the magnetic field B is related to the current and the rate of change of the electric field through the surface enclosed by the loop.
Electrostatic Precipitators : Electrostatic precipitators are used to remove fine particles like dust and smoke from exhaust gases in industrial plants by charging particles electrically and then capturing them on oppositely charged surfaces. Gauss’s Law (∇ . E = ρ/ϵ₀). helps calculate the electric field generated by a given distribution of charges on the electrodes, optimizing the efficiency of particle removal.
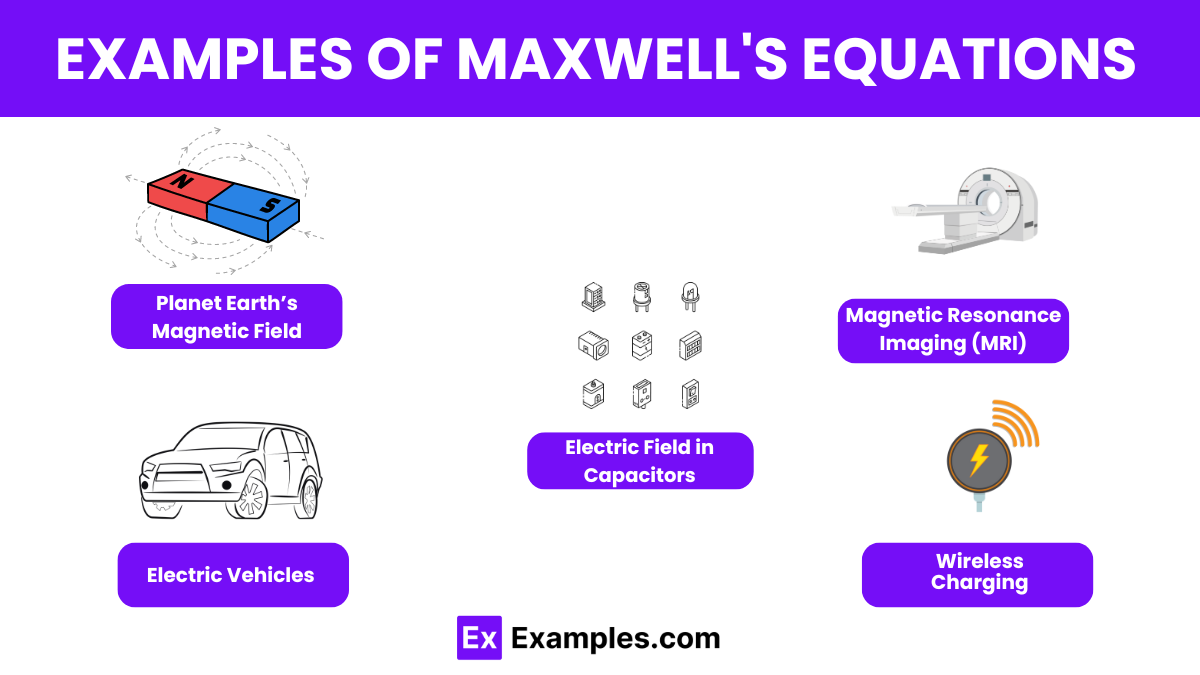
Planet Earth’s Magnetic Field : Gauss’s Law for magnetism ( ∇ . B = 0) indicates that magnetic monopoles do not exist. This principle explains why the Earth’s magnetic field, which resembles that of a giant bar magnet, has a north and a south pole rather than isolated poles. This law helps geophysicists understand planetary magnetism and its effects on things like compass navigation and auroral phenomena.
Hybrid Electric Vehicles : Faraday’s Law( ∇ × E = − ∂B/∂t) underpins the technology in hybrid electric vehicle (HEV) regenerative braking systems. When braking, the kinetic energy of the vehicle is converted into electrical energy rather than heat. A changing magnetic field in the alternator induces an electric current, recharging the battery and improving the vehicle’s efficiency.
Wireless Charging Systems : Ampere’s Law with Maxwell’s addition (∇ × B = μ₀J+μ₀ϵ₀ (∂E/∂t)) is essential in the design of wireless charging systems for devices like smartphones and electric toothbrushes. These systems use coils that generate time-varying magnetic fields, which induce currents in nearby coils located in the devices being charged. Maxwell’s addition of the displacement current allows these varying fields to transmit energy through the air or other non-metallic mediums.
Electric Field in Capacitors : Gauss’s Law for electricity (∇ . E = ρ/ϵ₀) is crucial in determining the electric field distribution in devices like capacitors. In a parallel-plate capacitor, the electric field between the plates can be calculated assuming a uniform charge distribution.
Magnetic Resonance Imaging (MRI) :In MRI machines, Gauss’s Law for magnetism is used to design the magnetic coils that create a highly uniform magnetic field essential for accurate imaging. The law’s assertion that there are no magnetic monopoles helps in creating stable and predictable magnetic fields which are crucial for the detailed images of internal body structures that MRI provides, aiding in medical diagnostics.
Power Generators : Faraday’s Law is the principle behind the operation of hydroelectric, wind, and other types of power generators. As the turbine blades turn, they rotate a magnetic field through coils of wire, inducing a voltage across the ends of the coils due to the change in magnetic flux. This induced voltage drives an electric current through the circuit, producing electrical power from mechanical energy.
Data Transmission in Fiber Optic Cables : Ampere’s Law with Maxwell’s addition plays a critical role in the functioning of fiber optic cables. These cables transmit data through light pulses, a form of electromagnetic wave. The understanding that changing electric fields can create magnetic fields (and vice versa) is crucial in managing the propagation of light within the cable.
Gauss’s Law for Electricity
Where Qenc is the total charge enclosed within a closed surface 𝑆.
Gauss’s Law for Magnetism
Reflects the fact that there are no magnetic charges enclosed within any surface.
Faraday’s Law of Electromagnetic Induction
The line integral of the electric field around a closed loop 𝐶 is equal to the negative rate of change of the magnetic flux through any surface 𝑆 bounded by 𝐶.
Ampere’s Law with Maxwell’s Addition
he line integral of the magnetic field around a closed loop is equal to the sum of the enclosed current and the rate of change of the electric flux through any surface 𝑆 bounded by 𝐶.
Telecommunications : Maxwell’s Equations describe how radio waves and other forms of electromagnetic radiation propagate, which is crucial for radio, TV, and mobile communications. They underpin the design of antennas that transmit and receive signals across various frequencies.
Optical Technologies : These equations govern the behavior of light as electromagnetic waves, influencing the design of optical fibers, lasers, and lenses. They are pivotal in developing technologies that rely on light propagation and manipulation.
Electrical Engineering : Maxwell’s Equations are essential in designing electrical devices like generators, motors, and transformers, which operate on the principles of electromagnetism, such as induction and magnetic effects of currents.
Weather Prediction and Climate Science : The equations are used in atmospheric science to understand electromagnetic phenomena like lightning and are fundamental in remote sensing technologies that monitor weather and environmental changes.
Medical Imaging : In medical imaging, particularly MRI, Maxwell’s Equations help explain how magnetic fields and radio waves can be used to produce detailed internal images of the human body, aiding in non-invasive diagnostics.
Quantum Mechanics and Relativity : Maxwell’s Equations influenced the development of quantum mechanics by describing light as electromagnetic waves, which led to the quantum description of light, and informed the theory of special relativity with the constant speed of light in vacuum.
Wireless Energy Transfer: These equations are utilized in designing systems for wireless energy transfer, such as inductive charging pads for electronic devices, through the use of changing magnetic fields to transfer energy without direct contact.
Yes, Maxwell’s Equations are still extensively used. They underpin much of modern engineering and physics, governing everything from telecommunications and electrical engineering to optics and radio technology.
Maxwell’s corkscrew rule, or the right-hand rule, is a mnemonic for determining the direction of the magnetic field (𝐵) in relation to the current’s direction (𝐼). It states that if you curl the fingers of your right hand in the direction of the current’s flow, your thumb points in the direction of the magnetic field.

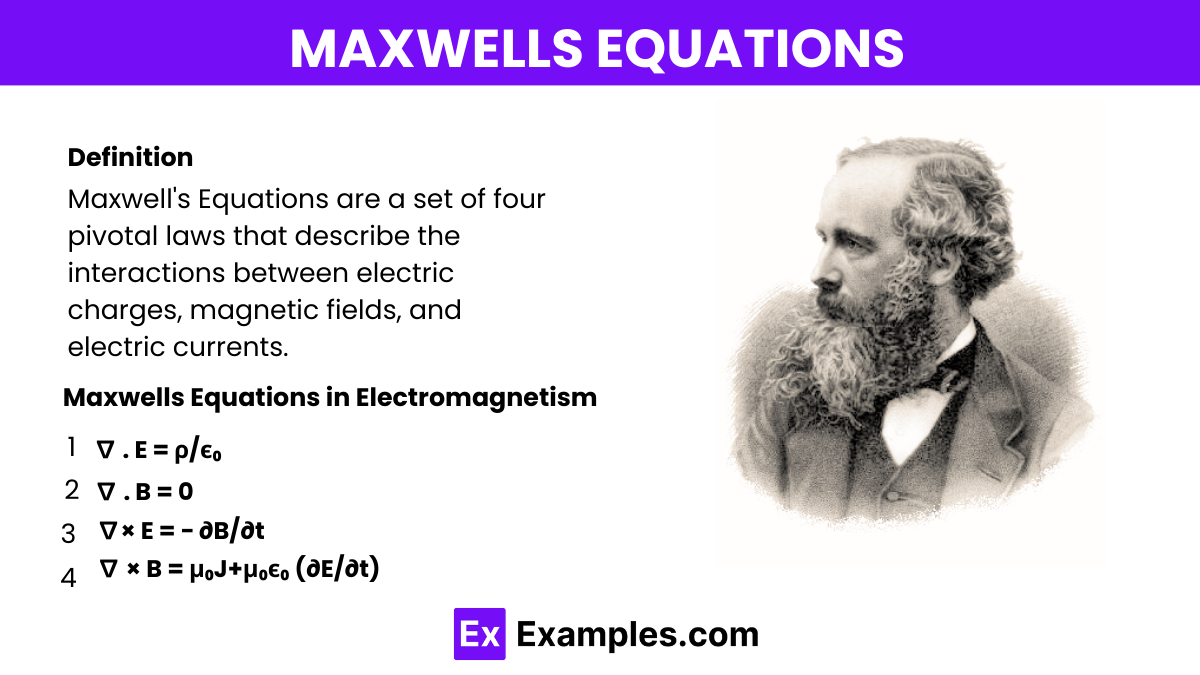
Maxwell’s Equations are a set of four pivotal laws that describe the interactions between electric charges, magnetic fields, and electric currents. These equations, formulated by the Scottish physicist James Clerk Maxwell in the mid-19th century, revolutionized our understanding of electromagnetic phenomena and laid the foundation for modern technologies like radio, television, and electrical power systems.
The first equation, known as Gauss’s Law for Electricity, asserts that the electric flux through a closed surface is proportional to the charge enclosed by that surface. This equation helps explain the behavior of electric fields as they emanate from or converge upon electric charges. The second, Gauss’s Law for Electromagnetism, states that the magnetic flux through a closed surface is zero, implying that magnetic poles always occur in pairs—north and south—and that isolated magnetic poles, or monopoles, do not exist in nature.
The third, Faraday’s Law of Electromagnetic Induction, describes how a changing magnetic field through a loop induces an electromotive force or voltage in the loop, forming the basis for electric generators and transformers. The final equation, Ampere’s Law with Maxwell’s addition, relates magnetic fields to the electric currents and the change in electric fields that produce them. This last addition by Maxwell introduced the concept of displacement current, which allowed him to predict that light is an electromagnetic wave. Maxwell’s Equations thus not only explain existing phenomena but also predict new, fundamental properties of light and electromagnetic radiation.
Maxwell’s Equations are fundamental to the theory of electromagnetism, linking electric and magnetic fields with their sources, electric charge and current. These equations form the basis for understanding classical electrodynamics, optics, and electrical circuits. Below, we explore each of Maxwell’s equations and outline their basic derivations.
Gauss’s Law for Electricity is a fundamental principle in electromagnetism that describes the distribution of electric field emanating from charges. The law is elegantly expressed in both integral and differential forms and provides a profound link between electric charge and the resulting electric field.
Equation: ∇ . E = ρ/ϵ₀
Derivation: Gauss’s Law for electricity states that the electric flux through a closed surface is proportional to the charge enclosed. Mathematically, it is derived from the inverse square law of Coulomb’s law generalized over a surface. The integral form can be converted to a differential form using the divergence theorem, leading to the equation where ∇ . E is the divergence of the electric field, 𝜌 is the charge density, and ϵ₀ is the permittivity of free space.
Gauss’s Law for Magnetism is one of Maxwell’s four fundamental equations of electromagnetism. It expresses a fundamental property of magnetic fields, particularly focusing on the nature of magnetic monopoles.
Equation : ∇ . B = 0
Derivation : This law implies that magnetic field lines neither start nor end but form continuous loops. It is derived based on the experimental observation that magnetic monopoles do not exist. The differential form ∇ . B = 0. irectly states that there are no sources (or sinks) of magnetic field lines, reflecting the fact that every magnetic field line that enters a volume must also exit it, effectively ensuring that the net magnetic flux is zero.
Faraday’s Law of Electromagnetic Induction is a fundamental principle that describes how electric currents and electromotive force (EMF) are generated by changing magnetic fields. This law, formulated by Michael Faraday in 1831, is one of the four Maxwell’s Equations and plays a crucial role in the operation of electrical generators, transformers, and many types of electrical machinery.
Equation : ∇ × E = − ∂B/∂t
Derivation: Faraday’s Law states that a changing magnetic field creates an electric field. This is derived by applying the principle of conservation of energy to a loop moving through a magnetic field. The line integral of the electric field around a closed loop is equal to the negative rate of change of the magnetic flux through any surface spanning the loop. Using Stokes’ Theorem, the integral form of Faraday’s Law is converted into a differential form, showing that the curl of E equals the negative rate of change of the magnetic field 𝐵.
Ampere’s Law, initially formulated by André-Marie Ampère, relates magnetic fields to the electric currents that generate them. The law states that the magnetic field created in space around an electric current is proportional to the current. However, James Clerk Maxwell later realized that the original formulation of Ampere’s Law was incomplete and added a crucial term to account for situations where electric fields change over time.
Equation : ∇ × B = μ₀J+μ₀ϵ₀ (∂E/∂t)
Derivation: Originally, Ampere’s Law related magnetic fields to the currents that produce them. Maxwell added the displacement current term ∂E/∂t to account for the changing electric fields acting like currents and producing magnetic fields. The full law can be derived by considering the magnetic effects of both conduction current and displacement current. Using Stokes’ Theorem, the loop integral around the magnetic field B is related to the current and the rate of change of the electric field through the surface enclosed by the loop.
Electrostatic Precipitators : Electrostatic precipitators are used to remove fine particles like dust and smoke from exhaust gases in industrial plants by charging particles electrically and then capturing them on oppositely charged surfaces. Gauss’s Law (∇ . E = ρ/ϵ₀). helps calculate the electric field generated by a given distribution of charges on the electrodes, optimizing the efficiency of particle removal.
Planet Earth’s Magnetic Field : Gauss’s Law for magnetism ( ∇ . B = 0) indicates that magnetic monopoles do not exist. This principle explains why the Earth’s magnetic field, which resembles that of a giant bar magnet, has a north and a south pole rather than isolated poles. This law helps geophysicists understand planetary magnetism and its effects on things like compass navigation and auroral phenomena.
Hybrid Electric Vehicles : Faraday’s Law( ∇ × E = − ∂B/∂t) underpins the technology in hybrid electric vehicle (HEV) regenerative braking systems. When braking, the kinetic energy of the vehicle is converted into electrical energy rather than heat. A changing magnetic field in the alternator induces an electric current, recharging the battery and improving the vehicle’s efficiency.
Wireless Charging Systems : Ampere’s Law with Maxwell’s addition (∇ × B = μ₀J+μ₀ϵ₀ (∂E/∂t)) is essential in the design of wireless charging systems for devices like smartphones and electric toothbrushes. These systems use coils that generate time-varying magnetic fields, which induce currents in nearby coils located in the devices being charged. Maxwell’s addition of the displacement current allows these varying fields to transmit energy through the air or other non-metallic mediums.
Electric Field in Capacitors : Gauss’s Law for electricity (∇ . E = ρ/ϵ₀) is crucial in determining the electric field distribution in devices like capacitors. In a parallel-plate capacitor, the electric field between the plates can be calculated assuming a uniform charge distribution.
Magnetic Resonance Imaging (MRI) :In MRI machines, Gauss’s Law for magnetism is used to design the magnetic coils that create a highly uniform magnetic field essential for accurate imaging. The law’s assertion that there are no magnetic monopoles helps in creating stable and predictable magnetic fields which are crucial for the detailed images of internal body structures that MRI provides, aiding in medical diagnostics.
Power Generators : Faraday’s Law is the principle behind the operation of hydroelectric, wind, and other types of power generators. As the turbine blades turn, they rotate a magnetic field through coils of wire, inducing a voltage across the ends of the coils due to the change in magnetic flux. This induced voltage drives an electric current through the circuit, producing electrical power from mechanical energy.
Data Transmission in Fiber Optic Cables : Ampere’s Law with Maxwell’s addition plays a critical role in the functioning of fiber optic cables. These cables transmit data through light pulses, a form of electromagnetic wave. The understanding that changing electric fields can create magnetic fields (and vice versa) is crucial in managing the propagation of light within the cable.
Gauss’s Law for Electricity
Equation : ∮ₛ E⋅dA= ϵ₀Q ₑₙ꜀
Where Qenc is the total charge enclosed within a closed surface 𝑆.
Gauss’s Law for Magnetism
∮ₛ B⋅dA=0
Reflects the fact that there are no magnetic charges enclosed within any surface.
Faraday’s Law of Electromagnetic Induction
∮꜀ E⋅dl=− d/dt ∫B⋅dA
The line integral of the electric field around a closed loop 𝐶 is equal to the negative rate of change of the magnetic flux through any surface 𝑆 bounded by 𝐶.
Ampere’s Law with Maxwell’s Addition
∮꜀ B⋅dl = μ0Ienc+μ₀ϵ₀ d/dt ∫SE⋅dA
he line integral of the magnetic field around a closed loop is equal to the sum of the enclosed current and the rate of change of the electric flux through any surface 𝑆 bounded by 𝐶.
Telecommunications : Maxwell’s Equations describe how radio waves and other forms of electromagnetic radiation propagate, which is crucial for radio, TV, and mobile communications. They underpin the design of antennas that transmit and receive signals across various frequencies.
Optical Technologies : These equations govern the behavior of light as electromagnetic waves, influencing the design of optical fibers, lasers, and lenses. They are pivotal in developing technologies that rely on light propagation and manipulation.
Electrical Engineering : Maxwell’s Equations are essential in designing electrical devices like generators, motors, and transformers, which operate on the principles of electromagnetism, such as induction and magnetic effects of currents.
Weather Prediction and Climate Science : The equations are used in atmospheric science to understand electromagnetic phenomena like lightning and are fundamental in remote sensing technologies that monitor weather and environmental changes.
Medical Imaging : In medical imaging, particularly MRI, Maxwell’s Equations help explain how magnetic fields and radio waves can be used to produce detailed internal images of the human body, aiding in non-invasive diagnostics.
Quantum Mechanics and Relativity : Maxwell’s Equations influenced the development of quantum mechanics by describing light as electromagnetic waves, which led to the quantum description of light, and informed the theory of special relativity with the constant speed of light in vacuum.
Wireless Energy Transfer: These equations are utilized in designing systems for wireless energy transfer, such as inductive charging pads for electronic devices, through the use of changing magnetic fields to transfer energy without direct contact.
Yes, Maxwell’s Equations are still extensively used. They underpin much of modern engineering and physics, governing everything from telecommunications and electrical engineering to optics and radio technology.
Maxwell’s corkscrew rule, or the right-hand rule, is a mnemonic for determining the direction of the magnetic field (𝐵) in relation to the current’s direction (𝐼). It states that if you curl the fingers of your right hand in the direction of the current’s flow, your thumb points in the direction of the magnetic field.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which of the following is Gauss's Law for electricity?
∇·E = ρ/ε₀
∇×E = -∂B/∂t
∇·B = 0
∇×B = μ₀J + μ₀ε₀∂E/∂t
What does Gauss's Law for magnetism state about magnetic monopoles?
They exist
They are abundant
They do not exist
They are unstable
Which Maxwell equation describes Faraday's Law of Induction?
∇·E = ρ/ε₀
∇×E = -∂B/∂t
∇·B = 0
∇×B = μ₀J + μ₀ε₀∂E/∂t
In the equation ∇·E = ρ/ε₀, what does ρ represent?
Electric field
Charge density
Magnetic field
Current density
What does the term ε₀ stand for in Maxwell's equations?
Magnetic permeability
Electric permittivity of free space
Electric field strength
Magnetic flux
Which Maxwell equation implies that magnetic field lines are continuous?
∇·E = ρ/ε₀
∇×E = -∂B/∂t
∇·B = 0
∇×B = μ₀J + μ₀ε₀∂E/∂t
In the equation ∇×E = -∂B/∂t, what does the term ∂B/∂t represent?
Electric flux
Magnetic flux
Time rate of change of the magnetic field
Time rate of change of the electric field
What is the significance of the term μ₀ in Maxwell's equations?
Electric permittivity
Magnetic permeability of free space
Electric flux density
Magnetic flux density
According to Maxwell's equations, what is the relationship between electric fields and magnetic fields in empty space?
They are independent
They are perpendicular to each other and propagate as waves
They are parallel to each other and static
They cancel each other out
What does the term μ₀ε₀ represent in the context of electromagnetic waves?
Speed of light in vacuum
Electric flux
Magnetic flux
Charge density
Before you leave, take our quick quiz to enhance your learning!

