What is the square of 19?
361
370
380
390

The “square of 19” refers to the result obtained when the number 19 is multiplied by itself.
In mathematical terms, it is denoted as 19². To calculate the square of 19, you simply multiply 19 by 19, which equals 361.
Calculating the square of 19 involves basic arithmetic, where you multiply 19 by itself to find the result. This operation is commonly encountered in various mathematical problems, especially in geometry and algebra.
√19 = 4.358898943540674
or
√19=4.358 up to three places of decimal
The square root of 19, denoted as √19, is a foundational concept in mathematics. It represents the value that, when multiplied by itself, results in 19. In simpler terms, it’s the number which, when squared, equals 19. However, unlike whole numbers or fractions, the square root of 19 is an irrational number. This means its decimal representation goes on infinitely without repeating a pattern. When calculated, the approximate value of the square root of 19 is 4.358898943540674. Despite its seemingly endless decimal expansion, the square root of 19 holds significance in various mathematical calculations, geometric problems, and real-world applications. Understanding this concept helps us grasp the relationship between numbers, shapes, and quantities, contributing to problem-solving and analytical skills in fields such as engineering, physics, and finance.
Square Root of 19: 4.358898943540674
Exponential Form: 19^½ or 19^0.5
Radical Form: √19
To determine whether the square root of 19 is rational or irrational, we need to consider its decimal representation. The square root of 19, when calculated, results in an infinite decimal expansion without a repeating pattern. Let’s see:
√19 ≈ 4.358898943540674
This approximation shows that the decimal part of √19 goes on infinitely without repeating. In other words, it’s a non-terminating, non-repeating decimal.
Now, let’s suppose for contradiction that √19 is rational, meaning it can be expressed as a fraction of two integers in the form a/b, where a and b are integers and b is not zero.
If √19 were rational, then it could be expressed as:
√19 = a/b
Squaring both sides to eliminate the square root, we get:
19 = (a²) / (b²)
Rearranging the equation, we find:
a² = 19 × (b²)
This implies that a² is a multiple of 19. However, since 19 is a prime number, a² must also be a multiple of 19. This means that a itself must be a multiple of 19.
Now, if a is a multiple of 19, let’s express it as a = 19 × k, where k is some integer.
Substituting this expression back into the original equation, we get:
(19 × k)² = 19 × (b²)
Expanding and simplifying, we have:
361 × k² = 19 × (b²)
This implies that b² must also be a multiple of 19. Similarly, as 19 is a prime number, b itself must also be a multiple of 19.
However, if both a and b are multiples of 19, then the fraction a/b would not be in its simplest form, as both a and b share a common factor of 19.
Therefore, we have reached a contradiction. The assumption that √19 is rational leads to a contradiction, so we conclude that √19 is irrational.
The square root of 19 is an irrational number.
In summary, the square root of 19 is irrational, as its decimal representation is non-terminating and non-repeating, and it cannot be expressed as a fraction of two integers.
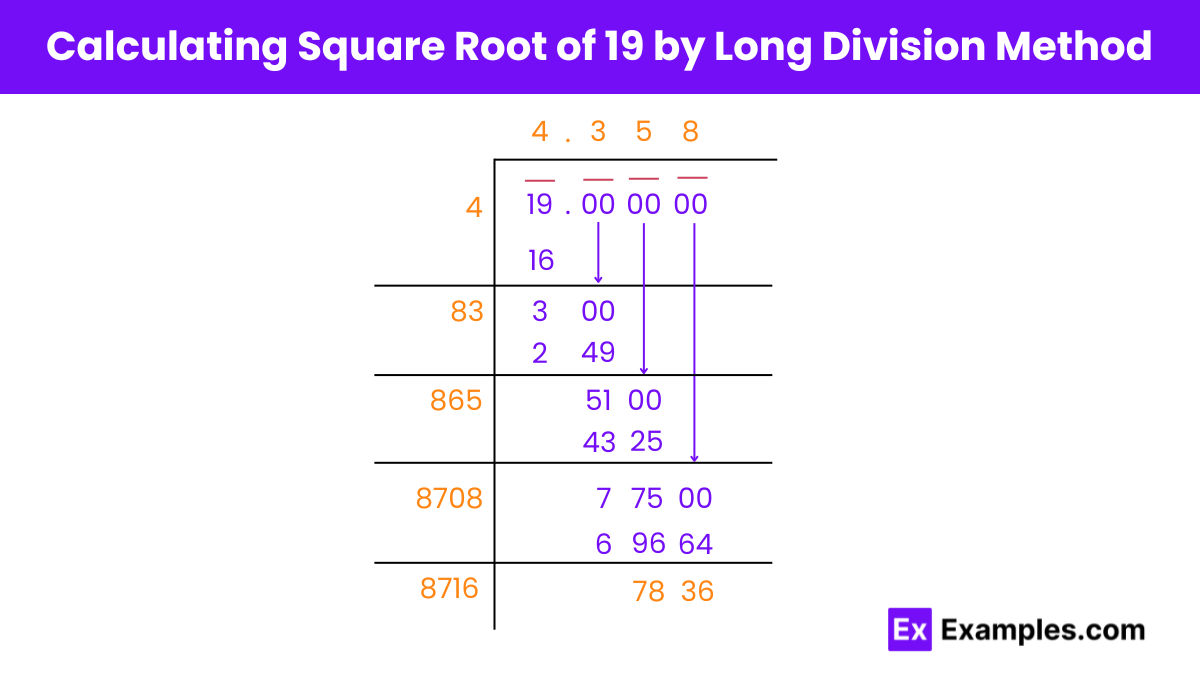
Finding the square root of 19 using the long division method involves the following steps:
Step 1: Preparation
Write 19 as 19.00 00 00, grouping digits in pairs from the decimal point. For 19, it looks like “19”.
Step 2: Find the Largest Square
Identify the largest square smaller than or equal to 19, which is 16 (4²). Place 4 above the line as the first digit of the root.
Step 3: Subtract and Bring Down
Subtract 16 from 19 to get 3, then bring down the next pair of zeros to make it 300.
Step 4: Double and Find the Next Digit
Double the current result (4) to get 8. Now, find a digit (X) such that 48 multiplied by X is less than or equal to 300. Here, X is 6, because 486×6=288.
Step 5: Repeat with Precision
Subtract 288 from 300 to get 12, bring down the next zeros to get 1200, then double the quotient (46) to get 92. Choose a digit (Y) so that 469 multiplied by Y is just under 1200.
Step 6: Finish at Desired Accuracy
Continue the process until reaching the desired level of accuracy. For the square root of 19, this method gives us about 4.358 as we extend the division
A perfect square root is a number that can be expressed as the product of an integer multiplied by itself. For instance, 4 (2 × 2) and 9 (3 × 3) are perfect square roots. To determine whether 19 is a perfect square root, we examine if it can be expressed as the square of an integer.
However, when we try to find an integer that, when multiplied by itself, equals 19, we realize that there are no such integers. In other words, there is no whole number x such that x × x = 19.
Since 19 cannot be expressed as the product of two identical integers, it doesn’t have an integer square root. While 19 does have a square root (√19), it’s an irrational number and not the result of multiplying any whole number by itself. Therefore, we conclude that 19 is not a perfect square root.
Is Root 19 a Real Number?
Yes, the square root of 19, denoted as √19, is a real number. Real numbers encompass all the numbers that can be located on the number line, including both rational and irrational numbers. Since √19 exists on the number line and is not an imaginary number (which involves the square root of negative numbers), it is classified as a real number.
Is 19 an Integer, Yes or No?
No, 19 is not an integer. Integers are whole numbers that can be positive, negative, or zero. However, 19 is specifically a positive whole number and does not include the negative integers or zero. Therefore, 19 is not an integer.
Is Square Root of 19 a Natural Number?
No, the square root of 19, represented as √19, is not a natural number. Natural numbers are the counting numbers starting from 1 (1, 2, 3, 4, 5, …), and they do not include fractions or decimals. Since the square root of 19 is an irrational number, it cannot be expressed as a whole number or a fraction. Therefore, √19 is not a natural number.

The “square of 19” refers to the result obtained when the number 19 is multiplied by itself.
In mathematical terms, it is denoted as 19². To calculate the square of 19, you simply multiply 19 by 19, which equals 361.
Mathematically, 19² (19×19) =361
Calculating the square of 19 involves basic arithmetic, where you multiply 19 by itself to find the result. This operation is commonly encountered in various mathematical problems, especially in geometry and algebra.
√19 = 4.358898943540674
or
√19=4.358 up to three places of decimal
The square root of 19, denoted as √19, is a foundational concept in mathematics. It represents the value that, when multiplied by itself, results in 19. In simpler terms, it’s the number which, when squared, equals 19. However, unlike whole numbers or fractions, the square root of 19 is an irrational number. This means its decimal representation goes on infinitely without repeating a pattern. When calculated, the approximate value of the square root of 19 is 4.358898943540674. Despite its seemingly endless decimal expansion, the square root of 19 holds significance in various mathematical calculations, geometric problems, and real-world applications. Understanding this concept helps us grasp the relationship between numbers, shapes, and quantities, contributing to problem-solving and analytical skills in fields such as engineering, physics, and finance.
Square Root of 19: 4.358898943540674
Exponential Form: 19^½ or 19^0.5
Radical Form: √19
To determine whether the square root of 19 is rational or irrational, we need to consider its decimal representation. The square root of 19, when calculated, results in an infinite decimal expansion without a repeating pattern. Let’s see:
√19 ≈ 4.358898943540674
This approximation shows that the decimal part of √19 goes on infinitely without repeating. In other words, it’s a non-terminating, non-repeating decimal.
Now, let’s suppose for contradiction that √19 is rational, meaning it can be expressed as a fraction of two integers in the form a/b, where a and b are integers and b is not zero.
If √19 were rational, then it could be expressed as:
√19 = a/b
Squaring both sides to eliminate the square root, we get:
19 = (a²) / (b²)
Rearranging the equation, we find:
a² = 19 × (b²)
This implies that a² is a multiple of 19. However, since 19 is a prime number, a² must also be a multiple of 19. This means that a itself must be a multiple of 19.
Now, if a is a multiple of 19, let’s express it as a = 19 × k, where k is some integer.
Substituting this expression back into the original equation, we get:
(19 × k)² = 19 × (b²)
Expanding and simplifying, we have:
361 × k² = 19 × (b²)
This implies that b² must also be a multiple of 19. Similarly, as 19 is a prime number, b itself must also be a multiple of 19.
However, if both a and b are multiples of 19, then the fraction a/b would not be in its simplest form, as both a and b share a common factor of 19.
Therefore, we have reached a contradiction. The assumption that √19 is rational leads to a contradiction, so we conclude that √19 is irrational.
The square root of 19 is an irrational number.
In summary, the square root of 19 is irrational, as its decimal representation is non-terminating and non-repeating, and it cannot be expressed as a fraction of two integers.
Prime Factorization Method:
Since 19 is a prime number, its prime factorization is simply 19 itself.
Therefore, the square root of 19 cannot be expressed as a rational number and is irrational.
Example: √19 is irrational because its prime factorization is 19, and it cannot be simplified further.
Long Division Method:
Start with an initial guess, such as 4.
Divide 19 by the guess: 19 ÷ 4 = 4.75.
Average the result with the guess: (4 + 4.75) ÷ 2 = 4.375.
Repeat the process until reaching the desired level of accuracy.
Example: Using long division, we can approximate √19 as 4.358, which is close to the actual value.
Approximation Techniques:
Apply Newton’s method: Start with an initial guess, like 4, and use the formula: xₙ₊₁=1/2(xₙ+19/xₙ)
Iterate this formula until reaching the desired precision.
Example: With an initial guess of 4, applying Newton’s method gives an approximation of √19 ≈ 4.3589 after a few iterations.
Calculator or Software:
Use a scientific calculator or mathematical software to find the square root of 19 directly.
Example: Entering √19 into a calculator yields the approximate value of 4.3589, which is the square root of 19.
Finding the square root of 19 using the long division method involves the following steps:
Step 1: Preparation
Write 19 as 19.00 00 00, grouping digits in pairs from the decimal point. For 19, it looks like “19”.
Step 2: Find the Largest Square
Identify the largest square smaller than or equal to 19, which is 16 (4²). Place 4 above the line as the first digit of the root.
Step 3: Subtract and Bring Down
Subtract 16 from 19 to get 3, then bring down the next pair of zeros to make it 300.
Step 4: Double and Find the Next Digit
Double the current result (4) to get 8. Now, find a digit (X) such that 48 multiplied by X is less than or equal to 300. Here, X is 6, because 486×6=288.
Step 5: Repeat with Precision
Subtract 288 from 300 to get 12, bring down the next zeros to get 1200, then double the quotient (46) to get 92. Choose a digit (Y) so that 469 multiplied by Y is just under 1200.
Step 6: Finish at Desired Accuracy
Continue the process until reaching the desired level of accuracy. For the square root of 19, this method gives us about 4.358 as we extend the division
A perfect square root is a number that can be expressed as the product of an integer multiplied by itself. For instance, 4 (2 × 2) and 9 (3 × 3) are perfect square roots. To determine whether 19 is a perfect square root, we examine if it can be expressed as the square of an integer.
However, when we try to find an integer that, when multiplied by itself, equals 19, we realize that there are no such integers. In other words, there is no whole number x such that x × x = 19.
Therefore,19 is not a perfect square root
Since 19 cannot be expressed as the product of two identical integers, it doesn’t have an integer square root. While 19 does have a square root (√19), it’s an irrational number and not the result of multiplying any whole number by itself. Therefore, we conclude that 19 is not a perfect square root.
Is Root 19 a Real Number?
Yes, the square root of 19, denoted as √19, is a real number. Real numbers encompass all the numbers that can be located on the number line, including both rational and irrational numbers. Since √19 exists on the number line and is not an imaginary number (which involves the square root of negative numbers), it is classified as a real number.
Is 19 an Integer, Yes or No?
No, 19 is not an integer. Integers are whole numbers that can be positive, negative, or zero. However, 19 is specifically a positive whole number and does not include the negative integers or zero. Therefore, 19 is not an integer.
Is Square Root of 19 a Natural Number?
No, the square root of 19, represented as √19, is not a natural number. Natural numbers are the counting numbers starting from 1 (1, 2, 3, 4, 5, …), and they do not include fractions or decimals. Since the square root of 19 is an irrational number, it cannot be expressed as a whole number or a fraction. Therefore, √19 is not a natural number.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 19?
361
370
380
390
Calculate the square root of 361.
17
18
19
20
What is the square of 20?
380
400
420
440
Find the square root of 400.
18
19
20
21
What is the square of 18?
324
340
360
380
Calculate the square root of 324.
16
17
18
19
What is the square of 21?
400
420
441
462
Find the square root of 441.
20
21
22
23
What is the square of 22?
440
462
484
506
Calculate the square root of 484.
21
22
23
24
Before you leave, take our quick quiz to enhance your learning!

