Calculate the square of 15.
100
225
256
144
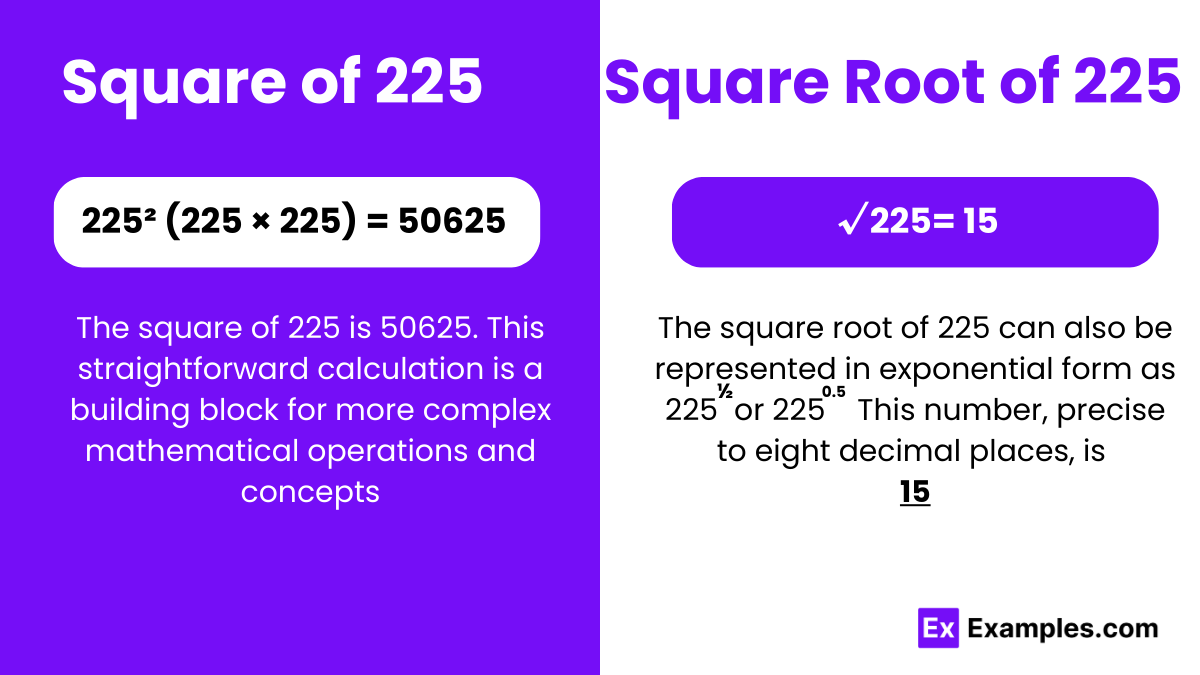
The square of 225 (225²) is calculated by multiplying 225 by itself:
225 × 225 = 50625.
So, the square of 225 is 50,625.
Geometrically, if you have a square with each side measuring 225 units, the total area enclosed by the square will be 50,625 square units. Understanding square number and the square of 225 is essential in various mathematical contexts, including geometry, algebra, and arithmetic. It finds applications in calculating areas, volumes, distances, and solving mathematical problems.
This is because 15 × 15 = 225.
Thus, the square root of 225 is exactly 15. This calculation is important in various mathematical and practical contexts, such as geometry, where it can represent the side length of a square with an area of 225 square units. It’s also significant in algebra and engineering, where understanding square roots helps in solving problems involving areas and other applications that require precise measurements.
Square Root of 225: 15
Exponential Form: 225^½ or 225^0.5
Radical Form: √225
This is because 225 is a perfect square, being 15 × 15 = 225. As a result, the square root of 225 is exactly 15, which can be expressed as a fraction 15/1, making it a rational number.
Prime Factorization Method:
Break down 225 into its prime factors, which are 3 × 3 × 5 × 5. Pair up identical factors and take one from each pair to get 3 × 5 = 15. Therefore, the square root of 225 is 15.
Repeated Subtraction Method:
Start subtracting consecutive odd numbers from 225 until you reach 0. Count how many times you subtracted, and that count is the square root. For 225, this method will result in 15 subtractions, indicating the square root is 15.
Estimation Method:
Since 15 is a known square root close to 225, you can estimate by finding a number close to 15 that squares to 225. By trial and error, you can quickly determine that 15 is indeed the square root of 225.
Calculator:
Use a calculator with a square root function to directly find the square root of 225, which is 15.
These methods offer different approaches to find the square root of 225, catering to various preferences and situations.
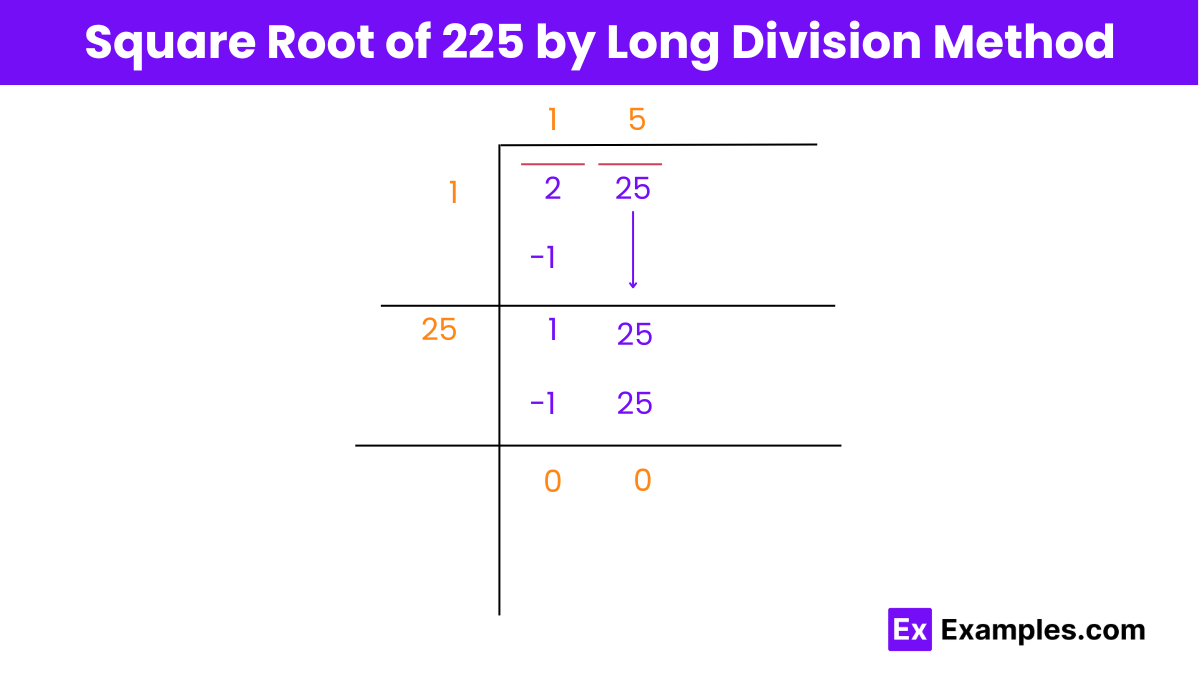
Here’s how to find the square root of 225 by the long division method:
Step 1: Start with the number 225 and group the digits into pairs from the right. So, from 225, we choose 25 as a pair, and 2 stands alone.
Step 2: We begin by dividing 2 with a number such that the number × number gives 2 or a number less than that.
Step 3: After dividing, we obtain 1 as the quotient and 1 as the remainder.
Step 4: Next, we bring down 25 for division so that the new dividend becomes 125.
Step 5: We double the divisor, meaning 1 + 1 = 2, and write this as one of the digits for the new divisor.
Step 6: Identify a number that can be placed next to 2 to obtain a two-digit number as a new divisor. This number should also be multiplied by itself to produce 125 or less than that.
Step 7: We find that 5 is the number, such that 25 × 5 gives 125.
Step 8: Finally, we obtain the quotient 15 and the remainder as 0.
By following these steps, we have successfully found that the square root of 225 is 15 using the long division method.
A perfect square is a number that can be expressed as the product of an integer multiplied by itself. In the case of 225, it can be expressed as 15 × 15, which equals 225. Therefore, 225 is indeed a perfect square.
The basic factors of 225 are 1, 3, 5, 15, and 225. These numbers can be multiplied together to get 225, making them its basic factors.
No, 225 is not a perfect cube.
A perfect cube is a number that can be expressed as the product of an integer multiplied by itself twice.

225² (225 × 225) = 50625
The square of 225 (225²) is calculated by multiplying 225 by itself:
225 × 225 = 50625.
So, the square of 225 is 50,625.
Geometrically, if you have a square with each side measuring 225 units, the total area enclosed by the square will be 50,625 square units. Understanding square number and the square of 225 is essential in various mathematical contexts, including geometry, algebra, and arithmetic. It finds applications in calculating areas, volumes, distances, and solving mathematical problems.
√225 = 15
This is because 15 × 15 = 225.
Thus, the square root of 225 is exactly 15. This calculation is important in various mathematical and practical contexts, such as geometry, where it can represent the side length of a square with an area of 225 square units. It’s also significant in algebra and engineering, where understanding square roots helps in solving problems involving areas and other applications that require precise measurements.
Square Root of 225: 15
Exponential Form: 225^½ or 225^0.5
Radical Form: √225
The square root of 225 is rational.
This is because 225 is a perfect square, being 15 × 15 = 225. As a result, the square root of 225 is exactly 15, which can be expressed as a fraction 15/1, making it a rational number.
Prime Factorization Method:
Break down 225 into its prime factors, which are 3 × 3 × 5 × 5. Pair up identical factors and take one from each pair to get 3 × 5 = 15. Therefore, the square root of 225 is 15.
Repeated Subtraction Method:
Start subtracting consecutive odd numbers from 225 until you reach 0. Count how many times you subtracted, and that count is the square root. For 225, this method will result in 15 subtractions, indicating the square root is 15.
Estimation Method:
Since 15 is a known square root close to 225, you can estimate by finding a number close to 15 that squares to 225. By trial and error, you can quickly determine that 15 is indeed the square root of 225.
Calculator:
Use a calculator with a square root function to directly find the square root of 225, which is 15.
These methods offer different approaches to find the square root of 225, catering to various preferences and situations.
Here’s how to find the square root of 225 by the long division method:
Step 1: Start with the number 225 and group the digits into pairs from the right. So, from 225, we choose 25 as a pair, and 2 stands alone.
Step 2: We begin by dividing 2 with a number such that the number × number gives 2 or a number less than that.
Step 3: After dividing, we obtain 1 as the quotient and 1 as the remainder.
Step 4: Next, we bring down 25 for division so that the new dividend becomes 125.
Step 5: We double the divisor, meaning 1 + 1 = 2, and write this as one of the digits for the new divisor.
Step 6: Identify a number that can be placed next to 2 to obtain a two-digit number as a new divisor. This number should also be multiplied by itself to produce 125 or less than that.
Step 7: We find that 5 is the number, such that 25 × 5 gives 125.
Step 8: Finally, we obtain the quotient 15 and the remainder as 0.
By following these steps, we have successfully found that the square root of 225 is 15 using the long division method.
Yes, 225 is a perfect square.
A perfect square is a number that can be expressed as the product of an integer multiplied by itself. In the case of 225, it can be expressed as 15 × 15, which equals 225. Therefore, 225 is indeed a perfect square.
The basic factors of 225 are 1, 3, 5, 15, and 225. These numbers can be multiplied together to get 225, making them its basic factors.
No, 225 is not a perfect cube.
A perfect cube is a number that can be expressed as the product of an integer multiplied by itself twice.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Calculate the square of 15.
100
225
256
144
If x² = 225, what are the possible values of x?
25
-25
15 and -15
225
What is the square of 225?
50,625
15,625
5000
900
Find the square root of 225.
10
15
20
25
What is the result of squaring the square root of 225?
225
230
235
240
What is 225 divided by the square root of 225?
15
225
10
30
What is the result when you subtract the square root of 225 from 225?
180
210
200
225
What is 225 raised to the power of 1/2?
10
15
20
25
Find the number whose square is 225.
15
-15
225
Both 15 and -15
What is the square root of 225 multiplied by 2?
20
30
40
50
Before you leave, take our quick quiz to enhance your learning!

