Square & Square Root of 44
Square & Square Root of 44
Square 44
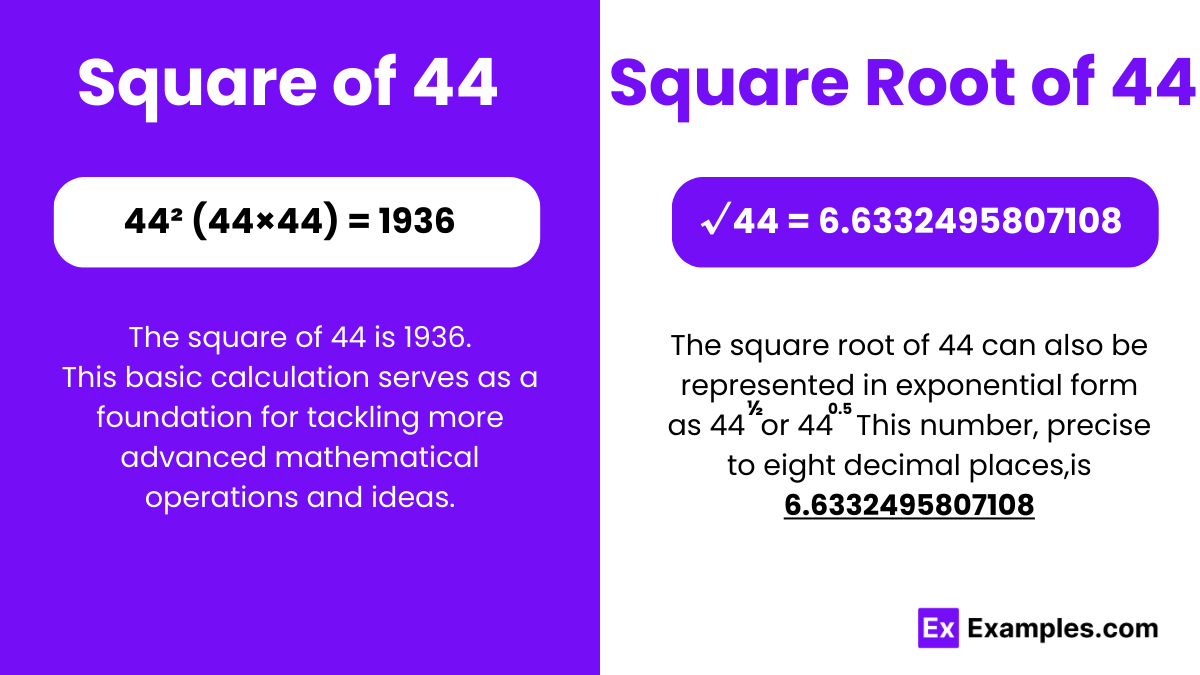
The square of 44 is essentially what you get when you multiply the number 44 by itself.
It’s a simple mathematical operation that gives us a specific result. When we say “44 squared” or “44 to the power of 2,” we’re referring to this operation.
To calculate the square of 44, you don’t need any fancy tricks or complex methods. You just multiply 44 by itself. In other words:
44Β² (44 Γ 44) = 1936
So, the square of 44 equals 1936.
Think of it this way: if you had a square with each side measuring 44 units, and you wanted to find out how many total units are inside that square, you’d simply multiply the length (44) by the width (44), which gives you the total area inside the square.
It’s a fundamental concept in mathematics, and understanding how to square a number like 44 lays the groundwork for more advanced mathematical operations.
Square Root of 44
The square root of a number is a value that, when multiplied by itself, gives the original number. So, the square root of 44 is the number which, when multiplied by itself, equals 44. To find it, we use a process called “taking the square root.”
To calculate the square root of 44, we can use different methods like the prime factorization method, long division method, or estimation method. However, for simplicity, let’s use a calculator or a mathematical software program.
When you input 44 and ask for its square root, you’ll get the result:
or
β44 = 6.633 up to three decimal places.
Understanding the square root of 44 holds significance in various mathematical calculations, geometric analyses, and practical applications. Understanding this concept aids in problem-solving across disciplines like engineering, physics, and finance.
Square Root of 44: 6.6332495807108
Exponential Form: 44^Β½ or 44^0.5
Radical Form: β44
Is the Square Root of 44 Rational or Irrational?
The square root of 44 is an irrational number
To understand why, letβs first define rational and irrational numbers.
Rational numbers are those expressible as a fraction of two integers, where the denominator is not zero, written as a/b.
Examples include 1/2, -3, and 5.
Irrational numbers, however, cannot be expressed as simple fractions of two integers. Their decimal representations are non-repeating and non-terminating.
Examples include β2, Ο (pi), and β3.
Square Root of 44 as Irrational:
When we calculate the square root of 44, we find it cannot be expressed as a fraction of two integers.
Its decimal expansion, approximately 6.6332495807108, continues infinitely without repeating a pattern.
Letβs simplify:
β44 = β(4 Γ 11) = β4 Γ β11 = 2 Γ β11
Since β11 is famously irrational, the product of a rational number (2) and an irrational number (β11) is also irrational.
In summary, the square root of 44 is irrational because it simplifies to an irrational number (β11) when multiplied by a rational number (2), resulting in an irrational product.
Method to Find Value of Root 44
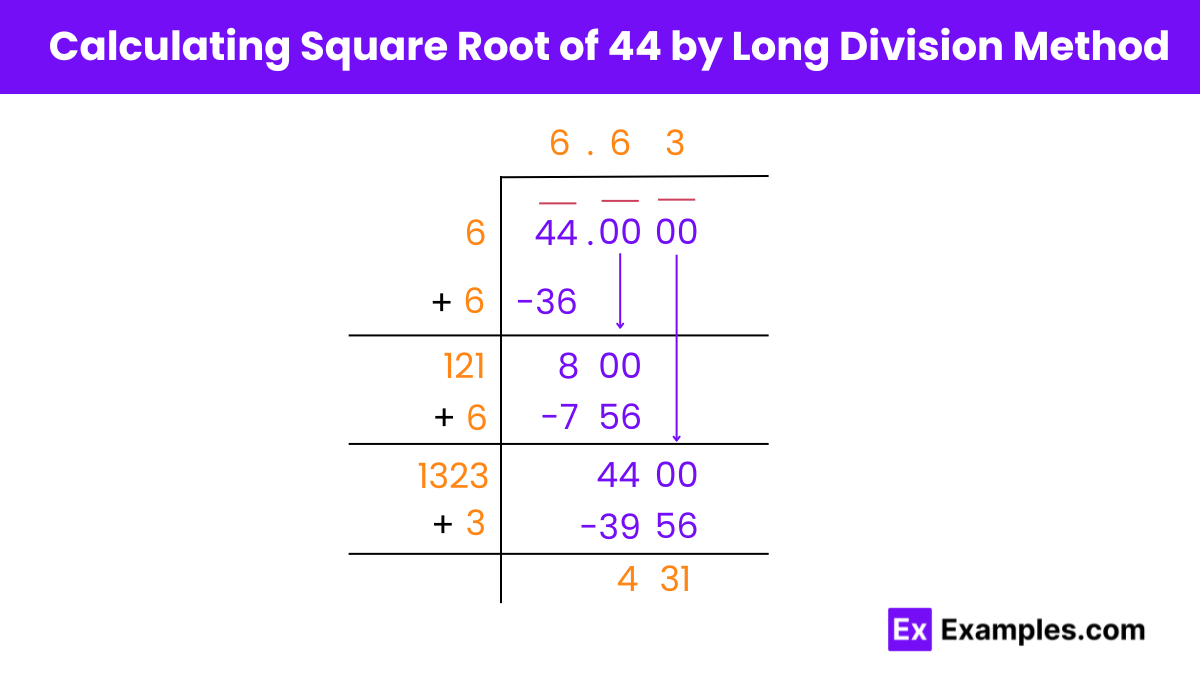
Finding the square root of 44 may seem challenging at first glance, but several methods can help us arrive at an approximation. Let’s explore these methods with examples:
- Prime Factorization Method:
- Prime factorization of 44: 44 = 2 Γ 2 Γ 11
- Grouping the factors: 2 Γ 11 Γ 2
- So, the square root of 44 is approximately 2 Γ 11 β2.
- Estimation Method:
- Identifying the nearest perfect squares: 36 and 49.
- Since 44 is closer to 49, we know the square root of 44 is between 6 and 7.
- Interpolating between the square roots of 36 (6) and 49 (7), we get an approximate value.
- Long Division Method:
- Let’s start with an initial guess of 6 for the square root of 44.
- Dividing 44 by 6 gives us a quotient.
- We then average this quotient with our guess to get a new guess, and repeat the process until we reach a satisfactory approximation.
- Newton’s Method:
- Newton’s method involves iteratively refining an initial guess using calculus.
- Starting with an initial guess for the square root of 44, we use Newton’s formula to update our guess until it converges to the actual value of the square root
Square Root of 44 by Long Division Method
Finding the square root of 44 using the long division method follows a similar process:
Step 1: Start by dividing the number 44 by 6, noting that 36 is the nearest perfect square less than 44. This is because 6Β² = 36.
Step 2: Keep the divisor, 6, as the same number used for the quotient. Now, multiply the quotient by the divisor (6 Γ 6) and subtract this product from 44 to get a new remainder.
Step 3: Retain the quotient, which is 6, and sum it with the divisor, also 6, to get a new value.
Step 4: Insert a decimal point after the quotient to extend the division and add two zeros to the remainder, turning it into 800. Find a digit that, when appended to 12 (from the sum of the quotient and divisor) and multiplied by itself, yields a number just below 800. For example, appending 6 to 12 to form 126 and multiplying it by 6 gives 756. Subtract this product from 800 to find a new remainder.
Step 5: Again, bring down two zeros, making the remainder 4400. Add 6 to 126 to get 132. Look for a digit that, when placed at the end of 132 and multiplied by itself, produces a result slightly less than 4400. Using 3 in this case, 1323 multiplied by 3 gives 3969. This number is then appended to the quotient, and the product is subtracted from 4400 to get the next remainder.
Step 6: Continue this procedure, bringing down more zeros and repeating the process until the remainder approaches zero. This method will yield the square root of 44 to two decimal places using a systematic division approach.
44 is Perfect Square root or Not
To determine whether 44 is a perfect square root, we follow a similar logic used for evaluating whether a number like 40 is a perfect square. A perfect square root is a number that can be expressed as the product of an integer with itself. Examples include 4, which is 2 Γ 2, and 9, which is 3 Γ 3. These numbers can be expressed as the square of an integer.
Upon inspecting 44, we search for any two integers (x) such that (x Γ x = 44). However, no such integer exists for 44. In simpler terms, there are no whole numbers that, when multiplied by themselves, result in 44. Therefore, 44 cannot be expressed as the square of any integer, making it not a perfect square root.
While 44 does have a square root, denoted as β44, this square root is an irrational number. It cannot be expressed neatly as a fraction or as the product of any two identical whole numbers.
In conclusion, 44 is not a perfect square root because it cannot be expressed as the product of two identical integers, similar to how 40 is not a perfect square. The square root of 44, while existent, is not an integer but an irrational number, indicating it doesn’t have an integer square root.
FAQβs
Is ββ44 a Real Number?
Yes, β44β is a real number. In mathematics, real numbers include all the rational numbers, such as integers and fractions, and all the irrational numbers. An irrational number cannot be expressed as a simple fraction but is a number that exists on the number line. Since β44β can be placed on the number line and is the distance from 0 to the point representing 44 on the number line, it is considered a real number. However, it’s an irrational number because it cannot be precisely represented as a fraction or a simple decimal.
How Do You Simplify 44Β²?
To simplify 44Β², you are essentially asked to find the square of 44, which means multiplying 44 by itself. Let’s calculate it:
44Β²=44Γ44
44Β²=1936
Therefore, the simplification of 44Β² is 1936.
What Square Root is Between 44?
To find out which square roots are around the number 44, we look for perfect squares nearest to 44. Since 44 is not a perfect square, we find the perfect squares just below and above it:
- The largest perfect square less than 44 is 36, which is 6Β².
- The smallest perfect square greater than 44 is 49, which is 7Β².
Thus, the square roots around 44 in terms of their positions between perfect squares are 6 and 7, meaning β44β lies between 6 and 7.
Share :
