What is the square of 50?
2400
2500
2600
2700


The “square of 50” represents the outcome when the number 50 is multiplied by itself.
In mathematical notation, it’s denoted as 50². To compute the square of 50, you multiply 50 by 50, resulting in 2500.
Calculating the square of 50 entails straightforward arithmetic, involving the multiplication of 50 by itself. This calculation is frequently encountered in various mathematical contexts, notably in geometry and algebra.
√50 = 7.071067811865475
or
√50 ≈ 7.071 up to three decimal places
The square root of 50, denoted as √50, is a fundamental mathematical concept representing the value that, when multiplied by itself, equals 50. Unlike whole numbers or fractions, the square root of 50 is an irrational number, meaning its decimal expansion is non-repeating and infinite. When approximated, the value of √50 is approximately 7.071067811865475. Despite its seemingly unpredictable decimal expansion, the square root of 50 is essential in various mathematical calculations, geometric analyses, and practical applications. Understanding this concept aids in problem-solving across disciplines like engineering, physics, and finance.
Square Root of 50: 7.071067811865475
Exponential Form: 50^½ or 50^0.5
Radical Form: √50
The square root of 50 is an irrational number
To understand why, let’s first define rational and irrational numbers.
Rational numbers are those expressible as a fraction of two integers, where the denominator is not zero, written as a/b.
Examples include 1/2, -3, and 5.
Irrational numbers, however, cannot be expressed as simple fractions of two integers. Their decimal representations are non-repeating and non-terminating.
Examples include √2, π (pi), and √3.
Square Root of 50 as Irrational:
When we calculate the square root of 50, we find it cannot be expressed as a fraction of two integers.
Its decimal expansion, approximately 7.071067811865475, continues infinitely without repeating a pattern.
Let’s simplify:
√50 = √(25 × 2) = √25 × √2 = 5 × √2
Since √2 is famously irrational, the product of a rational number (5) and an irrational number (√2) is also irrational.
In summary, the square root of 50 is irrational because it simplifies to an irrational number (√2) when multiplied by a rational number (5), resulting in an irrational product.
Finding the square root of a number can be achieved through various methods. Here are some common approaches to determine the value of the square root of 50:
These methods provide clear strategies for calculating the value of the square root of 50, catering to various skill levels and preferences in mathematical reasoning.
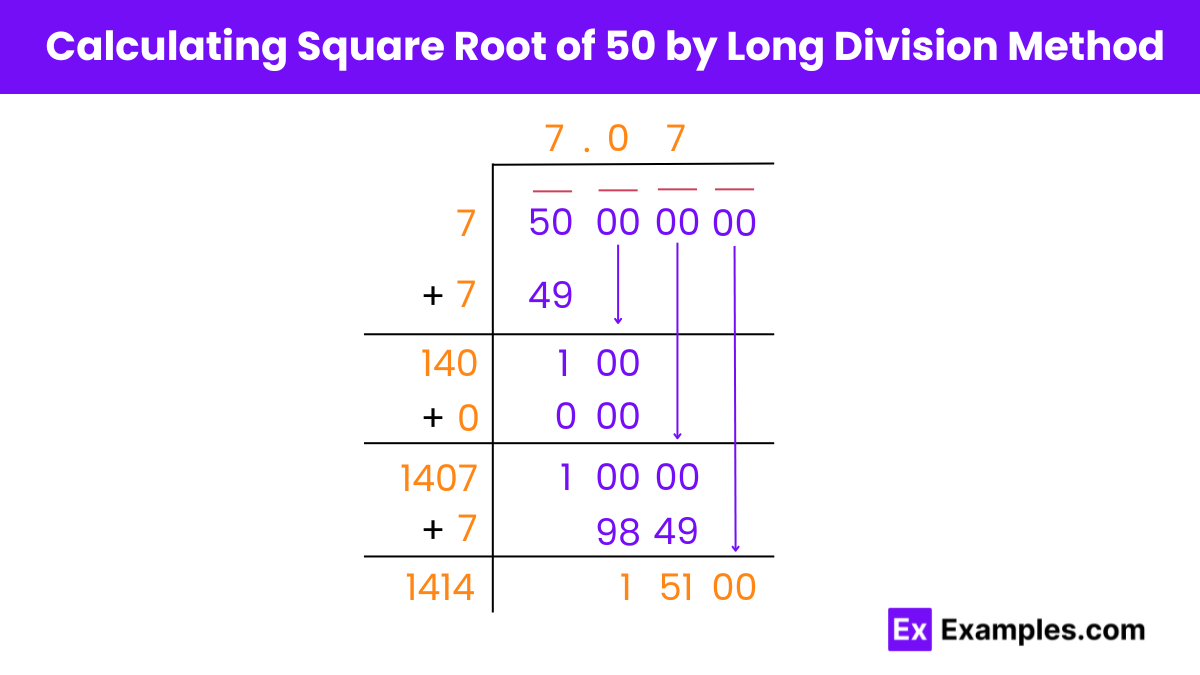
To accurately determine the square root of 50, which is not a perfect square and thus not a whole number, we employ the long division method. Follow these steps to find the value of √50:
Step 1: Place a decimal after 50 and add three pairs of zeros after it.
Step 2: Pair the digits and place a bar over them from left to right.
Step 3: Start with 50 as the dividend. Identify a number that, when multiplied by itself, yields a value equal to or less than 50. In this case, 7 x 7 = 49. Hence, 7 is the quotient.
Step 4: Add a decimal after 7 to divide the rest of the zeros after the decimal in the dividend part.
Step 5: Add 7 to the previous divisor to obtain a new divisor, resulting in 14.
Step 6: Using 14 as the divisor, determine a digit (let’s say A) that, when placed next to 14, results in a product (14A) less than or equal to 100. Since 140 x 0 = 0, the quotient remains 7.0.
Step 7: Add 0 to 140 to get the next divisor and write down the two zeros next to 100.
Step 8: Determine a digit (let’s say Y) that, when placed next to 140 (resulting in 140Y), yields a product less than or equal to 10,000. So, 1407 x 7 = 9849.
Step 9: The quotient is 7.07. This process can be repeated to obtain a more precise value.
By following these steps iteratively, we can achieve a progressively accurate approximation of the square root of 50. This method allows for the determination of the square root of non-perfect squares, facilitating precise calculations in various mathematical contexts.
A perfect square root is a number that can be expressed as the product of an integer multiplied by itself. For example, 4 (2 × 2) and 9 (3 × 3) are perfect square roots. To determine whether 50 is a perfect square root, we check if it can be written as the product of two identical integers.
However, upon examination, we find that there are no two integers x such that x × x equals 50. In other words, 50 cannot be expressed as the square of any integer.
Therefore, 50 is not a perfect square root. It does not have an integer square root. While it does have a square root (√50), it’s an irrational number and cannot be obtained by multiplying any whole number by itself.
In summary, 50 is not a perfect square root as it cannot be expressed as the product of two identical integers.
No, the square root of 50 is not a natural number. Natural numbers are whole numbers greater than zero, and the square root of 50, approximately equal to 7.071067811865475, is not a whole number.
The square root of 50 lies between the square roots of two consecutive perfect squares. The perfect squares closest to 50 are 49 (7²) and 64 (8²). Since 50 lies between 49 and 64, its square root lies between 7 and 8. Therefore, the square root of 50 is between 7 and 8.

The “square of 50” represents the outcome when the number 50 is multiplied by itself.
In mathematical notation, it’s denoted as 50². To compute the square of 50, you multiply 50 by 50, resulting in 2500.
Mathematically, 50² (50 × 50) = 2500
Calculating the square of 50 entails straightforward arithmetic, involving the multiplication of 50 by itself. This calculation is frequently encountered in various mathematical contexts, notably in geometry and algebra.
√50 = 7.071067811865475
or
√50 ≈ 7.071 up to three decimal places
The square root of 50, denoted as √50, is a fundamental mathematical concept representing the value that, when multiplied by itself, equals 50. Unlike whole numbers or fractions, the square root of 50 is an irrational number, meaning its decimal expansion is non-repeating and infinite. When approximated, the value of √50 is approximately 7.071067811865475. Despite its seemingly unpredictable decimal expansion, the square root of 50 is essential in various mathematical calculations, geometric analyses, and practical applications. Understanding this concept aids in problem-solving across disciplines like engineering, physics, and finance.
Square Root of 50: 7.071067811865475
Exponential Form: 50^½ or 50^0.5
Radical Form: √50
The square root of 50 is an irrational number
To understand why, let’s first define rational and irrational numbers.
Rational numbers are those expressible as a fraction of two integers, where the denominator is not zero, written as a/b.
Examples include 1/2, -3, and 5.
Irrational numbers, however, cannot be expressed as simple fractions of two integers. Their decimal representations are non-repeating and non-terminating.
Examples include √2, π (pi), and √3.
Square Root of 50 as Irrational:
When we calculate the square root of 50, we find it cannot be expressed as a fraction of two integers.
Its decimal expansion, approximately 7.071067811865475, continues infinitely without repeating a pattern.
Let’s simplify:
√50 = √(25 × 2) = √25 × √2 = 5 × √2
Since √2 is famously irrational, the product of a rational number (5) and an irrational number (√2) is also irrational.
In summary, the square root of 50 is irrational because it simplifies to an irrational number (√2) when multiplied by a rational number (5), resulting in an irrational product.
Finding the square root of a number can be achieved through various methods. Here are some common approaches to determine the value of the square root of 50:
Prime Factorization Method:
Decompose 50 into its prime factors: 2 × 5 × 5.
Group the prime factors: √(2 × 5 × 5).
Since 50 contains perfect square factors, simplify to obtain the square root in radical form.
Long Division Method:
Start with an initial approximation for the square root of 50.
Iteratively refine the approximation through successive long divisions.
Continue adjusting the approximation until achieving the desired level of accuracy.
Estimation Method:
Recognize that 50 is near the square of a whole number.
Identify nearby perfect squares: 49 (7²) and 64 (8²).
Since 50 lies between 49 and 64, estimate its square root to be between 7 and 8.
Babylonian Method (Heron’s Method):
Initiate with a tentative guess for the square root of 50, such as 7.
Utilize the Babylonian formula: xₙ₊₁=1/2(xₙ+40/xₙ) to iteratively enhance the guess.
Continue the iterations until reaching the desired precision level.
These methods provide clear strategies for calculating the value of the square root of 50, catering to various skill levels and preferences in mathematical reasoning.
To accurately determine the square root of 50, which is not a perfect square and thus not a whole number, we employ the long division method. Follow these steps to find the value of √50:
Step 1: Place a decimal after 50 and add three pairs of zeros after it.
Step 2: Pair the digits and place a bar over them from left to right.
Step 3: Start with 50 as the dividend. Identify a number that, when multiplied by itself, yields a value equal to or less than 50. In this case, 7 x 7 = 49. Hence, 7 is the quotient.
Step 4: Add a decimal after 7 to divide the rest of the zeros after the decimal in the dividend part.
Step 5: Add 7 to the previous divisor to obtain a new divisor, resulting in 14.
Step 6: Using 14 as the divisor, determine a digit (let’s say A) that, when placed next to 14, results in a product (14A) less than or equal to 100. Since 140 x 0 = 0, the quotient remains 7.0.
Step 7: Add 0 to 140 to get the next divisor and write down the two zeros next to 100.
Step 8: Determine a digit (let’s say Y) that, when placed next to 140 (resulting in 140Y), yields a product less than or equal to 10,000. So, 1407 x 7 = 9849.
Step 9: The quotient is 7.07. This process can be repeated to obtain a more precise value.
By following these steps iteratively, we can achieve a progressively accurate approximation of the square root of 50. This method allows for the determination of the square root of non-perfect squares, facilitating precise calculations in various mathematical contexts.
50 is Not a Perfect Square Root
A perfect square root is a number that can be expressed as the product of an integer multiplied by itself. For example, 4 (2 × 2) and 9 (3 × 3) are perfect square roots. To determine whether 50 is a perfect square root, we check if it can be written as the product of two identical integers.
However, upon examination, we find that there are no two integers x such that x × x equals 50. In other words, 50 cannot be expressed as the square of any integer.
Therefore, 50 is not a perfect square root. It does not have an integer square root. While it does have a square root (√50), it’s an irrational number and cannot be obtained by multiplying any whole number by itself.
In summary, 50 is not a perfect square root as it cannot be expressed as the product of two identical integers.
No, the square root of 50 is not a natural number. Natural numbers are whole numbers greater than zero, and the square root of 50, approximately equal to 7.071067811865475, is not a whole number.
The square root of 50 lies between the square roots of two consecutive perfect squares. The perfect squares closest to 50 are 49 (7²) and 64 (8²). Since 50 lies between 49 and 64, its square root lies between 7 and 8. Therefore, the square root of 50 is between 7 and 8.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 50?
2400
2500
2600
2700
What is the square root of 50 to the nearest tenth?
6.9
7.0
7.1
7.2
Which of the following is closest to the square root of 50?
6.5
7.0
7.5
8.0
Which expression represents the square root of 50?
5√2
5√3
6√2
6√3
What is the result when the square root of 50 is multiplied by 2?
10
10√2
10√3
15
If x² = 50 , what is x?
±5
±5√2
±6
±7
What is the approximate value of √50 + √50?
10
14
20
28
If √50 is between two consecutive integers, what are those integers?
6 and 7
7 and 8
8 and 9
9 and 10
What is the square of \frac{50}{10} ?
20
25
30
35
Which of the following represents the approximate value of \frac{50}{\sqrt{50}} ?
5
7
10
15
Before you leave, take our quick quiz to enhance your learning!

