Square & Square Root of 69 – Methods, Calculation, Formula, How to find

Square of 69

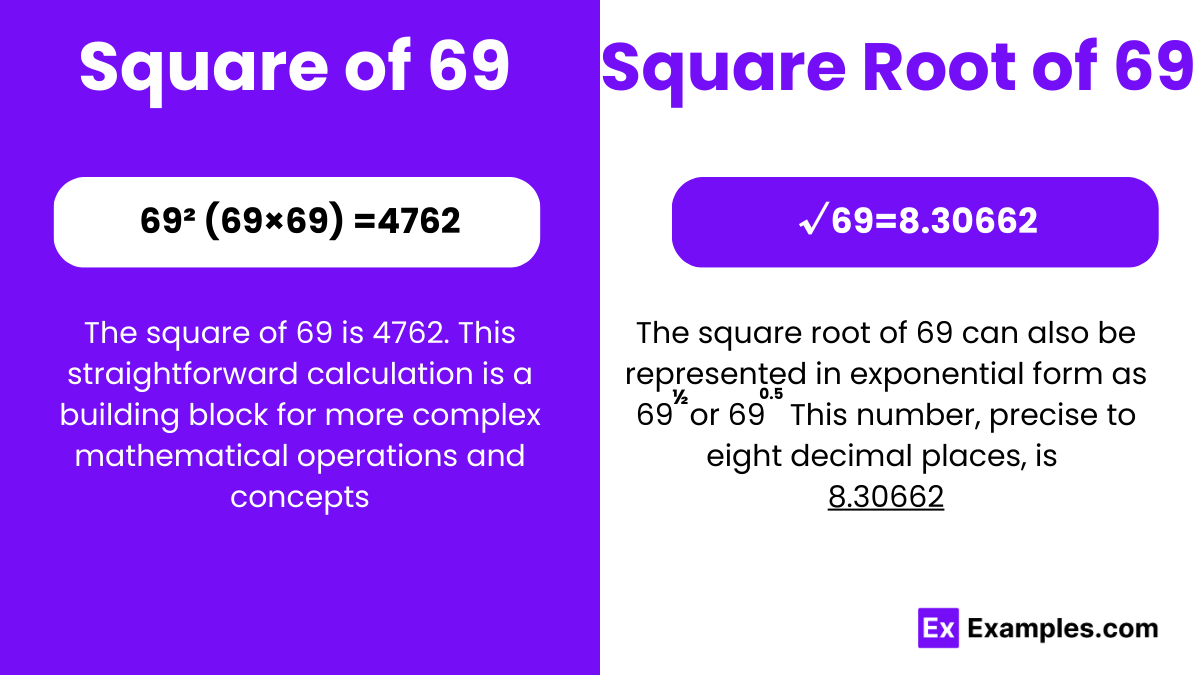
The square Number of 69 (69²) is the result of multiplying 69 by itself. In mathematical terms, 69 squared equals 4761.

Geometrically, this means that if you have a square with each side measuring 69 units, the total area enclosed by the square will be 4761 square units.

Understanding the square of 69 is important in various mathematical contexts, including geometry, algebra, and arithmetic. It’s a fundamental operation that finds applications in numerous real-world scenarios, such as calculating areas, volumes, distances, and solving mathematical problems.

Square Root of 69

Like the square root of 61, the square root of 69 is also an irrational number. This means it cannot be perfectly expressed as a fraction, and its decimal representation goes on without repeating.

Mathematically, √69 represents a number that, when squared, equals 69. Since 69 is not a perfect square, its square root is an irrational number, with its approximate value being around 8.30662. This value can be calculated through various numerical methods, such as the long division method, Newton’s method, or by using calculators or computer software designed for such mathematical computations.

In practical terms, the square root of 69 may come up in different fields including mathematics, engineering, physics, and others, where accurate measurements or complex calculations are involved.

Is the Square Root of 69 Rational or Irrational?

This means it cannot be expressed as a simple fraction where both the numerator and the denominator are integers. The decimal representation of the square root of 69 goes on indefinitely without repeating, which is a hallmark of irrational numbers.

The approximate value of the square root of 69 is about 8.30662386292, though it’s important to remember that this is only an approximation and the actual decimal expansion continues infinitely without repeating.

In various fields such as mathematics, engineering, physics, and others that require precise calculations, understanding the nature of numbers like √69 is crucial. Whether in solving equations, analyzing geometric shapes, or understanding the properties of materials, knowing that √69 is irrational helps in making accurate and meaningful interpretations.

Methods to Find Value of Root 69

Finding the square root of 69 can be approached using several different methods, much like how one would find the square root of 61. Here’s an overview of some common techniques:

Long Division Method : This manual method resembles traditional long division but is tailored for extracting square roots. It involves a process of subtracting perfect square numbers from the given number and bringing down pairs of digits step by step. The aim is to continue this process until you reach a desired level of precision, getting closer to the square root of 69 with each step.

Newton’s Method : Also known as the Newton-Raphson method, this is a numerical approach for finding the roots of real-valued functions. The method is iterative, meaning it refines the approximation of the square root through repeated application of a specific formula. With each iteration, the approximation becomes more accurate, converging towards the actual square root of 69.

Using a Calculator or Software : In the modern age, calculators and mathematical software offer a straightforward way to compute square roots. By simply entering √69 into a calculator or a mathematical software program, you can quickly obtain an accurate approximation of the square root. This method is by far the simplest and most accessible for most people.

Binomial Expansion : This method involves applying the binomial expansion theorem, which allows the expression of the square root as an infinite series. By truncating this series at a certain point, you can obtain an approximate value for the square root of 69. This method requires a good understanding of series and binomial expansion but offers a unique way to approach the problem.

Approximation with Known Square Roots : A more intuitive method involves using the square roots of known perfect squares that are close to 69, such as 64 (√64=8) and 81 (√81=9). By linearly interpolating between these known square roots, you can derive a rough estimate of the square root of 69. This method is less precise but can provide a quick ballpark figure.

Each of these methods offers a different pathway to approximate the square root of 69, ranging from manual calculations to quick estimates using digital tools.

Square Root of 69 by Long Division Method

Finding the square root of 69 using the long division method can be made simple with these steps:

Step 1: Pair the Digits: Since 69 is a two-digit number, we consider it as one pair. Put a bar over this pair to start.

Step 2: Find the Starting Number: Look for a number (let’s call it ‘a’) that when squared (a × a) is less than or equal to 69. For 69, this number ‘a’ is 8 because 8 × 8 = 64, which is the closest we can get to 69 without going over.

Step 3: Subtract and Find Remainder: Subtract 64 (8×8) from 69. The difference is 5, which is our remainder. Our starting quotient is 8 (since we used 8 × 8 to get close to 69).

Step 4: Double and Extend: Double the quotient we have (which is 8) to get 16. This is our new starting point for the next step. Then, to go further, add a decimal to 69 (making it 69.000) and to the quotient (making it 8.), and bring down two zeros to work with, making our new number to work with 500.

Step 5: Find the Next Number: Now, we need to find a new number (let’s call it ‘n’) to put next to 16, making a new number (16n), and then find n such that 16n × n is less than or equal to 500. For this step, ‘n’ would be 3 because 163 × 3 = 489, which is less than 500.

Step 6: Repeat as Needed: Add 3 to the quotient, making it 8.3. Now, you would subtract 489 from 500, get 11, bring down more zeros, and repeat the process to get more decimal places of the square root.

In a simpler way, you start with a guess (8 in this case), see how it fits, and then keep refining your guess by adding more digits to it (like the 3 we found), getting closer and closer to the actual square root with each step.

69 is Perfect Square root or Not?

A perfect square is a number that can be expressed as the product of an integer with itself. For example, 64 is a perfect square because it can be written as 8×88×8, and 81 is a perfect square because it is 9×99×9. Since there is no integer that, when multiplied by itself, equals 69, it is not considered a perfect square.