What is the square of 75?
5,625
7,500
6,250
5,000
To calculate the square of 75, you simply multiply 75 by itself:
Therefore, the square of 75 is 5625. This straightforward calculation is a building block for more complex mathematical operations and concepts, including algebraic equations, geometric formulas, and statistical models.
The square root of 75, denoted as √75, equals approximately 8.66. To compute it, you find the number that, when multiplied by itself, results in 75. In mathematical terms, finding the square root of a number means determining the number that, when raised to the power of 2 (squared), equals the original number. Visually, you can represent the square root of 75 as one side of a square with an area of 75 square units, where each side of the square is approximately 8.66 units in length. Understanding square roots is fundamental in various mathematical concepts and applications, such as geometry, algebra, and solving problems that involve areas or other quantities that are squared. In real-world scenarios, knowing the square root of 75 aids in calculations involving the measurement of areas or in any situation where you need to reverse a squaring operation to find an original quantity.
Exponential Form: 75^1/2 or 75^0.5
Radical Form: √75
To understand why, let’s break it down:
An irrational number is a number that cannot be expressed as a simple fraction (a ratio of two integers). In contrast, a rational number can be expressed as a fraction.
The square root of 75 simplifies to √75=√25×√3=5√3. Since √3 cannot be expressed as a fraction of two integers (it’s an endless, non-repeating decimal), it means that 5√3 is also irrational.
Thus, the square root of 75 is an irrational number because it cannot be written as a precise fraction or a decimal with a repeating or terminating sequence.
Finding the value of the square root of 75 (√75) can be approached in several ways, each varying in complexity and precision. Here are some methods:
1. Simplification Method
This involves simplifying √75 to a more manageable form by factoring out perfect squares.
This simplification shows that √75 is the same as 5 times the square root of 3, although it doesn’t give an exact decimal value.
2. Decimal Approximation Using a Calculator
For most practical purposes, you can use a calculator to find the square root of 75 directly.
3. Long Division Method
The long division method is a manual technique to find the square root of any number to any desired precision. It’s a bit complex and involves a step-by-step procedure similar to traditional long division.
4. By Using Prime Factorization
Prime factorization can also help in understanding the components of √75 but does not directly offer a numerical approximation.
Factor 75 into its prime components (as shown in the simplification method) and then draw the square root from those factors.
4. Estimation method
To estimate the value of the square root of 75, you can use a method that involves finding the nearest perfect squares around 75. Here’s a quick and effective way to do it:
A more precise estimate can be made by considering that 75 is about three-quarters of the way from 64 to 81 (since 64+34×(81−64)=76.7564+43×(81−64)=76.75, which is close to 75). So, we could estimate the square root of 75 to be about three-quarters of the way from 8 to 9, which is approximately 8+34×(9−8)=8.758+43×(9−8)=8.75.
However, the actual square root of 75 is roughly 8.66, which means our estimation method gets us pretty close to the actual value with minimal calculation!
Let’s find the square root of 75 using a simpler explanation of the long division method:
Start with 75: Write it as 75.000000, taking numbers in pairs from the right. So, we begin with 75 as our number to work on.
First division: Find a number that, when multiplied by itself, is close to or just less than 75. That number is 8 because 8 times 8 is 64. Subtract 64 from 75, and you get 11.
Double and continue: Double your current quotient (which is 8), getting 16. Then, we add a decimal to our quotient, making it 8., and bring down two zeros to make our new number 1100.
Next steps: Find a number to add to 160 (16 doubled plus this number as the new digit in our quotient), and when multiplied by the same number, it’s less than 1100. That’s 6, because 166 times 6 is 996. Subtract this from 1100, leaving us with 104.
Repeat: Bring down another pair of zeros to get 10400. Double the last quotient (now thinking of it as 86 to get 172), and find a number (6 again) that makes 1726 times 6 equal to 10356, just under 10400.
Keep going: Continue the process, adding zeros and finding the next digit until you’re satisfied with the accuracy. After these steps, we see that the square root of 75 is approximately 8.66, found through dividing and carefully choosing numbers.
This way, we’ve estimated the square root of 75 to be about 8.66, doing it step by step with long division.
A perfect square is a number that can be expressed as the square of an integer, and there is no whole number whose square equals 75.
The simplified form of √75 is 5√3. This simplification comes from factoring 75 into 25×3 and taking the square root of 25 out of the radical as 5.
No, 75 is not a perfect cube. A perfect cube is a number that can be expressed as the cube of an integer. The prime factorization of 75 doesn’t allow for this.
No, the square root of 75 does not terminate. It is an irrational number, which means its decimal representation goes on forever without repeating.

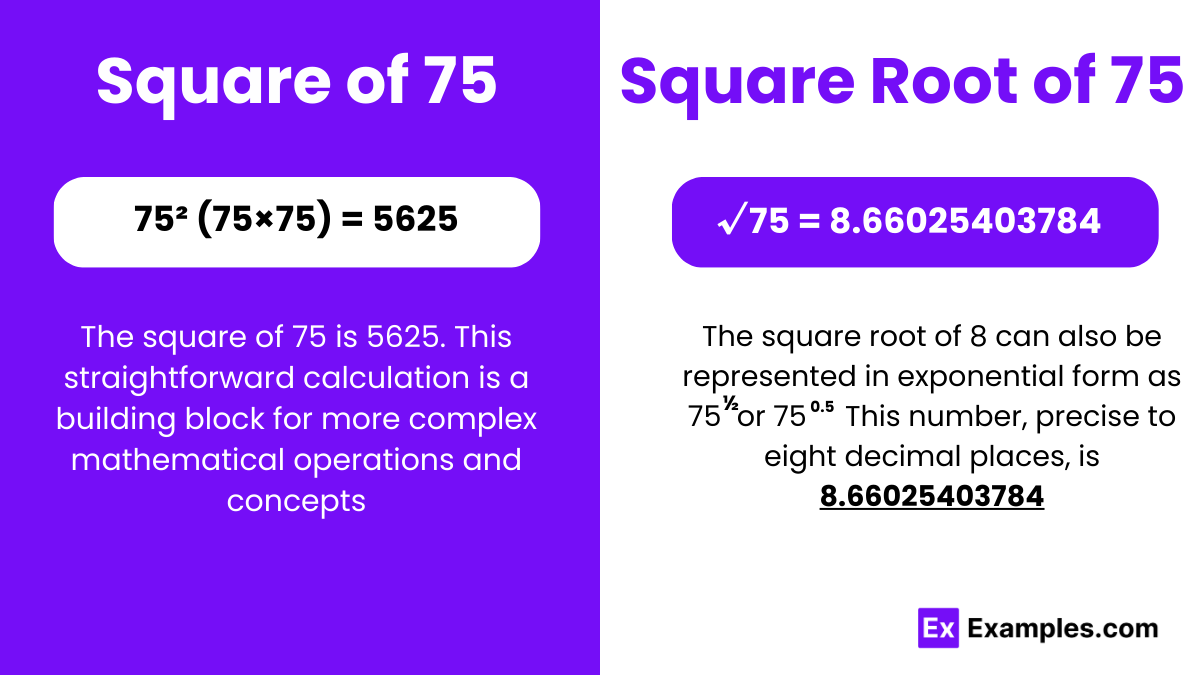
75² (75×75) = 5625
To calculate the square of 75, you simply multiply 75 by itself:
Therefore, the square of 75 is 5625. This straightforward calculation is a building block for more complex mathematical operations and concepts, including algebraic equations, geometric formulas, and statistical models.
√75 = 8.66025403784
The square root of 75, denoted as √75, equals approximately 8.66. To compute it, you find the number that, when multiplied by itself, results in 75. In mathematical terms, finding the square root of a number means determining the number that, when raised to the power of 2 (squared), equals the original number. Visually, you can represent the square root of 75 as one side of a square with an area of 75 square units, where each side of the square is approximately 8.66 units in length. Understanding square roots is fundamental in various mathematical concepts and applications, such as geometry, algebra, and solving problems that involve areas or other quantities that are squared. In real-world scenarios, knowing the square root of 75 aids in calculations involving the measurement of areas or in any situation where you need to reverse a squaring operation to find an original quantity.
Square Root of 75: “8.66025403784”
Exponential Form: 75^1/2 or 75^0.5
Radical Form: √75
A rational number is any number that can be expressed as a fraction a/b where a and b are integers, and b is not equal to zero. It includes integers, fractions, and finite or repeating decimals.
An irrational number is a number that cannot be expressed as a simple fraction. Irrational numbers have non-repeating, non-terminating decimal expansions.
The square root of 75 is irrational
To understand why, let’s break it down:
An irrational number is a number that cannot be expressed as a simple fraction (a ratio of two integers). In contrast, a rational number can be expressed as a fraction.
The square root of 75 simplifies to √75=√25×√3=5√3. Since √3 cannot be expressed as a fraction of two integers (it’s an endless, non-repeating decimal), it means that 5√3 is also irrational.
Thus, the square root of 75 is an irrational number because it cannot be written as a precise fraction or a decimal with a repeating or terminating sequence.
Finding the value of the square root of 75 (√75) can be approached in several ways, each varying in complexity and precision. Here are some methods:
1. Simplification Method
This involves simplifying √75 to a more manageable form by factoring out perfect squares.
Step 1: Factor 75 into its prime factors: 75 = 3 × 5².
Step 2: Simplify the square root: √75 = √(3 × 5²) = 5√3.
This simplification shows that √75 is the same as 5 times the square root of 3, although it doesn’t give an exact decimal value.
2. Decimal Approximation Using a Calculator
For most practical purposes, you can use a calculator to find the square root of 75 directly.
Simply enter 75 and press the square root button (√) to get an approximate decimal value, which is about 8.66.
3. Long Division Method
The long division method is a manual technique to find the square root of any number to any desired precision. It’s a bit complex and involves a step-by-step procedure similar to traditional long division.
You would divide 75 into pairs from the decimal point, estimate products, subtract, bring down the next pair of zeros, and continue the process until you reach the desired level of accuracy.
4. By Using Prime Factorization
Prime factorization can also help in understanding the components of √75 but does not directly offer a numerical approximation.
Factor 75 into its prime components (as shown in the simplification method) and then draw the square root from those factors.
4. Estimation method
To estimate the value of the square root of 75, you can use a method that involves finding the nearest perfect squares around 75. Here’s a quick and effective way to do it:
Identify the nearest perfect squares: The nearest perfect squares around 75 are 64 (which is 8282) and 81 (which is 9292). So, the square root of 75 is between 8 and 9.
Estimate the position of 75 between the squares: Since 75 is closer to 81 than to 64, its square root will be closer to 9 than to 8.
Use simple interpolation: 75 is 11 units away from 64 and 6 units away from 81. This means it’s closer to 81, so its square root will be closer to 9. You could roughly estimate it as leaning towards the higher number in the 8 to 9 range due to the proximity.
A more precise estimate can be made by considering that 75 is about three-quarters of the way from 64 to 81 (since 64+34×(81−64)=76.7564+43×(81−64)=76.75, which is close to 75). So, we could estimate the square root of 75 to be about three-quarters of the way from 8 to 9, which is approximately 8+34×(9−8)=8.758+43×(9−8)=8.75.
However, the actual square root of 75 is roughly 8.66, which means our estimation method gets us pretty close to the actual value with minimal calculation!
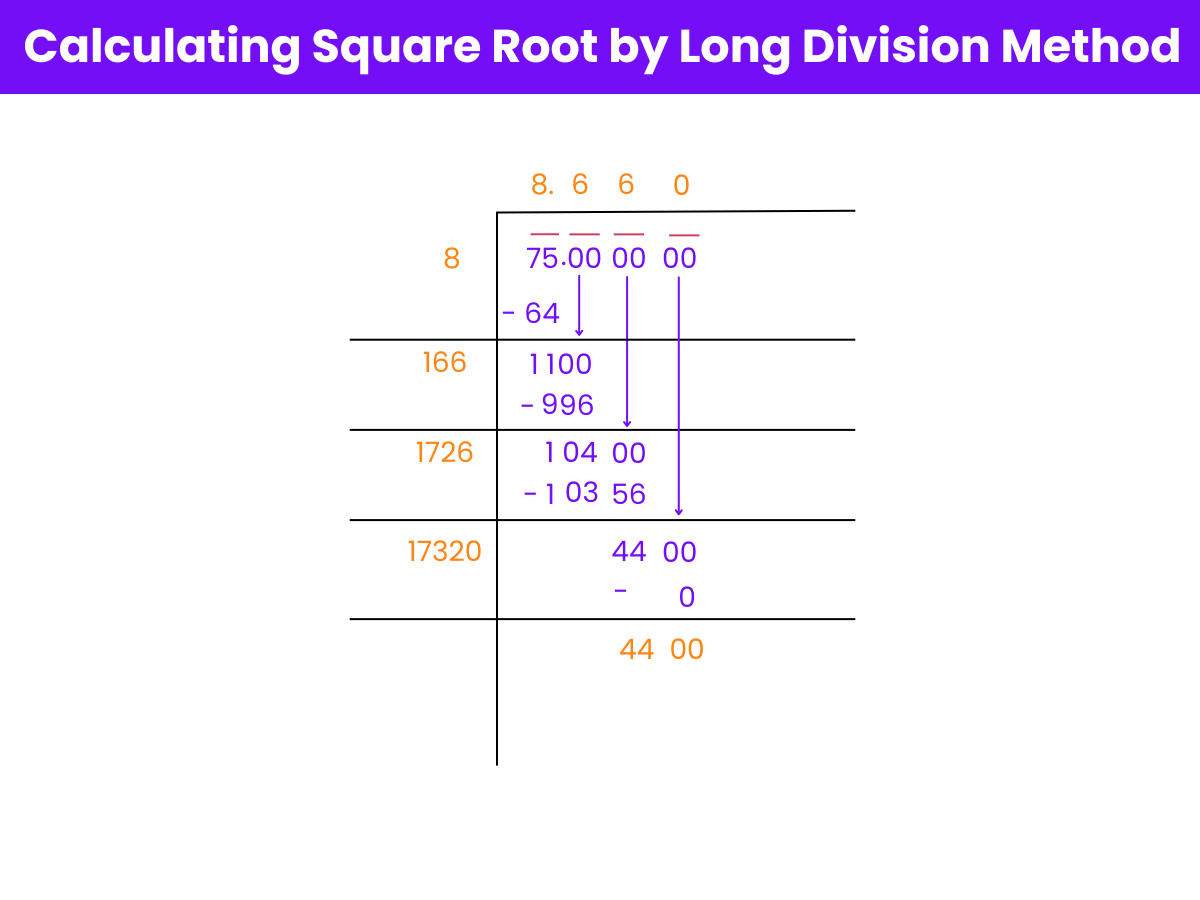
Let’s find the square root of 75 using a simpler explanation of the long division method:
Start with 75: Write it as 75.000000, taking numbers in pairs from the right. So, we begin with 75 as our number to work on.
First division: Find a number that, when multiplied by itself, is close to or just less than 75. That number is 8 because 8 times 8 is 64. Subtract 64 from 75, and you get 11.
Double and continue: Double your current quotient (which is 8), getting 16. Then, we add a decimal to our quotient, making it 8., and bring down two zeros to make our new number 1100.
Next steps: Find a number to add to 160 (16 doubled plus this number as the new digit in our quotient), and when multiplied by the same number, it’s less than 1100. That’s 6, because 166 times 6 is 996. Subtract this from 1100, leaving us with 104.
Repeat: Bring down another pair of zeros to get 10400. Double the last quotient (now thinking of it as 86 to get 172), and find a number (6 again) that makes 1726 times 6 equal to 10356, just under 10400.
Keep going: Continue the process, adding zeros and finding the next digit until you’re satisfied with the accuracy. After these steps, we see that the square root of 75 is approximately 8.66, found through dividing and carefully choosing numbers.
This way, we’ve estimated the square root of 75 to be about 8.66, doing it step by step with long division.
No, 75 is not a perfect square
A perfect square is a number that can be expressed as the square of an integer, and there is no whole number whose square equals 75.
The simplified form of √75 is 5√3. This simplification comes from factoring 75 into 25×3 and taking the square root of 25 out of the radical as 5.
No, 75 is not a perfect cube. A perfect cube is a number that can be expressed as the cube of an integer. The prime factorization of 75 doesn’t allow for this.
No, the square root of 75 does not terminate. It is an irrational number, which means its decimal representation goes on forever without repeating.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 75?
5,625
7,500
6,250
5,000
hat is the square root of 75 expressed in simplest radical form?
5√3
15√2
7√27
10√3
What is the approximate value of the square root of 75 to the nearest tenth?
8.7
8.5
8.2
8.1
If x² = 75, what is the value of x?
±7.5
±8.7
±5√3
±8.6
What is the value of 75 squared minus 75?
5,550
5,625
5,700
5,475
Which of the following is the closest integer to the square root of 75?
6
7
8
9
What is the square root of the sum of 75 and 25?
8
9
10
11
What is the value of 75 divided by its square root?
10
15
20
25
What is the product of the square root of 75 and 5?
25√3
50
75√3
20√3
What is the value of 2 × √75?
10√3
15√3
5√3
30
Before you leave, take our quick quiz to enhance your learning!

