What is the square of 85?
7025
7225
6525
7355
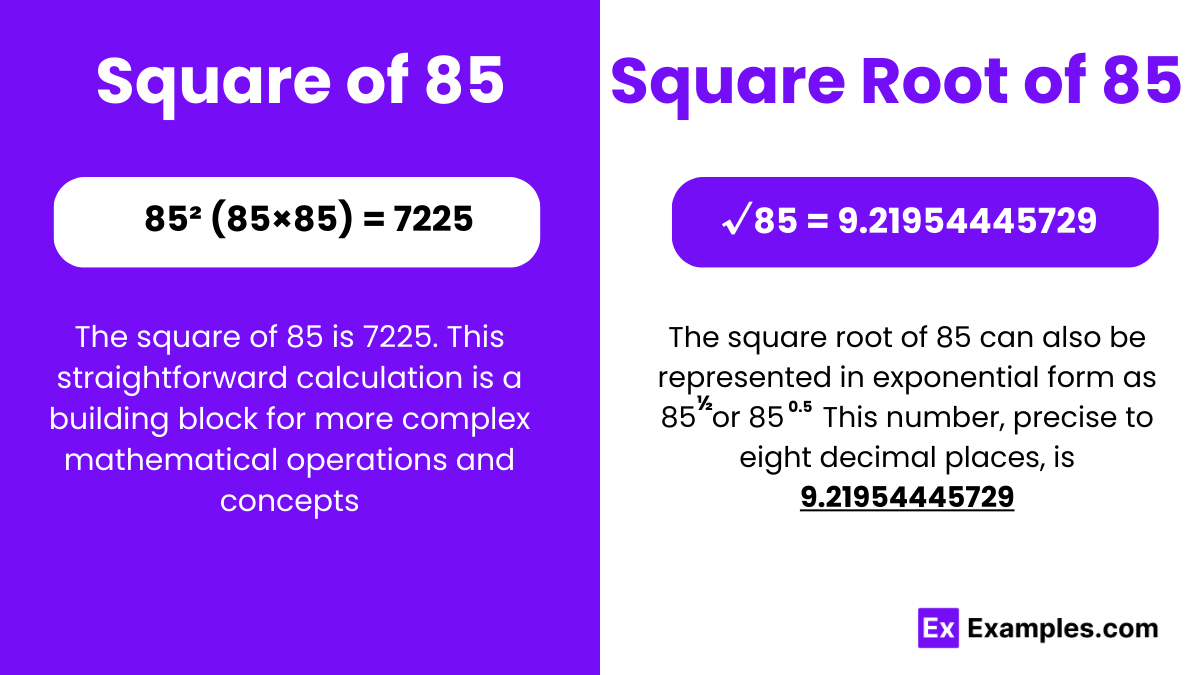
To calculate the square of 85, you simply multiply 85 by itself:
Therefore, the square of 85 is 7225. This straightforward calculation is a building block for more complex mathematical operations and concepts, including algebraic equations, geometric formulas, and statistical models.
The square root of 85, denoted as √85, approximately equals 9.2195. To compute it, you seek the number which, when multiplied by itself, results in 85. Mathematically, finding a square root means determining the number that raised to the power of 2 gives the original number. Visually, you can represent the square root of 85 as a square with sides of length √85 units, where the area of the square is 85 square units. Understanding square roots is crucial in various mathematical disciplines and real-world applications, such as geometry, algebra, and when determining the actual lengths or measures from area values. In practical scenarios, knowing the square root of 85 is useful for calculations involving the root extraction of squared quantities or measurements.
Exponential Form: 85^1/2 or 85^0.5
Radical Form: √85
This is because 85 cannot be expressed as a perfect square of any integer or rational number. In mathematics, an irrational number is one that cannot be expressed as a fraction ᵃ/ᵇ, where a and b are integers and b≠0. Irrational numbers have non-repeating, non-terminating decimal expansions. Since no fraction of integers squared equals 85, the square root of 85 does not resolve to a rational number and therefore is irrational.
Finding the value of the square root of 85 (√85) can be approached through several methods, each varying in complexity and precision. Here’s an overview of some common methods:
1. Trial and Error
This method involves guessing and checking. You try squaring numbers close to what you think the square root might be until you find two consecutive integers where one squared is less than 85 and the other squared is more than 85. This gives a rough estimate.
2. Long Division Method
A more traditional and manual method, similar to the division you learn in elementary school but adapted for extracting square roots. It’s a step-by-step procedure that helps you find a more accurate value by dividing and averaging, though it can be time-consuming.
3. Using a Calculator
The most straightforward and accurate method for most purposes. Modern calculators and computing devices can give the square root of any number instantly, with a degree of precision that’s adjustable or based on the device’s default settings.
4 .Estimation with Perfect Squares
Knowing that 81 has a square root of 9 (since 9^2 = 81) and 100 has a square root of 10 (since 10^2 = 100), you can estimate √85 to be between 9 and 10. Further refinement can be done by interpolation or additional reasoning about the distances between 81, 85, and 100.

To find the square root of 85 using the long division method, you can follow these simplified steps:
Start with a close perfect square: Choose 9 as your starting point because 9²=81, which is the closest perfect square less than 85.
Initial subtraction: Subtract 81 (which is 9×9) from 85 to get 44.
Form the new divisor: Double the current divisor (9) to get 18 and place it with a space next to it for the next digit.
Extend the division: Add a decimal point to your current answer (9), bring down 00 to make 400, and find a digit to place in the space that makes the new divisor’s product with it as close to 400 as possible without going over. In this case, 2 works because 182×2=364.
Repeat with precision: Subtract 364 from 400 to get 36, bring down another 00 to make 3600, update your divisor (add the 2 to 182 to get 184), and find the next digit that fits the method.
Continue as needed: Keep repeating the process, extending the division for more decimal places until you’re satisfied with the precision.
Through these steps, you incrementally approximate the square root of 85 to your desired level of accuracy.
A perfect square is a number that can be expressed as the square of an integer. Since there is no whole number that squared equals 85, it is not considered a perfect square.
The number closest to the square root of 85 is 9, since √85 is approximately 9.22. Nine is the nearest whole number to this value, making it the closest approximation.
The square root of 85 does not have factors in the traditional sense, as it is an irrational number. However, its approximation, 9.22, is not factorable into integers.
The cube root of 85 is approximately 4.39. This value represents the number that, when multiplied by itself three times (cubed), equals 85.

85² (85×85) = 7225
To calculate the square of 85, you simply multiply 85 by itself:
Therefore, the square of 85 is 7225. This straightforward calculation is a building block for more complex mathematical operations and concepts, including algebraic equations, geometric formulas, and statistical models.
√85 = 9.21954445729
The square root of 85, denoted as √85, approximately equals 9.2195. To compute it, you seek the number which, when multiplied by itself, results in 85. Mathematically, finding a square root means determining the number that raised to the power of 2 gives the original number. Visually, you can represent the square root of 85 as a square with sides of length √85 units, where the area of the square is 85 square units. Understanding square roots is crucial in various mathematical disciplines and real-world applications, such as geometry, algebra, and when determining the actual lengths or measures from area values. In practical scenarios, knowing the square root of 85 is useful for calculations involving the root extraction of squared quantities or measurements.
Square Root of 85: “9.21954445729”
Exponential Form: 85^1/2 or 85^0.5
Radical Form: √85
The square root of 85 is irrational
This is because 85 cannot be expressed as a perfect square of any integer or rational number. In mathematics, an irrational number is one that cannot be expressed as a fraction ᵃ/ᵇ, where a and b are integers and b≠0. Irrational numbers have non-repeating, non-terminating decimal expansions. Since no fraction of integers squared equals 85, the square root of 85 does not resolve to a rational number and therefore is irrational.
Finding the value of the square root of 85 (√85) can be approached through several methods, each varying in complexity and precision. Here’s an overview of some common methods:
1. Trial and Error
This method involves guessing and checking. You try squaring numbers close to what you think the square root might be until you find two consecutive integers where one squared is less than 85 and the other squared is more than 85. This gives a rough estimate.
2. Long Division Method
A more traditional and manual method, similar to the division you learn in elementary school but adapted for extracting square roots. It’s a step-by-step procedure that helps you find a more accurate value by dividing and averaging, though it can be time-consuming.
3. Using a Calculator
The most straightforward and accurate method for most purposes. Modern calculators and computing devices can give the square root of any number instantly, with a degree of precision that’s adjustable or based on the device’s default settings.
4 .Estimation with Perfect Squares
Knowing that 81 has a square root of 9 (since 9^2 = 81) and 100 has a square root of 10 (since 10^2 = 100), you can estimate √85 to be between 9 and 10. Further refinement can be done by interpolation or additional reasoning about the distances between 81, 85, and 100.
To find the square root of 85 using the long division method, you can follow these simplified steps:
Start with a close perfect square: Choose 9 as your starting point because 9²=81, which is the closest perfect square less than 85.
Initial subtraction: Subtract 81 (which is 9×9) from 85 to get 44.
Form the new divisor: Double the current divisor (9) to get 18 and place it with a space next to it for the next digit.
Extend the division: Add a decimal point to your current answer (9), bring down 00 to make 400, and find a digit to place in the space that makes the new divisor’s product with it as close to 400 as possible without going over. In this case, 2 works because 182×2=364.
Repeat with precision: Subtract 364 from 400 to get 36, bring down another 00 to make 3600, update your divisor (add the 2 to 182 to get 184), and find the next digit that fits the method.
Continue as needed: Keep repeating the process, extending the division for more decimal places until you’re satisfied with the precision.
Through these steps, you incrementally approximate the square root of 85 to your desired level of accuracy.
No, 85 is not a perfect square
A perfect square is a number that can be expressed as the square of an integer. Since there is no whole number that squared equals 85, it is not considered a perfect square.
The number closest to the square root of 85 is 9, since √85 is approximately 9.22. Nine is the nearest whole number to this value, making it the closest approximation.
The square root of 85 does not have factors in the traditional sense, as it is an irrational number. However, its approximation, 9.22, is not factorable into integers.
The cube root of 85 is approximately 4.39. This value represents the number that, when multiplied by itself three times (cubed), equals 85.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 85?
7025
7225
6525
7355
What is the square root of 85?
8.5
9.2
8.7
9.5
Which of the following is closest to the square root of 85?
8.6
8.7
8.8
8.5
What is the result of squaring the square root of 85?
85
85.5
90
80
If x² = 85, what is x approximately?
8.6
8.7
9.0
8.5
What is 85 raised to the power of 1/2?
8.5
8.6
8.7
8.8
What is the value of 85² - 85?
7225 - 85 = 7140
7225 - 85 = 7150
7225 - 85 = 7200
7225 - 85 = 7100
Which number is a perfect square close to 85?
81
64
100
121
What is the square of 8.5?
72.25
70.25
74.25
75.25
What is the approximate value of the square root of 84?
8.6
8.7
8.8
8.9
Before you leave, take our quick quiz to enhance your learning!

