What is the square of 98?
9604
9801
9600
9800

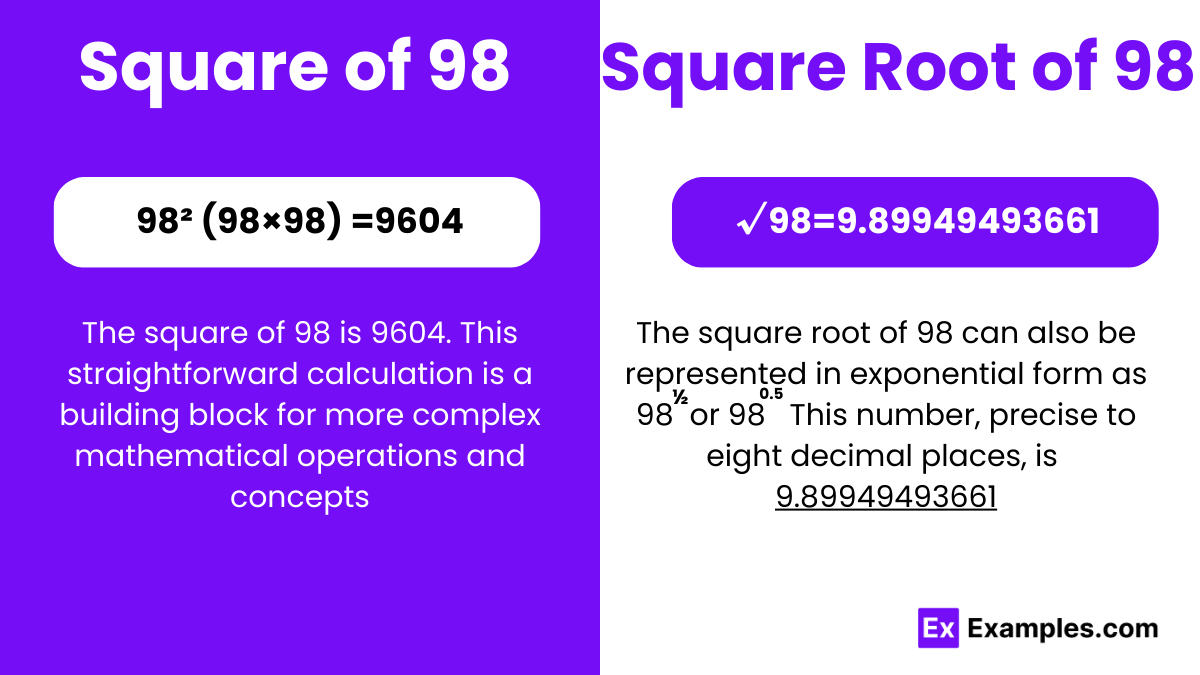
To calculate the square Number of 98, you multiply 98 by itself:
Therefore, the square of 98 is 9604. This calculation is done by multiplying 98 by itself, which results in 9604. Understanding how to find the square of a number is important for various mathematical applications, including algebra, geometry, and statistics, as it lays the groundwork for more complex equations and models.
The square root of 98 is an irrational number, similar to the square roots of numbers like 24, as it cannot be precisely expressed as a fraction of two integers. Its approximate value is 9.89949493661. This value is significant in various mathematical contexts, including geometry, where it represents the length of the side of a square with an area of 98 square units. Additionally, the square root of 98 finds applications in algebraic equations, engineering calculations, and physics formulas, playing a crucial role in understanding spatial relationships and numerical approximations.
Square Root of 98: 9.89949493661
Exponential Form: 98^0.5 or 98¹/²
Radical Form: √98
It cannot be expressed as a simple fraction of two integers. Its decimal representation is non-terminating and non-repeating, indicating its irrational nature. The approximate value of √98 is 9.89949493761. Like other irrational numbers, √98 plays a role in various mathematical contexts, including geometry, algebra, engineering, and physics, aiding in calculations and understanding spatial relationships.
There are several methods to find the value of the square root of 98:
Prime Factorization Method: Decompose 98 into its prime factors (2 × 7× 7). Take the square root of perfect squares within these factors (2) and multiply them together. The result is the square root of 98.
Estimation Method: Recognize that 98 is between the squares of two consecutive integers (9^2 = 81 and 10^2 = 100). Estimate that the square root of 98 is between 9 and 10, then refine the approximation through trial and error or other numerical methods.
Long Division Method: Employ the long division method to approximate the square root of 98. This involves a step-by-step process of division similar to long division for whole numbers.
Calculator or Software: Utilize a calculator or mathematical software to directly compute the square root of 98. Most modern calculators and software have built-in functions to find square roots.
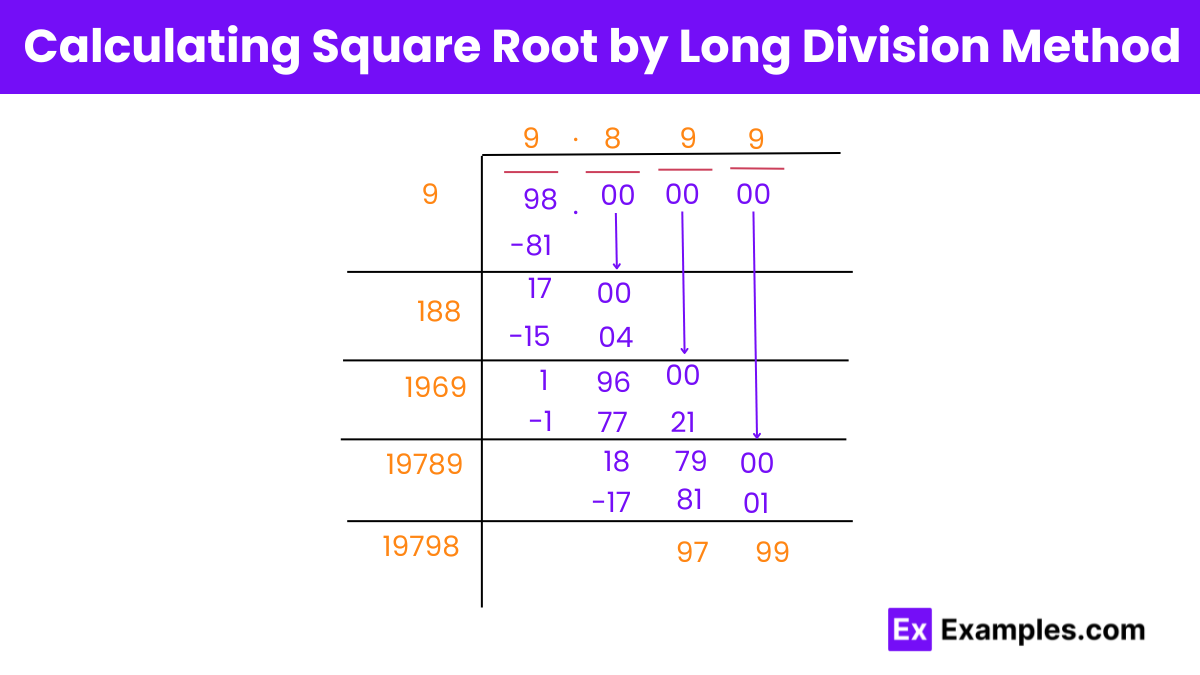
Step 1: Set Up: First, pair the digits of the number from right to left. Since 98 is a two-digit number, it will be treated as one pair, 98.
Step 2: Initial Guess: Start by finding the largest square number less than or equal to 98. The largest whole square less than 98 is 81, which is 9²
Subtraction: Subtract 81 from 98, which gives you 17.
Step 3:Bring Down Next Pair: Normally, you would bring down the next pair of digits, but since there are no more digits, you append a pair of zeros to the remainder, making it 1700.
Step 4: Finding the Next Digit
After subtracting 81 from 98 and bringing down a pair of zeros, we have 1700 as the new dividend. The divisor at this point is 9, which we then double. The doubled divisor is 18. Now, we need to find the next digit, which we’ll call Y. The number Y should be the highest digit such that 18Y×Y is less than or equal to 1700.
Step 5: Repeat Process: Place Y beside 18 making it 18Y, then multiply it by Y and subtract the result from 1700. Repeat this process by bringing down more pairs of zeros and continue the division.
Step 6:Continuation and Precision: You can continue this process to get more decimal places, each time bringing down a pair of zeros and finding the next digit.
A perfect squares is a number that can be expressed as the product of an integer multiplied by itself. For example, 9 is a perfect square because 3 × 3 = 9. However, there is no integer that, when multiplied by itself, gives 98. Therefore, 98 is not a perfect square.
√98=√2×7×7=√2×7²=7√2.
Factors of 98 are 1, 2, 7, 14, 49 and 98.

98² (98×98) = 9604
To calculate the square Number of 98, you multiply 98 by itself:
Therefore, the square of 98 is 9604. This calculation is done by multiplying 98 by itself, which results in 9604. Understanding how to find the square of a number is important for various mathematical applications, including algebra, geometry, and statistics, as it lays the groundwork for more complex equations and models.
√98 = 9.89949493661
The square root of 98 is an irrational number, similar to the square roots of numbers like 24, as it cannot be precisely expressed as a fraction of two integers. Its approximate value is 9.89949493661. This value is significant in various mathematical contexts, including geometry, where it represents the length of the side of a square with an area of 98 square units. Additionally, the square root of 98 finds applications in algebraic equations, engineering calculations, and physics formulas, playing a crucial role in understanding spatial relationships and numerical approximations.
Square Root of 98: 9.89949493661
Exponential Form: 98^0.5 or 98¹/²
Radical Form: √98
The square root of 98 is an irrational number.
It cannot be expressed as a simple fraction of two integers. Its decimal representation is non-terminating and non-repeating, indicating its irrational nature. The approximate value of √98 is 9.89949493761. Like other irrational numbers, √98 plays a role in various mathematical contexts, including geometry, algebra, engineering, and physics, aiding in calculations and understanding spatial relationships.
There are several methods to find the value of the square root of 98:
Prime Factorization Method: Decompose 98 into its prime factors (2 × 7× 7). Take the square root of perfect squares within these factors (2) and multiply them together. The result is the square root of 98.
Estimation Method: Recognize that 98 is between the squares of two consecutive integers (9^2 = 81 and 10^2 = 100). Estimate that the square root of 98 is between 9 and 10, then refine the approximation through trial and error or other numerical methods.
Long Division Method: Employ the long division method to approximate the square root of 98. This involves a step-by-step process of division similar to long division for whole numbers.
Calculator or Software: Utilize a calculator or mathematical software to directly compute the square root of 98. Most modern calculators and software have built-in functions to find square roots.
Step 1: Set Up: First, pair the digits of the number from right to left. Since 98 is a two-digit number, it will be treated as one pair, 98.
Step 2: Initial Guess: Start by finding the largest square number less than or equal to 98. The largest whole square less than 98 is 81, which is 9²
Subtraction: Subtract 81 from 98, which gives you 17.
Step 3:Bring Down Next Pair: Normally, you would bring down the next pair of digits, but since there are no more digits, you append a pair of zeros to the remainder, making it 1700.
Step 4: Finding the Next Digit
After subtracting 81 from 98 and bringing down a pair of zeros, we have 1700 as the new dividend. The divisor at this point is 9, which we then double. The doubled divisor is 18. Now, we need to find the next digit, which we’ll call Y. The number Y should be the highest digit such that 18Y×Y is less than or equal to 1700.
Step 5: Repeat Process: Place Y beside 18 making it 18Y, then multiply it by Y and subtract the result from 1700. Repeat this process by bringing down more pairs of zeros and continue the division.
Step 6:Continuation and Precision: You can continue this process to get more decimal places, each time bringing down a pair of zeros and finding the next digit.
No, 98 is not a perfect square.
A perfect squares is a number that can be expressed as the product of an integer multiplied by itself. For example, 9 is a perfect square because 3 × 3 = 9. However, there is no integer that, when multiplied by itself, gives 98. Therefore, 98 is not a perfect square.
√98=√2×7×7=√2×7²=7√2.
Factors of 98 are 1, 2, 7, 14, 49 and 98.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 98?
9604
9801
9600
9800
What is the approximate value of the square root of 98?
9.8
9.9
10.0
10.1
Which number is closest to the square of 98?
9500
9600
9700
9800
If x^2 = 98 , what is the approximate value of x ?
9.8
9.9
10.0
10.1
Which statement is true about the square root of 98?
It is a whole number.
It is a rational number.
It is an irrational number.
It is an integer.
What is the result of 98 squared minus 100?
9504
9604
9404
9704
Which of the following is the closest integer to the square root of 98?
9
10
11
12
What is the difference between 10000 and the square of 98?
396
404
406
424
If the square root of a number is approximately 9.9, what is the number?
98
100
101
102
Which value represents the closest approximation for the square root of 98 when rounded to one decimal place?
9.8
9.9
10.0
10.1
Before you leave, take our quick quiz to enhance your learning!

