What is the square of 1521?
23184
22944
23100
23121

Within algebraic studies, the significance of squares and square roots is foundational. Squaring, exemplified by multiplying a number like 1521 by itself to yield 2313441, is fundamental. This operation is key in exploring properties of rational and irrational numbers. Understanding these concepts enriches comprehension of mathematical relationships and patterns. In algebraic mathematics, squares and square roots are pivotal. Squaring, illustrated by the multiplication of 1521 by itself to produce 2313441, serves as a cornerstone. This process underpins investigations into rational and irrational numbers. A grasp of these fundamental concepts enhances understanding of mathematical relationships and patterns, facilitating deeper comprehension within algebraic studies.
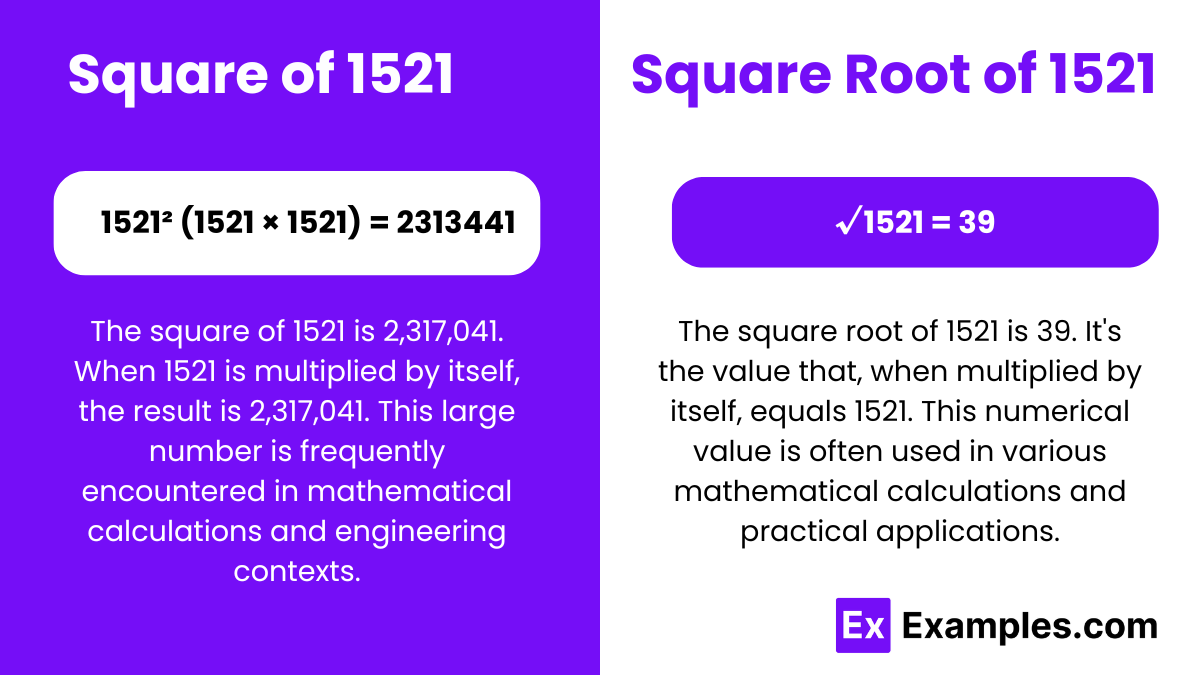
A square number, like 1521, is the result of multiplying an integer by itself. The square of 1521 equals 2,313,441. Understanding square numbers elucidates fundamental mathematical concepts, serving as a basis for exploring mathematical patterns and relationships, crucial in algebraic studies and beyond.
The square root of 1521, a square number, is 39. Understanding square roots entails deciphering the number that, when multiplied by itself, yields the original value. Mastery of square roots is fundamental in mathematics, laying the groundwork for comprehending algebraic concepts and unlocking deeper insights into numerical relationships and patterns.
Square Root of 1521: 39
Exponential Form: 1521^½ or 1521^0.5
Radical Form: √1521
The square root of 1521 is a rational number because it equals 39, which can be expressed as a fraction of two integers (39/1). Rational numbers can be written as a quotient of two integers where the denominator is not zero.
Rational Numbers: Rational numbers are expressible as the quotient of two integers, where the denominator isn’t zero. They’re represented as 𝑎𝑏, where 𝑎 and 𝑏 are integers and 𝑏≠0.
Examples include fractions like 1/2, -3, and 5/5.
Irrational Numbers: Irrational numbers can’t be written as fractions of integers. Their decimal representations are non-repeating and non-terminating.
Examples include square roots of non-perfect squares like √2, √3, √5, and transcendental numbers like π (pi).
In summary, rational numbers have finite or repeating decimals, while irrational numbers have non-repeating, non-terminating decimal expansions.
There are several methods to find the square root of 1521:
Prime Factorization Method: Express 1521 as a product of prime factors (3² × 11²), then take the square root of each prime factor.
Long Division Method: Iterate through digits of 1521, pairing them off and finding the largest number whose square is less than or equal to the current remainder.
Estimation Method: Use approximation techniques like the Newton-Raphson method to iteratively approach the square root of 1521.
Calculator: Simply input 1521 into a calculator and press the square root button to obtain the result directly.
Long Division Method for Finding Square Root of 1521
Step 1: Digit Pairing
Pair digits of the given number starting from the right, indicating with a horizontal bar.
Step 2: Initial Quotient
Find a number whose square is less than or equal to the first paired digits (15). Since 3² = 9 < 15, the quotient is 3. The difference is calculated as 15 – 9 = 6.
Step 3: Bringing Down
Bring down the next pair of digits (21) and multiply the quotient (3) by 2 to get 6, which is the starting digit of the new divisor.
Step 4: Further Division
Place the quotient (9) obtained from the previous step at the one’s place of the new divisor (69). Multiply 69 by 9 to get 621. The remainder is 0.
Step 5: Final Result
Since the remainder is 0, the process terminates. Thus, the square root of 1521 is 39.
Yes, the number 1521 is a perfect square
Yes, 1521 is a perfect square. Its square root is 39, meaning that 39 multiplied by itself equals 1521. In mathematical terms, 1521 = 39², confirming that it is indeed a perfect square.
The square of 1521 is a positive integer, while its square root is a positive rational number.
You can verify your calculation by squaring the square root value obtained. If the result is 1521, then your calculation is correct.
Understanding squares and square roots is essential in various fields such as engineering, physics, computer science, and finance for tasks like calculating areas, distances, and determining quantities.
No, the square root of 1521, which is 39, is already in its simplest form because it is a perfect square.
No, 1521 is not a prime number because it has factors other than 1 and itself.
Understanding squares and square roots serves as a foundational step in algebra, helping to comprehend concepts like equations, inequalities, and polynomial functions.

Within algebraic studies, the significance of squares and square roots is foundational. Squaring, exemplified by multiplying a number like 1521 by itself to yield 2313441, is fundamental. This operation is key in exploring properties of rational and irrational numbers. Understanding these concepts enriches comprehension of mathematical relationships and patterns. In algebraic mathematics, squares and square roots are pivotal. Squaring, illustrated by the multiplication of 1521 by itself to produce 2313441, serves as a cornerstone. This process underpins investigations into rational and irrational numbers. A grasp of these fundamental concepts enhances understanding of mathematical relationships and patterns, facilitating deeper comprehension within algebraic studies.
1521² (1521 × 1521) = 2313441
A square number, like 1521, is the result of multiplying an integer by itself. The square of 1521 equals 2,313,441. Understanding square numbers elucidates fundamental mathematical concepts, serving as a basis for exploring mathematical patterns and relationships, crucial in algebraic studies and beyond.
√1521 = 39
The square root of 1521, a square number, is 39. Understanding square roots entails deciphering the number that, when multiplied by itself, yields the original value. Mastery of square roots is fundamental in mathematics, laying the groundwork for comprehending algebraic concepts and unlocking deeper insights into numerical relationships and patterns.
Square Root of 1521: 39
Exponential Form: 1521^½ or 1521^0.5
Radical Form: √1521
Yes, the square root of 1521 is a rational number
The square root of 1521 is a rational number because it equals 39, which can be expressed as a fraction of two integers (39/1). Rational numbers can be written as a quotient of two integers where the denominator is not zero.
Rational Numbers: Rational numbers are expressible as the quotient of two integers, where the denominator isn’t zero. They’re represented as 𝑎𝑏, where 𝑎 and 𝑏 are integers and 𝑏≠0.
Examples include fractions like 1/2, -3, and 5/5.
Irrational Numbers: Irrational numbers can’t be written as fractions of integers. Their decimal representations are non-repeating and non-terminating.
Examples include square roots of non-perfect squares like √2, √3, √5, and transcendental numbers like π (pi).
In summary, rational numbers have finite or repeating decimals, while irrational numbers have non-repeating, non-terminating decimal expansions.
There are several methods to find the square root of 1521:
Prime Factorization Method: Express 1521 as a product of prime factors (3² × 11²), then take the square root of each prime factor.
Long Division Method: Iterate through digits of 1521, pairing them off and finding the largest number whose square is less than or equal to the current remainder.
Estimation Method: Use approximation techniques like the Newton-Raphson method to iteratively approach the square root of 1521.
Calculator: Simply input 1521 into a calculator and press the square root button to obtain the result directly.

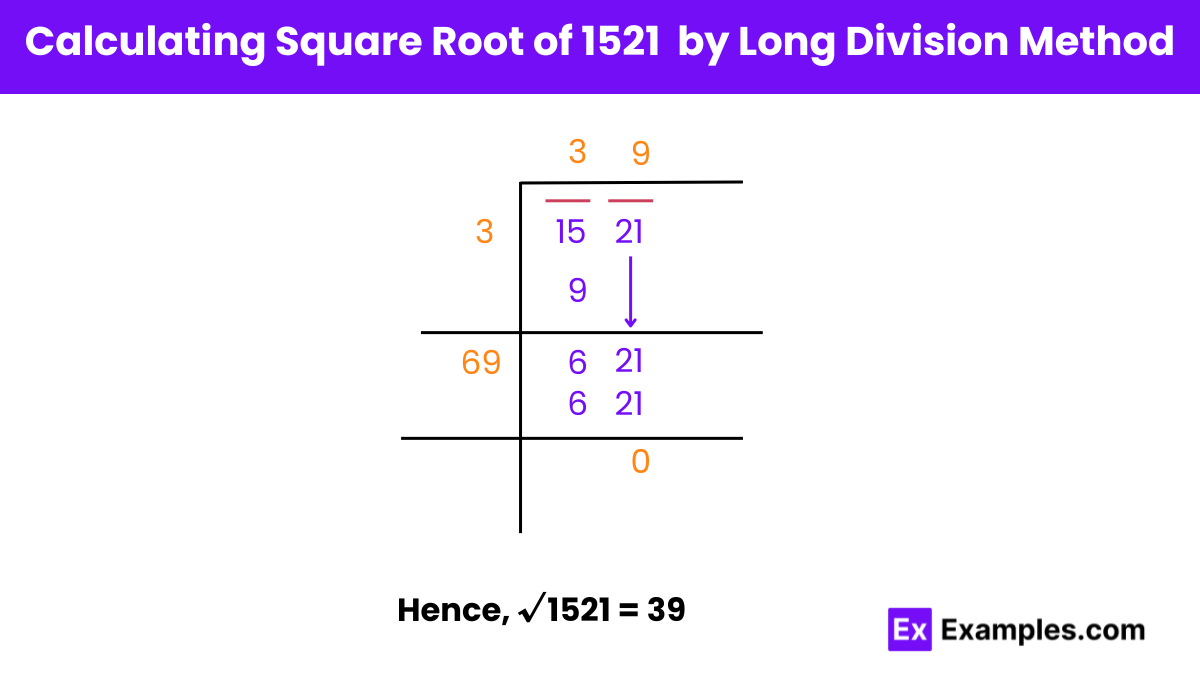
Long Division Method for Finding Square Root of 1521
Step 1: Digit Pairing
Pair digits of the given number starting from the right, indicating with a horizontal bar.
Step 2: Initial Quotient
Find a number whose square is less than or equal to the first paired digits (15). Since 3² = 9 < 15, the quotient is 3. The difference is calculated as 15 – 9 = 6.
Step 3: Bringing Down
Bring down the next pair of digits (21) and multiply the quotient (3) by 2 to get 6, which is the starting digit of the new divisor.
Step 4: Further Division
Place the quotient (9) obtained from the previous step at the one’s place of the new divisor (69). Multiply 69 by 9 to get 621. The remainder is 0.
Step 5: Final Result
Since the remainder is 0, the process terminates. Thus, the square root of 1521 is 39.
Yes, the number 1521 is a perfect square
Yes, 1521 is a perfect square. Its square root is 39, meaning that 39 multiplied by itself equals 1521. In mathematical terms, 1521 = 39², confirming that it is indeed a perfect square.
The square of 1521 is a positive integer, while its square root is a positive rational number.
You can verify your calculation by squaring the square root value obtained. If the result is 1521, then your calculation is correct.
Understanding squares and square roots is essential in various fields such as engineering, physics, computer science, and finance for tasks like calculating areas, distances, and determining quantities.
No, the square root of 1521, which is 39, is already in its simplest form because it is a perfect square.
No, 1521 is not a prime number because it has factors other than 1 and itself.
Understanding squares and square roots serves as a foundational step in algebra, helping to comprehend concepts like equations, inequalities, and polynomial functions.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 1521?
23184
22944
23100
23121
What is the square root of 1521?
28
30
39
43
If x² = 1521, what is the value of x?
37
38
39
40
What is the product of 39 and its square root?
1521
1560
1400
1600
Find the value of 1521 − 39².
0
100
50
200
If y² = 1521, what is y?
39
40
41
42
How is 1521 related to 39 in terms of squaring?
1521 is the square of 39.
1521 is one less than the square of 39.
1521 is one more than the square of 39.
1521 is double the square of 39.
What is √1521 when expressed in simplest form?
39
40
41
42
If you square the number 38, what is the result compared to 1521?
1521 is greater.
1521 is less.
1521 is equal.
1521 is much less.
Which number squared gives a result closest to 1521?
38
39
40
41
Before you leave, take our quick quiz to enhance your learning!

