Which of the following is the general form of the bending equation?
M/I = σ/y = E/R
M/I = σ/R = E/y
M/R = σ/I = E/y
M/I = σ/y = R/E

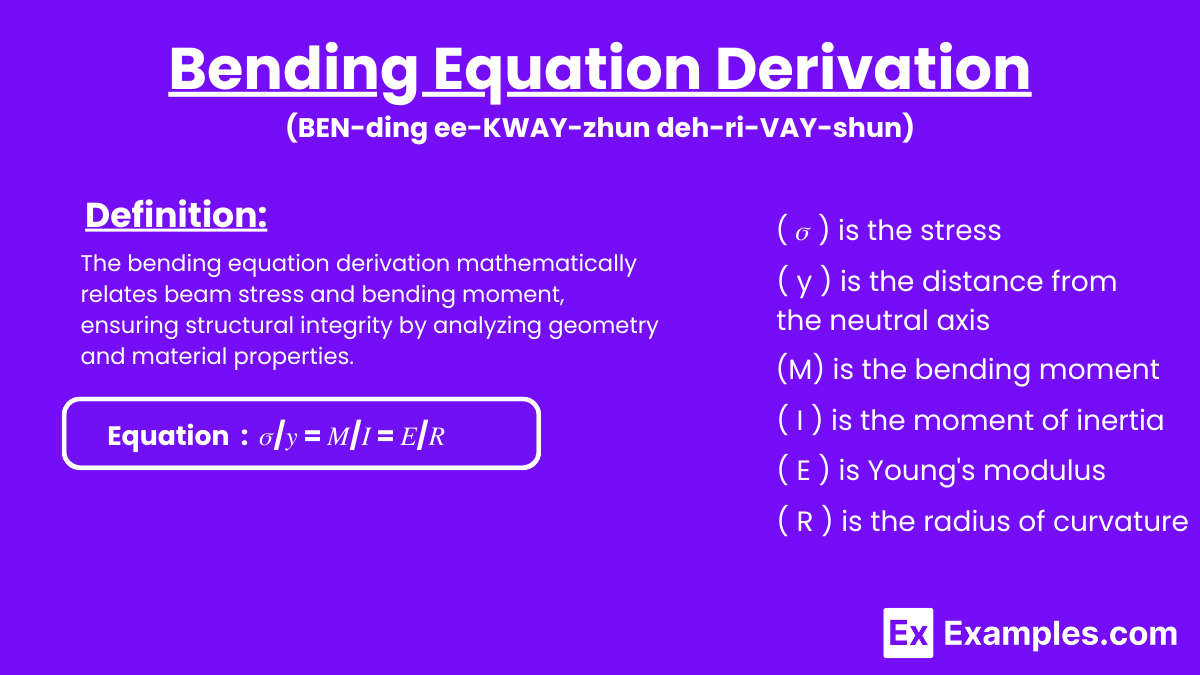
The bending equation is fundamental in structural engineering, relating the internal stress in a beam to its curvature under a load. Derived from the assumptions of linear elasticity, small deformations, and plane sections remaining plane, the equation is expressed as (𝜎/𝑦 = 𝑀/𝐼 = 𝐸/𝑅). Here, (𝜎) is the stress, (y) is the distance from the neutral axis, (M) is the bending moment, (I) is the moment of inertia, (E) is Young’s modulus, and (R) is the radius of curvature. This relationship helps in designing beams to ensure they can withstand applied loads without failing.
The bending stress equation, which relates the internal stress in a beam subjected to bending to the applied moment and the beam’s geometry, is expressed as:
σ = Mᵧ/I
where:
This equation helps engineers determine the maximum stress within a beam to ensure it remains within safe limits under loading conditions.
Consider a Beam Segment:
A small segment of a beam under bending has a length ( dx ).
Neutral Axis:
Define the neutral axis where no longitudinal stress occurs during bending.
Curvature of the Beam:
Radius of curvature of the neutral axis is ( R ).
Longitudinal Strain:
At a distance ( y ) from the neutral axis:
( ϵ = Δl/l = R/y )
Longitudinal Stress:
Hooke’s Law gives:
[ σ = Eϵ = Ey/r ]
Bending Moment:
The internal bending moment ( M )
M=∫AσydA]
Substituting ( σ ):
[ M = ∫AE y/r)ydA = E/R∫Ay²dA]
[ M = E/R I ]
Where ( I =∫Ay² dA ) is the moment of inertia.
Bending Equation:
[ M/I = E/R ]
Relating stress ((σ))
[ σ = Ey/r} ]
Substituting ( 1/R)
[ σ = My/r ]
[σ/y = M/I = E/R ]
This is the bending equation, relating internal stress, bending moment, and beam geometry.
The bending equation is derived under several key assumptions to simplify the analysis and ensure accurate results. These assumptions are:
Material Homogeneity and Isotropy : The beam material is uniform in composition and properties, and its mechanical properties are the same in all directions.
Linear Elasticity : The material obeys Hooke’s Law, meaning stress is proportional to strain within the elastic limit of the material.
Small Deformations : The deformations of the beam are small enough that the original geometry of the beam is not significantly altered. This allows the linear relationships between stress, strain, and bending moment to hold.
Plane Sections Remain Plane : Sections of the beam that are plane and perpendicular to the neutral axis before bending remain plane and perpendicular to the neutral axis after bending. This implies that there is no warping or distortion of the cross-sections.
Neutral Axis Stays Unstressed : The neutral axis, which is the line within the beam where the fiber experiences no longitudinal stress or strain, remains unchanged in length during bending.
No Shear Deformations : The shear deformations are neglected, meaning the bending stresses are dominant, and the influence of shear stresses is minimal.
Beam Geometry : The cross-sectional dimensions of the beam are small compared to its length, ensuring that the beam theory (Euler-Bernoulli beam theory) applies effectively.
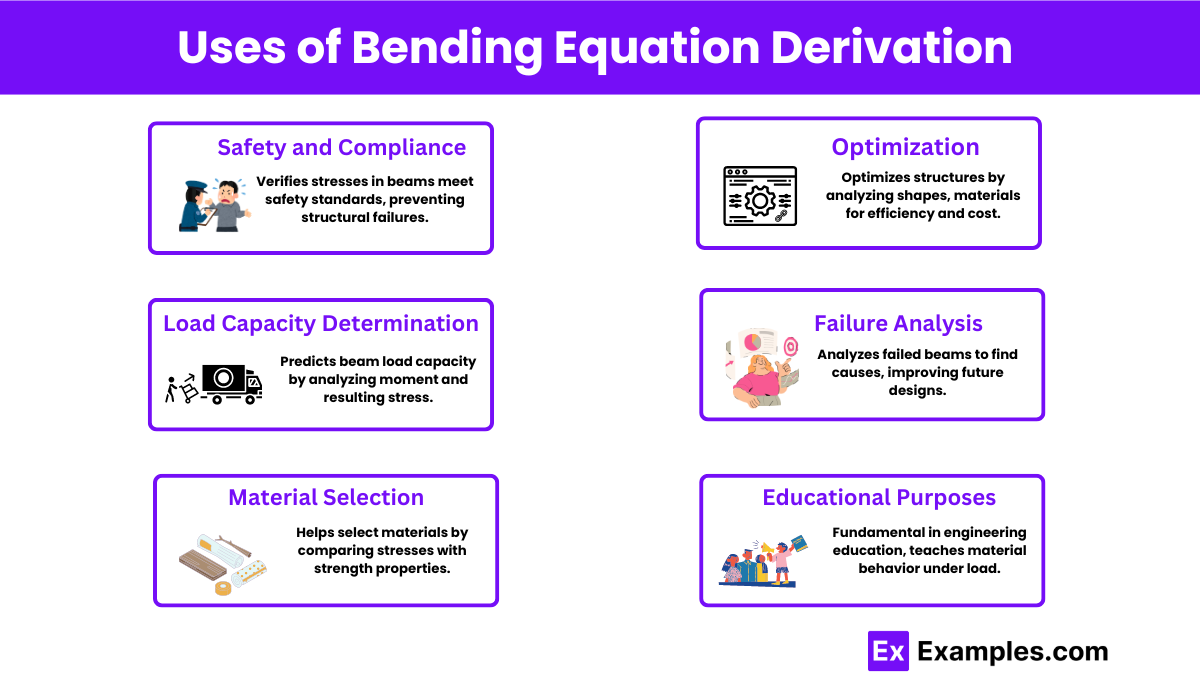
The bending equation derivation has several important uses in engineering and structural analysis, providing a fundamental tool for understanding and designing beam structures. Here are some key uses:
Structural Design : Helps engineers design beams and other structural elements to ensure they can safely withstand applied loads without failure. By calculating the maximum bending stress, engineers can select appropriate materials and cross-sectional dimensions.
Safety and Compliance : Ensures that structures comply with safety standards and building codes. By verifying that the stresses in a beam do not exceed the material’s allowable stress, the bending equation helps prevent structural failures.
Load Capacity Determination : Determines the load-carrying capacity of beams and structural members. By understanding the relationship between the applied moment and the resulting stress, engineers can predict how much load a beam can support before yielding or breaking.
Material Selection : Assists in selecting suitable materials for construction. By comparing the calculated stresses with the material’s strength properties, engineers can choose materials that provide adequate safety margins.
Deflection Analysis : In conjunction with other equations, it helps predict the deflection of beams under various loading conditions. Deflection analysis is crucial for ensuring that beams do not deform excessively, which could compromise structural integrity or functionality.
Optimization : Enables the optimization of structural elements for weight, cost, and performance. By analyzing different cross-sectional shapes and materials, engineers can design efficient structures that meet performance criteria while minimizing material usage.
Failure Analysis : Used in failure analysis to investigate the causes of structural failures. By analyzing the stresses and moments in a failed beam, engineers can identify the reasons for failure and improve future designs.
Educational Purposes : Serves as a fundamental concept in engineering education, helping students understand the behavior of materials and structures under load, and forming the basis for more advanced studies in structural engineering and mechanics.
Overall, the bending equation is a crucial tool in ensuring the safety, efficiency, and reliability of structural designs in various engineering applications.
Consider a cantilever beam with one end fixed and the other end free. A point load is applied at the free end of the beam. To determine the maximum bending stress at the fixed end, start by calculating the bending moment, which is greatest at the fixed end. The moment of inertia for a rectangular cross-section, along with the maximum distance from the neutral axis, are used in the bending stress equation. By substituting the calculated values into this equation, you can find the maximum bending stress at the fixed end of the beam.
Imagine a simply supported beam with supports at both ends and a load distributed evenly along its length. The maximum bending moment in this scenario occurs at the midpoint of the beam. To find the maximum bending stress, you need the moment of inertia for the beam’s cross-section and the maximum distance from the neutral axis. These values are then substituted into the bending stress equation. This process reveals the maximum bending stress at the midpoint of the beam.
Consider a simply supported beam with a circular cross-section and a point load applied at its midpoint. The maximum bending moment also occurs at the midpoint. The moment of inertia for a circular cross-section and the maximum distance from the neutral axis are determined. Using these values in the bending stress equation allows you to calculate the maximum bending stress at the midpoint of the beam.
These examples demonstrate the practical application of the bending equation to determine the maximum bending stress in beams subjected to different loading conditions. By understanding these principles, engineers can ensure that beams are designed to withstand the applied loads safely.
It helps determine the stress distribution within a beam under load, ensuring the design is safe and effective.
Assumptions include linear elasticity, small deformations, homogeneous material, plane sections remaining plane, and no shear deformations.
It relates the bending stress in a beam to the bending moment and the geometric properties of the beam’s cross-section
The moment of inertia (𝐼) quantifies the beam’s resistance to bending and appears in the denominator of the stress formula.
Young’s modulus (E) represents the material’s stiffness and is part of the relationship between stress and curvature.
The bending moment (𝑀M) is calculated based on the applied load and the distance from the point of interest along the beam.
The neutral axis is the line within the beam where the fibers experience no longitudinal stress or strain during bending.
By ensuring that the calculated stress does not exceed the material’s allowable stress, appropriate materials can be chosen.
It allows engineers to design beams that can withstand applied loads without exceeding material strength limits.
The equation assumes linear elasticity, small deformations, and does not account for shear deformations or large deflections.

The bending equation is fundamental in structural engineering, relating the internal stress in a beam to its curvature under a load. Derived from the assumptions of linear elasticity, small deformations, and plane sections remaining plane, the equation is expressed as (𝜎/𝑦 = 𝑀/𝐼 = 𝐸/𝑅). Here, (𝜎) is the stress, (y) is the distance from the neutral axis, (M) is the bending moment, (I) is the moment of inertia, (E) is Young’s modulus, and (R) is the radius of curvature. This relationship helps in designing beams to ensure they can withstand applied loads without failing.
The bending stress equation, which relates the internal stress in a beam subjected to bending to the applied moment and the beam’s geometry, is expressed as:
σ = Mᵧ/I
where:
(σ) is the bending stress at a distance (y) from the neutral axis,
(M) is the applied bending moment,
(y) is the perpendicular distance from the neutral axis to the point where the stress is being calculated,
(I) is the moment of inertia of the cross-sectional area about the neutral axis.
This equation helps engineers determine the maximum stress within a beam to ensure it remains within safe limits under loading conditions.
Consider a Beam Segment:
A small segment of a beam under bending has a length ( dx ).
Neutral Axis:
Define the neutral axis where no longitudinal stress occurs during bending.
Curvature of the Beam:
Radius of curvature of the neutral axis is ( R ).
Longitudinal Strain:
At a distance ( y ) from the neutral axis:
( ϵ = Δl/l = R/y )
Longitudinal Stress:
Hooke’s Law gives:
[ σ = Eϵ = Ey/r ]
Bending Moment:
The internal bending moment ( M )
M=∫AσydA]
Substituting ( σ ):
[ M = ∫AE y/r)ydA = E/R∫Ay²dA]
[ M = E/R I ]
Where ( I =∫Ay² dA ) is the moment of inertia.
Bending Equation:
[ M/I = E/R ]
Relating stress ((σ))
[ σ = Ey/r} ]
Substituting ( 1/R)
[ σ = My/r ]
[σ/y = M/I = E/R ]
This is the bending equation, relating internal stress, bending moment, and beam geometry.
The bending equation is derived under several key assumptions to simplify the analysis and ensure accurate results. These assumptions are:
Material Homogeneity and Isotropy : The beam material is uniform in composition and properties, and its mechanical properties are the same in all directions.
Linear Elasticity : The material obeys Hooke’s Law, meaning stress is proportional to strain within the elastic limit of the material.
Small Deformations : The deformations of the beam are small enough that the original geometry of the beam is not significantly altered. This allows the linear relationships between stress, strain, and bending moment to hold.
Plane Sections Remain Plane : Sections of the beam that are plane and perpendicular to the neutral axis before bending remain plane and perpendicular to the neutral axis after bending. This implies that there is no warping or distortion of the cross-sections.
Neutral Axis Stays Unstressed : The neutral axis, which is the line within the beam where the fiber experiences no longitudinal stress or strain, remains unchanged in length during bending.
No Shear Deformations : The shear deformations are neglected, meaning the bending stresses are dominant, and the influence of shear stresses is minimal.
Beam Geometry : The cross-sectional dimensions of the beam are small compared to its length, ensuring that the beam theory (Euler-Bernoulli beam theory) applies effectively.

The bending equation derivation has several important uses in engineering and structural analysis, providing a fundamental tool for understanding and designing beam structures. Here are some key uses:
Structural Design : Helps engineers design beams and other structural elements to ensure they can safely withstand applied loads without failure. By calculating the maximum bending stress, engineers can select appropriate materials and cross-sectional dimensions.
Safety and Compliance : Ensures that structures comply with safety standards and building codes. By verifying that the stresses in a beam do not exceed the material’s allowable stress, the bending equation helps prevent structural failures.
Load Capacity Determination : Determines the load-carrying capacity of beams and structural members. By understanding the relationship between the applied moment and the resulting stress, engineers can predict how much load a beam can support before yielding or breaking.
Material Selection : Assists in selecting suitable materials for construction. By comparing the calculated stresses with the material’s strength properties, engineers can choose materials that provide adequate safety margins.
Deflection Analysis : In conjunction with other equations, it helps predict the deflection of beams under various loading conditions. Deflection analysis is crucial for ensuring that beams do not deform excessively, which could compromise structural integrity or functionality.
Optimization : Enables the optimization of structural elements for weight, cost, and performance. By analyzing different cross-sectional shapes and materials, engineers can design efficient structures that meet performance criteria while minimizing material usage.
Failure Analysis : Used in failure analysis to investigate the causes of structural failures. By analyzing the stresses and moments in a failed beam, engineers can identify the reasons for failure and improve future designs.
Educational Purposes : Serves as a fundamental concept in engineering education, helping students understand the behavior of materials and structures under load, and forming the basis for more advanced studies in structural engineering and mechanics.
Overall, the bending equation is a crucial tool in ensuring the safety, efficiency, and reliability of structural designs in various engineering applications.
Consider a cantilever beam with one end fixed and the other end free. A point load is applied at the free end of the beam. To determine the maximum bending stress at the fixed end, start by calculating the bending moment, which is greatest at the fixed end. The moment of inertia for a rectangular cross-section, along with the maximum distance from the neutral axis, are used in the bending stress equation. By substituting the calculated values into this equation, you can find the maximum bending stress at the fixed end of the beam.
Imagine a simply supported beam with supports at both ends and a load distributed evenly along its length. The maximum bending moment in this scenario occurs at the midpoint of the beam. To find the maximum bending stress, you need the moment of inertia for the beam’s cross-section and the maximum distance from the neutral axis. These values are then substituted into the bending stress equation. This process reveals the maximum bending stress at the midpoint of the beam.
Consider a simply supported beam with a circular cross-section and a point load applied at its midpoint. The maximum bending moment also occurs at the midpoint. The moment of inertia for a circular cross-section and the maximum distance from the neutral axis are determined. Using these values in the bending stress equation allows you to calculate the maximum bending stress at the midpoint of the beam.
These examples demonstrate the practical application of the bending equation to determine the maximum bending stress in beams subjected to different loading conditions. By understanding these principles, engineers can ensure that beams are designed to withstand the applied loads safely.
It helps determine the stress distribution within a beam under load, ensuring the design is safe and effective.
Assumptions include linear elasticity, small deformations, homogeneous material, plane sections remaining plane, and no shear deformations.
It relates the bending stress in a beam to the bending moment and the geometric properties of the beam’s cross-section
The moment of inertia (𝐼) quantifies the beam’s resistance to bending and appears in the denominator of the stress formula.
Young’s modulus (E) represents the material’s stiffness and is part of the relationship between stress and curvature.
The bending moment (𝑀M) is calculated based on the applied load and the distance from the point of interest along the beam.
The neutral axis is the line within the beam where the fibers experience no longitudinal stress or strain during bending.
By ensuring that the calculated stress does not exceed the material’s allowable stress, appropriate materials can be chosen.
It allows engineers to design beams that can withstand applied loads without exceeding material strength limits.
The equation assumes linear elasticity, small deformations, and does not account for shear deformations or large deflections.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which of the following is the general form of the bending equation?
M/I = σ/y = E/R
M/I = σ/R = E/y
M/R = σ/I = E/y
M/I = σ/y = R/E
In the bending equation M/I = σ/y = E/R, what does M represent?
Moment of inertia
Modulus of elasticity
Bending moment
Radius of curvature
What does \'I\' stand for in the bending equation M/I = σ/y = E/R?
Modulus of elasticity
Moment of inertia
Stress
Distance from the neutral axis
How is the neutral axis defined in the context of bending?
Line of maximum stress
Line of zero stress
Line of maximum curvature
Line of maximum displacement
What is the significance of the radius of curvature (R) in the bending equation?
It indicates the stiffness of the material
It represents the amount of deflection
It describes the curvature of the bent beam
It measures the bending stress
What does \'E\' represent in the bending equation M/I = σ/y = E/R?
Bending moment
Modulus of elasticity
Stress
Distance from the neutral axis
What is the relationship between the stress (σ) and the distance from the neutral axis (y) in the bending equation?
σ is directly proportional to y
σ is inversely proportional to y
σ is independent of y
σ is directly proportional to y²
How does the moment of inertia (I) affect the bending stress in a beam?
Increasing I increases bending stress
Increasing I decreases bending stress
I has no effect on bending stress
I affects bending stress non-linearly
What physical property does the modulus of elasticity (E) describe in the context of bending?
Density of the material
Stiffness of the material
Strength of the material
Ductility of the material
In which direction does the maximum bending stress occur in a beam under bending?
At the neutral axis
Along the entire length uniformly
In the middle of the cross-section
At the top and bottom surfaces
Before you leave, take our quick quiz to enhance your learning!

