What is the general form of the one-dimensional wave equation?
∂²u/∂t² = c² ∂²u/∂x²
∂u/∂t = c ∂u/∂x
∂u/∂t² = c ∂u/∂x²
∂²u/∂t = c² ∂²u/∂x


The one-dimensional wave equation is fundamental in understanding wave phenomena in various fields such as acoustics, electromagnetics, and fluid dynamics. It describes how waves propagate in a medium over time. The derivation starts from Newton’s second law applied to a small segment of a vibrating string. By considering the forces due to tension and the resulting acceleration, we can express the transverse displacement as a function of position and time. This approach leads to the formation of a partial differential equation that characterizes wave motion
One-dimensional waves are characterized by their propagation in a single spatial dimension, with time being the primary independent variable. These waves can be observed in various phenomena, such as sound waves, ocean waves, vibrations from musical instruments, and electromagnetic radiation. In classical physics, waves are studied within the realms of mechanics, acoustics, and optics.
A wave is defined as a disturbance that travels through a medium, transferring energy from one point to another. When the disturbance is singular, it is referred to as a pulse. When the disturbance is repetitive, it is known as a periodic wave. The medium through which the wave travels consists of a series of interconnected particles. These particles exhibit wave-like behavior by interacting with each other, which enables the disturbance to propagate through the medium.
Mechanical Waves : Mechanical waves require a medium to travel through, such as air, water, or solids. Examples include sound waves, ocean waves, and seismic waves. These waves propagate by causing the particles of the medium to oscillate.
Electromagnetic Waves : Electromagnetic waves do not require a medium and can travel through a vacuum. They are produced by the oscillation of electric and magnetic fields. Examples include light waves, radio waves, and X-rays.
Transverse Waves : In transverse waves, the particles of the medium move perpendicular to the direction of wave propagation. Examples include waves on a string and electromagnetic waves.
Longitudinal Waves : Longitudinal waves have particles that oscillate parallel to the direction of wave travel. Sound waves in air and compression waves in a slinky are typical examples.
Surface Waves : Surface waves travel along the interface between two different media, such as air and water. Ocean waves are a common example, combining both transverse and longitudinal motion.
The wave equation is a fundamental mathematical description of how waves propagate through a medium. It is a partial differential equation that relates the spatial and temporal variations of a wave. In one dimension, the wave equation is given by:
∂²y/∂t² = v²∂²y/∂x²
where 𝑦(𝑥,𝑡) represents the displacement of the wave at position x and time t, and 𝑣 is the speed of the wave. This equation applies to both mechanical and electromagnetic waves and is crucial for understanding wave behavior in various physical contexts.
The one-dimensional wave equation describes how waves propagate along a string or similar medium. This derivation uses Newton’s second law and principles of calculus.
Take a small segment of the string with length Δx. Let T be the tension in the string, which is assumed to be constant. The segment experiences forces due to the tension at its ends.
The vertical components of the tension at the ends of the segment can be expressed as:
Tsin(θ1) ≈ T∂y/∂x∣ₓ
𝑇sin(𝜃2) ≈ 𝑇∂𝑦/∂𝑥∣ₓ₊Δₓ
where ( θ1 ) and ( θ2 ) are the angles the string makes with the horizontal at ( x ) and ( x +Δx ), respectively. For small angles, sin(θ) ≈ tan(θ).
The net vertical force ( F ) on the segment is:
F = 𝑇∂𝑦/∂𝑥∣ₓ₊Δₓ ] – T∂y/∂x∣ₓ
Using the definition of the derivative:
[ F = T 𝑇∂𝑦/∂𝑥∣ₓ₊Δₓ ] – ∂y/∂x∣ₓ ≈ T∂²y/∂x²Δₓ
According to Newton’s second law, the net force is equal to the mass of the segment times its acceleration:
F=m∂²𝑦/∂t²
The mass ( m ) of the segment is:
m = ∂Δx
where ( ∂ ) is the mass per unit length. Therefore:
∂²y/∂x² = ∂²y/∂t²
Divide through by (Δₓ )
∂²y/∂x² = μ∂²y/∂t²
Rearrange to get the standard form of the one-dimensional wave equation:
∂²y/∂t² = ∂²y/∂x²
Define the wave speed ( v ) as:
v = √t/μ
Thus, the wave equation becomes:
∂²y/∂t² = v²∂²y/∂x²
This equation describes the propagation of waves along a string or similar medium, where ( v ) is the speed of the wave, ( y(x,t) ) is the displacement, ( x ) is the position, and ( t ) is the time.
Hooke’s Law is a fundamental principle in physics that describes the behavior of springs and other elastic materials. It states that the force required to extend or compress a spring by some distance is proportional to that distance. Mathematically, Hooke’s Law is expressed as:
𝐹=−𝑘𝑥
where:
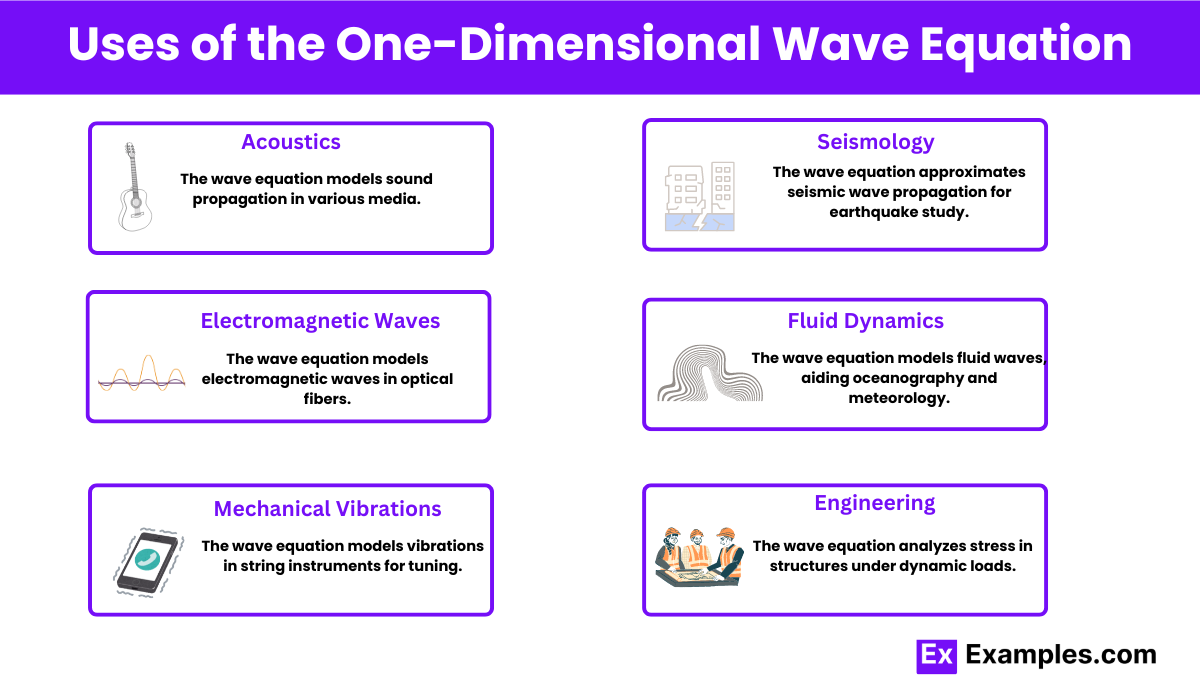
Acoustics : The wave equation describes how sound waves propagate through different media, such as air, water, and solids. It helps in designing acoustical systems, musical instruments, and understanding speech and hearing.
Electromagnetic Waves : The wave equation is used to understand the propagation of electromagnetic waves in one-dimensional structures like optical fibers and waveguides. This is essential in telecommunications and fiber-optic technologies.
Mechanical Vibrations : The wave equation models the vibrations of strings in musical instruments like guitars and pianos. It helps in designing and tuning these instruments for desired sound quality.
Seismology : The propagation of seismic waves through the Earth can be approximated using the one-dimensional wave equation. This aids in the study of earthquakes and the structure of the Earth’s interior.
Fluid Dynamics : The wave equation is used to model waves in fluids, such as waves on the surface of water and internal waves within ocean layers. This has applications in oceanography and meteorology.
Engineering : Engineers use the wave equation to analyze the stress and strain in structures subjected to dynamic loads, such as bridges and buildings during earthquakes or wind loads.
To derive the one-dimensional wave equation for a vibrating string, we start by considering a small segment of the string with a given length and constant tension. The vertical components of the tension at the ends of this segment can be approximated by considering how the displacement changes along the string. The net vertical force acting on this segment is the difference between these components. Using Newton’s second law, we equate this net force to the mass of the segment times its acceleration. By simplifying and dividing by the segment length, we derive the wave equation, which describes how waves propagate along the string.
For sound waves in a tube, consider a small segment of air within a long, narrow tube. The net force on this air segment arises from pressure differences at its ends. This force can be expressed using the pressure gradient and the tube’s cross-sectional area. Applying Newton’s second law, we relate this net force to the mass of the air segment and its acceleration. By using the relationship between pressure variations and particle displacement, we substitute into our equation and simplify. This leads to the wave equation for sound waves, which describes how sound propagates through the air in the tube.
The equation can model sound waves, waves on a string, seismic waves, and certain electromagnetic waves in confined structures.
Newton’s second law is used to relate the net force acting on a segment of the medium to its mass and acceleration.
Tension provides the restoring force that acts to return the string to its equilibrium position, crucial for wave propagation.
The vertical components are approximated using the partial derivatives of the displacement with respect to position.
Partial derivatives are used to express how the displacement changes with respect to both time and position.
It refers to the distribution of mass along the length of the string, which affects how the string responds to tension and displacement.
The pressure gradient represents the change in pressure along the length of the tube, driving the motion of the air particles.
The bulk modulus is a measure of a medium’s resistance to compression, influencing the speed of sound in the medium.
Key assumptions include a linear medium, small displacements, constant tension (for a string), and uniform properties of the medium.
The wave speed is the rate at which waves travel through the medium, determined by the properties of the medium such as tension and mass per unit length.

The one-dimensional wave equation is fundamental in understanding wave phenomena in various fields such as acoustics, electromagnetics, and fluid dynamics. It describes how waves propagate in a medium over time. The derivation starts from Newton’s second law applied to a small segment of a vibrating string. By considering the forces due to tension and the resulting acceleration, we can express the transverse displacement as a function of position and time. This approach leads to the formation of a partial differential equation that characterizes wave motion
One-dimensional waves are characterized by their propagation in a single spatial dimension, with time being the primary independent variable. These waves can be observed in various phenomena, such as sound waves, ocean waves, vibrations from musical instruments, and electromagnetic radiation. In classical physics, waves are studied within the realms of mechanics, acoustics, and optics.
A wave is defined as a disturbance that travels through a medium, transferring energy from one point to another. When the disturbance is singular, it is referred to as a pulse. When the disturbance is repetitive, it is known as a periodic wave. The medium through which the wave travels consists of a series of interconnected particles. These particles exhibit wave-like behavior by interacting with each other, which enables the disturbance to propagate through the medium.
Mechanical Waves : Mechanical waves require a medium to travel through, such as air, water, or solids. Examples include sound waves, ocean waves, and seismic waves. These waves propagate by causing the particles of the medium to oscillate.
Electromagnetic Waves : Electromagnetic waves do not require a medium and can travel through a vacuum. They are produced by the oscillation of electric and magnetic fields. Examples include light waves, radio waves, and X-rays.
Transverse Waves : In transverse waves, the particles of the medium move perpendicular to the direction of wave propagation. Examples include waves on a string and electromagnetic waves.
Longitudinal Waves : Longitudinal waves have particles that oscillate parallel to the direction of wave travel. Sound waves in air and compression waves in a slinky are typical examples.
Surface Waves : Surface waves travel along the interface between two different media, such as air and water. Ocean waves are a common example, combining both transverse and longitudinal motion.
The wave equation is a fundamental mathematical description of how waves propagate through a medium. It is a partial differential equation that relates the spatial and temporal variations of a wave. In one dimension, the wave equation is given by:
∂²y/∂t² = v²∂²y/∂x²
where 𝑦(𝑥,𝑡) represents the displacement of the wave at position x and time t, and 𝑣 is the speed of the wave. This equation applies to both mechanical and electromagnetic waves and is crucial for understanding wave behavior in various physical contexts.
The one-dimensional wave equation describes how waves propagate along a string or similar medium. This derivation uses Newton’s second law and principles of calculus.
Take a small segment of the string with length Δx. Let T be the tension in the string, which is assumed to be constant. The segment experiences forces due to the tension at its ends.
The vertical components of the tension at the ends of the segment can be expressed as:
Tsin(θ1) ≈ T∂y/∂x∣ₓ
𝑇sin(𝜃2) ≈ 𝑇∂𝑦/∂𝑥∣ₓ₊Δₓ
where ( θ1 ) and ( θ2 ) are the angles the string makes with the horizontal at ( x ) and ( x +Δx ), respectively. For small angles, sin(θ) ≈ tan(θ).
The net vertical force ( F ) on the segment is:
F = 𝑇∂𝑦/∂𝑥∣ₓ₊Δₓ ] – T∂y/∂x∣ₓ
Using the definition of the derivative:
[ F = T 𝑇∂𝑦/∂𝑥∣ₓ₊Δₓ ] – ∂y/∂x∣ₓ ≈ T∂²y/∂x²Δₓ
According to Newton’s second law, the net force is equal to the mass of the segment times its acceleration:
F=m∂²𝑦/∂t²
The mass ( m ) of the segment is:
m = ∂Δx
where ( ∂ ) is the mass per unit length. Therefore:
∂²y/∂x² = ∂²y/∂t²
Divide through by (Δₓ )
∂²y/∂x² = μ∂²y/∂t²
Rearrange to get the standard form of the one-dimensional wave equation:
∂²y/∂t² = ∂²y/∂x²
Define the wave speed ( v ) as:
v = √t/μ
Thus, the wave equation becomes:
∂²y/∂t² = v²∂²y/∂x²
This equation describes the propagation of waves along a string or similar medium, where ( v ) is the speed of the wave, ( y(x,t) ) is the displacement, ( x ) is the position, and ( t ) is the time.
Hooke’s Law is a fundamental principle in physics that describes the behavior of springs and other elastic materials. It states that the force required to extend or compress a spring by some distance is proportional to that distance. Mathematically, Hooke’s Law is expressed as:
𝐹=−𝑘𝑥
where:
𝐹 is the force applied to the spring,
𝑘 is the spring constant (a measure of the stiffness of the spring),
𝑥 is the displacement from the spring’s equilibrium position.

Acoustics : The wave equation describes how sound waves propagate through different media, such as air, water, and solids. It helps in designing acoustical systems, musical instruments, and understanding speech and hearing.
Electromagnetic Waves : The wave equation is used to understand the propagation of electromagnetic waves in one-dimensional structures like optical fibers and waveguides. This is essential in telecommunications and fiber-optic technologies.
Mechanical Vibrations : The wave equation models the vibrations of strings in musical instruments like guitars and pianos. It helps in designing and tuning these instruments for desired sound quality.
Seismology : The propagation of seismic waves through the Earth can be approximated using the one-dimensional wave equation. This aids in the study of earthquakes and the structure of the Earth’s interior.
Fluid Dynamics : The wave equation is used to model waves in fluids, such as waves on the surface of water and internal waves within ocean layers. This has applications in oceanography and meteorology.
Engineering : Engineers use the wave equation to analyze the stress and strain in structures subjected to dynamic loads, such as bridges and buildings during earthquakes or wind loads.
To derive the one-dimensional wave equation for a vibrating string, we start by considering a small segment of the string with a given length and constant tension. The vertical components of the tension at the ends of this segment can be approximated by considering how the displacement changes along the string. The net vertical force acting on this segment is the difference between these components. Using Newton’s second law, we equate this net force to the mass of the segment times its acceleration. By simplifying and dividing by the segment length, we derive the wave equation, which describes how waves propagate along the string.
For sound waves in a tube, consider a small segment of air within a long, narrow tube. The net force on this air segment arises from pressure differences at its ends. This force can be expressed using the pressure gradient and the tube’s cross-sectional area. Applying Newton’s second law, we relate this net force to the mass of the air segment and its acceleration. By using the relationship between pressure variations and particle displacement, we substitute into our equation and simplify. This leads to the wave equation for sound waves, which describes how sound propagates through the air in the tube.
The equation can model sound waves, waves on a string, seismic waves, and certain electromagnetic waves in confined structures.
Newton’s second law is used to relate the net force acting on a segment of the medium to its mass and acceleration.
Tension provides the restoring force that acts to return the string to its equilibrium position, crucial for wave propagation.
The vertical components are approximated using the partial derivatives of the displacement with respect to position.
Partial derivatives are used to express how the displacement changes with respect to both time and position.
It refers to the distribution of mass along the length of the string, which affects how the string responds to tension and displacement.
The pressure gradient represents the change in pressure along the length of the tube, driving the motion of the air particles.
The bulk modulus is a measure of a medium’s resistance to compression, influencing the speed of sound in the medium.
Key assumptions include a linear medium, small displacements, constant tension (for a string), and uniform properties of the medium.
The wave speed is the rate at which waves travel through the medium, determined by the properties of the medium such as tension and mass per unit length.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the general form of the one-dimensional wave equation?
∂²u/∂t² = c² ∂²u/∂x²
∂u/∂t = c ∂u/∂x
∂u/∂t² = c ∂u/∂x²
∂²u/∂t = c² ∂²u/∂x
What does the term ∂²u/∂t² represent in the wave equation?
Wave speed
Second derivative of displacement with respect to time
Second derivative of displacement with respect to position
First derivative of displacement with respect to time
In the wave equation ∂²u/∂t² = c² ∂²u/∂x², what does the constant c represent?
Frequency of the wave
Amplitude of the wave
Wave speed
Wavelength
What does the term ∂²u/∂x² signify in the one-dimensional wave equation?
Second derivative of displacement with respect to time
First derivative of displacement with respect to time
Second derivative of displacement with respect to position
First derivative of displacement with respect to position
How can the one-dimensional wave equation be derived from Newton's second law?
By considering the force balance on an infinitesimal element
By integrating the displacement function
By differentiating the wave speed
By equating potential and kinetic energy
Which physical quantity remains constant for a wave described by the one-dimensional wave equation?
Displacement
Velocity
Wave speed
Amplitude
What assumption is made about the medium in deriving the one-dimensional wave equation?
The medium is inhomogeneous
The medium is compressible
The medium is rigid
The medium is homogeneous and elastic
What kind of partial differential equation is the one-dimensional wave equation?
First-order
Second-order
Third-order
Fourth-order
Which boundary conditions are typically associated with the one-dimensional wave equation?
Periodic boundary conditions
Dirichlet boundary conditions
Neumann boundary conditions
All of the above
How does the solution of the one-dimensional wave equation generally depend on initial conditions?
It does not depend on initial conditions
It depends only on initial displacement
It depends only on initial velocity
It depends on both initial displacement and initial velocity
Before you leave, take our quick quiz to enhance your learning!

