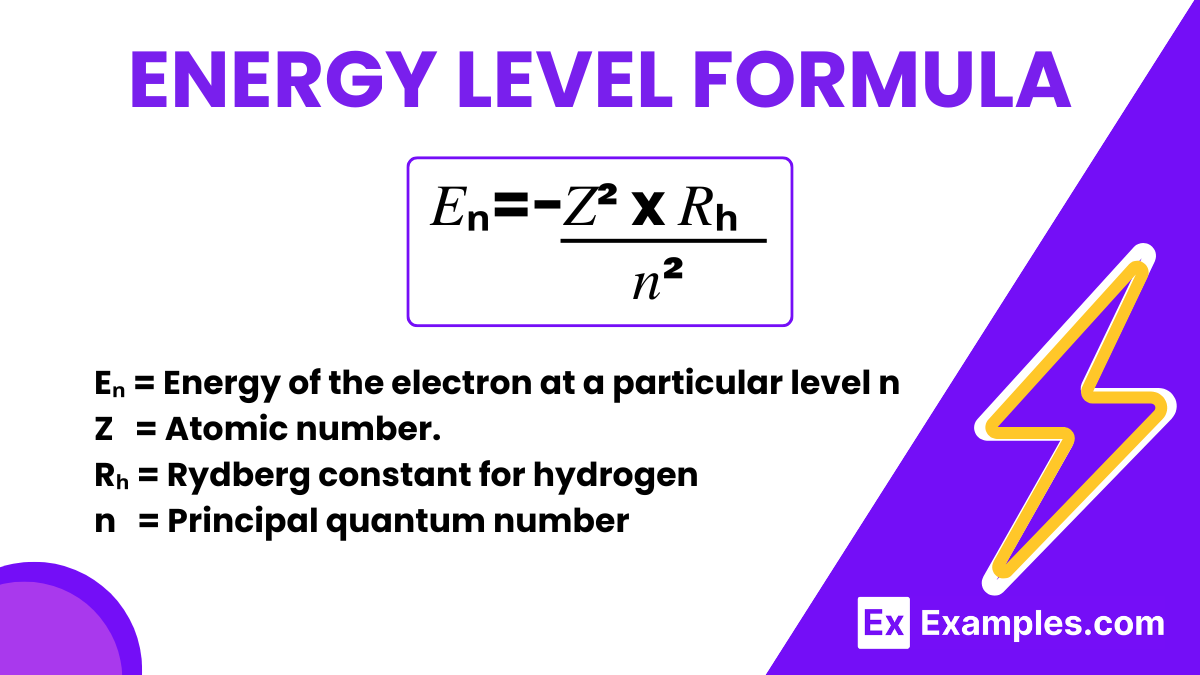
Energy Level Formula

What is Energy Level Formula?

Energy levels in physics refer to the distinct quantities of energy that an electron in an atom can possess. These levels are quantized, meaning electrons can only occupy specific energy states, not values in between. The concept of energy levels is pivotal in understanding atomic structure and electron behavior.

The energy level formula, particularly for hydrogen-like atoms, is given by:

𝐸ₙ=−𝑍² x 𝑅ₕ / 𝑛²

Eₙ represents the energy of the electron at a particular level n.
Z is the atomic number.
Rₕ is the Rydberg constant for hydrogen (approximately 2.18 × 10⁻¹⁸ joules)
n is the principal quantum number, an integer (1, 2, 3, …) that increases with increasing energy levels.

This formula was derived from the Bohr model of the atom, proposed by Niels Bohr in 1913. Bohr introduced the idea that electrons orbit the nucleus in specific stable orbits without radiating energy, and these orbits correspond to certain quantized energy levels. Energy is only absorbed or emitted by an electron when it moves from one energy level to another, not while it remains in a stable orbit.

The minus sign in the formula indicates that the energy levels are bound states (the electron is bound to the nucleus), and the energy level decreases (becomes more negative) as the electron gets closer to the nucleus. As n increases, the electron’s energy becomes less negative, indicating it is less tightly bound and closer to escaping the atom’s influence.

This simple yet profound explanation helps us understand atomic spectra, chemical bonding, and other fundamental aspects of physics and chemistry. Niels Bohr’s contributions have been foundational in both theoretical and applied physics.

Applications of the Energy Level Formula

Atomic Spectra Analysis: This formula helps predict the wavelengths of light that an atom can emit or absorb. Each transition between energy levels corresponds to a specific spectral line, crucial for identifying elements through spectroscopy.
Quantum Chemistry: Chemists use the energy level formula to understand electron configurations, chemical bonding, and reaction mechanisms. It’s essential for predicting how atoms will interact in chemical reactions.
Laser Technology: The principles of electron transitions between energy levels underpin the operation of lasers. By stimulating electrons to move to higher energy levels and then return to lower levels, lasers produce coherent light.
Medical Imaging: Techniques like MRI and PET scans rely on the principles of atomic energy transitions. Understanding these energy levels enables the precise manipulation of atomic particles in medical imaging technologies.
Astrophysics and Cosmology: Astrophysicists use the energy level formula to determine the composition of distant stars and galaxies.
Nanotechnology: In the realm of nanotechnology, understanding electron energy levels is crucial for designing and manipulating materials at the atomic scale. This knowledge helps in the development of more efficient solar cells, LEDs, and quantum dots, which are used in display technologies and biomedical imaging.
Educational Tools: The energy level formula is also an essential part of physics and chemistry curricula in educational institutions. It helps students grasp the fundamentals of quantum mechanics and the electronic structure of atoms, serving as a gateway to more advanced studies in physical sciences.
Environmental Monitoring: This formula assists in environmental science by enabling the analysis of pollutant elements in the atmosphere or soil through spectroscopic techniques. By identifying specific absorption or emission lines, scientists can detect and quantify pollutant levels, helping in the assessment and management of environmental health risks.

Examples Problems on Energy Level Formula

Problem 1: Calculating the Energy of an Electron

Problem: Calculate the energy of an electron in the third energy level (𝑛=3) of a hydrogen atom (𝑍=1).

Solution: Using the formula:

E₃ = – (1² x 2.18 x 10⁻¹⁸ J ) / 3² = – (2.18 x 10⁻¹⁸ J ) / 9 = -0.2422 x 10⁻¹⁸ J

E₃ = -2.422 x 10⁻¹⁹ J

The energy of an electron in the third level of a hydrogen atom is approximately −2.422×10⁻¹⁹ J

Problem 2: Transition Energy Calculation

Problem: Determine the energy released when an electron transitions from the fifth energy level (n=5) to the second energy level (n=2) in a hydrogen atom (Z=1).

Solution: First, calculate the energy at n=5 andn=2:

E₅ = – (1² x 2.18 x 10⁻¹⁸ J ) / 5² = – 2.18 x 10⁻¹⁸ J / 25 = – 0.0872 x 10⁻¹⁸ J

E₂ = – (1² x 2.18 x 10⁻¹⁸ J ) /2² = – 2.18 x 10⁻¹⁸ J / 4 = -0.545 x 10⁻¹⁸ J

The energy released during the transition:

Δ𝐸=E₂ −E₅ = (− 0.545 x 10⁻¹⁸ J ) – (-0.0872 x 10⁻¹⁸ J)

Δ𝐸 = -0.545 x 10⁻¹⁸ J + 0.0872 x 10⁻¹⁸ J

Δ𝐸 = -0.4578 x 10⁻¹⁸ J

The energy released is approximately −0.4578×10−18 J, which signifies energy emission as the electron moves to a lower energy state.

Problem 3: Comparing Energy Levels in Different Atoms

Problem: Calculate and compare the energy of an electron in the first energy level (𝑛=1) of hydrogen (𝑍=1) and helium-ion (𝑍=2).

Solution: For hydrogen (𝑍=1):

𝐸₁ᴴ = − (1² x 2.18×10⁻¹⁸ J ) / 1²=−2.18×10⁻¹⁸ J

For helium-ion (Z=2):

𝐸₁ʰᵉ⁺=− (2²⋅2.18 × 10⁻¹⁸ J )/ 12 =− (4 x 2.18 × 10⁻¹⁸ J) / 1=−8.72×10⁻¹⁸ J

FAQs

How Do You Find the Energy Level?

Use the energy level formula: 𝐸ₙ=−𝑍²⋅𝑅ₕ / 𝑛², where Z is the atomic number and n the principal quantum number.

What is the Famous Formula of Energy?

The famous energy formula is 𝐸=𝑚𝑐², introduced by Einstein, relating energy (E), mass (m), and the speed of light (c).

What is the SI Unit of Energy?

The SI unit of energy is the joule (J), measuring energy, work, or heat in scientific contexts.

Energy Level Formula