Energy Momentum Formula – Formulas, Applications, Example Problems
The Energy-Momentum Formula is a fundamental equation in relativistic physics, establishing the relationship between the energy, momentum, and mass of a particle. This formula is crucial for understanding the behavior of particles at high velocities, where classical mechanics no longer applies.
What is Energy Momentum Formula?
The Energy-Momentum Formula is expressed as:
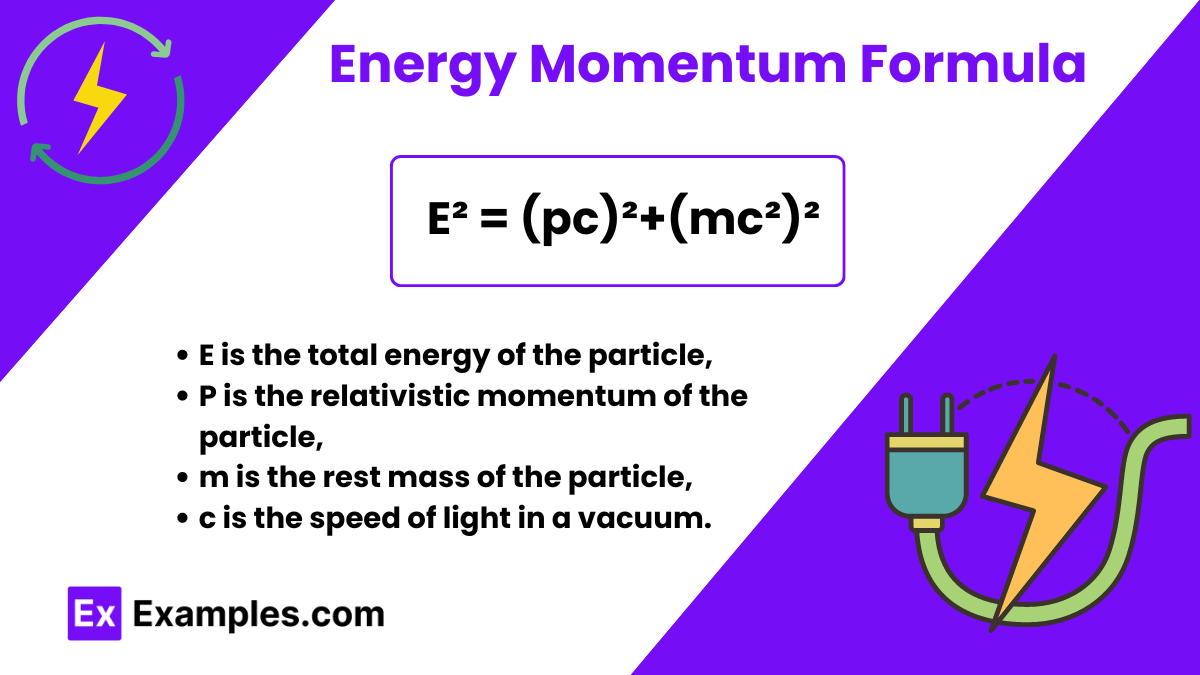
E² = (pc)²+(mc²)²
where:
- E is the total energy of the particle,
- P is the relativistic momentum of the particle,
- m is the rest mass of the particle,
- c is the speed of light in a vacuum.
Applications of Energy Momentum Formula
- High-Energy Particle Physics: Physicists use this formula to calculate the energies and momenta of particles produced in collisions in particle accelerators like the Large Hadron Collider (LHC).
- Astrophysics and Cosmology: The formula helps describe the behavior of particles in extreme environments, like near black holes or in the early universe. It calculates the energy and momentum of cosmic rays, aiding in the understanding of phenomena like gamma-ray bursts and supernovae.
- Nuclear Reactions: The formula is crucial in analyzing nuclear reactions, such as fission and fusion. It determines the energy released in these reactions, vital for energy production and understanding stellar processes.
- Relativistic Mechanics: The formula describes the motion of particles moving at speeds close to the speed of light. This is important in designing particle accelerators and space travel scenarios where relativistic effects are significant.
- Quantum Field Theory: The formula helps describe the properties of quantum fields and particles, aiding in understanding interactions and fundamental forces. This is critical for developing theories like the Standard Model of particle physics.
- Medical Physics: The formula is applied in cancer treatments like proton therapy and radiation therapy, where precise calculations of particle energy and momentum are crucial for targeting cancer cells while minimizing damage to healthy tissue.
- Radiation Protection: The formula calculates the potential impact of radiation on biological tissues, guiding safety standards and protective measures.
- Space Exploration: The formula is vital for space exploration, helping in planning missions involving high-speed travel and understanding the energy requirements for spacecraft propulsion.
- Material Science: The formula helps understand interactions between particles and matter, important for developing new materials and technologies, like semiconductor devices.
- Neutrino Physics: In studying neutrinos, the formula helps understand their behavior and interactions, essential for experiments in neutrino observatories.
Example Problems of Energy Momentum Formula
Problem 1: Photon Energy Calculation
A photon has a momentum of 2×10−20 kg⋅m/s2×10−20kg⋅m/s. Calculate its energy.
Solution:
- 𝐸=𝑝𝑐
- 𝐸=(2×10⁻²⁰ kg⋅m/s)×(3×10⁸ m/s)
- 𝐸=6×10⁻¹² J
Problem 2: A photon has a momentum of 3×10⁻¹⁹ kg⋅m/s Calculate its energy.
Solution: For a photon, the rest mass 𝑚₀ is zero. Therefore, the energy-momentum relation simplifies to:
𝐸=𝑝𝑐
- Given: 𝑝=3×10⁻¹⁹ kg⋅m/s
- 𝑐=3×10⁸ m/s
- Calculating the energy:
- 𝐸=(3×10⁻¹⁹ kg⋅m/s)×(3×10⁸ m/s)
- 𝐸=9×10⁻¹¹ J
- So, the energy of the photon is 9×10⁻¹¹ J.
FAQ’s
Who formulated the energy-momentum relationship?
Albert Einstein formulated the energy-momentum relationship as part of his theory of relativity.
What do the symbols in the energy-momentum formula represent?
E is total energy, p is momentum, 𝑚₀ is rest mass, and c is the speed of light.
What happens to the energy-momentum formula at low velocities?
At low velocities, the formula approximates to classical kinetic energy and momentum.
How does the energy-momentum formula help in understanding cosmic phenomena?
It explains energy and momentum transfer in high-energy cosmic events like supernovae.
What role does the speed of light play in the energy-momentum formula?
The speed of light c links energy and momentum, reflecting relativity’s principles.
Why is the energy-momentum formula important in physics?
It unifies energy and momentum, essential in both special relativity and quantum mechanics.
Can the energy-momentum formula be used for all particles?
Yes, it applies to all particles, regardless of whether they have mass.
How does the energy-momentum formula affect particle collisions?
It conserves energy and momentum in particle collisions, crucial for understanding high-energy physics.
What is the significance of the rest mass in the energy-momentum formula?
The rest mass contributes to a particle’s total energy when at rest.
How does the energy-momentum formula relate to massless particles?
For massless particles, energy is directly proportional to momentum.
Energy Momentum Formula – Formulas, Applications, Example Problems
The Energy-Momentum Formula is a fundamental equation in relativistic physics, establishing the relationship between the energy, momentum, and mass of a particle. This formula is crucial for understanding the behavior of particles at high velocities, where classical mechanics no longer applies.
What is Energy Momentum Formula?
The Energy-Momentum Formula is a fundamental equation in relativistic physics, linking the total energy, momentum, and rest mass of a particle. This relationship is crucial for understanding the behavior of particles moving at velocities close to the speed of light, where classical mechanics no longer applies.
The Energy-Momentum Formula is expressed as:
E² = (pc)²+(mc²)²
where:
E is the total energy of the particle,
P is the relativistic momentum of the particle,
m is the rest mass of the particle,
c is the speed of light in a vacuum.
Applications of Energy Momentum Formula
High-Energy Particle Physics: Physicists use this formula to calculate the energies and momenta of particles produced in collisions in particle accelerators like the Large Hadron Collider (LHC).
Astrophysics and Cosmology: The formula helps describe the behavior of particles in extreme environments, like near black holes or in the early universe. It calculates the energy and momentum of cosmic rays, aiding in the understanding of phenomena like gamma-ray bursts and supernovae.
Nuclear Reactions: The formula is crucial in analyzing nuclear reactions, such as fission and fusion. It determines the energy released in these reactions, vital for energy production and understanding stellar processes.
Relativistic Mechanics: The formula describes the motion of particles moving at speeds close to the speed of light. This is important in designing particle accelerators and space travel scenarios where relativistic effects are significant.
Quantum Field Theory: The formula helps describe the properties of quantum fields and particles, aiding in understanding interactions and fundamental forces. This is critical for developing theories like the Standard Model of particle physics.
Medical Physics: The formula is applied in cancer treatments like proton therapy and radiation therapy, where precise calculations of particle energy and momentum are crucial for targeting cancer cells while minimizing damage to healthy tissue.
Radiation Protection: The formula calculates the potential impact of radiation on biological tissues, guiding safety standards and protective measures.
Space Exploration: The formula is vital for space exploration, helping in planning missions involving high-speed travel and understanding the energy requirements for spacecraft propulsion.
Material Science: The formula helps understand interactions between particles and matter, important for developing new materials and technologies, like semiconductor devices.
Neutrino Physics: In studying neutrinos, the formula helps understand their behavior and interactions, essential for experiments in neutrino observatories.
Example Problems of Energy Momentum Formula
Problem 1: Photon Energy Calculation
A photon has a momentum of 2×10−20 kg⋅m/s2×10−20kg⋅m/s. Calculate its energy.
Solution:
𝐸=𝑝𝑐
𝐸=(2×10⁻²⁰ kg⋅m/s)×(3×10⁸ m/s)
𝐸=6×10⁻¹² J
Problem 2: A photon has a momentum of 3×10⁻¹⁹ kg⋅m/s Calculate its energy.
Solution: For a photon, the rest mass 𝑚₀ is zero. Therefore, the energy-momentum relation simplifies to:
𝐸=𝑝𝑐
Given: 𝑝=3×10⁻¹⁹ kg⋅m/s
𝑐=3×10⁸ m/s
Calculating the energy:
𝐸=(3×10⁻¹⁹ kg⋅m/s)×(3×10⁸ m/s)
𝐸=9×10⁻¹¹ J
So, the energy of the photon is 9×10⁻¹¹ J.
FAQ’s
Who formulated the energy-momentum relationship?
Albert Einstein formulated the energy-momentum relationship as part of his theory of relativity.
What do the symbols in the energy-momentum formula represent?
E is total energy, p is momentum, 𝑚₀ is rest mass, and c is the speed of light.
What happens to the energy-momentum formula at low velocities?
At low velocities, the formula approximates to classical kinetic energy and momentum.
How does the energy-momentum formula help in understanding cosmic phenomena?
It explains energy and momentum transfer in high-energy cosmic events like supernovae.
What role does the speed of light play in the energy-momentum formula?
The speed of light c links energy and momentum, reflecting relativity’s principles.
Why is the energy-momentum formula important in physics?
It unifies energy and momentum, essential in both special relativity and quantum mechanics.
Can the energy-momentum formula be used for all particles?
Yes, it applies to all particles, regardless of whether they have mass.
How does the energy-momentum formula affect particle collisions?
It conserves energy and momentum in particle collisions, crucial for understanding high-energy physics.
What is the significance of the rest mass in the energy-momentum formula?
The rest mass contributes to a particle’s total energy when at rest.
How does the energy-momentum formula relate to massless particles?
For massless particles, energy is directly proportional to momentum.


