What is the formula for Helmholtz free energy (A)?
A = U - TS
A = H - TS
A = U + TS
A = G - TS

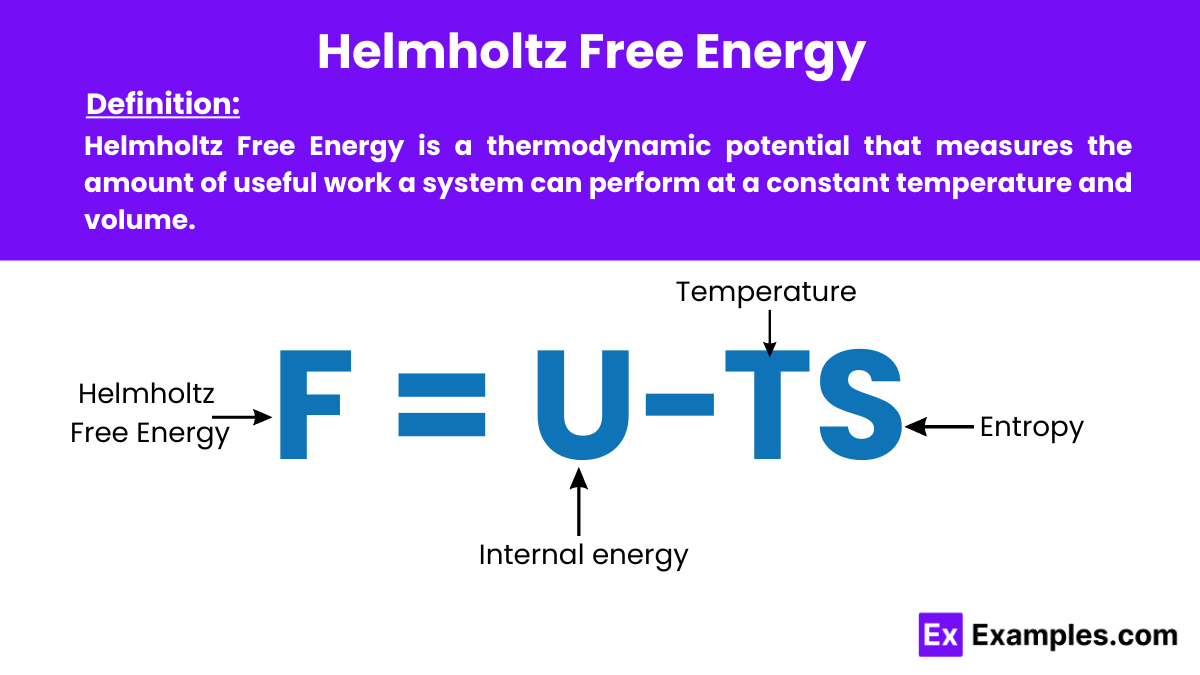
Helmholtz Free Energy is a thermodynamics potential that measures the amount of useful work a system can perform at a constant temperature and volume. It is particularly important in physics for predicting the equilibrium state of systems under these conditions.
The formula for the Helmholtz Free Energy is:
where:
This formula captures the balance between the system’s internal energy and the energy unavailable due to entropy at a given temperature. Providing insight into the usable energy available for work at constant temperature and volume.
To derive the Helmholtz equation effectively using the first law of thermodynamics and apply it to a closed system:
First Law of Thermodynamics: For a closed system, the first law of thermodynamics states:
𝛿𝑄=𝛿𝑊+𝑑𝑈
Heat and Work Definitions:
The heat transferred (𝛿𝑄) is given by: 𝛿𝑄=𝑇𝑑𝑆 where 𝑇 is the absolute temperature, and 𝑑𝑆 is the change in entropy.
The work done (𝛿𝑊) is given by: 𝛿𝑊=𝑃𝑑𝑉 where 𝑃 is the pressure, and 𝑑𝑉 is the change in volume.
Substituting Into the First Law: Substitute these expressions into the first law equation:
𝑇𝑑𝑆=𝑃𝑑𝑉+𝑑𝑈
Rearrange to Isolate 𝑑𝑈: 𝑑𝑈=𝑇𝑑𝑆−𝑃𝑑𝑉
Define the Helmholtz Free Energy (𝐹): The Helmholtz Free Energy (𝐹) is defined as: 𝐹=𝑈−𝑇𝑆
Taking the differential: 𝑑𝐹=𝑑𝑈−𝑇𝑑𝑆−𝑆𝑑𝑇
Substituting 𝑑𝑈 into the 𝑑𝐹 Expression: Substitute the expression for 𝑑𝑈 into the equation for
𝑑𝐹: 𝑑𝐹=𝑇𝑑𝑆−𝑃𝑑𝑉−𝑇𝑑𝑆−𝑆𝑑𝑇
Simplify this to: 𝑑𝐹=−𝑆𝑑𝑇−𝑃𝑑𝑉
This results in the desired formula: 𝑑𝐹=−𝑆𝑑𝑇−𝑃𝑑𝑉
This expression shows that the differential change in the Helmholtz Free Energy (𝑑𝐹) depends on changes in temperature and volume.
| Aspect | Helmholtz Free Energy (F) | Gibbs Free Energy (G) |
|---|---|---|
| Definition | A thermodynamic potential that measures the useful work obtainable from a system at constant temperature and volume. | A thermodynamic potential that measures the useful work obtainable from a system at constant temperature and pressure. |
| Formula | 𝐹=𝑈−𝑇𝑆 | 𝐺=𝐻−𝑇𝑆 |
| Variables Held Constant | Temperature (T) and Volume (V) | Temperature (T) and Pressure (P) |
| Applicability | Useful for systems involving fixed volumes, such as certain closed systems or chemical reactions in sealed containers. | Useful for processes involving constant pressure, like many chemical reactions occurring in open containers. |
| Significance in Work | Indicates the maximum work obtainable under constant temperature and volume. | Indicates the maximum non-expansion work obtainable under constant temperature and pressure. |
| Relation to Equilibrium | System reaches equilibrium when 𝐹 is minimized. | System reaches equilibrium when 𝐺 is minimized. |
Both are valuable tools in thermodynamics, providing insight into the energy changes and equilibrium conditions of different systems.

The Helmholtz Free Energy (𝐹) has several practical uses in physics and thermodynamics:
Here are some examples where you can apply Helmholtz Free Energy:
Helmholtz Free Energy measures a system’s capacity for useful work at constant temperature and volume.
Helmholtz Free Energy pertains to constant temperature and volume, while Gibbs Free Energy is at constant temperature and pressure.
It predicts process spontaneity under constant temperature and volume conditions.
Combines system’s internal energy and temperature-scaled entropy.
Indicates spontaneous work capacity at constant temperature and volume.
Yes, predicts stable phases based on minimum Free Energy.
Changes in Helmholtz Free Energy (ΔF) are computed using Δ𝐹=Δ𝑈−𝑇Δ𝑆.
Understands chemical reactions, phase transitions, and molecular behavior under constant temperature and volume.
At equilibrium, Helmholtz Free Energy is minimized, driving reactions towards lower Free Energy states.
Lower Helmholtz Free Energy indicates greater stability under constant temperature and volume conditions.

Helmholtz Free Energy is a thermodynamics potential that measures the amount of useful work a system can perform at a constant temperature and volume. It is particularly important in physics for predicting the equilibrium state of systems under these conditions.
The Helmholtz Free Energy is a thermodynamic function that reflects the amount of energy in a system available to do useful work when the system is kept at a constant temperature and volume. It is useful for determining how much energy can be harnessed from a system or to predict its behavior under specific conditions.
The Helmholtz Function, also known as the Helmholtz Free Energy, is a thermodynamic potential that measures the useful work obtainable from a closed system at constant temperature and volume. It gives insight into the energy balance of the system and is particularly useful in predicting equilibrium states.
The formula for the Helmholtz Free Energy is:
F=U−TS
where:
F: Helmholtz Free Energy
U: Internal energy of the system
T: Absolute temperature (in Kelvin)
S: Entropy of the system
This formula captures the balance between the system’s internal energy and the energy unavailable due to entropy at a given temperature. Providing insight into the usable energy available for work at constant temperature and volume.
To derive the Helmholtz equation effectively using the first law of thermodynamics and apply it to a closed system:
First Law of Thermodynamics: For a closed system, the first law of thermodynamics states:
𝛿𝑄=𝛿𝑊+𝑑𝑈
Heat and Work Definitions:
The heat transferred (𝛿𝑄) is given by: 𝛿𝑄=𝑇𝑑𝑆 where 𝑇 is the absolute temperature, and 𝑑𝑆 is the change in entropy.
The work done (𝛿𝑊) is given by: 𝛿𝑊=𝑃𝑑𝑉 where 𝑃 is the pressure, and 𝑑𝑉 is the change in volume.
Substituting Into the First Law: Substitute these expressions into the first law equation:
𝑇𝑑𝑆=𝑃𝑑𝑉+𝑑𝑈
Rearrange to Isolate 𝑑𝑈: 𝑑𝑈=𝑇𝑑𝑆−𝑃𝑑𝑉
Define the Helmholtz Free Energy (𝐹): The Helmholtz Free Energy (𝐹) is defined as: 𝐹=𝑈−𝑇𝑆
Taking the differential: 𝑑𝐹=𝑑𝑈−𝑇𝑑𝑆−𝑆𝑑𝑇
Substituting 𝑑𝑈 into the 𝑑𝐹 Expression: Substitute the expression for 𝑑𝑈 into the equation for
𝑑𝐹: 𝑑𝐹=𝑇𝑑𝑆−𝑃𝑑𝑉−𝑇𝑑𝑆−𝑆𝑑𝑇
Simplify this to: 𝑑𝐹=−𝑆𝑑𝑇−𝑃𝑑𝑉
This results in the desired formula: 𝑑𝐹=−𝑆𝑑𝑇−𝑃𝑑𝑉
This expression shows that the differential change in the Helmholtz Free Energy (𝑑𝐹) depends on changes in temperature and volume.
Aspect | Helmholtz Free Energy (F) | Gibbs Free Energy (G) |
|---|---|---|
Definition | A thermodynamic potential that measures the useful work obtainable from a system at constant temperature and volume. | A thermodynamic potential that measures the useful work obtainable from a system at constant temperature and pressure. |
Formula | 𝐹=𝑈−𝑇𝑆 | 𝐺=𝐻−𝑇𝑆 |
Variables Held Constant | Temperature (T) and Volume (V) | Temperature (T) and Pressure (P) |
Applicability | Useful for systems involving fixed volumes, such as certain closed systems or chemical reactions in sealed containers. | Useful for processes involving constant pressure, like many chemical reactions occurring in open containers. |
Significance in Work | Indicates the maximum work obtainable under constant temperature and volume. | Indicates the maximum non-expansion work obtainable under constant temperature and pressure. |
Relation to Equilibrium | System reaches equilibrium when 𝐹 is minimized. | System reaches equilibrium when 𝐺 is minimized. |
Both are valuable tools in thermodynamics, providing insight into the energy changes and equilibrium conditions of different systems.
The Helmholtz Free Energy (𝐹) has several practical uses in physics and thermodynamics:
Predicting Equilibrium States: You can use Helmholtz Free Energy to determine the equilibrium state of a system at constant temperature and volume. A system reaches equilibrium when its Helmholtz Free Energy minimizes.
Calculating Work Capacity: It allows you to calculate the maximum amount of useful work a system. It can perform under constant temperature and volume conditions.
Analyzing Phase Transitions: Helmholtz Free Energy helps you analyze phase transitions. And determine the conditions under which a material changes its phase.
Understanding Stability: By examining changes in the Helmholtz Free Energy, you can assess the stability of different states and predict whether a state will remain stable or transition to another state.
Formulating Statistical Mechanics: In statistical mechanics, you can relate the Helmholtz Free Energy to the partition function, which provides insights into the distribution of particles and energy within the system.
Modeling Chemical Reactions: You can use it to model the energy changes during chemical reactions that occur in a controlled volume. Predicting the direction and extent of the reaction.
Here are some examples where you can apply Helmholtz Free Energy:
Determining Chemical Reaction Equilibrium: You can use Helmholtz Free Energy to determine the equilibrium position of chemical reactions in closed containers. For instance, in a reaction involving gas-phase reactants and products, you can calculate changes in free energy to identify the point where the system reaches equilibrium at constant volume and temperature.
Analyzing Phase Changes: You can apply Helmholtz Free Energy to analyze phase changes, like the transition of water from liquid to ice. By comparing the free energy of the liquid and solid phases at different temperatures, you can predict the temperature at which ice will form in a closed environment.
Evaluating Stability in Magnetic Systems: When studying magnetic systems, you can rely on Helmholtz Free Energy to understand the stability of different magnetic phases. For instance, you can calculate the energy differences between various magnetic states and predict which one will be the most stable.
Modeling Molecular Systems: In molecular simulations, you can use Helmholtz Free Energy to model molecular interactions, especially in closed systems. This method helps you estimate the relative stability of different molecular conformations by considering their energy and entropy changes.
Designing Thermodynamic Cycles: You can also apply Helmholtz Free Energy to design thermodynamic cycles in refrigeration or heat pump systems. By evaluating the energy balance between the cooling or heating cycles, you can maximize the system’s efficiency.
Helmholtz Free Energy measures a system’s capacity for useful work at constant temperature and volume.
Helmholtz Free Energy pertains to constant temperature and volume, while Gibbs Free Energy is at constant temperature and pressure.
It predicts process spontaneity under constant temperature and volume conditions.
Combines system’s internal energy and temperature-scaled entropy.
Indicates spontaneous work capacity at constant temperature and volume.
Yes, predicts stable phases based on minimum Free Energy.
Changes in Helmholtz Free Energy (ΔF) are computed using Δ𝐹=Δ𝑈−𝑇Δ𝑆.
Understands chemical reactions, phase transitions, and molecular behavior under constant temperature and volume.
At equilibrium, Helmholtz Free Energy is minimized, driving reactions towards lower Free Energy states.
Lower Helmholtz Free Energy indicates greater stability under constant temperature and volume conditions.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the formula for Helmholtz free energy (A)?
A = U - TS
A = H - TS
A = U + TS
A = G - TS
In the formula A = U - TS, what does U represent?
Internal energy
Enthalpy
Gibbs free energy
Entropy
In the Helmholtz free energy formula, what does T stand for?
Time
Temperature
Total energy
Thermal conductivity
How does Helmholtz free energy relate to work done by a system?
It represents the maximum work done by a system at constant temperature and volume
It represents the minimum work done by a system at constant temperature and volume
It represents the total work done by a system
It represents the internal energy of a system
What does the S in the Helmholtz free energy formula represent?
Entropy
Enthalpy
Energy
Specific heat
Which thermodynamic potential is used to determine the equilibrium of a system at constant temperature and volume?
Gibbs free energy
Helmholtz free energy
Internal energy
Enthalpy
For a reversible process at constant temperature and volume, what is the change in Helmholtz free energy (ΔA)?
Equal to zero
Less than zero
Greater than zero
Undefined
What is the unit of Helmholtz free energy in the International System of Units (SI)?
Joules
Kelvin
Watts
Newtons
In a thermodynamic system, when is the Helmholtz free energy at its minimum?
At equilibrium
During a spontaneous process
When temperature is maximum
When volume is maximum
How is Helmholtz free energy different from Gibbs free energy?
Helmholtz is used for constant volume, while Gibbs is used for constant pressure
Helmholtz is used for constant pressure, while Gibbs is used for constant volume
Helmholtz measures total energy, Gibbs measures internal energy
They are identical in all aspects
Before you leave, take our quick quiz to enhance your learning!

