What is the formula for the mass-energy equivalence?
E = mc²
E = mv²/2
E = hf
E = kT
of 10
Mass Energy Formula – Formula, Derivation, Applications, Example Problems
Mass Energy Formula – Formula, Derivation, Applications, Example Problems
What is Mass Energy Formula?
The Mass Energy Formula, represented as
is a fundamental equation in physics that was discovered by Albert Einstein in 1905. This formula expresses the idea that mass and energy are interchangeable; it states that the energy of a system is equal to the mass of the object multiplied by the square of the speed of light . Here,
- E = Energy.
- m = Mass.
- c = Speed of light in a vacuum, which is approximately 299,792,458 meters per second.
The equation is a key principle of Einstein’s theory of special relativity, showing that even a small amount of mass can be converted into a large amount of energy.
This formula has transformed our understanding of energy and mass, revealing that they are not distinct elements but rather different forms of the same thing. It underscores many modern technologies and theoretical applications, from nuclear power generation to the basic principles of particle physics. The Mass Energy Formula is pivotal because it provides a method to calculate the enormous energy potential of a tiny amount of mass.
Derivation of Mass Energy Formula
The derivation of the Mass Energy Formula, 𝐸=𝑚𝑐², begins by considering the principles of special relativity, introduced by Albert Einstein. First, let’s understand the relationship between energy, mass, and the speed of light within the framework of physics.
Starting with the concept of energy and momentum: In special relativity, the total energy (E) of a particle is related to its momentum (p) and mass (m) through the equation:
Here, 𝑐 represents the speed of light in vacuum.
Considering a particle at rest: When a particle is at rest, its momentum p is zero.
Substituting 𝑝=0 into the equation simplifies it to:
Taking the square root of both sides, we obtain:
This reveals that the energy of a particle at rest is solely dependent on its mass and the square of the speed of light.
This derivation shows that mass itself is a form of energy. Einstein’s formula 𝐸=𝑚𝑐2E=mc2 indicates that mass can be converted into energy and vice versa, highlighting the interchangeable nature of mass and energy.
Applications of Mass Energy Formula
- Nuclear Power Generation: This formula helps explain how nuclear reactors produce energy. In reactors, the nuclei of atoms split (nuclear fission) or combine (nuclear fusion), converting a small amount of mass into a large amount of energy.
- Nuclear Weapons: The destructive power of nuclear weapons comes from the conversion of mass into energy, following Einstein’s equation. A tiny amount of mass can release a tremendous amount of energy.
- Medical Applications: In treatments like cancer radiotherapy, machines use the principles of 𝐸=𝑚𝑐² to generate high-energy particles that can target and destroy tumor cells.
- Astrophysics: The formula helps astrophysicists understand processes like stellar evolution and black hole formation, where massive energy outputs result from mass conversion.
- Cosmology: The Big Bang theory utilizes 𝐸=𝑚𝑐² to explain how the early universe’s energy transformed into matter.
Example Problems on Mass Energy Formula
Problem 1: Calculating Energy from Mass
Question: Calculate the energy produced when 1 gram of matter is completely converted into energy.
Solution:
Mass, 𝑚=1 gram = 0.001 kg (since 1 gram = 0.001 kg)
Speed of light, 𝑐=299,792,458 m/s
Using the formula 𝐸=𝑚𝑐² :
𝐸=0.001 × (299,792,458)²
𝐸=0.001 × 8.9875517873681764 × 10¹⁶ Joules
𝐸 ≈ 89.875 Terajoules
Therefore, 1 gram of matter converted into energy yields about 89.875 Terajoules.
Problem 2: Comparing Mass Loss in Nuclear Reaction
Question: If 0.002 kg of mass is lost in a nuclear reaction, how much energy is released?
Solution:
Mass loss, Δ𝑚=0.002 kg
Using 𝐸=𝑚𝑐²:
𝐸= 0.002 × (299,792,458)²
𝐸 = 0.002 × 8.9875517873681764 × 10¹⁶ Joules
𝐸 ≈ 179.75 Terajoules
The energy released from losing 0.002 kg of mass in a nuclear reaction is approximately 179.75 Terajoules.
Problem 3: Energy Content in a Sugar Cube
Question: Estimate the energy content in a sugar cube weighing 3 grams if all its mass could be converted to energy. Solution:
Mass of the sugar cube, 𝑚=3 grams = 0.003 kg
Using 𝐸=𝑚𝑐²:
𝐸= 0.003 × (299,792,458)²
𝐸 = 0.003 × 8.9875517873681764 ×10¹⁶ Joules
𝐸 ≈ 269.63 Terajoules
The theoretical energy content of a 3-gram sugar cube, if completely converted into energy, would be about 269.63 Terajoules.
FAQs
How Do You Convert Mass to Energy?
Convert mass to energy by applying the Mass Energy Formula, 𝐸=𝑚𝑐². Multiply the mass by the square of the speed of light to find energy.
What is the Einstein’s Mass Energy Relation?
The Einstein mass energy relation, 𝐸=𝑚𝑐², states that energy equals mass multiplied by the speed of light squared, linking mass and energy.
Do Photons Violate 𝐸=𝑚𝑐²?
Photons do not violate 𝐸=𝑚𝑐²; they are massless but carry energy and momentum, consistent with Einstein’s theory of relativity.
Share :
Mass Energy Formula – Formula, Derivation, Applications, Example Problems
What is Mass Energy Formula?
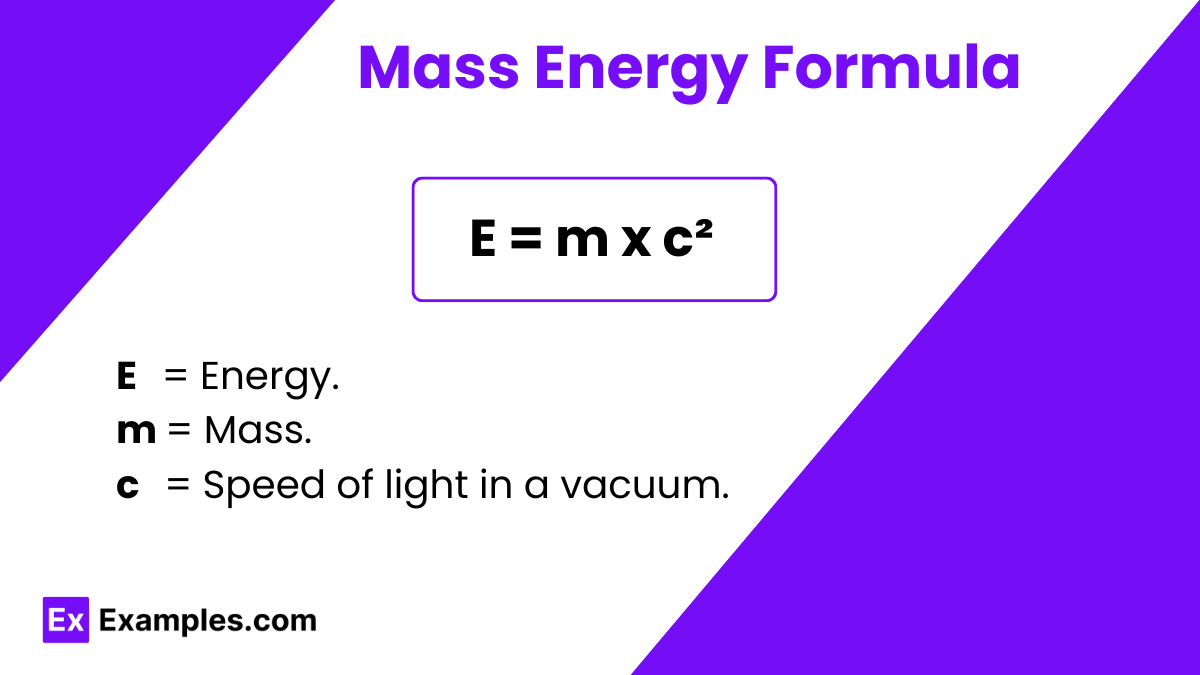
The Mass Energy Formula, represented as
E = m x c²
is a fundamental equation in physics that was discovered by Albert Einstein in 1905. This formula expresses the idea that mass and energy are interchangeable; it states that the energy of a system is equal to the mass of the object multiplied by the square of the speed of light . Here,
E = Energy.
m = Mass.
c = Speed of light in a vacuum, which is approximately 299,792,458 meters per second.
The equation is a key principle of Einstein’s theory of special relativity, showing that even a small amount of mass can be converted into a large amount of energy.
This formula has transformed our understanding of energy and mass, revealing that they are not distinct elements but rather different forms of the same thing. It underscores many modern technologies and theoretical applications, from nuclear power generation to the basic principles of particle physics. The Mass Energy Formula is pivotal because it provides a method to calculate the enormous energy potential of a tiny amount of mass.
Derivation of Mass Energy Formula
The derivation of the Mass Energy Formula, 𝐸=𝑚𝑐², begins by considering the principles of special relativity, introduced by Albert Einstein. First, let’s understand the relationship between energy, mass, and the speed of light within the framework of physics.
Starting with the concept of energy and momentum: In special relativity, the total energy (E) of a particle is related to its momentum (p) and mass (m) through the equation:
𝐸²=𝑝²𝑐²+𝑚²𝑐⁴
Here, 𝑐 represents the speed of light in vacuum.
Considering a particle at rest: When a particle is at rest, its momentum p is zero.
Substituting 𝑝=0 into the equation simplifies it to:
𝐸²=𝑚²𝑐⁴
Taking the square root of both sides, we obtain:
𝐸=𝑚𝑐²
This reveals that the energy of a particle at rest is solely dependent on its mass and the square of the speed of light.
This derivation shows that mass itself is a form of energy. Einstein’s formula 𝐸=𝑚𝑐2E=mc2 indicates that mass can be converted into energy and vice versa, highlighting the interchangeable nature of mass and energy.
Applications of Mass Energy Formula
Nuclear Power Generation: This formula helps explain how nuclear reactors produce energy. In reactors, the nuclei of atoms split (nuclear fission) or combine (nuclear fusion), converting a small amount of mass into a large amount of energy.
Nuclear Weapons: The destructive power of nuclear weapons comes from the conversion of mass into energy, following Einstein’s equation. A tiny amount of mass can release a tremendous amount of energy.
Medical Applications: In treatments like cancer radiotherapy, machines use the principles of 𝐸=𝑚𝑐² to generate high-energy particles that can target and destroy tumor cells.
Astrophysics: The formula helps astrophysicists understand processes like stellar evolution and black hole formation, where massive energy outputs result from mass conversion.
Cosmology: The Big Bang theory utilizes 𝐸=𝑚𝑐² to explain how the early universe’s energy transformed into matter.
Example Problems on Mass Energy Formula
Problem 1: Calculating Energy from Mass
Question: Calculate the energy produced when 1 gram of matter is completely converted into energy.
Solution:
Mass, 𝑚=1 gram = 0.001 kg (since 1 gram = 0.001 kg)
Speed of light, 𝑐=299,792,458 m/s
Using the formula 𝐸=𝑚𝑐² :
𝐸=0.001 × (299,792,458)²
𝐸=0.001 × 8.9875517873681764 × 10¹⁶ Joules
𝐸 ≈ 89.875 Terajoules
Therefore, 1 gram of matter converted into energy yields about 89.875 Terajoules.
Problem 2: Comparing Mass Loss in Nuclear Reaction
Question: If 0.002 kg of mass is lost in a nuclear reaction, how much energy is released?
Solution:
Mass loss, Δ𝑚=0.002 kg
Using 𝐸=𝑚𝑐²:
𝐸= 0.002 × (299,792,458)²
𝐸 = 0.002 × 8.9875517873681764 × 10¹⁶ Joules
𝐸 ≈ 179.75 Terajoules
The energy released from losing 0.002 kg of mass in a nuclear reaction is approximately 179.75 Terajoules.
Problem 3: Energy Content in a Sugar Cube
Question: Estimate the energy content in a sugar cube weighing 3 grams if all its mass could be converted to energy. Solution:
Mass of the sugar cube, 𝑚=3 grams = 0.003 kg
Using 𝐸=𝑚𝑐²:
𝐸= 0.003 × (299,792,458)²
𝐸 = 0.003 × 8.9875517873681764 ×10¹⁶ Joules
𝐸 ≈ 269.63 Terajoules
The theoretical energy content of a 3-gram sugar cube, if completely converted into energy, would be about 269.63 Terajoules.
FAQs
How Do You Convert Mass to Energy?
Convert mass to energy by applying the Mass Energy Formula, 𝐸=𝑚𝑐². Multiply the mass by the square of the speed of light to find energy.
What is the Einstein’s Mass Energy Relation?
The Einstein mass energy relation, 𝐸=𝑚𝑐², states that energy equals mass multiplied by the speed of light squared, linking mass and energy.
Do Photons Violate 𝐸=𝑚𝑐²?
Photons do not violate 𝐸=𝑚𝑐²; they are massless but carry energy and momentum, consistent with Einstein’s theory of relativity.
Practice Test
Who proposed the mass-energy equivalence formula?
Isaac Newton
Albert Einstein
Niels Bohr
James Clerk Maxwell
of 10
In the equation E = mc², what does 'c' represent?
Charge of an electron
Speed of light
Coulomb constant
Gravitational constant
of 10
What does the mass-energy equivalence principle imply about mass and energy?
They are separate entities
They are interchangeable
Mass can be converted into volume
Energy can be converted into charge
of 10
If 1 kg of mass is converted entirely into energy, what is the amount of energy produced? (c = 3 x 10⁸ m/s)
9 x 10¹⁶ J
3 x 10⁸ J
1 x 10⁴ J
9 x 10¹² J
of 10
How does the mass-energy equivalence relate to nuclear reactions?
It explains the energy produced in chemical reactions
It explains the energy released in nuclear reactions
It has no relation to nuclear reactions
It only applies to gravitational energy
of 10
What is the unit of energy in the mass-energy equivalence equation?
Joules
Kilograms
Meters per second
Newtons
of 10
If an object has a mass of 2 kg, what is its equivalent energy? (c = 3 x 10⁸ m/s)
1.8 x 10¹⁷ J
6 x 10⁸ J
1 x 10⁶ J
4.5 x 10¹⁶
of 10
Which concept does the mass-energy equivalence support in physics?
Conservation of mass only
Conservation of energy only
Conservation of both mass and energy
Conservation of momentum
of 10
In the context of mass-energy equivalence, what happens to the mass of an object as it approaches the speed of light?
It decreases
It remains constant
It becomes zero
It increases
of 10