What is the formula for linear speed in terms of distance and time?
v = d/t
v = t/d
v = d*t
v = 2d/t
of 10
Linear Speed Formula – Formula, Applications, Example Problems
What is Linear Speed Formula?
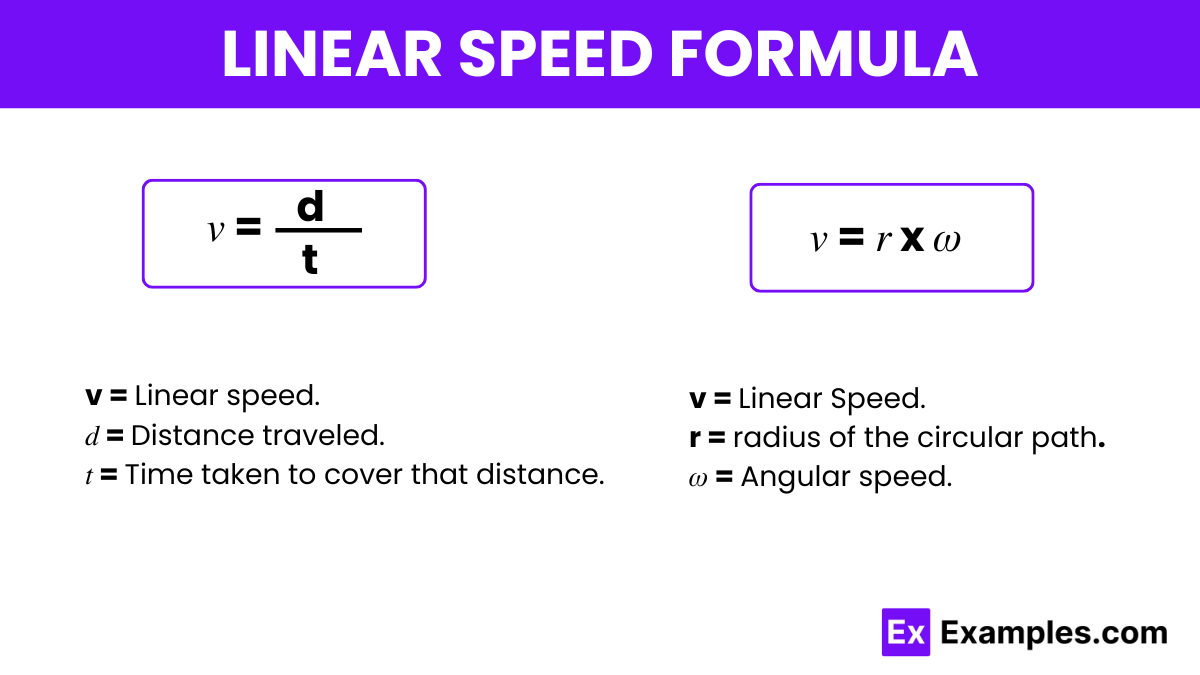
Linear speed, often simply referred to as speed, is a measure of the distance traveled per unit of time by an object moving along a straight or curved path. In physics, the linear speed formula is crucial for understanding how objects move in space. The formula for linear speed (𝑣) is expressed as
- v represents linear speed.
- 𝑑 represents the distance traveled.
- 𝑡 denotes the time taken to cover that distance.
The relationship between linear speed and angular speed also forms an integral part of kinematics in physics. Linear Speed Formula, in the sense of angular speed, is articulated as
- v represents linear speed.
- 𝑟 is the radius of the circular path.
- 𝜔 (omega) is the angular speed.
This formula highlights how the linear speed increases with both the radius of the path and the rate at which an object moves around the path. This specific articulation was developed from the broader understanding of motion and kinematics, dating back to the foundational works of Sir Isaac Newton and other key figures in classical mechanics.
Applications of Linear Speed Formula
- Automotive Engineering: Engineers use the linear speed formula to design speedometers that accurately measure the speed of vehicles.
- Sports Science: Coaches calculate the speed of athletes, such as runners or cyclists, to enhance training programs and improve performance.
- Amusement Park Rides: Ride designers apply the formula to ensure the safety and thrill of rides, particularly for those involving circular motions like roller coasters.
- Astronomy: Astronomers determine the speed of planets and satellites in their orbits, helping predict their positions and movements accurately.
- Industrial Machinery: In factories, the formula helps in configuring the speeds of conveyor belts to optimize production efficiency.
- Consumer Electronics: In devices like CD and DVD players, understanding linear speed is crucial for the accurate reading and writing of data.
Example Problems on Linear Speed Formula
Problem 1: Calculating Speed of a Car
Question: A car travels a distance of 150 kilometers in 3 hours. What is the average speed of the car?
Solution: To find the average speed, use the formula: 𝑣=𝑑 / 𝑡 Where:
- 𝑑=150 (distance traveled)
- 𝑡=3 hours (time taken)
Substitute the values into the formula: 𝑣=150 km / 3 hours=50 km/hour
Answer: The average speed of the car is 50 km/hour.
Problem 2: Speed of a Bicycle on a Circular Track
Question: A bicycle rides along a circular track with a radius of 30 meters. If the rider completes one full circle in 2 minutes, what is the linear speed of the bicycle?
Solution: First, convert the time from minutes to seconds: 𝑡 = 2 minutes = 120 seconds
Calculate the circumference of the circle, which is the distance traveled:
𝑑 = 2𝜋 x 𝑟=2𝜋 x (30) = 60𝜋 meters
Now, use the linear speed formula:
𝑣 = 𝑑 / 𝑡 = 60𝜋 meters / 120 seconds=𝜋 / 2 meters/second
Answer: The linear speed of the bicycle is 𝜋 / 2 meters/second, approximately 1.57 meters/second.
Problem 3: Angular Speed to Linear Speed Conversion
Question: A wheel rotates at an angular speed of 100 radians per minute. If the radius of the wheel is 0.5 meters, what is the linear speed at the rim of the wheel?
Solution: First, convert the angular speed from radians per minute to radians per second: 𝜔=100 radians/minute=100 / 60 radians/second
Now, use the formula connecting linear speed and angular speed:
𝑣=𝑟𝜔=0.5 × (100 / 60) = 50 / 60 meters/second
Simplify: 𝑣 = 25 / 30 meters/second ≈ 0.83 meters/second
Answer: The linear speed at the rim of the wheel is approximately 0.83 meters/second.
FAQs
How Do You Find Linear Speed?
Calculate linear speed with the formula 𝑣=𝑑t, where 𝑑 is distance and 𝑡 is time.
What Is the Formula for Linear Speed in Trig?
In trigonometric terms, we express linear speed 𝑣v as 𝑣=𝑟𝜔, linking the radius r with the angular speed ω.
Is Linear Speed the Same as Speed?
Yes, linear speed refers to the magnitude of velocity, indicating how fast an object travels along a path.
Share :
Practice Test
If a car travels 100 kilometers in 2 hours, what is its linear speed?
25 km/h
50 km/h
75 km/h
100 km/h
of 10
What is the unit of linear speed in the International System of Units (SI)?
meters per second (m/s)
kilometers per hour (km/h)
miles per hour (mph)
feet per second (ft/s)
of 10
How does increasing the distance affect the linear speed, assuming the time remains constant?
Linear speed decreases
Linear speed remains the same
Linear speed increases
Linear speed becomes zero
of 10
What is the linear speed of an object that covers 60 meters in 3 seconds?
15 m/s
10 m/s
20 m/s
5 m/s
of 10
If the time taken to cover a certain distance is halved, how does the linear speed change?
It is halved
It remains the same
It doubles
It quadruples
of 10
Which of the following correctly defines linear speed?
The rate at which an object changes its position
The total distance traveled by an object
The time taken by an object to travel a certain distance
The acceleration of an object
of 10
How does the linear speed change if both the distance and time are doubled?
It remains the same
It doubles
It halves
It quadruples
of 10
What is the formula for linear speed in terms of angular speed (ω) and radius (r)?
v = ωr
v = ω/r
v = r/ω
v = ωr²
of 10
If an object has an angular speed of 2 rad/s and a radius of 3 meters, what is its linear speed?
6 m/s
3 m/s
2 m/s
5 m/s
of 10