Resonant Frequency Formula

What is Resonant Frequency Formula?

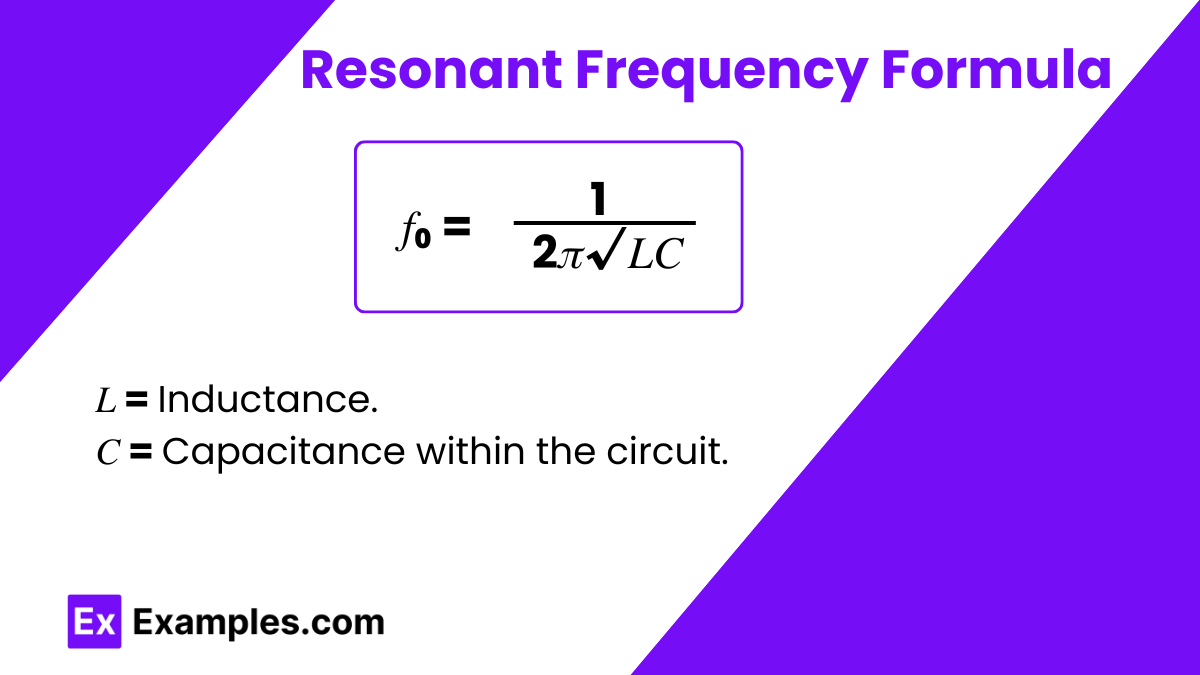
The resonant frequency formula is a fundamental equation in the field of physics, particularly in the study of electrical circuits. his formula calculates the natural frequency at which a circuit will oscillate when no external forces are driving it. It is expressed as:

𝑓₀ = 1 / ( 2 x 𝜋 x √𝐿 x 𝐶​ )

• 𝐿 represents the inductance.
• 𝐶 represents the capacitance within the circuit.
• The term 2𝜋 provides the necessary scaling factor to convert the angular frequency, typically used in calculations involving radians, into a more universally applicable form.

The concept of the resonant frequency formula was developed in the early 20th century, significantly influenced by the work of the physicist Heinrich Hertz. Hertz was not directly responsible for this specific formula, but his pioneering work on electromagnetic waves set the stage for the further development of electrical resonant systems. The formula is essential for designing circuits in radios, televisions, and other electronic devices that need to filter out specific frequencies from a spectrum of signals. Understanding and applying this formula allows engineers and physicists to precisely control the frequencies at which circuits operate, optimizing performance across a wide range of technologies.

Derivation of Resonant Frequency Formula

To understand the derivation of the resonant frequency formula in a series RLC circuit (resistor 𝑅, inductor 𝐿, and capacitor 𝐶 connected in series), we start by calculating the total impedance 𝑍 of the circuit when excited by an alternating current (AC) source. The impedance 𝑍 is given by:

𝑍 = 𝑅 + 𝑗𝜔𝐿 − ( 𝑗 / 𝜔𝐶​ )

where 𝜔ω is the angular frequency, and 𝑗j represents the imaginary unit. Simplifying the imaginary components, we write:

𝑍 = 𝑅 + 𝑗 (𝜔𝐿− (1 / 𝜔𝐶) )

At resonance, the circuit behaves purely resistively, which implies that the imaginary part of the impedance must be zero. This condition ensures that the inductive and capacitive reactances cancel each other out. Thus, setting the imaginary part equal to zero, we get:

𝜔𝐿 − ( 1 / 𝜔𝐶 ) = 0

Solving for 𝜔ω, the angular frequency at resonance, we find:

𝜔𝐿 = 1 / 𝜔𝐶

𝜔² = 1 / 𝐿𝐶

𝜔=1 / √𝐿𝐶​

The angular frequency 𝜔ω is related to the frequency 𝑓f by the relation 𝜔=2𝜋𝑓ω=2πf. Substituting 𝜔ω in the formula for frequency, we derive:

𝑓 = 𝜔² 𝜋 = 1 / 2𝜋 √𝐿𝐶

Thus, the resonant frequency 𝑓₀​ for the series RLC circuit is given by:

𝑓₀ = 1 / 2𝜋 √𝐿𝐶

Usage of Resonant Frequency Formula

1. Radio Tuning: Engineers use the formula to design radio receivers and transmitters that can select and isolate specific frequencies from the broad spectrum of radio waves.
2. Filter Design: The formula helps in designing filters that pass or block specific frequency bands, crucial in a and telecommunications systems.
3. Antenna Design: It determines the optimal operation frequency for antennas, ensuring they efficiently transmit and receive electromagnetic waves.
4. Medical Equipment: In medical imaging technologies, such as MRI machines, the formula is applied to tune circuits to precise frequencies needed for accurate imaging.
5. Oscillators: Electronic oscillators use this formula to generate stable frequencies for clocks, watches, and signal generators.

Example Problems on Resonant Frequency Formula

Problem 1: Basic Calculation

Question: Determine the resonant frequency of a circuit if the inductance 𝐿 is 10 millihenries and the capacitance 𝐶 is 100 picofarads.

Solution:

Convert units: 𝐿=10 millihenries = 10 × 10⁻³ henries, 𝐶=100 picofarads = 100 ×10⁻¹² farads.

Apply the formula:

𝑓₀ = 1/ 2𝜋 √( (10 × 10⁻³) x (100×10⁻¹²) ) =1 / 2𝜋 √10⁻⁶ = 1 / 2𝜋×10⁻³ =10.002 x 𝜋 ≈159.155 kHz

Problem 2: Effects of Changing Capacitance

Question: If the inductance in a circuit is fixed at 5 millihenries and the resonant frequency needs to be 200 kHz, what must be the capacitance?

Solution:

Convert the inductance to henries: 𝐿=5 millihenries = 5×10⁻³ henries.

Rearrange the resonant frequency formula to solve for 𝐶:

𝑓₀=1 / 2𝜋 √ 𝐿𝐶​

𝐶=1 / (2𝜋𝑓₀ )² 𝐿 =1 / ( 2𝜋 × 200 × 10³ )² × 5 × 10⁻³ ≈31.831 picofarads

Problem 3: Designing a Radio Tuner

Question: A radio tuner circuit requires a resonant frequency of 1 MHz. If the available capacitor is 500 picofarads, what inductance is necessary?

Solution:

Convert the capacitance: 𝐶=500 picofarads = 500 × 10⁻¹² farads.

Rearrange the formula to solve for 𝐿:

𝐿=1 / (2𝜋𝑓₀)² 𝐶 = 1 / (2𝜋 × 1 × 10⁶ )² × 500 × 10⁻¹² ≈ 10.178 microhenries

FAQs

What is the Resonant Frequency of a Room?

The resonant frequency of a room depends on its dimensions and reflects the sound frequency most amplified within that space.

How Do You Calculate Resonant Frequency?

Calculate resonant frequency using 𝑓₀ = 1 / 2𝜋√𝐿𝐶, where 𝐿 is inductance and 𝐶 is capacitance.

Does Everything Have a Resonant Frequency?

Yes, all objects have a resonant frequency, which is the frequency at which they naturally oscillate when disturbed.