What is the square of 77?
5929
5930
5931
5932

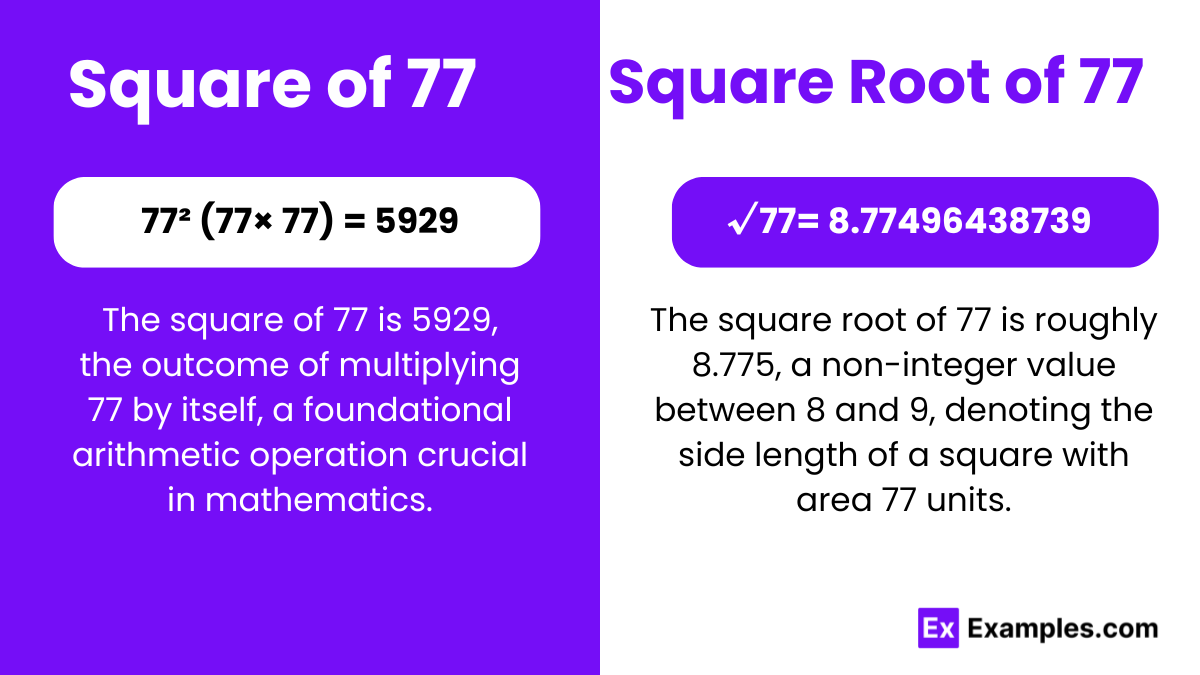
In the domain of mathematics, particularly within algebraic studies, the foundational principles of squares and square roots stand as paramount. Squaring a number, such as taking 77 and multiplying it by itself to yield 5929, is a fundamental operation. This process serves as a cornerstone in exploring the properties inherent in both rational numbers (expressible as a fraction of two integers) and irrational numbers (which defy expression as a neat fraction). Understanding these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, fostering a deeper insight into the subject’s intricacies.
77² (77 × 77) = 5929
A square number, exemplified by the square of 77, results from multiplying a number by itself. In this case, squaring 77 yields 5929. These numbers play a fundamental role in mathematics, serving as building blocks for understanding various mathematical concepts and relationships.
√77 = 8.77496438739
Or
√77 = 8.774 Upto 3 decimals
The square root of a number, such as the square root of 5929, reveals the original number that, when multiplied by itself, yields the given square. In the case of 77 squared, its square root is 77, indicating the number that produces 5929 when squared.
Square Root of 77: 8.77496438739
Exponential Form: 77^½ or 77^0.5
Radical Form: √77
The square root of 77 is an irrational number
The square root of 77 is an irrational number because it cannot be expressed as a fraction of two integers. It is a non-repeating, non-terminating decimal. This classification aligns with the general property of square roots of non-perfect squares being irrational.
Rational Number:
A rational number can be expressed as a fraction of two integers, denoted as a/b, where b isn’t zero. Examples include positive, negative, or zero values, like 3/4, -5/2, 0, 1, -2, etc.
Example: For instance, 3/4 is rational as both 3 and 4 are integers, and the denominator isn’t zero.
Irrational Number:
An irrational number, such as √2 or π, cannot be expressed as a fraction of two integers. Its decimal expansion neither ends nor repeats, thus defying representation as a/b.
Example: Consider √2; its decimal expansion (√2 ≈ 1.41421356…) is non-repeating and non-terminating, making it irrational.
Finding the exact value of the square root of 77 can be challenging because it is an irrational number. However,
you can approximate its value using various methods such as the Babylonian method or numerical methods like Newton’s method. Here’s a brief explanation of the Babylonian method:
Initial Guess: Start with a reasonable initial guess. A common choice is to start with half of the number you’retrying to find the square root of, so for √77, you could start with around 8.5 because 8.5² is 72.25, which is close to 77.
Iteration: Use the formula (xⁿ⁺¹ = 1/2 xₙ + S/xₙ) where (xₙ) is the current guess and (S) is the number you’re finding the square root of (in this case, 77). Repeat this formula with the initial guess to get a better approximation.
Repeat: Keep applying the formula until you reach a desired level of accuracy or until the approximation stops changing significantly.
This method converges relatively quickly to the actual value of the square root.
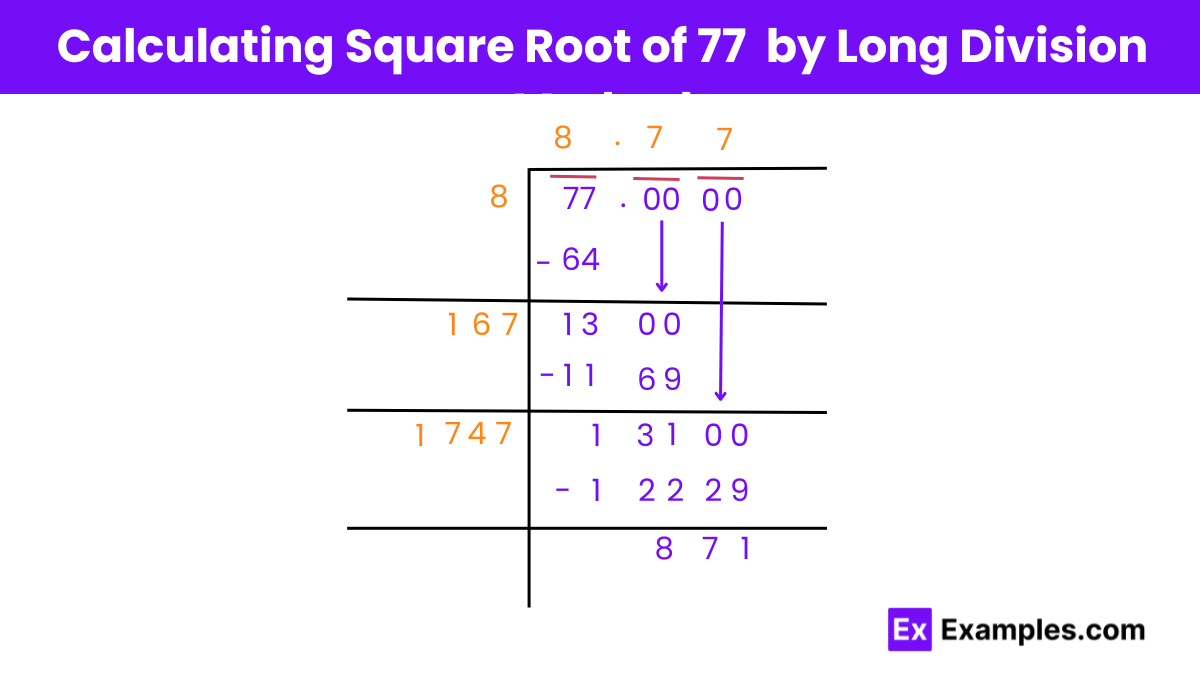
Sure, here’s a breakdown of the steps to find the square root of 77 using the long division method, organized into subheadings:
Starting from the right, pair up the digits of 77 by placing a bar above them.
Find a number that, when multiplied by itself, gives a product less than or equal to 77.
The number 8 fits here as (8² = 64).
Divide 77 by 8, giving a quotient of 8 and a remainder of 13.
Drag a pair of 0s down and place them next to the remainder (13) to make the new dividend 1300.
Double the divisor (8) to get 16 and enter it below with a blank digit on its right.
Think of a number that is greater than or equal to the dividend, i.e., 1300.
167 is a suitable number to divide 1300.
Multiply 167 by 7 ((167 × 7 = 1169 < 1300)) and write the product below.
Subtract the product from 1300, leaving a remainder of 131.
Repeat the process until you get the desired level of accuracy in your approximation of the square root of 77.
Following these steps systematically will help you find the square root of 77 using the long division method.
No, 77 is not a perfect square number
No, 77 is not a perfect square. A perfect square is a number that can be expressed as the square of an integer. Since the square root of 77 is not an integer (it’s approximately 8.774964387), 77 is not a perfect square.
No, the square root of 77 cannot be simplified further as 77 is not a perfect square and has no perfect square factors other than 1.
The prime factorization of 77 is 7 × 11
Knowing the square root of a number is useful in various fields such as mathematics, physics, engineering, and computer science for solving equations, calculating distances, and analyzing data.
The square of a number represents the area of a square with sides of that length. It is also used in various algebraic and geometric calculations.

In the domain of mathematics, particularly within algebraic studies, the foundational principles of squares and square roots stand as paramount. Squaring a number, such as taking 77 and multiplying it by itself to yield 5929, is a fundamental operation. This process serves as a cornerstone in exploring the properties inherent in both rational numbers (expressible as a fraction of two integers) and irrational numbers (which defy expression as a neat fraction). Understanding these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, fostering a deeper insight into the subject’s intricacies.
77² (77 × 77) = 5929
A square number, exemplified by the square of 77, results from multiplying a number by itself. In this case, squaring 77 yields 5929. These numbers play a fundamental role in mathematics, serving as building blocks for understanding various mathematical concepts and relationships.
√77 = 8.77496438739
Or
√77 = 8.774 Upto 3 decimals
The square root of a number, such as the square root of 5929, reveals the original number that, when multiplied by itself, yields the given square. In the case of 77 squared, its square root is 77, indicating the number that produces 5929 when squared.
Square Root of 77: 8.77496438739
Exponential Form: 77^½ or 77^0.5
Radical Form: √77
The square root of 77 is an irrational number
The square root of 77 is an irrational number because it cannot be expressed as a fraction of two integers. It is a non-repeating, non-terminating decimal. This classification aligns with the general property of square roots of non-perfect squares being irrational.
Rational Number:
A rational number can be expressed as a fraction of two integers, denoted as a/b, where b isn’t zero. Examples include positive, negative, or zero values, like 3/4, -5/2, 0, 1, -2, etc.
Example: For instance, 3/4 is rational as both 3 and 4 are integers, and the denominator isn’t zero.
Irrational Number:
An irrational number, such as √2 or π, cannot be expressed as a fraction of two integers. Its decimal expansion neither ends nor repeats, thus defying representation as a/b.
Example: Consider √2; its decimal expansion (√2 ≈ 1.41421356…) is non-repeating and non-terminating, making it irrational.
Finding the exact value of the square root of 77 can be challenging because it is an irrational number. However,
you can approximate its value using various methods such as the Babylonian method or numerical methods like Newton’s method. Here’s a brief explanation of the Babylonian method:
Initial Guess: Start with a reasonable initial guess. A common choice is to start with half of the number you’retrying to find the square root of, so for √77, you could start with around 8.5 because 8.5² is 72.25, which is close to 77.
Iteration: Use the formula (xⁿ⁺¹ = 1/2 xₙ + S/xₙ) where (xₙ) is the current guess and (S) is the number you’re finding the square root of (in this case, 77). Repeat this formula with the initial guess to get a better approximation.
Repeat: Keep applying the formula until you reach a desired level of accuracy or until the approximation stops changing significantly.
This method converges relatively quickly to the actual value of the square root.

Sure, here’s a breakdown of the steps to find the square root of 77 using the long division method, organized into subheadings:
Starting from the right, pair up the digits of 77 by placing a bar above them.
Also, pair up the 0s in the decimal part from left to right.
Find a number that, when multiplied by itself, gives a product less than or equal to 77.
The number 8 fits here as (8² = 64).
Divide 77 by 8, giving a quotient of 8 and a remainder of 13.
Drag a pair of 0s down and place them next to the remainder (13) to make the new dividend 1300.
Double the divisor (8) to get 16 and enter it below with a blank digit on its right.
Think of a number that is greater than or equal to the dividend, i.e., 1300.
167 is a suitable number to divide 1300.
Multiply 167 by 7 ((167 × 7 = 1169 < 1300)) and write the product below.
Subtract the product from 1300, leaving a remainder of 131.
Repeat the process until you get the desired level of accuracy in your approximation of the square root of 77.
Following these steps systematically will help you find the square root of 77 using the long division method.
No, 77 is not a perfect square number
No, 77 is not a perfect square. A perfect square is a number that can be expressed as the square of an integer. Since the square root of 77 is not an integer (it’s approximately 8.774964387), 77 is not a perfect square.
No, the square root of 77 cannot be simplified further as 77 is not a perfect square and has no perfect square factors other than 1.
The prime factorization of 77 is 7 × 11
Knowing the square root of a number is useful in various fields such as mathematics, physics, engineering, and computer science for solving equations, calculating distances, and analyzing data.
The square of a number represents the area of a square with sides of that length. It is also used in various algebraic and geometric calculations.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 77?
5929
5930
5931
5932
Which number is closest to the square root of 77?
8.5
8.7
8.8
8.9
If x² = 77, what is the approximate value of x?
8.6
8.7
8.8
8.9
What is √77 rounded to the nearest whole number?
8
9
10
11
Simplify √77 to its decimal form.
8.73
8.74
8.75
8.76
What is the result of 77²?
5929
5930
5931
5932
Which integer squared is closest to 77?
8²
9²
10²
11²
If √77 is represented as a fraction, what is its approximate value?
8.73
8.74
8.75
8.76
Calculate 77 × 77.
5924
5926
5928
5929
Which two consecutive integers does √77 fall between?
8 and 9
7 and 8
9 and 10
8 and 10
Before you leave, take our quick quiz to enhance your learning!

