What is the square of 841?
688,921
705,600
705,841
730,001

In the domain of algebraic mathematics, the foundational principles of squares and square roots are pivotal. Squaring a number, demonstrated by multiplying 841 by itself to obtain 706440, is fundamental. This operation forms a cornerstone for exploring properties within rational numbers (expressible as a fraction) and irrational numbers (resisting neat fraction representation). Understanding these core concepts enriches comprehension of mathematical relationships and patterns. Mastery of these fundamentals enhances one’s ability to analyze equations, discern numerical trends, and grasp the essence of mathematical structures. Thus, within algebraic studies, squares, square roots, and fractions collectively form the bedrock upon which deeper mathematical understanding is built.
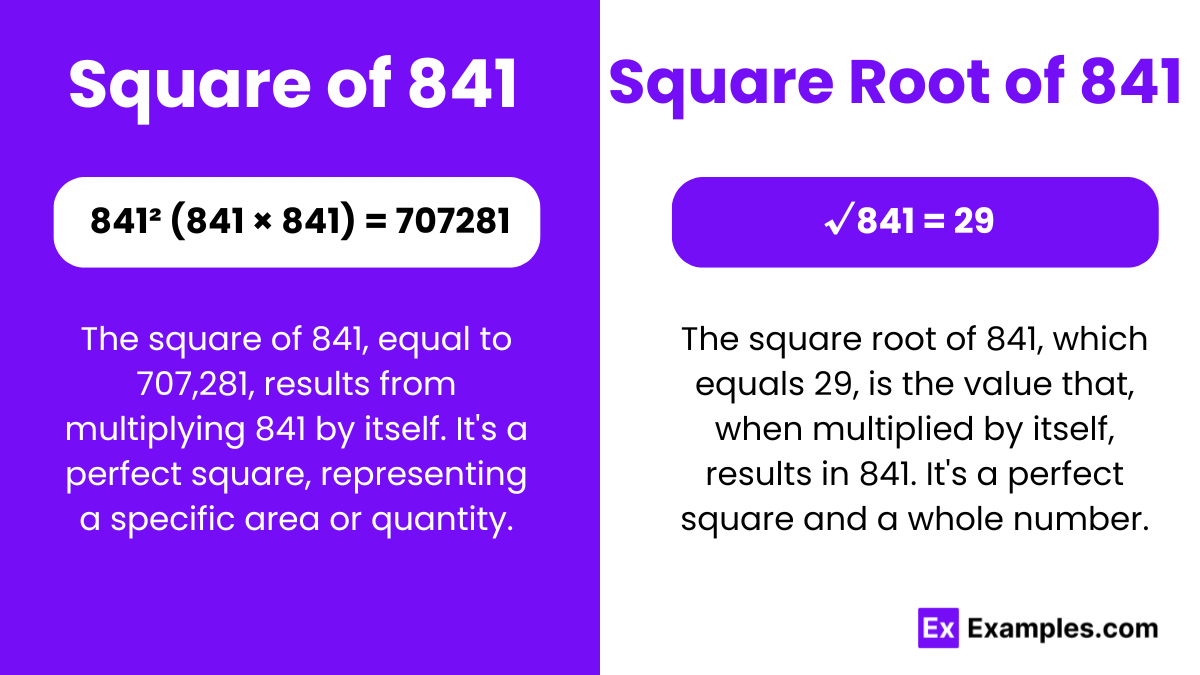
The square of 841, a square number, equals 706,481. In mathematics, a square number results from multiplying a number by itself. Understanding square numbers, like 841, is crucial in algebraic studies, providing foundational knowledge for exploring numerical relationships and patterns in various mathematical contexts.
The square root of 841, a square number, is 29. In mathematics, a square root determines the number that, when multiplied by itself, equals the given number. Knowing square roots, like that of 841, is essential in algebra, facilitating comprehension of equations and mathematical relationships.
Square Root of 841: 29
Exponential Form: 841^½ or 841^0.5
Radical Form: √841
The square root of 841 is rational. This is because 841 is a perfect square (29 * 29 = 841), making its square root a whole number, which is a subset of rational numbers.
Rational Numbers:
Rational numbers are ratios of integers, with a non-zero denominator. They are expressed as a/b where a and b are integers and b ≠ 0. Examples include integers and fractions such as 1/2, -3, and 5/7.
Irrational Numbers:
Irrational numbers cannot be written as fractions of integers and have non-repeating, non-terminating decimal forms. Examples include square roots of non-perfect squares like √2, √3, and √5, and transcendental numbers like π.
In essence, rational numbers can be shown as fractions with finite or repeating decimals, whereas irrational numbers lack this property, with decimal expansions that neither repeat nor terminate.
To find the square root of 841, you can use various methods:
Manual Calculation: Divide 841 by trial-and-error with numbers until you find one that, when squared, equals 841.
Prime Factorization: Express 841 as a product of prime factors (29 * 29), then take the square root of each prime factor.
Estimation: Use approximation techniques like the Babylonian method, starting with an initial guess (e.g., 30) and refining it through successive approximations.
Calculator: Utilize a scientific or graphing calculator with a square root function to directly compute the square root of 841.
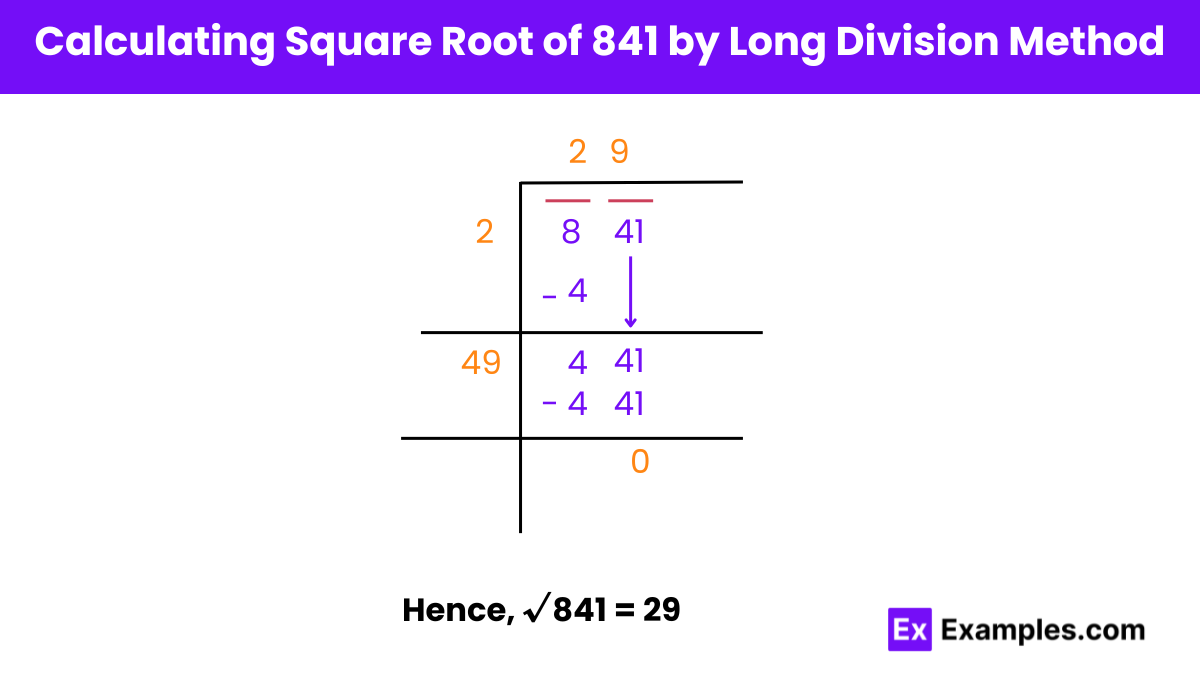
Long Division Method to Find Square Root of 841
Step 1: Setup
Begin with writing the number 841. Pair the digits by placing a bar on top from right to left.
Step 2: Initial Division
Divide the first digit, 8, by a number such that the product is less than or equal to 8. In this case, 2×2=4. Hence, quotient = 2, remainder = 4.
Step 3: Iterative Division
Double the quotient (2), obtaining 4, and make it the new divisor. Bring down the next digit (1), yielding 41. Determine a number such that (40 + that number) × that number ≤ 441. (40+9) × 9 = 441.
Step 4: Subtraction
Subtract the product from the previous dividend. The remainder is 00, and the new quotient is 29.
Step 5: Conclusion
Therefore, the square root of 841, √841, is 29.
Yes, 841 is a perfect square. Its square root is 29. In mathematics, a perfect square is a number that can be expressed as the product of an integer multiplied by itself. Since 29 * 29 = 841, 841 is indeed a perfect square.
No, the square root of 841 is an integer (29), so it cannot be expressed as a fraction.
How does finding the square root of 841 relate to real-life situations?
The square root of 841 might be relevant in scenarios involving area or dimensions of a square with an area of 841 square units.
What is the significance of the number 841 in mathematics?
841 is the square of 29 and holds importance as a perfect square, contributing to patterns and relationships within number theory.
How does the square of 841 contribute to understanding patterns in mathematics?
The square of 841 is part of a sequence of perfect squares, aiding in recognizing patterns and relationships in numerical sequences.
Can you provide practical examples of using the square root of 841?
The square root of 841 might be used in engineering calculations, construction, or any situation requiring precise measurement of area or length.

In the domain of algebraic mathematics, the foundational principles of squares and square roots are pivotal. Squaring a number, demonstrated by multiplying 841 by itself to obtain 706440, is fundamental. This operation forms a cornerstone for exploring properties within rational numbers (expressible as a fraction) and irrational numbers (resisting neat fraction representation). Understanding these core concepts enriches comprehension of mathematical relationships and patterns. Mastery of these fundamentals enhances one’s ability to analyze equations, discern numerical trends, and grasp the essence of mathematical structures. Thus, within algebraic studies, squares, square roots, and fractions collectively form the bedrock upon which deeper mathematical understanding is built.
841² (841 × 841) = 706440
The square of 841, a square number, equals 706,481. In mathematics, a square number results from multiplying a number by itself. Understanding square numbers, like 841, is crucial in algebraic studies, providing foundational knowledge for exploring numerical relationships and patterns in various mathematical contexts.
√841 = 29
The square root of 841, a square number, is 29. In mathematics, a square root determines the number that, when multiplied by itself, equals the given number. Knowing square roots, like that of 841, is essential in algebra, facilitating comprehension of equations and mathematical relationships.
Square Root of 841: 29
Exponential Form: 841^½ or 841^0.5
Radical Form: √841
Yes, the square root of 841 is a rational number
The square root of 841 is rational. This is because 841 is a perfect square (29 * 29 = 841), making its square root a whole number, which is a subset of rational numbers.
Rational Numbers:
Rational numbers are ratios of integers, with a non-zero denominator. They are expressed as a/b where a and b are integers and b ≠ 0. Examples include integers and fractions such as 1/2, -3, and 5/7.
Irrational Numbers:
Irrational numbers cannot be written as fractions of integers and have non-repeating, non-terminating decimal forms. Examples include square roots of non-perfect squares like √2, √3, and √5, and transcendental numbers like π.
In essence, rational numbers can be shown as fractions with finite or repeating decimals, whereas irrational numbers lack this property, with decimal expansions that neither repeat nor terminate.
To find the square root of 841, you can use various methods:
Manual Calculation: Divide 841 by trial-and-error with numbers until you find one that, when squared, equals 841.
Prime Factorization: Express 841 as a product of prime factors (29 * 29), then take the square root of each prime factor.
Estimation: Use approximation techniques like the Babylonian method, starting with an initial guess (e.g., 30) and refining it through successive approximations.
Calculator: Utilize a scientific or graphing calculator with a square root function to directly compute the square root of 841.

Long Division Method to Find Square Root of 841
Step 1: Setup
Begin with writing the number 841. Pair the digits by placing a bar on top from right to left.
Step 2: Initial Division
Divide the first digit, 8, by a number such that the product is less than or equal to 8. In this case, 2×2=4. Hence, quotient = 2, remainder = 4.
Step 3: Iterative Division
Double the quotient (2), obtaining 4, and make it the new divisor. Bring down the next digit (1), yielding 41. Determine a number such that (40 + that number) × that number ≤ 441. (40+9) × 9 = 441.
Step 4: Subtraction
Subtract the product from the previous dividend. The remainder is 00, and the new quotient is 29.
Step 5: Conclusion
Therefore, the square root of 841, √841, is 29.
Yes, 841 is a perfect square. Its square root is 29. In mathematics, a perfect square is a number that can be expressed as the product of an integer multiplied by itself. Since 29 * 29 = 841, 841 is indeed a perfect square.
No, the square root of 841 is an integer (29), so it cannot be expressed as a fraction.
How does finding the square root of 841 relate to real-life situations?
The square root of 841 might be relevant in scenarios involving area or dimensions of a square with an area of 841 square units.
What is the significance of the number 841 in mathematics?
841 is the square of 29 and holds importance as a perfect square, contributing to patterns and relationships within number theory.
How does the square of 841 contribute to understanding patterns in mathematics?
The square of 841 is part of a sequence of perfect squares, aiding in recognizing patterns and relationships in numerical sequences.
Can you provide practical examples of using the square root of 841?
The square root of 841 might be used in engineering calculations, construction, or any situation requiring precise measurement of area or length.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 841?
688,921
705,600
705,841
730,001
Which of the following is the square root of 841?
28
29
30
31
If x² = 841, what is the value of x?
28
29
30
31
Which number, when squared, results in 841?
27
28
29
30
Calculate the approximate square root of 841.
28.5
29.0
29.5
30.0
What is the result of squaring the number 29?
800
820
841
860
If the square root of a number is 29, what is the number?
811
821
831
841
What is the value of 841 raised to the power of 0.5?
25
28
29
32
If you know the square root of 841 is 29, what is 29²?
810
830
841
860
What is the value of 841 minus 841²?
0
841
1682
1681
Before you leave, take our quick quiz to enhance your learning!

