What is the square of 97?
9409
9604
9801
10000

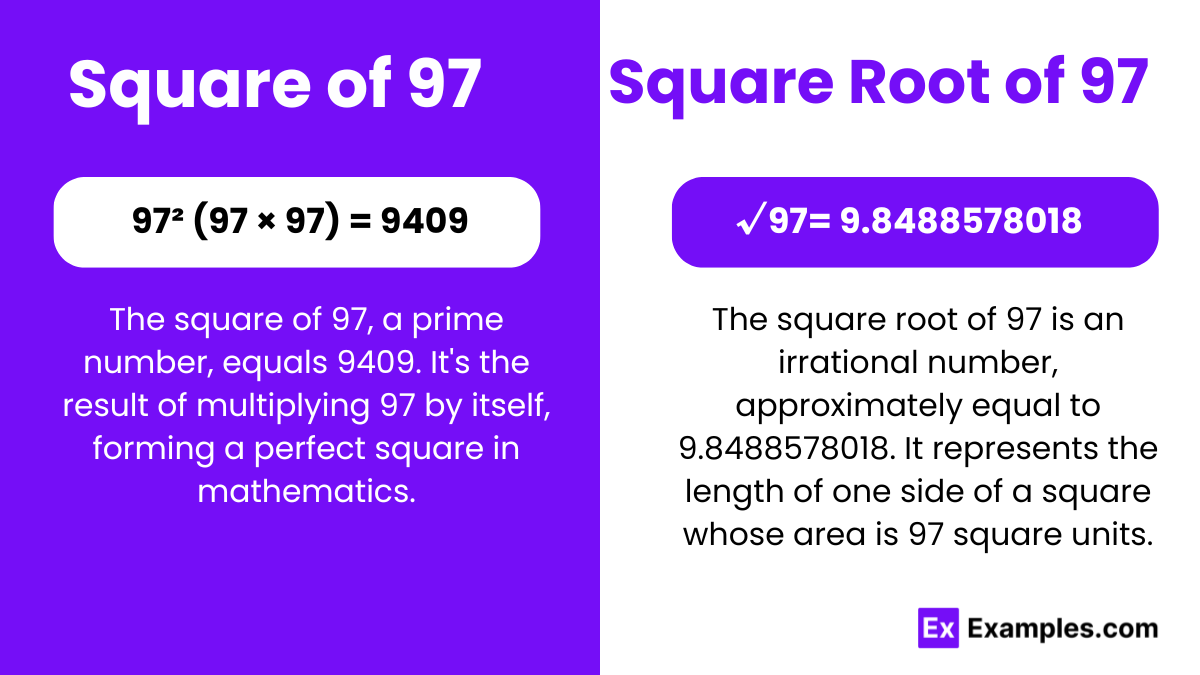
Within the realm of mathematics, particularly in algebraic studies, the foundational concepts of squares and square roots are pivotal. The process of squaring a number, such as 97, entails multiplying it by itself to yield 9409, underscoring a fundamental operation. This operation serves as a cornerstone in probing the properties inherent in both rational numbers, expressible as fractions, and irrational numbers, defying neat fractional expressions. Understanding these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, essential in exploring the intricacies of numerical systems. These concepts are integral to various mathematical disciplines, fostering a deeper insight into the interconnectedness and significance of algebraic principles in mathematical discourse.
97² (97 × 97) = 9409
A square number, like 576, is the result of multiplying an integer by itself. Its square root is 24, the number that, when multiplied by itself, equals 576. In contrast, the square root of 97 is an irrational number, approximately 9.85, requiring methods like prime factorization for calculation.
√97 = 9.8488578018
Or
√97 = 9.848 Upto 3 decimals
“The square root of 576, a perfect square, is 24, as 24 multiplied by itself equals 576. Conversely, the square root of 97 is an irrational number, approximately 9.85. Understanding square roots unveils the relationship between numbers and their squares, integral to mathematical exploration and problem-solving.”
Square Root of 97: 9.8488578018
Exponential Form: 97^½ or 97^0.5
Radical Form: √97
The square root of 97 is an irrational number
The square root of 97 is an irrational number. It cannot be expressed as a fraction of two integers. Approximated to about 9.85, it signifies a non-repeating, non-terminating decimal. This characteristic distinguishes it from rational numbers, illustrating the diverse nature of mathematical expressions.
Rational number : Rational number is one that can be expressed as a fraction of two integers, such as 3/4 or -5/2. These numbers possess finite or repeating decimal representations.
Irrational number : Irrational number cannot be expressed as a fraction of two integers, and its decimal expansion neither terminates nor repeats. For instance, the square root of 2 (√2) is approximately 1.41421356…, exemplifying irrationality. These numbers unveil a distinct property in mathematics due to their non-repeating, non-terminating nature, challenging conventional numerical representations.
Several methods can be employed to find the value of the square root of 97:
Each method offers its own advantages and may be chosen based on computational ease and accuracy requirements.
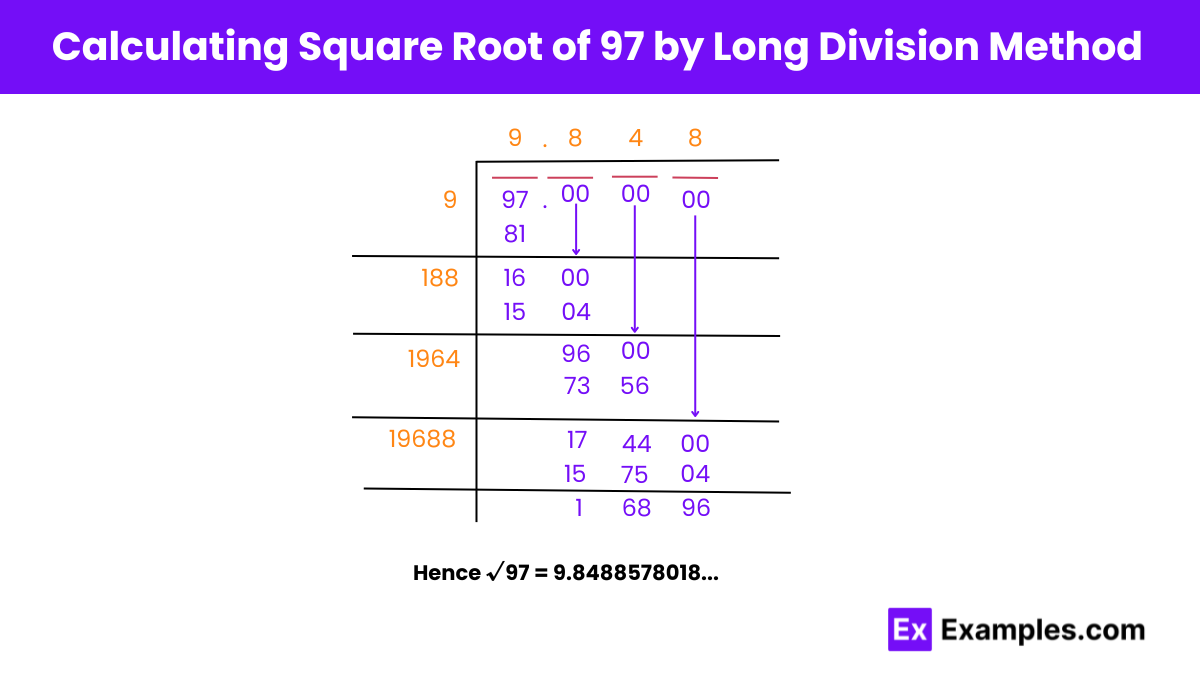
Finding Square Root of 97 Using Long Division
Step 1: Pairing Digits
Start by pairing the digits of the given number, starting with the digit at the one’s place, and indicate the pairing with a horizontal bar.
Step 2: Initial Divisor
Find a number that, when squared, gives a product less than or equal to 97. Since 9 × 9 = 81 ≤ 97, the initial divisor is 9, and the quotient is also 9. Proceed with the division.
Step 3: Updating Divisor
Bring down the next pair of digits (00) and multiply the quotient by 2 to get 18, which becomes the starting digits of the new divisor.
Step 4: Division
Determine the next digit of the quotient by finding the largest digit that, when appended to the current quotient, produces a product less than or equal to the current partial dividend. Proceed with the division to get the remainder.
Step 5-8: Repeat the Process
Continue bringing down pairs of digits and updating the divisor until the desired level of precision is reached or until a satisfactory approximation of the square root of 97 is obtained. Repeat the division process until the remainder is negligible or within an acceptable margin of error.
No, 97 is not a perfect square number
97 is not a perfect square; it lacks integer factors. Therefore, its square root is irrational. Approximated to about 9.85, it denotes a non-repeating, non-terminating decimal. The absence of integer factors distinguishes it from perfect squares, showcasing the diverse nature of mathematical expressions.
No, the square root of 97 cannot be expressed as a fraction of two integers since it is an irrational number.
No, the square root of 97 is neither terminating nor repeating; it is a non-repeating, non-terminating decimal.
It depends on your level of precision. Typically, approximating to several decimal places like 9.8488578 is sufficient for most calculations.
No, the square root of 97 cannot be simplified further using radicals since it is an irrational number.
The square root of 97 may be used in various fields such as mathematics, physics, engineering, and finance for calculations involving areas, distances, and measurements.
Compared to perfect square roots, like √100 = 10, the square root of 97 is irrational and lies between the perfect squares of 9 and 10.

Within the realm of mathematics, particularly in algebraic studies, the foundational concepts of squares and square roots are pivotal. The process of squaring a number, such as 97, entails multiplying it by itself to yield 9409, underscoring a fundamental operation. This operation serves as a cornerstone in probing the properties inherent in both rational numbers, expressible as fractions, and irrational numbers, defying neat fractional expressions. Understanding these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, essential in exploring the intricacies of numerical systems. These concepts are integral to various mathematical disciplines, fostering a deeper insight into the interconnectedness and significance of algebraic principles in mathematical discourse.
97² (97 × 97) = 9409
A square number, like 576, is the result of multiplying an integer by itself. Its square root is 24, the number that, when multiplied by itself, equals 576. In contrast, the square root of 97 is an irrational number, approximately 9.85, requiring methods like prime factorization for calculation.
√97 = 9.8488578018
Or
√97 = 9.848 Upto 3 decimals
“The square root of 576, a perfect square, is 24, as 24 multiplied by itself equals 576. Conversely, the square root of 97 is an irrational number, approximately 9.85. Understanding square roots unveils the relationship between numbers and their squares, integral to mathematical exploration and problem-solving.”
Square Root of 97: 9.8488578018
Exponential Form: 97^½ or 97^0.5
Radical Form: √97
The square root of 97 is an irrational number
The square root of 97 is an irrational number. It cannot be expressed as a fraction of two integers. Approximated to about 9.85, it signifies a non-repeating, non-terminating decimal. This characteristic distinguishes it from rational numbers, illustrating the diverse nature of mathematical expressions.
Rational number : Rational number is one that can be expressed as a fraction of two integers, such as 3/4 or -5/2. These numbers possess finite or repeating decimal representations.
Irrational number : Irrational number cannot be expressed as a fraction of two integers, and its decimal expansion neither terminates nor repeats. For instance, the square root of 2 (√2) is approximately 1.41421356…, exemplifying irrationality. These numbers unveil a distinct property in mathematics due to their non-repeating, non-terminating nature, challenging conventional numerical representations.
Several methods can be employed to find the value of the square root of 97:
Prime Factorization: Express 97 as a product of prime numbers and extract the square root.
Newton’s Method: Iteratively refine an initial guess to approximate the square root.
Binomial Expansion: Use binomial expansion to approximate the square root.
Continued Fractions: Express the square root as a continued fraction and approximate its value.
Each method offers its own advantages and may be chosen based on computational ease and accuracy requirements.

Finding Square Root of 97 Using Long Division
Step 1: Pairing Digits
Start by pairing the digits of the given number, starting with the digit at the one’s place, and indicate the pairing with a horizontal bar.
Step 2: Initial Divisor
Find a number that, when squared, gives a product less than or equal to 97. Since 9 × 9 = 81 ≤ 97, the initial divisor is 9, and the quotient is also 9. Proceed with the division.
Step 3: Updating Divisor
Bring down the next pair of digits (00) and multiply the quotient by 2 to get 18, which becomes the starting digits of the new divisor.
Step 4: Division
Determine the next digit of the quotient by finding the largest digit that, when appended to the current quotient, produces a product less than or equal to the current partial dividend. Proceed with the division to get the remainder.
Step 5-8: Repeat the Process
Continue bringing down pairs of digits and updating the divisor until the desired level of precision is reached or until a satisfactory approximation of the square root of 97 is obtained. Repeat the division process until the remainder is negligible or within an acceptable margin of error.
No, 97 is not a perfect square number
97 is not a perfect square; it lacks integer factors. Therefore, its square root is irrational. Approximated to about 9.85, it denotes a non-repeating, non-terminating decimal. The absence of integer factors distinguishes it from perfect squares, showcasing the diverse nature of mathematical expressions.
No, the square root of 97 cannot be expressed as a fraction of two integers since it is an irrational number.
No, the square root of 97 is neither terminating nor repeating; it is a non-repeating, non-terminating decimal.
It depends on your level of precision. Typically, approximating to several decimal places like 9.8488578 is sufficient for most calculations.
No, the square root of 97 cannot be simplified further using radicals since it is an irrational number.
The square root of 97 may be used in various fields such as mathematics, physics, engineering, and finance for calculations involving areas, distances, and measurements.
Compared to perfect square roots, like √100 = 10, the square root of 97 is irrational and lies between the perfect squares of 9 and 10.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 97?
9409
9604
9801
10000
What is the approximate value of the square root of 97?
9.5
9.8
9.7
9.6
Which of the following is closest to the square of 97?
9600
9700
9800
9900
If x² = 97, what is the approximate value of x?
9.6
9.7
9.8
9.9
Which statement is true about the square root of 97?
It is a whole number.
It is a rational number.
It is an irrational number.
It is an integer.
What is the value of 97 squared minus 1?
9800
9801
9802
9803
Which of the following is the closest integer to the square root of 97?
9
10
11
12
What is the difference between 10000 and the square of 97?
199
200
201
202
If the square root of a number is approximately 9.8, what is the number?
97
98
99
100
Which value represents the closest approximation for the square root of 97 when rounded to one decimal place?
9.7
9.8
9.9
10
Before you leave, take our quick quiz to enhance your learning!

