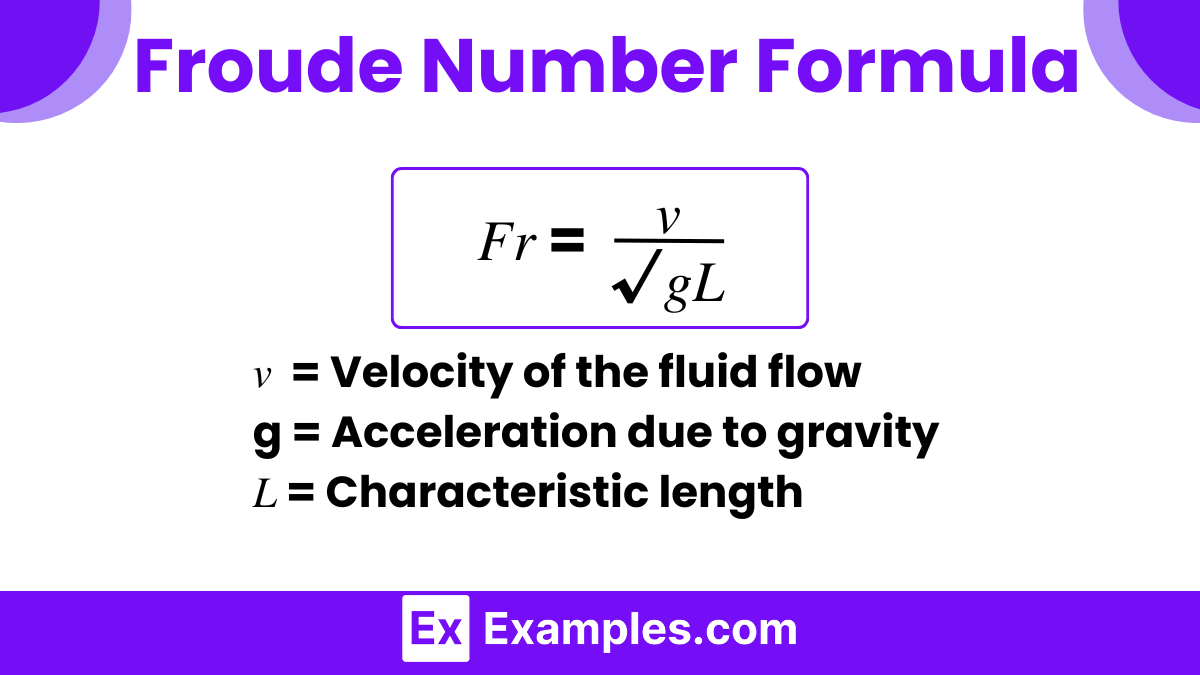
Froude Number Formula

What is Froude Number Formula?

The Froude Number Formula is a fundamental concept in the field of physics, specifically within the study of fluid dynamics. This formula is instrumental in comparing the ratio of inertial forces to gravitational forces in fluid motion scenarios. It was developed by the English engineer William Froude, who discovered it in the 19th century. Froude’s work was primarily aimed at improving ship design and understanding how objects, such as ship hulls, interact with water at various speeds. Derivation of the Froude Number Formula starts by examining the forces that influence a body moving through a fluid. The formula is

𝐹𝑟=𝑣 / √𝑔𝐿

𝑣 stands for the velocity of the fluid flow
g represents the acceleration due to gravity
𝐿 is the characteristic length involved, such as the length of the ship or the wavelength of the waves caused by the movement.

This formula helps determine whether the flow regime in a particular situation is dominated by gravitational forces or by the inertia of the moving fluid. By using the Froude number, physicists and engineers can predict phenomena like wave formation and fluid flow behavior around objects.

This formula has become a cornerstone in the analysis and design of various engineering projects, especially in scenarios where understanding the interaction between fluid and gravitational forces is crucial. It provides a clear, quantitative measure that helps guide decisions in complex fluid dynamic environments.

Applications of Froude Number Formula

Ship Design: Engineers use the Froude number to determine optimal hull shapes and engine power, ensuring stability and efficiency in water.
Bridge Construction: This formula helps assess the potential impact of river currents on bridge piers, guiding design choices to improve safety and durability.
Environmental Studies: The Froude number aids in understanding river flows, which is crucial for managing flood risks and designing flood defenses.
Hydraulic Engineering: Engineers apply it in designing hydraulic structures like weirs and spillways to ensure they effectively handle expected water flow rates.
Coastal Engineering: Engineers use the formula to predict wave breaking patterns and their effects on coastal structures and beach erosion.

Limitations of Froude Number Formula

Scale Sensitivity: The Froude number may not accurately predict fluid behavior at very small or very large scales due to differences in fluid properties and interactions.
Complex Flows: It struggles to provide reliable results in scenarios with complex fluid dynamics, such as turbulent flows or multi-phase flows.
Limited Scenarios: This formula primarily applies to scenarios dominated by gravity and inertial forces, limiting its use in situations where other forces, like viscous forces, are more significant.
Simplifications Needed: To use the Froude number effectively, you often need to simplify the physical model, which can result in less accurate predictions.
Specific Conditions: It does not account for the effects of fluid viscosity and compressibility, which can be critical in certain engineering applications.

Example Problems on Froude Number Formula

Example 1: Calculating the Froude Number for a Boat

Problem: A boat is traveling at a speed of 8 meters per second. The characteristic length of the boat, typically the length of the waterline, is 20 meters. Calculate the Froude number for the boat and determine the type of flow around the boat. Assume gravity 𝑔=9.81 m/s².

Solution:

1) Identify the known values:

Velocity 𝑣=8 m/s

Characteristic length 𝐿=20 m

Acceleration due to gravity 𝑔=9.81 m/s²

2) Apply the Froude number formula:𝐹𝑟=𝑣 / √𝑔𝐿.

𝐹𝑟=8 / √9.81×20

𝐹𝑟 = 8 / √196.2 ≈ 814.01 ≈ 0.571.

3) Interpretation: A Froude number of 0.571 suggests that the flow is sub critical, which means that the gravitational effects are more significant than the inertial effects. This is typical for boats moving at moderate speeds.

Example 2: Determining Boat Speed from a Known Froude Number

Problem: Determine the speed a boat must travel to achieve a Froude number of 0.8 if the characteristic length of the boat is 30 meters.

Solution:

1) Identify the known values:

Froude number 𝐹𝑟=0.8

Characteristic length 𝐿=30 m

Acceleration due to gravity 𝑔=9.81 m/s2

2) Rearrange the Froude number formula to solve for velocity 𝑣: 𝑣=𝐹𝑟 × √𝑔𝐿

𝑣 = 0.8 × √9.81×30

𝑣 = 0.8 × √294.3 ≈ 0.8 × 17.15 ≈ 13.72 m/s

Conclusion: The boat needs to travel at approximately 13.72 meters per second to achieve a Froude number of 0.8. This speed is in the transitional range, nearing the critical point where inertial forces begin to match gravitational forces.

FAQs

What Does Froude Number Tell You?

The Froude number indicates the relationship between a fluid’s inertial and gravitational forces, essential for analyzing fluid dynamics.

How Is the Froude Number Calculated?

The Froude number is calculated by using the formula 𝐹𝑟 = 𝑣 / √𝑔𝐿. Here, v is velocity, 𝑔 gravity, and 𝐿 characteristic length.

What Is the Froude Number for Strong Jump?

A strong hydraulic jump occurs at a Froude number greater than 1, indicating supercritical flow conditions before the jump.

Froude Number Formula