What is the Greatest Common Factor (GCF) of 18 and 30?
2
3
6
9

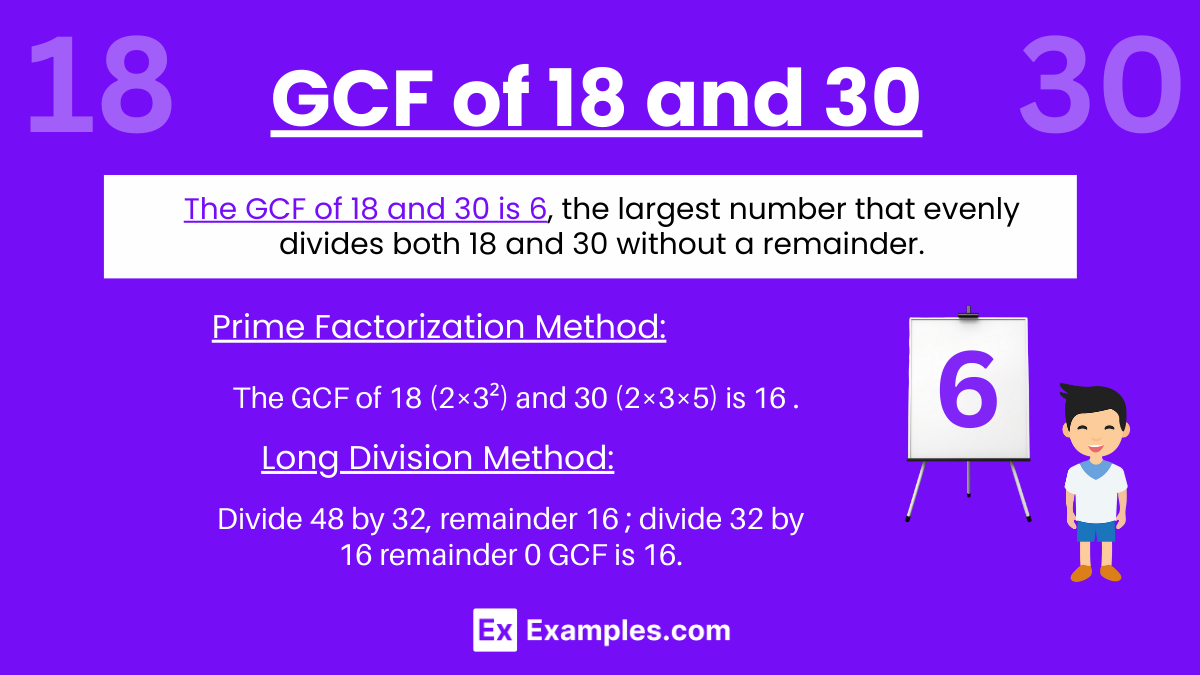
To find the Greatest Common Factor (GCF) of 18 and 30, list the factors of each numbers: the factors of 18 are 1, 2, 3, 6, 9, and 18, while the factors of 30 are 1, 2, 3, 5, 6, 10, 15, and 30. The common factors are 1, 2, 3, and 6, with the greatest common factor being 6. Using the prime factorization method, 18 can be expressed as 2 × 3² and 30 as 2 × 3 × 5, confirming that the common prime factors are 2 and 3. Multiplying these common prime factors (2 × 3) also gives the GCF as 6. Thus, the GCF of 18 and 30 is 6.
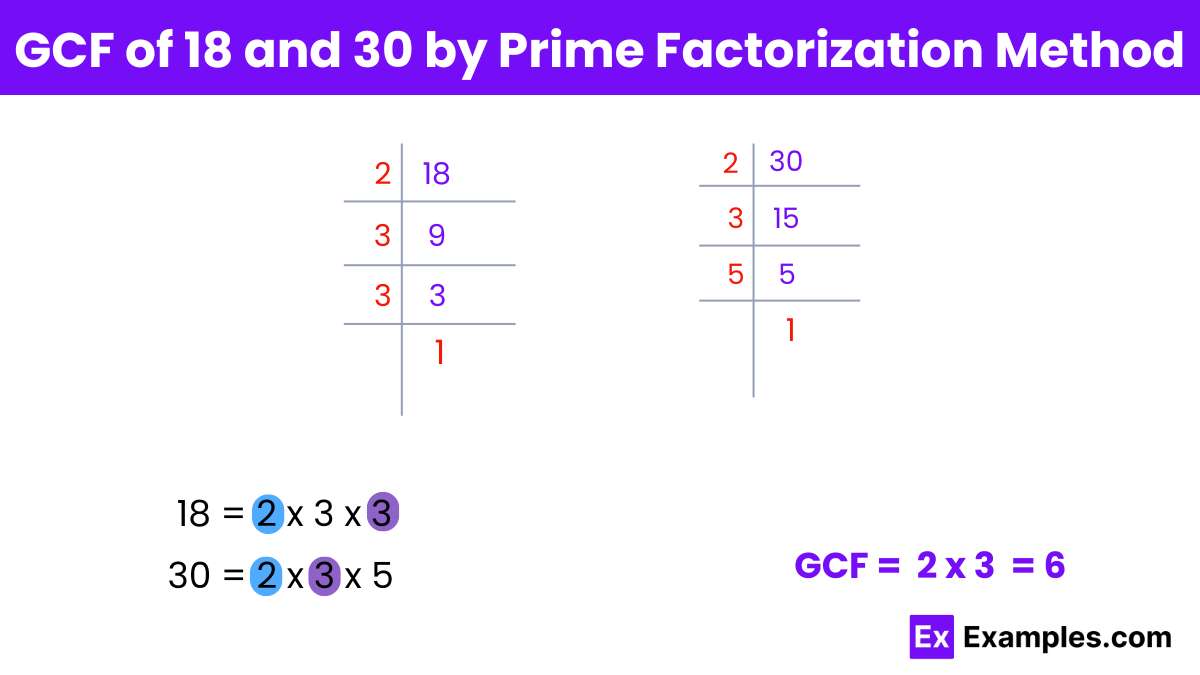
To find the Greatest Common Factor (GCF) of 18 and 30 using the prime factorization method, follow these steps:
Prime factorization of 18:
18 = 2 × 3 × 3 = 2 × 3²
Prime factorization of 30:
30 = 2 × 3 × 5
The common prime factors of 18 and 30 are 2 and 3.
The lowest powers of the common prime factors are 2 and 3. Therefore, multiply these together: 2 × 3 = 6.
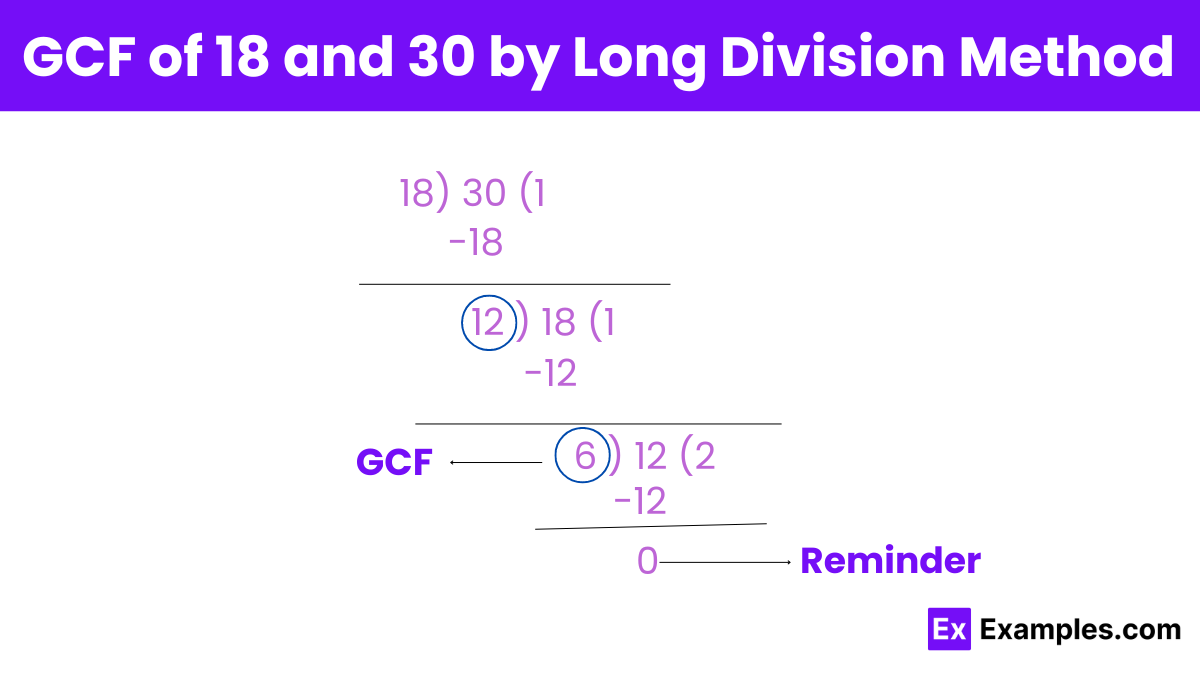
To find the Greatest Common Factor (GCF) of 18 and 30 using the long division method, follow these steps:
30 ÷ 18 = 1 with a remainder of 12.
Now, we have 18 and 12.
18 ÷ 12 = 1 with a remainder of 6.
12 ÷ 6 = 2 with a remainder of 0.
The last non-zero remainder is 6. So, the GCF of 18 and 30 is 6.
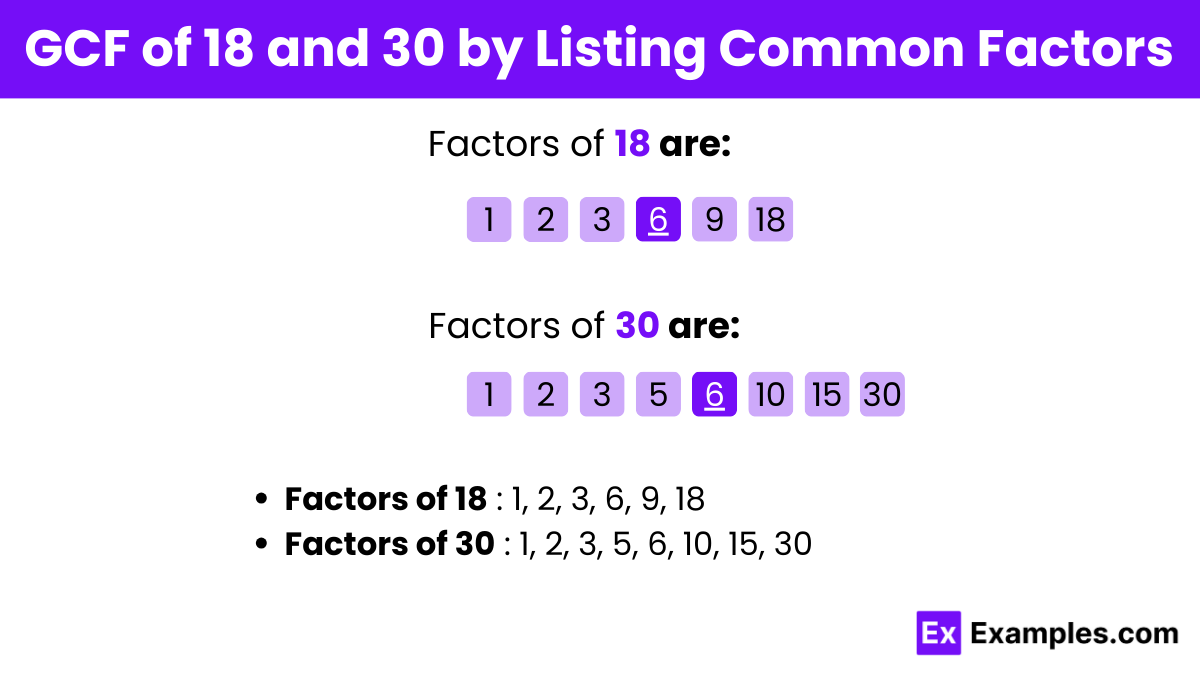
To find the Greatest Common Factor (GCF) of 18 and 30 by listing common factors, follow these steps:
Factors of 18:
1, 2, 3, 6, 9, 18
Factors of 30:
1, 2, 3, 5, 6, 10, 15, 30
Common factors of 18 and 30:
1, 2, 3, 6
The largest common factor is 6. Therefore, the GCF of 18 and 30 is 6.
It helps in tasks such as reducing ratios and simplifying fractions for practical applications.
No, the GCF is never zero.
The LCM of 18 and 30 is 90.
By dividing both numbers by the GCF to check if the quotients are integers.
Yes, if one number is a multiple of the other. For 18 and 30, the GCF is 6.
The common factors of 18 and 30 are 1, 2, 3, and 6.

To find the Greatest Common Factor (GCF) of 18 and 30, list the factors of each numbers: the factors of 18 are 1, 2, 3, 6, 9, and 18, while the factors of 30 are 1, 2, 3, 5, 6, 10, 15, and 30. The common factors are 1, 2, 3, and 6, with the greatest common factor being 6. Using the prime factorization method, 18 can be expressed as 2 × 3² and 30 as 2 × 3 × 5, confirming that the common prime factors are 2 and 3. Multiplying these common prime factors (2 × 3) also gives the GCF as 6. Thus, the GCF of 18 and 30 is 6.
Prime Factorization Method
Long Division Method
Listing Common Factors
To find the Greatest Common Factor (GCF) of 18 and 30 using the prime factorization method, follow these steps:
Prime factorization of 18:
18 = 2 × 3 × 3 = 2 × 3²
Prime factorization of 30:
30 = 2 × 3 × 5
The common prime factors of 18 and 30 are 2 and 3.
The lowest powers of the common prime factors are 2 and 3. Therefore, multiply these together: 2 × 3 = 6.
To find the Greatest Common Factor (GCF) of 18 and 30 using the long division method, follow these steps:
30 ÷ 18 = 1 with a remainder of 12.
Now, we have 18 and 12.
18 ÷ 12 = 1 with a remainder of 6.
12 ÷ 6 = 2 with a remainder of 0.
The last non-zero remainder is 6. So, the GCF of 18 and 30 is 6.
To find the Greatest Common Factor (GCF) of 18 and 30 by listing common factors, follow these steps:
Factors of 18:
1, 2, 3, 6, 9, 18
Factors of 30:
1, 2, 3, 5, 6, 10, 15, 30
Common factors of 18 and 30:
1, 2, 3, 6
The largest common factor is 6. Therefore, the GCF of 18 and 30 is 6.
It helps in tasks such as reducing ratios and simplifying fractions for practical applications.
No, the GCF is never zero.
The LCM of 18 and 30 is 90.
By dividing both numbers by the GCF to check if the quotients are integers.
Yes, if one number is a multiple of the other. For 18 and 30, the GCF is 6.
The common factors of 18 and 30 are 1, 2, 3, and 6.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the Greatest Common Factor (GCF) of 18 and 30?
2
3
6
9
Which number is a common factor of both 18 and 30?
4
5
6
7
What is the number of factors of the GCF of 18 and 30?
2
3
4
6
Which of these numbers is the greatest common factor of 18 and 30?
2
6
3
15
What is the smallest prime factor of the GCF of 18 and 30?
2
3
5
7
Which number shares the same GCF as 18 and 30?
20 and 25
24 and 30
18 and 45
18 and 36
If the GCF of 18 and 30 is added to the number 7, what is the result?
13
14
15
16
Which of the following numbers is not divisible by the GCF of 18 and 30?
30
36
42
54
What is the product of the GCF of 18 and 30 and 3?
12
18
24
30
What is the result when the GCF of 18 and 30 is divided by 2?
2
3
6
9
Before you leave, take our quick quiz to enhance your learning!

