What is the greatest common factor (GCF) of 24 and 30?
2
4
6
8

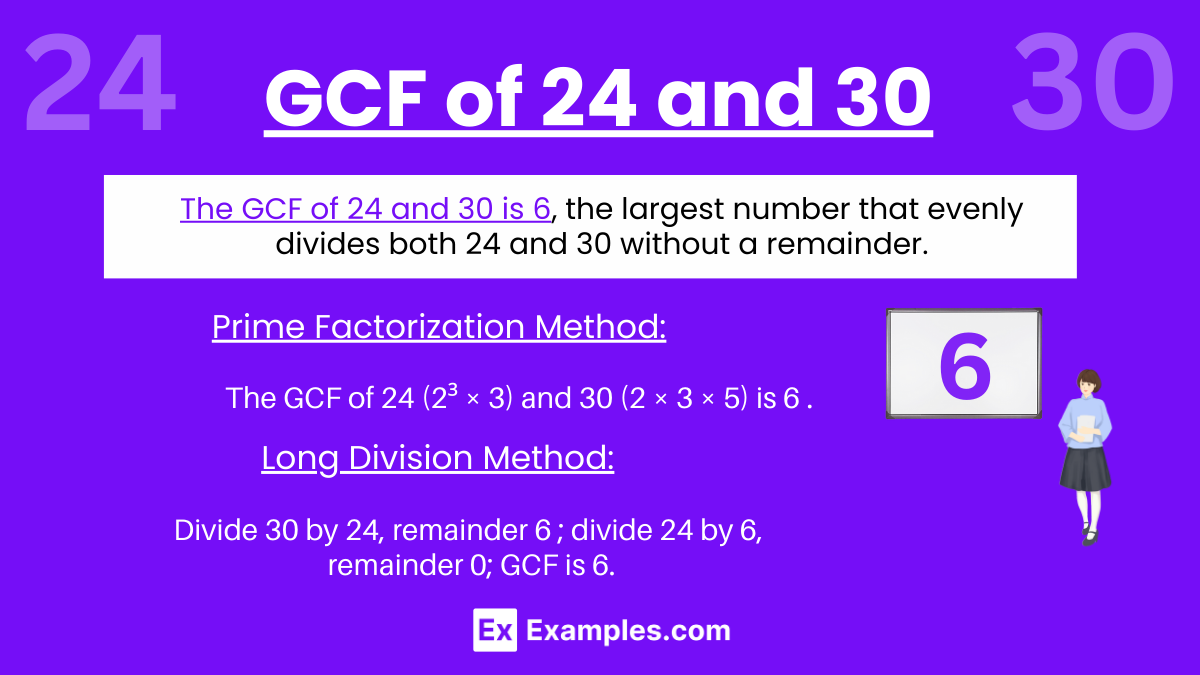
The greatest common factor (GCF) of 24 and 30 is 6. This can be efficiently determined using several straightforward methods such as listing factors, prime factorization, or the Euclidean algorithm. When listing factors, you find that the common factors of 24 (1, 2, 3, 4, 6, 8, 12, 24) and 30 (1, 2, 3, 5, 6, 10, 15, 30) are 1, 2, 3, and 6, with 6 being the largest. Prime factorization reveals that 24 is 2³ × 3 and 30 is 2 × 3 × 5, with the common prime factors being 2 and 3, which multiply to 6. The Euclidean algorithm, using division and remainders, also identifies 6 as the GCF. This value is key for simplifying fractions and other calculations involving these numbers.
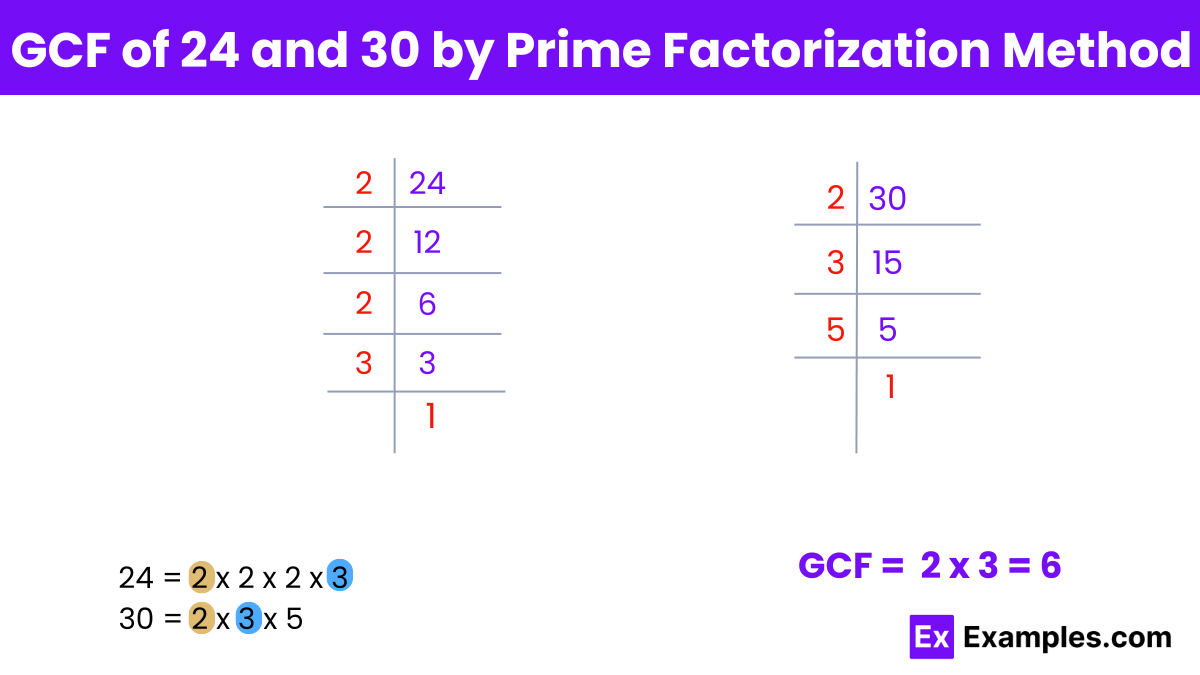
To find the greatest common factor (GCF) of 24 and 30 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 24: 24 = 2³ × 3
For 30: 30 = 2 × 3 × 5
Step 2: Identify the common prime factors and their lowest powers:
Step 3: Multiply the common prime factors with their lowest powers to determine the GCF:
GCF = 2¹ × 3¹ = 2 × 3 = 6
Therefore, the greatest common factor (GCF) of 24 and 30 by the prime factorization method is 6.
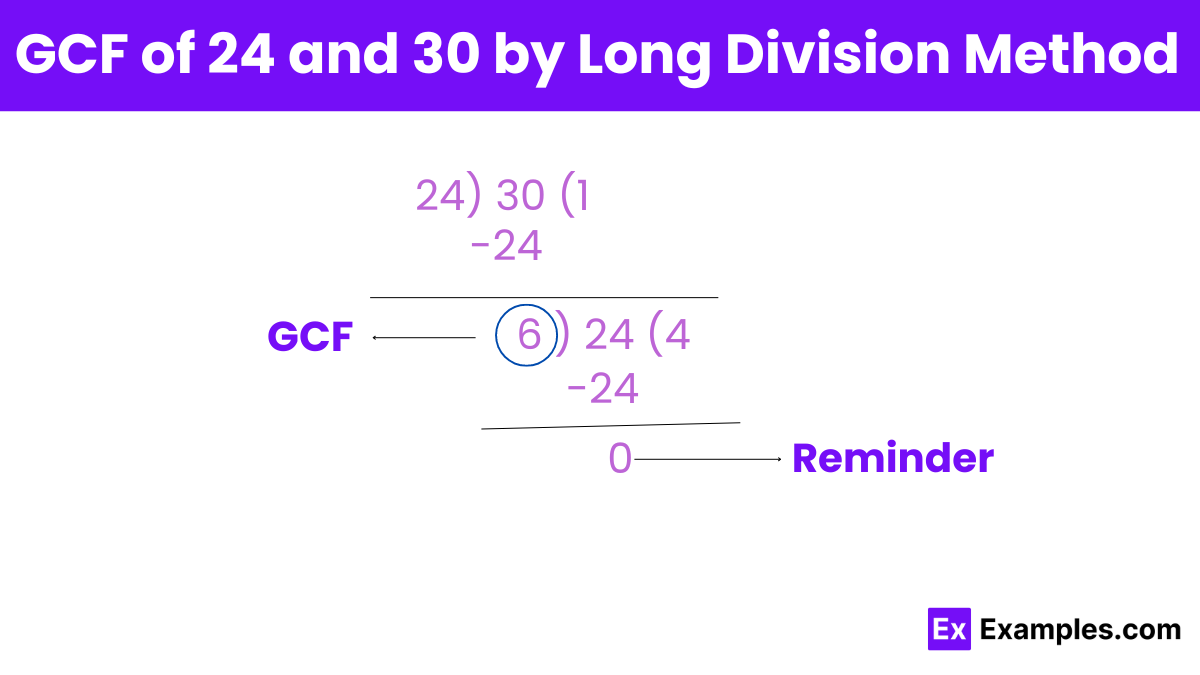
To find the greatest common factor (GCF) of 24 and 30 using the long division method:
Step 1: Start by dividing the larger number (30) by the smaller number (24).
30 ÷ 24 = 1 with a remainder of 6.
Step 2: Now take the remainder (6) and divide the previous divisor (24) by it.
24 ÷ 6 = 4 with a remainder of 0.
Since the remainder is now 0, the division process stops here.
Step 3: The divisors at this step where the remainder becomes zero is the greatest common factor (GCF).
GCF = 6.
Therefore, the greatest common factor (GCF) of 24 and 30 by the long division method is 6.
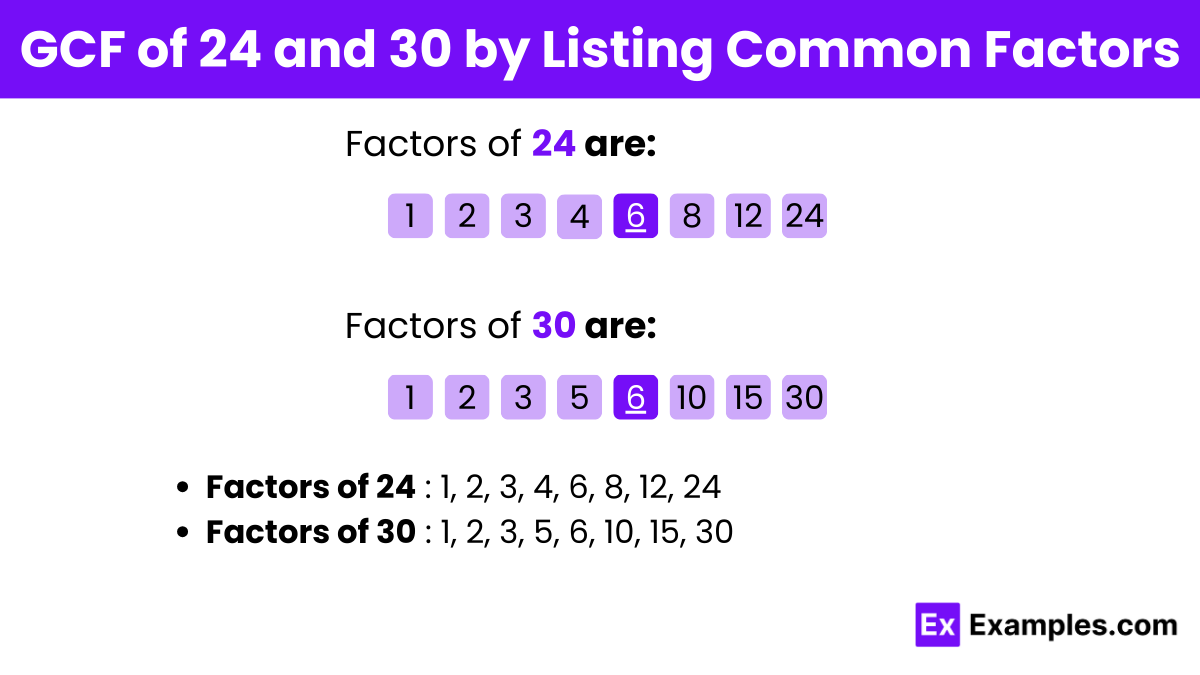
To find the greatest common factor (GCF) of 24 and 30 by listing common factors:
Step 1: List the factors of each number.
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Step 2: Identify the common factors.
Step 3: Determine the greatest common factor.
Therefore, the greatest common factor (GCF) of 24 and 30 by listing common factors is 6.
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24; factors of 30: 1, 2, 3, 5, 6, 10, 15, 30.
Listing factors is straightforward and effective for smaller numbers.
Yes, most scientific calculators and mathematical software can compute the GCF.
Confusing GCF with LCM or failing to identify all common factors.
It is crucial for simplifying algebraic expressions and solving equations.
Using visual aids like Venn diagrams, factor trees, and interactive quizzes.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the greatest common factor (GCF) of 24 and 30?
2
4
6
8
Which of the following is a common factor of both 24 and 30?
3
7
9
12
What is the GCF of 24 and 30 using prime factorization?
2
3
6
12
If the GCF of 24 and 30 is used to simplify the fraction 24/30, what is the simplified result?
2/5
3/5
4/5
5/6
Which number is not a factor of both 24 and 30?
2
4
6
12
Which method can be used to find the GCF of 24 and 30?
Subtraction method
Division method
LCM method
Addition method
What is the largest number that divides both 24 and 30 evenly?
2
4
6
8
The GCF of 24 and 30 is equal to which of the following?
The sum of the numbers
The highest common factor
The product of the numbers
The smallest common factor
If you add the GCF of 24 and 30 to 24, what is the result?
28
30
32
34
Which number is closest to the GCF of 24 and 30?
4
5
6
7
Before you leave, take our quick quiz to enhance your learning!

