What is the Greatest Common Factor (GCF) of 30 and 36?
2
3
6
9

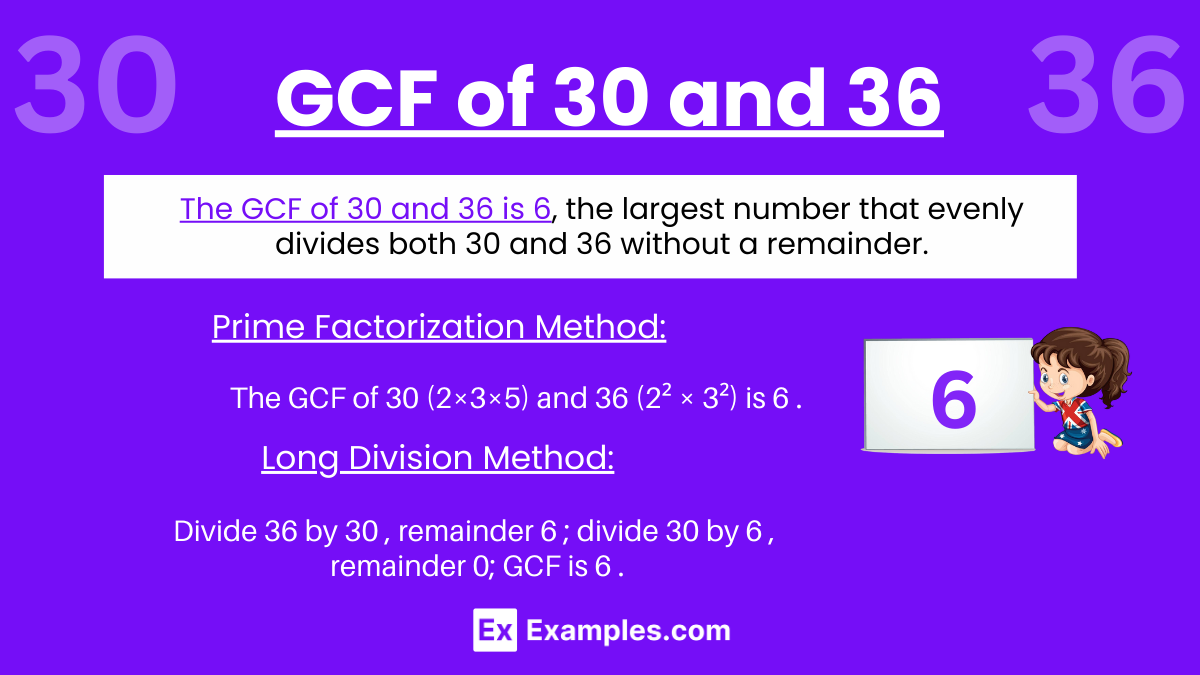
The greatest common factor (GCF) of 30 and 36 is 6. To find the GCF, one can utilize methods such as prime factorization, listing common factors, or employing the Euclidean algorithm. By analyzing the prime factorization of both numbers, it becomes evident that they share common prime factors, with the lowest power of 2 and 3 being 1 for both. Therefore, the GCF is determined as the product of these common prime factors raised to their lowest powers, resulting in a GCF of 6. This GCF represents the largest integer that divides both 30 and 36 without leaving a remainder, making it a crucial factor in simplifying fractions or finding common divisors.
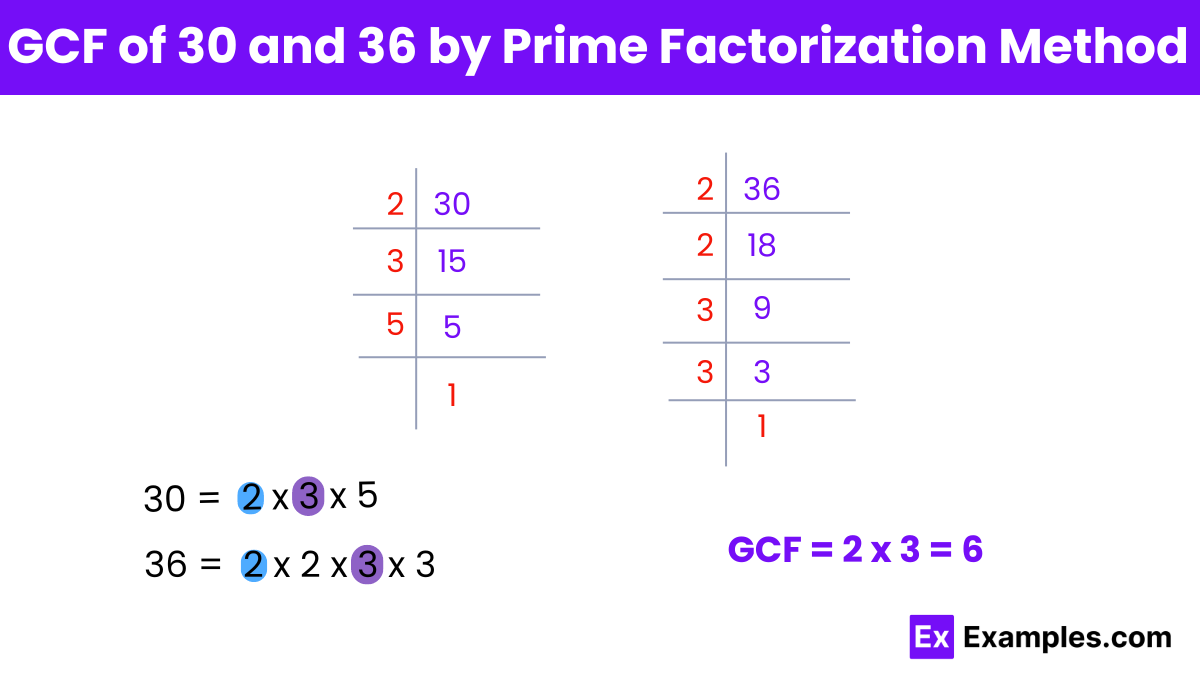
To find the greatest common factor (GCF) of 30 and 36 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 30: 30 = 2×3×5
For 36: 36 = 2² × 3²
Step 2: Identify common prime factors and their lowest powers:
Step 3: Multiply the common prime factors with their lowest powers: GCF=2¹×3¹ = 2×3 = 6
Therefore, the greatest common factor (GCF) of 30 and 36 by prime factorization method is 6.
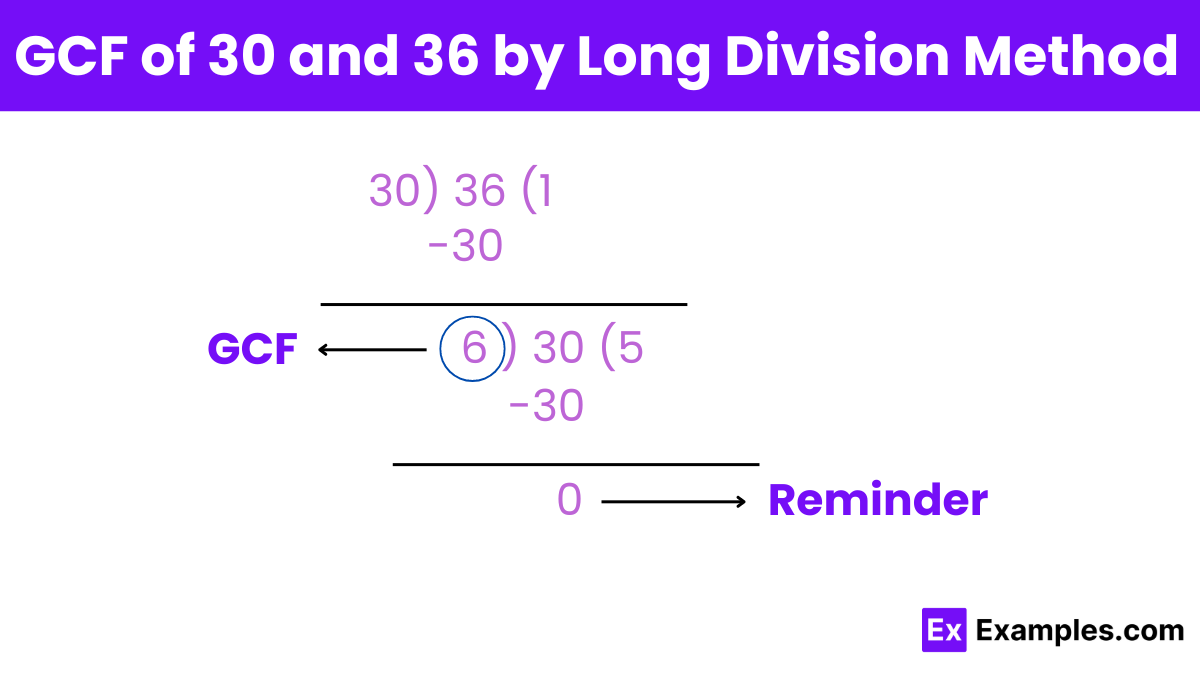
To find the greatest common factor (GCF) of 30 and 36 using the long division method:
Step 1: Start by dividing the larger number (36) by the smaller number (30).
36 ÷ 30 =1 with a remainder of 6.
Step 2: Then, take the divisor (30) and divide it by the remainder (6).
30 ÷ 6 = 5.
Step 3: Continue this process until there is no remainder.
6 ÷ 5 =1 with a remainder of 1.
5 ÷ 1 = 5.
1 ÷ 1 = 1.
Step 4: The last divisor before reaching 1 is the greatest common factor (GCF).
GCF = 6.
Therefore, the greatest common factor (GCF) of 30 and 36 by the long division method is 6.
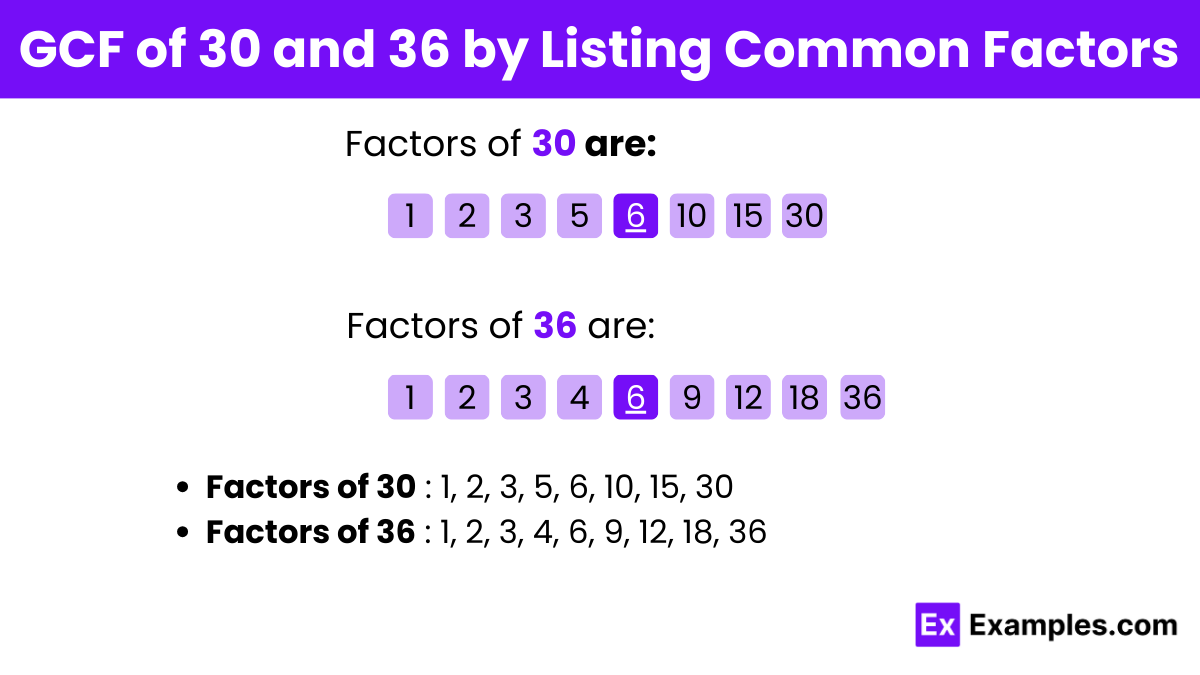
To find the greatest common factor (GCF) of 30 and 36 by listing common factors:
Step 1: List the factors of each number.
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36
Step 2: Identify the common factors. Common factors: 1, 2, 3, 6
Step 3: Determine the greatest common factor. GCF = 6.
Therefore, the greatest common factor (GCF) of 30 and 36 by listing common factors is 6.
The GCF of 30 and 36 is the greatest common divisor, as it represents the largest divisor common to both numbers.
The GCF can be used to reduce fractions to their simplest form by dividing both the numerator and denominator by the GCF.
No, the GCF cannot be larger than both numbers. It is always a factor of both numbers.
30 and 36 have four common factors: 1, 2, 3, and 6.
You can calculate the GCF of 30 and 36 using methods such as prime factorization, listing common factors, or long division.

The greatest common factor (GCF) of 30 and 36 is 6. To find the GCF, one can utilize methods such as prime factorization, listing common factors, or employing the Euclidean algorithm. By analyzing the prime factorization of both numbers, it becomes evident that they share common prime factors, with the lowest power of 2 and 3 being 1 for both. Therefore, the GCF is determined as the product of these common prime factors raised to their lowest powers, resulting in a GCF of 6. This GCF represents the largest integer that divides both 30 and 36 without leaving a remainder, making it a crucial factor in simplifying fractions or finding common divisors.
To find the greatest common factor (GCF) of 30 and 36 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 30: 30 = 2×3×5
For 36: 36 = 2² × 3²
Step 2: Identify common prime factors and their lowest powers:
Both 30 and 36 have common prime factors of 2 and 3. The lowest power of 2 is 1, and the lowest power of 3 is 1.
Step 3: Multiply the common prime factors with their lowest powers: GCF=2¹×3¹ = 2×3 = 6
Therefore, the greatest common factor (GCF) of 30 and 36 by prime factorization method is 6.
To find the greatest common factor (GCF) of 30 and 36 using the long division method:
Step 1: Start by dividing the larger number (36) by the smaller number (30).
36 ÷ 30 =1 with a remainder of 6.
Step 2: Then, take the divisor (30) and divide it by the remainder (6).
30 ÷ 6 = 5.
Step 3: Continue this process until there is no remainder.
6 ÷ 5 =1 with a remainder of 1.
5 ÷ 1 = 5.
1 ÷ 1 = 1.
Step 4: The last divisor before reaching 1 is the greatest common factor (GCF).
GCF = 6.
Therefore, the greatest common factor (GCF) of 30 and 36 by the long division method is 6.
To find the greatest common factor (GCF) of 30 and 36 by listing common factors:
Step 1: List the factors of each number.
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36
Step 2: Identify the common factors. Common factors: 1, 2, 3, 6
Step 3: Determine the greatest common factor. GCF = 6.
Therefore, the greatest common factor (GCF) of 30 and 36 by listing common factors is 6.
The GCF of 30 and 36 is the greatest common divisor, as it represents the largest divisor common to both numbers.
The GCF can be used to reduce fractions to their simplest form by dividing both the numerator and denominator by the GCF.
No, the GCF cannot be larger than both numbers. It is always a factor of both numbers.
30 and 36 have four common factors: 1, 2, 3, and 6.
You can calculate the GCF of 30 and 36 using methods such as prime factorization, listing common factors, or long division.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the Greatest Common Factor (GCF) of 30 and 36?
2
3
6
9
What is the smallest prime factor of the GCF of 30 and 36?
2
3
5
7
Which number is the largest common factor of 30 and 36?
2
3
6
12
The GCF of 30 and 36 is a multiple of which of the following numbers?
1
2
4
8
How many factors does the GCF of 30 and 36 have?
2
3
4
5
Which pair of numbers has the same GCF as 30 and 36?
18 and 24
12 and 18
24 and 30
20 and 28
What is the GCF of 30 and 36 multiplied by 3?
12
15
18
24
Which of the following numbers is not divisible by the GCF of 30 and 36?
18
24
30
42
What is the sum of the GCF of 30 and 36 and the number 10?
12
15
16
18
The GCF of 30 and 36 is what fraction of 36?
1/2
1/3
1/4
1/6
Before you leave, take our quick quiz to enhance your learning!

