Square & Square Root of 164 – Examples, Methods, Calculation
Within the realm of mathematics, particularly in algebraic studies, the foundational principles of squares and square roots are of utmost significance. Squaring a number, as illustrated by multiplying a number, such as 164, by itself to produce a result of 26896, represents a fundamental operation. This operation forms a cornerstone in exploring the properties intrinsic to both rational numbers (those expressible as a fraction of two integers) and irrational numbers (which resist expression as a neat fraction). An understanding of these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, contributing to a deeper insight into the intricate fabric of mathematical theory.
Square of 164
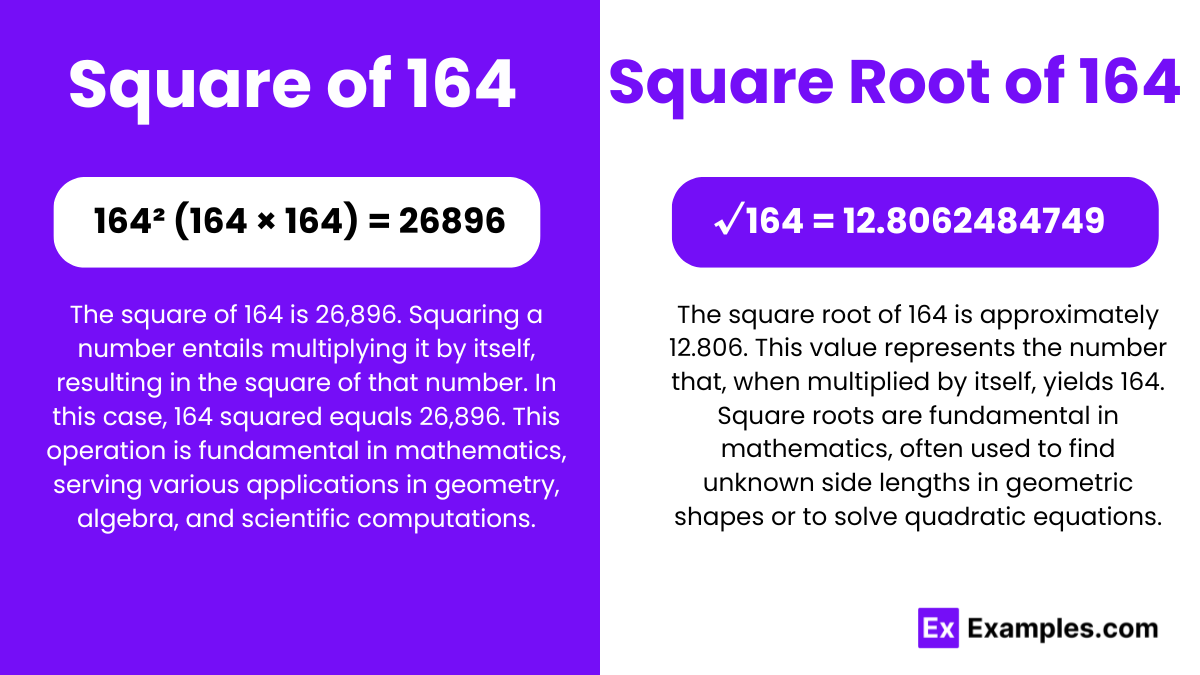
164² (164 × 164) = 26896
The square number of 164 is 26,896. A square number is obtained by multiplying a number by itself. In this case, 164 multiplied by 164 equals 26,896. Square numbers hold significance in algebraic studies, showcasing fundamental operations and laying the groundwork for exploring mathematical relationships and patterns.
Square root of 164
√164 = 12.8062484749
Or
√164 = 12.806 Upto 3 decimals
The square root of 164, an irrational number, is approximately 12.806248474865697. It represents the number that, when multiplied by itself, equals 164. Understanding square roots illuminates mathematical principles, offering insights into numerical relationships and facilitating problem-solving across various disciplines.
Square Root of 164: 12.8062484749
Exponential Form: 164^½ or 164^0.5
Radical Form: √164
Is the Square Root of 164 Rational or Irrational?
The square root of 164 is an irrational number
The square root of 164 is irrational. It cannot be expressed as a simple fraction of two integers. Instead, it’s a non-repeating, non-terminating decimal. This classification distinguishes it from rational numbers, which can be represented as fractions of integers.
Rational Number:
A rational number can be represented as a fraction of two integers, denoted as a/b, where the denominator isn’t zero. Examples include positive, negative, or zero values, such as 3/4, -5/2, 0, 1, -2, etc.
Example: Consider 3/4; since both 3 and 4 are integers, and the denominator isn’t zero, 3/4 is rational.
Irrational Number:
An irrational number, like √2 or π, cannot be expressed as a fraction of two integers. Its decimal expansion neither ends nor repeats, thus it cannot be represented in the form a/b.
Example: √2 has a non-repeating, non-terminating decimal expansion (√2 ≈ 1.41421356…), making it irrational.
Methods to Find Value of Root 164
To find the value of √164, several methods are available:
Prime Factorization: Break down 164 into prime factors (2² × 41) and simplify.
Approximation: Use estimation techniques or a calculator to approximate the square root.
Newton’s Method: Iterative algorithm for finding successively better approximations.
Babylonian Method: Iterative algorithm based on averages for approximation.
Using a Calculator: Directly input 164 and find its square root.
Square Root of 164 by Long Division Method
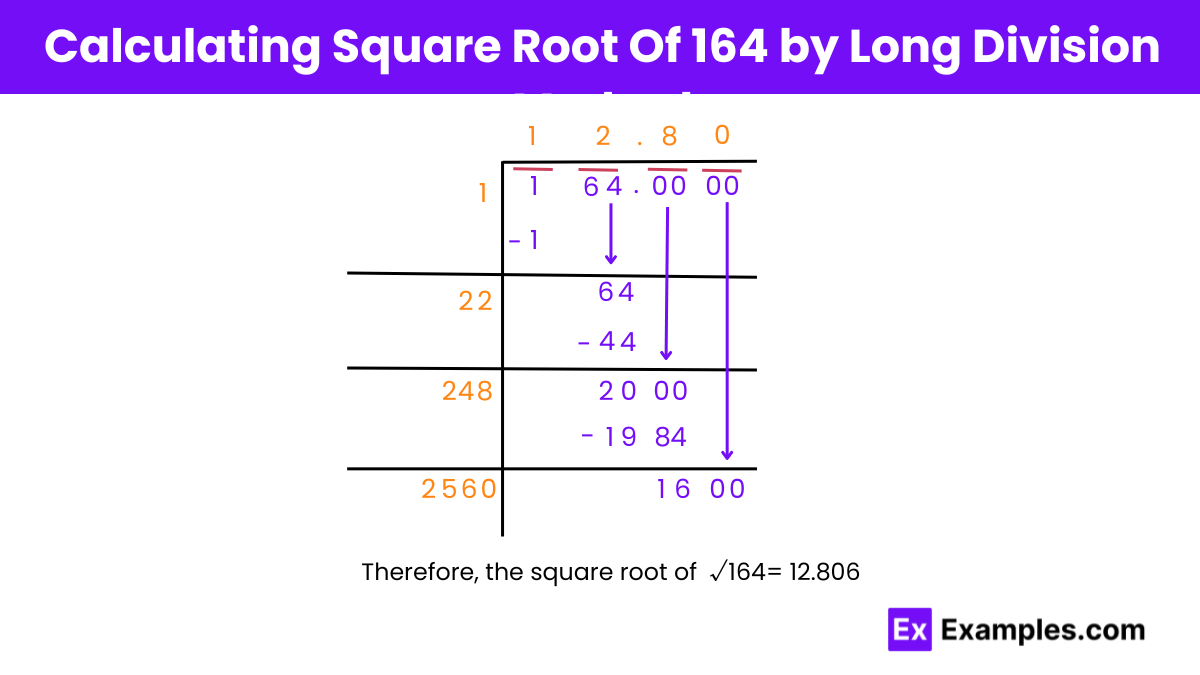
Long Division Method for Finding the Square Root of 164
Step 1: Pairing the Digits
- Group the digits of 164, placing a bar above 64 and 1 separately.
- Group the decimal 0s in pairs of 2 from left to right.
Step 2: Initial Division
- Identify the largest number that, when squared, is less than or equal to 1.
- Place this number (1) as the quotient and divisor, resulting in a remainder of 0.
Step 3: Continuing the Division
- Bring down the next pair of digits (64) beside the remainder.
- Double the divisor (1 + 1 = 2) and write it below.
Step 4: Iterative Division
- Determine a number (X) such that 2X × X is less than or equal to 64.
- The number 2 fits this criterion, so place it next to 2 in the divisor and in the quotient.
Step 5: Updating the Divisor
- Update the divisor by adding X to itself, resulting in a new divisor of 24.
Step 6: Iterative Process
- Repeat the process to obtain additional decimal places as needed.
164 is Perfect Square root or Not
No, 164 is not a perfect square number
No, 164 is not a perfect square because it does not have an integer square root. The square root of 164 is an irrational number, approximately 12.806, meaning it cannot be expressed as a simple fraction and has a non-repeating, non-terminating decimal expansion.
FAQs
Can the square root of 164 be simplified?
No, the square root of 164 cannot be simplified further since it is an irrational number.
What is the square of 164 expressed in exponential form?
The square of 164 can be expressed as 164^2.
How does the square of 164 compare to other squares?
The square of 164 is larger than the squares of numbers less than 164 and smaller than the squares of numbers greater than 164.
What is the square root of 164 rounded to the nearest integer?
The square root of 164 rounded to the nearest integer is 13.
How many digits are there in the square root of 164?
The square root of 164 has an infinite number of digits after the decimal point.
What are some applications of the square root of 164 in real life?
The square root of 164 may be used in fields such as engineering, physics, and finance for various calculations and measurements.
Square & Square Root of 164 – Examples, Methods, Calculation
Within the realm of mathematics, particularly in algebraic studies, the foundational principles of squares and square roots are of utmost significance. Squaring a number, as illustrated by multiplying a number, such as 164, by itself to produce a result of 26896, represents a fundamental operation. This operation forms a cornerstone in exploring the properties intrinsic to both rational numbers (those expressible as a fraction of two integers) and irrational numbers (which resist expression as a neat fraction). An understanding of these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, contributing to a deeper insight into the intricate fabric of mathematical theory.
Square of 164
164² (164 × 164) = 26896
The square number of 164 is 26,896. A square number is obtained by multiplying a number by itself. In this case, 164 multiplied by 164 equals 26,896. Square numbers hold significance in algebraic studies, showcasing fundamental operations and laying the groundwork for exploring mathematical relationships and patterns.
Square root of 164
√164 = 12.8062484749
Or
√164 = 12.806 Upto 3 decimals
The square root of 164, an irrational number, is approximately 12.806248474865697. It represents the number that, when multiplied by itself, equals 164. Understanding square roots illuminates mathematical principles, offering insights into numerical relationships and facilitating problem-solving across various disciplines.
Square Root of 164: 12.8062484749
Exponential Form: 164^½ or 164^0.5
Radical Form: √164
Is the Square Root of 164 Rational or Irrational?
The square root of 164 is an irrational number
The square root of 164 is irrational. It cannot be expressed as a simple fraction of two integers. Instead, it’s a non-repeating, non-terminating decimal. This classification distinguishes it from rational numbers, which can be represented as fractions of integers.
Rational Number:
A rational number can be represented as a fraction of two integers, denoted as a/b, where the denominator isn’t zero. Examples include positive, negative, or zero values, such as 3/4, -5/2, 0, 1, -2, etc.
Example: Consider 3/4; since both 3 and 4 are integers, and the denominator isn’t zero, 3/4 is rational.
Irrational Number:
An irrational number, like √2 or π, cannot be expressed as a fraction of two integers. Its decimal expansion neither ends nor repeats, thus it cannot be represented in the form a/b.
Example: √2 has a non-repeating, non-terminating decimal expansion (√2 ≈ 1.41421356…), making it irrational.
Methods to Find Value of Root 164
To find the value of √164, several methods are available:
Prime Factorization: Break down 164 into prime factors (2² × 41) and simplify.
Approximation: Use estimation techniques or a calculator to approximate the square root.
Newton’s Method: Iterative algorithm for finding successively better approximations.
Babylonian Method: Iterative algorithm based on averages for approximation.
Using a Calculator: Directly input 164 and find its square root.
Square Root of 164 by Long Division Method

Long Division Method for Finding the Square Root of 164
Step 1: Pairing the Digits
Group the digits of 164, placing a bar above 64 and 1 separately.
Group the decimal 0s in pairs of 2 from left to right.
Step 2: Initial Division
Identify the largest number that, when squared, is less than or equal to 1.
Place this number (1) as the quotient and divisor, resulting in a remainder of 0.
Step 3: Continuing the Division
Bring down the next pair of digits (64) beside the remainder.
Double the divisor (1 + 1 = 2) and write it below.
Step 4: Iterative Division
Determine a number (X) such that 2X × X is less than or equal to 64.
The number 2 fits this criterion, so place it next to 2 in the divisor and in the quotient.
Step 5: Updating the Divisor
Update the divisor by adding X to itself, resulting in a new divisor of 24.
Step 6: Iterative Process
Repeat the process to obtain additional decimal places as needed.
164 is Perfect Square root or Not
No, 164 is not a perfect square number
No, 164 is not a perfect square because it does not have an integer square root. The square root of 164 is an irrational number, approximately 12.806, meaning it cannot be expressed as a simple fraction and has a non-repeating, non-terminating decimal expansion.
FAQs
Can the square root of 164 be simplified?
No, the square root of 164 cannot be simplified further since it is an irrational number.
What is the square of 164 expressed in exponential form?
The square of 164 can be expressed as 164^2.
How does the square of 164 compare to other squares?
The square of 164 is larger than the squares of numbers less than 164 and smaller than the squares of numbers greater than 164.
What is the square root of 164 rounded to the nearest integer?
The square root of 164 rounded to the nearest integer is 13.
How many digits are there in the square root of 164?
The square root of 164 has an infinite number of digits after the decimal point.
What are some applications of the square root of 164 in real life?
The square root of 164 may be used in fields such as engineering, physics, and finance for various calculations and measurements.


