The De Broglie Hypothesis is a fundamental concept in proposed by the French physicist Louis de Broglie in 1924. This groundbreaking idea introduced the wave-particle duality of matter, suggesting that not only light (previously understood to exhibit both wave-like and particle-like properties) but all forms of matter have wave-like characteristics.
Louis de Broglie hypothesized that if light can display dual characteristics (both wave-like and particle-like properties), then particles, such as electrons, might also exhibit similar dual characteristics. His derivation was based on the parallels between the equations for energy and momentum in both light and material particles.
For photons (light particles), the energy (𝐸E) and momentum (𝑝p) are related by the equations:
Here, ℎh is Planck’s constant, 𝑓f is the frequency of the photon, and 𝑐c is the speed of light. By substituting the energy equation into the momentum equation, we get:
Since the wavelength (λ) of a photon is related to its frequency by 𝑐 = 𝜆𝑓, we can rewrite 𝑓 as:
Substituting back, the momentum of a photon can be expressed as:
De Broglie proposed that if light (which was known to have wave-like properties) has a wavelength given 𝜆 = ℎ/𝑝, then particles, such as electrons, should also have a wavelength describable by a similar relationship, even though they have mass. Thus, he extended the equation to all matter, proposing that:
where p is now the momentum of the particle, which for a non-relativistic particle is given by:
Here, m is the mass of the particle and v is its velocity.
Combining the expressions, the de Broglie wavelength for any particle is thus given by:
This equation implies that every moving particle has a wave associated with it, and the wavelength of that wave is inversely proportional to the particle’s momentum. This groundbreaking idea led to the development of wave mechanics and has been fundamental in many areas of quantum physics, such as the theory behind quantum fields and elementary particles.
To calculate the De Broglie wavelength of an electron, we use the formula derived by Louis de Broglie which relates a particle’s wavelength to its momentum. The formula is:
where:
The momentum 𝑝p of an electron can be calculated using the formula: 𝑝=𝑚𝑣p=mv where:
The De Broglie equation, 𝜆 = ℎ/𝑝, is a cornerstone in quantum mechanics, providing a profound understanding of the wave-particle duality of matter. Its implications extend far beyond theoretical physics, impacting various scientific fields and technologies.
The equation integrates wave-like behavior into the description of elementary particles, bridging a gap between classical and quantum physics. This wave-particle duality is essential for the development of quantum mechanics, influencing the theoretical framework that describes how subatomic particles behave.
De Broglie’s insights laid the groundwork for Schrödinger to formulate his wave equation, which uses the concept of wavefunctions to describe the statistical behavior of systems. The wave-particle duality concept is integral to quantum field theory, which extends quantum mechanics to more complex systems including fields and forces.
The equation has been empirically validated through experiments such as electron diffraction and neutron diffraction, which demonstrate that particles exhibit wave-like behavior under certain conditions. These experiments are pivotal for technologies such as electron microscopes, which rely on electron waves to achieve high-resolution imaging beyond the capability of traditional optical microscopes.
Understanding the wave properties of particles enables the exploitation of phenomena such as quantum tunneling, utilized in devices like tunnel diodes and the scanning tunneling microscope. These applications are crucial in electronics and materials science, where quantum effects are significant.
The De Broglie equation has also profoundly impacted educational approaches in physics, providing a fundamental concept that challenges and expands our understanding of the natural world. It encourages a more nuanced view of matter, essential for students and researchers delving into quantum physics.
Louis de Broglie introduced his theory of electron waves in 1924, which proposed that particles could exhibit properties of waves. His famous equation relates the wavelength of a particle to its momentum: 𝜆 = ℎ/𝑝 where 𝜆is the wavelength, ℎ is Planck’s constant, and 𝑝p is the momentum of the particle.
Niels Bohr proposed his model of the atom in 1913. His key hypothesis was that electrons orbit the nucleus in distinct orbits without radiating energy, contrary to what classical electromagnetism would predict. To explain the stability of these orbits, Bohr introduced the concept of quantization:
De Broglie’s theory was revolutionary because it provided a theoretical justification for Bohr’s quantization condition by interpreting the electron not just as a particle, but as a wave that must form a standing wave pattern around the nucleus. For the electron wave to be stable and not interfere destructively with itself, the circumference of the electron’s orbit must be an integer multiple of its wavelength:
where 𝑟 is the radius of the electron’s orbit, and n is an integer. This condition ensures that the wave ‘fits’ perfectly into its orbital path around the nucleus.
By substituting De Broglie’s expression for the wavelength into the condition for a stable orbit, we get:
Using the expression for momentum 𝑝=𝑚𝑣p=mv and the definition of angular momentum 𝐿=𝑚𝑣𝑟L=mvr, we can relate this to Bohr’s quantization of angular momentum:
Thus, De Broglie’s hypothesis not only supported Bohr’s model but also suggested a deeper wave nature of the electron. It bridged the gap between the quantized orbits of Bohr’s atom model and the wave-like behavior of particles, paving the way for modern quantum mechanics, which would further refine and expand these ideas in the Schrodinger equation and beyond.
One of the first confirmations of De Broglie’s hypothesis was the observation of electron diffraction patterns. When electrons are passed through thin metal foils or across a crystal, they produce diffraction patterns similar to those produced by light waves, confirming that electrons behave as waves under certain conditions.
The scanning tunneling microscope, which can image surfaces at the atomic level, operates based on the quantum tunneling of electrons between the microscope’s tip and the surface. The wave nature of electrons, as predicted by De Broglie, is fundamental to the operation of this instrument.
De Broglie’s ideas extended the Bohr model by providing a theoretical basis for the quantization of electron orbits in atoms. His hypothesis suggested that electrons form standing wave patterns around the nucleus, which only occur at certain discrete (quantized) orbits.
The concept of matter waves is essential in fields like quantum mechanics and has led to further developments in wave mechanics. This includes the use of neutrons, atoms, and molecules in wave-like applications, similar to how light and electrons are used.
Neutron beams, used in neutron interferometry, exhibit wave-like interference effects. These experiments have provided precise measurements of neutron properties and fundamental quantum phenomena, supporting De Broglie’s hypothesis at larger scales.
AFM, like STM, uses the principles of quantum mechanics and the wave-like properties of atoms on a surface to achieve high-resolution imaging. The forces between the tip’s atoms and the sample’s atoms are influenced by their wave functions.
Louis de Broglie proposed that particles of matter, like electrons, could exhibit wave-like properties similar to light. Combining Einstein’s equation relating energy and mass (𝐸 = 𝑚𝑐²) with Planck’s equation relating energy and frequency (𝐸 = ℎ𝑓), and considering the wave equation (𝑐 = 𝑓𝜆), De Broglie derived his hypothesis that matter behaves as waves.
The De Broglie Equation is crucial for understanding quantum mechanics as it introduces the concept of wave-particle duality. This concept states that every particle or quantum entity can exhibit both particle-like and wave-like behavior. It forms the basis for the development of quantum theory, particularly in the formulation of wave mechanics.
While theoretically applicable to all matter, in practice, the wave-like properties described by the De Broglie Equation are significant only for very small objects, like subatomic particles. For larger objects, the wavelengths calculated by the equation become so small that they are not detectable with current technology.
Wave-particle duality is a fundamental concept of quantum mechanics that suggests that every particle or quantum entity may be partly described in terms not only of particles, but also of waves. It means that elementary particles such as electrons and photons exhibit both particle-like and wave-like properties, depending on the experimental setup.
The De Broglie Hypothesis is a fundamental concept in proposed by the French physicist Louis de Broglie in 1924. This groundbreaking idea introduced the wave-particle duality of matter, suggesting that not only light (previously understood to exhibit both wave-like and particle-like properties) but all forms of matter have wave-like characteristics.
The De Broglie Hypothesis proposes that all matter exhibits wave-like properties, extending wave-particle duality beyond light to include electrons and other particles. It quantifies this wave behavior with the equation 𝜆 = ℎ𝑝 , where λ is wavelength, h is Planck’s constant, and p is momentum.
Louis de Broglie hypothesized that if light can display dual characteristics (both wave-like and particle-like properties), then particles, such as electrons, might also exhibit similar dual characteristics. His derivation was based on the parallels between the equations for energy and momentum in both light and material particles.
For photons (light particles), the energy (𝐸E) and momentum (𝑝p) are related by the equations:
𝐸 = ℎ𝑓
p = E/c
Here, ℎh is Planck’s constant, 𝑓f is the frequency of the photon, and 𝑐c is the speed of light. By substituting the energy equation into the momentum equation, we get:
p = hf/c
Since the wavelength (λ) of a photon is related to its frequency by 𝑐 = 𝜆𝑓, we can rewrite 𝑓 as:
𝑓 = 𝑐/𝜆
Substituting back, the momentum of a photon can be expressed as:
p = h/λ
De Broglie proposed that if light (which was known to have wave-like properties) has a wavelength given 𝜆 = ℎ/𝑝, then particles, such as electrons, should also have a wavelength describable by a similar relationship, even though they have mass. Thus, he extended the equation to all matter, proposing that:
𝜆 = ℎ/𝑝
where p is now the momentum of the particle, which for a non-relativistic particle is given by:
p = mv
Here, m is the mass of the particle and v is its velocity.
Combining the expressions, the de Broglie wavelength for any particle is thus given by:
𝜆 = ℎ/𝑚𝑣
This equation implies that every moving particle has a wave associated with it, and the wavelength of that wave is inversely proportional to the particle’s momentum. This groundbreaking idea led to the development of wave mechanics and has been fundamental in many areas of quantum physics, such as the theory behind quantum fields and elementary particles.
To calculate the De Broglie wavelength of an electron, we use the formula derived by Louis de Broglie which relates a particle’s wavelength to its momentum. The formula is:
𝜆 = ℎ/𝑝
where:
𝜆 is the wavelength,
ℎ is Planck’s constant, approximately 6.626×10⁻³⁴ Joule seconds,
𝑝 is the momentum of the electron.
The momentum 𝑝p of an electron can be calculated using the formula: 𝑝=𝑚𝑣p=mv where:
𝑚 is the mass of the electron, approximately 9.109×10⁻³¹ kg,
𝑣 is the velocity of the electron.
The De Broglie equation, 𝜆 = ℎ/𝑝, is a cornerstone in quantum mechanics, providing a profound understanding of the wave-particle duality of matter. Its implications extend far beyond theoretical physics, impacting various scientific fields and technologies.
The equation integrates wave-like behavior into the description of elementary particles, bridging a gap between classical and quantum physics. This wave-particle duality is essential for the development of quantum mechanics, influencing the theoretical framework that describes how subatomic particles behave.
De Broglie’s insights laid the groundwork for Schrödinger to formulate his wave equation, which uses the concept of wavefunctions to describe the statistical behavior of systems. The wave-particle duality concept is integral to quantum field theory, which extends quantum mechanics to more complex systems including fields and forces.
The equation has been empirically validated through experiments such as electron diffraction and neutron diffraction, which demonstrate that particles exhibit wave-like behavior under certain conditions. These experiments are pivotal for technologies such as electron microscopes, which rely on electron waves to achieve high-resolution imaging beyond the capability of traditional optical microscopes.
Understanding the wave properties of particles enables the exploitation of phenomena such as quantum tunneling, utilized in devices like tunnel diodes and the scanning tunneling microscope. These applications are crucial in electronics and materials science, where quantum effects are significant.
The De Broglie equation has also profoundly impacted educational approaches in physics, providing a fundamental concept that challenges and expands our understanding of the natural world. It encourages a more nuanced view of matter, essential for students and researchers delving into quantum physics.
Louis de Broglie introduced his theory of electron waves in 1924, which proposed that particles could exhibit properties of waves. His famous equation relates the wavelength of a particle to its momentum: 𝜆 = ℎ/𝑝 where 𝜆is the wavelength, ℎ is Planck’s constant, and 𝑝p is the momentum of the particle.
Niels Bohr proposed his model of the atom in 1913. His key hypothesis was that electrons orbit the nucleus in distinct orbits without radiating energy, contrary to what classical electromagnetism would predict. To explain the stability of these orbits, Bohr introduced the concept of quantization:
Electrons can only occupy certain allowed orbits.
The angular momentum of electrons in these orbits is quantized, specifically, it is an integer multiple of the reduced Planck constant
(ℏ): 𝐿 = 𝑛×ℎ/2𝜋 = 𝑛ℏ
where 𝐿L is the angular momentum, n is a positive integer (quantum number), and h is Planck’s constant.
De Broglie’s theory was revolutionary because it provided a theoretical justification for Bohr’s quantization condition by interpreting the electron not just as a particle, but as a wave that must form a standing wave pattern around the nucleus. For the electron wave to be stable and not interfere destructively with itself, the circumference of the electron’s orbit must be an integer multiple of its wavelength:
2𝜋𝑟 = 𝑛𝜆
where 𝑟 is the radius of the electron’s orbit, and n is an integer. This condition ensures that the wave ‘fits’ perfectly into its orbital path around the nucleus.
By substituting De Broglie’s expression for the wavelength into the condition for a stable orbit, we get:
2πr=n×h/p
Using the expression for momentum 𝑝=𝑚𝑣p=mv and the definition of angular momentum 𝐿=𝑚𝑣𝑟L=mvr, we can relate this to Bohr’s quantization of angular momentum:
𝑚𝑣𝑟 = 𝑛×ℎ/2𝜋
Thus, De Broglie’s hypothesis not only supported Bohr’s model but also suggested a deeper wave nature of the electron. It bridged the gap between the quantized orbits of Bohr’s atom model and the wave-like behavior of particles, paving the way for modern quantum mechanics, which would further refine and expand these ideas in the Schrodinger equation and beyond.
One of the first confirmations of De Broglie’s hypothesis was the observation of electron diffraction patterns. When electrons are passed through thin metal foils or across a crystal, they produce diffraction patterns similar to those produced by light waves, confirming that electrons behave as waves under certain conditions.
The scanning tunneling microscope, which can image surfaces at the atomic level, operates based on the quantum tunneling of electrons between the microscope’s tip and the surface. The wave nature of electrons, as predicted by De Broglie, is fundamental to the operation of this instrument.
De Broglie’s ideas extended the Bohr model by providing a theoretical basis for the quantization of electron orbits in atoms. His hypothesis suggested that electrons form standing wave patterns around the nucleus, which only occur at certain discrete (quantized) orbits.
The concept of matter waves is essential in fields like quantum mechanics and has led to further developments in wave mechanics. This includes the use of neutrons, atoms, and molecules in wave-like applications, similar to how light and electrons are used.
Neutron beams, used in neutron interferometry, exhibit wave-like interference effects. These experiments have provided precise measurements of neutron properties and fundamental quantum phenomena, supporting De Broglie’s hypothesis at larger scales.
AFM, like STM, uses the principles of quantum mechanics and the wave-like properties of atoms on a surface to achieve high-resolution imaging. The forces between the tip’s atoms and the sample’s atoms are influenced by their wave functions.
Louis de Broglie proposed that particles of matter, like electrons, could exhibit wave-like properties similar to light. Combining Einstein’s equation relating energy and mass (𝐸 = 𝑚𝑐²) with Planck’s equation relating energy and frequency (𝐸 = ℎ𝑓), and considering the wave equation (𝑐 = 𝑓𝜆), De Broglie derived his hypothesis that matter behaves as waves.
The De Broglie Equation is crucial for understanding quantum mechanics as it introduces the concept of wave-particle duality. This concept states that every particle or quantum entity can exhibit both particle-like and wave-like behavior. It forms the basis for the development of quantum theory, particularly in the formulation of wave mechanics.
While theoretically applicable to all matter, in practice, the wave-like properties described by the De Broglie Equation are significant only for very small objects, like subatomic particles. For larger objects, the wavelengths calculated by the equation become so small that they are not detectable with current technology.
Wave-particle duality is a fundamental concept of quantum mechanics that suggests that every particle or quantum entity may be partly described in terms not only of particles, but also of waves. It means that elementary particles such as electrons and photons exhibit both particle-like and wave-like properties, depending on the experimental setup.
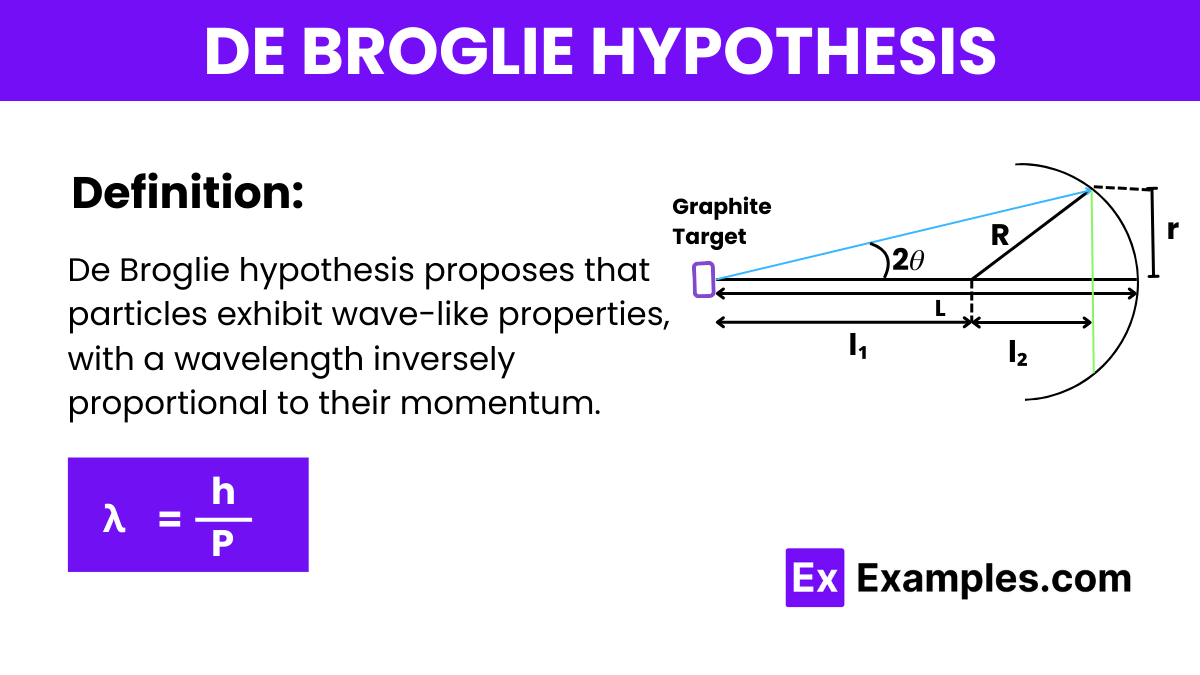
Which of the following statements best describes the de Broglie hypothesis?
Particles exhibit only wave-like properties.
Particles exhibit only particle-like properties.
Particles exhibit both wave-like and particle-like properties.
Particles exhibit neither wave-like nor particle-like properties.
According to de Broglie, what is the wavelength associated with a particle of mass mm and velocity v?
(\lambda = \frac{h}{mv}\)
\lambda = \frac{mv}{h}
\lambda = hmv
\lambda = \frac{h}{mv^2}
For which of the following particles is the de Broglie wavelength the longest, assuming all have the same kinetic energy?
Electron
Proton
Neutron
Alpha particle
The de Broglie wavelength of a moving particle is directly proportional to:
Its mass
Its velocity
Its momentum
Planck’s constant
Which of the following experiments confirmed the wave nature of electrons as proposed by de Broglie?
Michelson-Morley experiment
Rutherford scattering experiment
Davisson-Germer experiment
Stern-Gerlach experiment
If the velocity of a particle is doubled, what happens to its de Broglie wavelength?
It remains the same.
It doubles.
It halves.
It quadruples.
Which of the following is true regarding the de Broglie wavelength of macroscopic objects?
It is significant and measurable.
It is significant but not measurable.
It is negligible and not measurable.
It is negligible but measurable.
The de Broglie hypothesis applies to:
Only photons
Only electrons
Only particles with mass
All particles
In the context of de Broglie waves, what does the term "wave-particle duality" refer to?
Waves can be converted into particles.
Particles can be converted into waves.
Particles exhibit both wave and particle properties.
Waves and particles are two separate phenomena.
Calculate the de Broglie wavelength of an electron moving with a velocity of 2 × 10⁶ m/s. (Use h = 6.63×10⁻³⁴ Js, mass of electron m=9.11×10⁻³¹ kg)
m
2.73×10⁻¹⁰
m
m