Ficks Law of Diffusion

Fick’s Law of Diffusion is a key concept in the field of physics, particularly in the study of how particles such as atoms, ions, or molecules move from areas of higher concentration to areas of lower concentration. This movement is driven by a gradient in concentration, and the law quantifies this diffusion process, which is essential in understanding various natural and engineered systems.

What is Ficks Law of Diffusion?

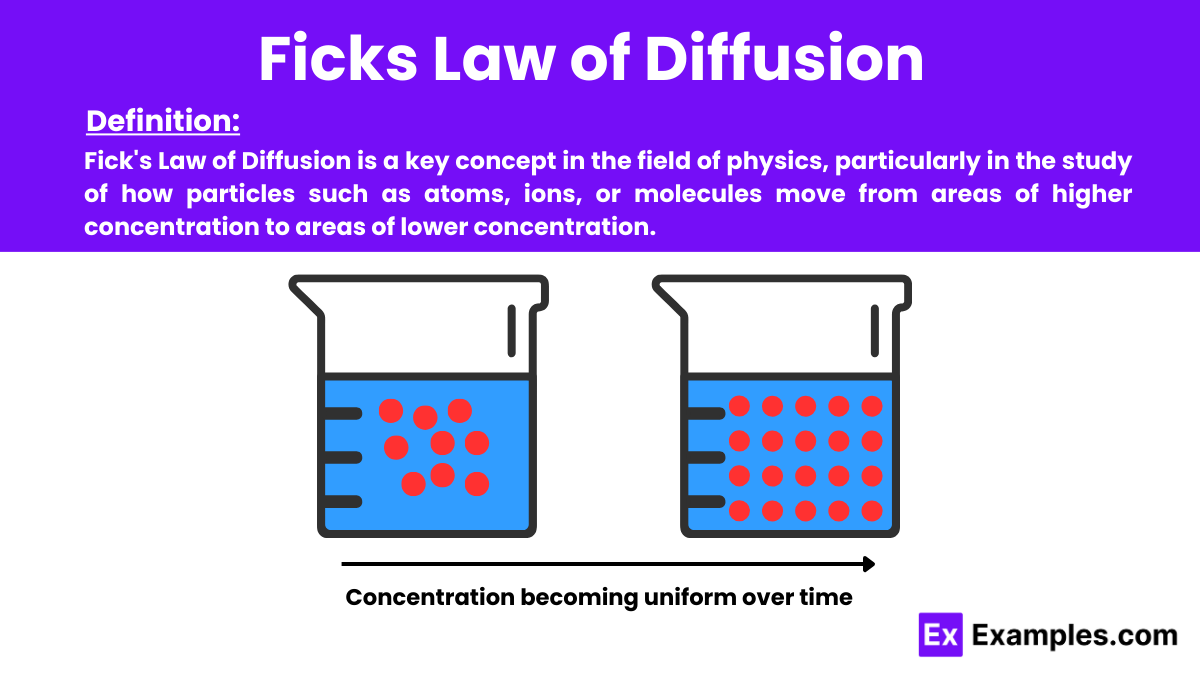
Fick’s Law of Diffusion is an essential principle in the field of physics, particularly in studying how particles move from regions of higher concentration to regions of lower concentration across a gradient. This law provides a mathematical framework. For predicting and understanding the rate and direction of diffusion in various materials and environments.

Fick’s First Law

Fick’s First Law quantifies the rate at which particles diffuse across a medium, providing a foundational concept in the study of diffusion processes. It essentially states that the diffusion flux is directly proportional to, and flows in the direction opposite to, the concentration gradient.

The law defines diffusion flux, 𝐽, as the amount of substance that passes through a unit area per unit time. It indicates that this flux occurs from regions of higher concentration to regions of lower concentration.

Mathematically, Fick’s First Law is:

𝐽=−𝐷∇𝐶

• J is the diffusion flux,
• 𝐷 is the diffusion coefficient, which measures the diffusivity of the substance,
• ∇𝐶 represents the gradient in concentration, and the negative sign signifies that diffusion occurs in the direction that decreases concentration.

Fick’s Second Law

Fick’s Second Law states that the rate of change of concentration with respect to time is proportional to the spatial derivative of the concentration gradient. This indicates that diffusion causes the concentration distribution to evolve, seeking equilibrium across the medium.

The mathematical expression of Fick’s Second Law is:

∂𝐶/∂𝑡=𝐷∂²𝐶/∂𝑥²

• ∂𝐶/∂𝑡​ is the partial derivative of concentration with respect to time, showing how concentration changes over time.
• 𝐷 is the diffusion coefficient, representing the diffusivity of the substance.
• ∂²𝐶/∂𝑥² is the second spatial derivative of concentration, representing the curvature of the concentration profile across space.

Importance of Ficks Law of Diffusion

Fick’s Law of Diffusion is crucial for understanding how particles move from high to low concentration areas. This simple yet powerful law is key in many fields:

• Medical Treatments: Helps design drug delivery systems that release medicine at the right rate.
• Environmental Science: Explains how pollutants spread in air and water, aiding cleanup efforts.
• Engineering: Guides the creation of materials that control moisture or gas flow, enhancing product durability and function.
• Food Industry: Assists in processes like drying or salting, ensuring product quality and safety.

Overall, Fick’s Law is essential for any application where materials mix or react, providing a foundation for innovation and efficient design across various industries.

Uses of Ficks Law of Diffusion

Fick’s Law of Diffusion is instrumental in several areas, providing insights into how substances move through various mediums. Here are some key applications:

• Medical Technologies: Fick’s Law helps design controlled drug delivery systems where precise diffusion rates are crucial for effective treatment.
• Environmental Engineering: Engineers use this law to model the spread of pollutants in air and water, aiding in the development of remediation strategies.
• Food Processing: In the food industry, Fick’s Law is applied to optimize processes like curing and drying, ensuring uniformity and quality.
• Material Science: It guides the development of new materials with specific diffusion properties, useful in fabricating membranes and filters.
• Chemical Engineering: Fick’s Law is essential for understanding mass transfer in processes like distillation and extraction.
• Biological Research: The law explains how nutrients and gases diffuse across cell membranes, supporting studies in physiology and health.

Examples for Ficks Law of Diffusion

• Medical Patches: In healthcare, transdermal patches deliver medication through the skin. Fick’s Law explains how the drug molecules move from the patch into the bloodstream at controlled rates, ensuring consistent medication levels.
• Preserving Food: In the food industry, salt or sugar cures food by creating a concentration gradient. Fick’s Law describes how these substances penetrate the food, storing it and increasing flavor.
• Air Quality Monitoring: Environmental scientists apply Fick’s Law to predict how pollutants disperse in the atmosphere from a source, aiding in the design of effective monitoring and control strategies.
• Battery Design: In energy technology, Fick’s Law assists in optimizing the diffusion of ions in batteries. This understanding leads to improved battery efficiency and life.
• Waste Treatment: In environmental engineering, Fick’s Law helps design systems for treating wastewater through aeration, where oxygen diffuses into water bodies, crucial for degrading pollutants.

FAQ’S

Is Fick’s law a gas law?

No, Fick’s law is not a gas law; it specifically addresses diffusion processes in liquids, gases, and solids, focusing on concentration gradients.

Does Fick’s law apply to osmosis?

Yes, Fick’s law can apply to osmosis to some extent, as it helps describe how solvent molecules move through a semipermeable membrane from lower to higher solute concentrations.

What affects Fick’s law?

Several factors impact Fick’s law: the diffusion coefficient, the concentration gradient, and the temperature. Each altering how quickly and efficiently diffusion occurs.