What is the value of the acceleration due to gravity on Earth\'s surface?
8.8 m/s²
9.8 m/s²
10.8 m/s²
11.8 m/s²

Gravitational acceleration refers to the acceleration of an object caused by the force of gravity from another object, typically a planet or a moon. The most common formula to calculate gravitational acceleration is derived from Newton’s Law of Universal Gravitation, discovered by Sir Isaac Newton in the late 17th century. Newton’s groundbreaking work established the foundational principles that describe how objects attract each other with a force proportional to their masses and inversely proportional to the square of the distance between their centers.
We express the standard formula for gravitational acceleration as:
For objects not at the Earth’s surface but at a height h above it, the formula adjusts to account for the increased distance from the Earth’s center. This variation is important for calculations involving satellites or high-altitude flights. The modified formula becomes:
As h increases, g decreases, illustrating the inverse square law inherent in gravitational interactions.
Another essential formula relates to objects in free fall near the Earth’s surface, where gravitational acceleration is approximated as a constant:
This value simplifies many physics calculations involving motion near the Earth’s surface and assumes a uniform gravitational field without significant variation in altitude or geographical location. These formulas allow physicists and engineers to calculate how quickly objects will accelerate towards each other or the ground, providing crucial data for everything from engineering to aerospace development.
Newton’s Law of Universal Gravitation states that two masses (M and m) separated by a distance (r) exert a force (F) on each other as given by: F = G x ( M × m) / r²
Newton’s second law states that force equals mass times acceleration (𝐹 = 𝑚a). In the context of gravitational force, the acceleration due to gravity (g) on the smaller mass m can be expressed as:
Setting the two expressions for force equal to each other gives:
To find the gravitational acceleration g, divide both sides by m (assuming 𝑚≠0):
It states that the Gravitational acceleration (g) at a distance 𝑟 from the center of mass 𝑀 is directly proportional to 𝑀 and inversely proportional to the square of 𝑟.
An example of gravitational acceleration is an apple falling from a tree due to Earth’s gravity, accelerating at approximately 9.81 m/s².
The formula calculates the acceleration due to gravity exerted by a mass (like a planet) on objects at a specific distance from its center.
Devices called Gravimeters measure gravitational acceleration near Earth by detecting changes in Gravitational pull.

Gravitational acceleration refers to the acceleration of an object caused by the force of gravity from another object, typically a planet or a moon. The most common formula to calculate gravitational acceleration is derived from Newton’s Law of Universal Gravitation, discovered by Sir Isaac Newton in the late 17th century. Newton’s groundbreaking work established the foundational principles that describe how objects attract each other with a force proportional to their masses and inversely proportional to the square of the distance between their centers.
We express the standard formula for gravitational acceleration as:
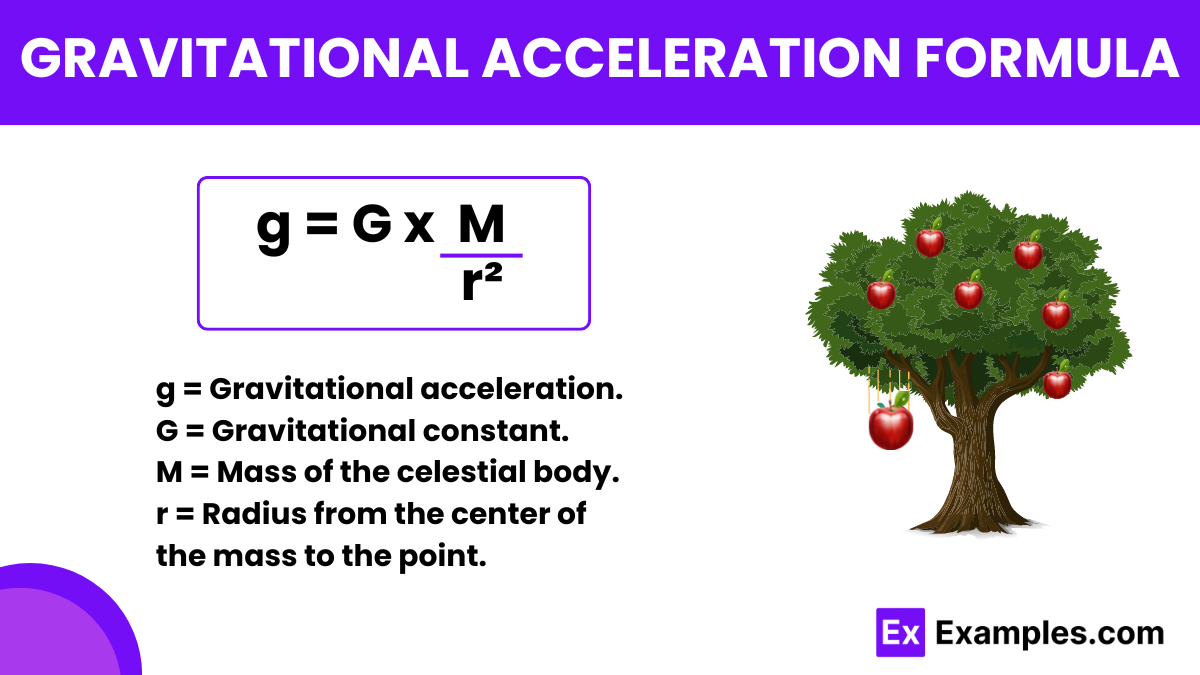
g = G x (M / r²)
g is the gravitational acceleration.
G is the gravitational constant (6.67430 ×10⁻¹¹ m³ kg⁻¹ s⁻²).
M is the mass of the celestial body (like Earth).
r is the radius from the center of the mass to the point where the acceleration is being measured.
For objects not at the Earth’s surface but at a height h above it, the formula adjusts to account for the increased distance from the Earth’s center. This variation is important for calculations involving satellites or high-altitude flights. The modified formula becomes:
g = G x (M / (R+h)²)
R is the Earth’s radius.
As h increases, g decreases, illustrating the inverse square law inherent in gravitational interactions.
Another essential formula relates to objects in free fall near the Earth’s surface, where gravitational acceleration is approximated as a constant:
g ≈ 9.81 m/s²
This value simplifies many physics calculations involving motion near the Earth’s surface and assumes a uniform gravitational field without significant variation in altitude or geographical location. These formulas allow physicists and engineers to calculate how quickly objects will accelerate towards each other or the ground, providing crucial data for everything from engineering to aerospace development.
Newton’s Law of Universal Gravitation states that two masses (M and m) separated by a distance (r) exert a force (F) on each other as given by: F = G x ( M × m) / r²
F is the gravitational force,
G is the gravitational constant (approximately 6.674×10⁻¹¹ m³ kg⁻¹ s⁻² ),
M is the mass of the larger object (e.g., Earth),
m is the mass of the smaller object,
𝑟 is the distance between the centers of the two masses.
Newton’s second law states that force equals mass times acceleration (𝐹 = 𝑚a). In the context of gravitational force, the acceleration due to gravity (g) on the smaller mass m can be expressed as:
𝐹 = 𝑚 × 𝑔
Setting the two expressions for force equal to each other gives:
𝑚 × 𝑔= (𝐺(𝑀 × 𝑚)) / 𝑟²
To find the gravitational acceleration g, divide both sides by m (assuming 𝑚≠0):
𝑔=𝐺𝑀 / 𝑟²
It states that the Gravitational acceleration (g) at a distance 𝑟 from the center of mass 𝑀 is directly proportional to 𝑀 and inversely proportional to the square of 𝑟.
Space Exploration: It helps calculate satellite orbits and plan spacecraft trajectories.
Aerospace Engineering: Engineers use it to design flight paths and simulate space conditions.
Geophysics: The formula aids in estimating Earth’s mass distribution, essential for understanding internal structures.
Oceanography: It impacts modeling of tides and ocean currents.
Civil Engineering: Knowing gravitational acceleration is vital for building structures like skyscrapers and bridges.
Point Mass Assumption: It simplifies objects to point masses, which isn’t always accurate for large or close bodies.
Uniform Sphere Assumption: It assumes celestial bodies are perfectly spherical with uniform density.
Excludes External Forces: The formula doesn’t consider forces like electromagnetic influences or Atmospheric drag.
Non-relativistic: General Relativity becomes necessary near black holes or at high velocities where the standard formulas are not suitable.
Ignores Variations: It overlooks changes in gravitational pull due to altitude, Latitude, or Earth’s rotation.
An example of gravitational acceleration is an apple falling from a tree due to Earth’s gravity, accelerating at approximately 9.81 m/s².
The formula calculates the acceleration due to gravity exerted by a mass (like a planet) on objects at a specific distance from its center.
Devices called Gravimeters measure gravitational acceleration near Earth by detecting changes in Gravitational pull.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the value of the acceleration due to gravity on Earth\'s surface?
8.8 m/s²
9.8 m/s²
10.8 m/s²
11.8 m/s²
Which formula represents the relationship between gravitational force, mass, and acceleration due to gravity?
F = ma
F = mg
F = m/g
F = mg/2
If an object has a mass of 10 kg, what is the gravitational force acting on it on Earth's surface?
9.8 N
98 N
980 N
9800 N
Which of the following correctly defines gravitational acceleration?
The rate of change of velocity per unit time
The force per unit mass due to gravity
The force per unit area due to gravity
The distance per unit time due to gravity
What is the weight of a 50 kg object on Earth?
50 N
100 N
490 N
500 N
An object is dropped from a height. What is its acceleration just before it hits the ground (ignoring air resistance)?
0 m/s²
9.8 m/s²
19.6 m/s²
98 m/s²
If a 70 kg astronaut is on a planet where the acceleration due to gravity is
5
m/s
2
5m/s
2
, what is their weight on that planet?
35 N
140 N
350 N
700 N
Which of the following statements is true about the acceleration due to gravity?
The weight decreases
The weight increases
The weight remains the same
The weight becomes zero
A ball is thrown upward with an initial velocity of 20 m/s. What is its acceleration at the highest point?
0 m/s²
9.8 m/s² upward
9.8 m/s² downward
20 m/s² downward
What is the gravitational force on a 25 kg object at Earth's surface?
245 N
250 N
275 N
300 N
Before you leave, take our quick quiz to enhance your learning!

