What does the Maxwell-Boltzmann distribution describe?
Distribution of electric charge
Distribution of particle speeds in a gas
Distribution of magnetic fields
Distribution of photon energies
of 10
Maxwell Boltzmann Formula – Formula, Usage, Example Problems
Maxwell Boltzmann Formula – Formula, Usage, Example Problems
What is Maxwell Boltzmann Formula?
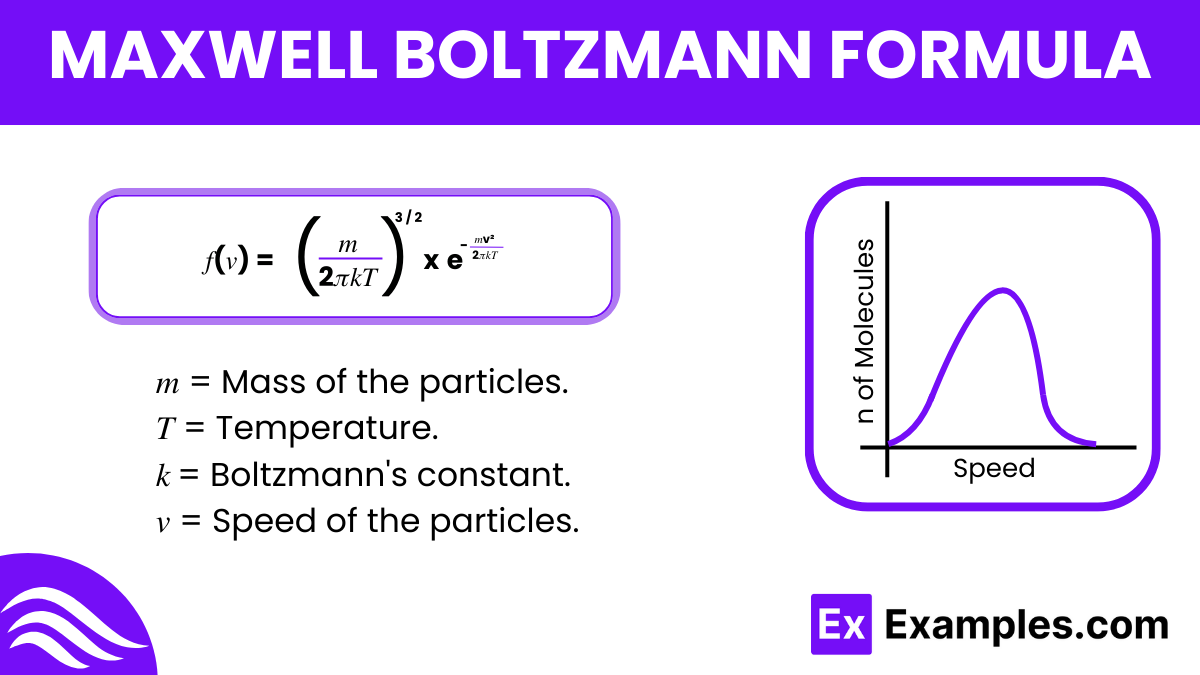
The Maxwell-Boltzmann formula, developed by James Clerk Maxwell and Ludwig Boltzmann in the 19th century, describes the speed distribution of particles in a gas at thermal equilibrium. This formula is pivotal in physics, especially in the study of statistical mechanics. It helps in understanding how particle speeds in a gas vary at a given temperature.
The formula is expressed as
- 𝑚 is the mass of the particles.
- 𝑇 is the temperature.
- 𝑘 is Boltzmann’s constant.
- 𝑣 is the speed of the particles.
This equation shows that most particles in a gas move at moderate speeds, with very few moving very quickly or very slowly, providing a statistical view of molecular motion in gases.
Usages of Maxwell Boltzmann Formula
- Predicting Gas Properties: It calculates properties like pressure and temperature of gases, crucial for understanding gas behavior in various conditions.
- Engineering Applications: Engineers use this formula to design and optimize systems involving gas flow, such as jet engines and exhaust systems.
- Chemical Reaction Rates: The formula helps in estimating the speeds of molecules which is vital for determining how fast chemical reactions occur.
- Astrophysics: It aids in analyzing the distribution of speeds of particles in stars and planetary atmospheres, offering insights into their thermal properties.
- Material Science: Understanding the diffusion of gases through materials, important for creating efficient filters and membranes.
Example Problems on Maxwell Boltzmann Formula
Problem 1: Calculating Particle Speed
Question: In a container of helium gas at 300 K, estimate the most probable speed of the helium atoms. Assume the mass of a helium atom is 4×10⁻²⁷ kg.
Solution:
The most probable speed 𝑣𝑝vp can be calculated using the formula: 𝑣𝑝 = √ 2𝑘𝑇 / 𝑚 Where:
𝑘=1.38×10⁻²³ J/K (Boltzmann constant),
𝑇=300 K,
𝑚=4×10⁻²⁷ kg.
Plugging in the values:
𝑣𝑝 = √( ( 2 × 1.38 × 10⁻²³ × 300 ) / ( 4 × 10⁻²⁷ ) ) ≈ 1370 m/s
Problem 2: Comparing Particle Speeds
Question: Compare the most probable speeds of hydrogen and oxygen molecules in a gas mixture at 400 K. Assume the mass of a hydrogen molecule (H₂) is 2 × 10⁻²⁷ kg and the mass of an oxygen molecule (O₂) is 32×10⁻²⁷ kg.
Solution: Calculate the most probable speed for each:
Hydrogen:
𝑣𝑝, H₂ = √ 2𝑘𝑇 / 𝑚H₂ = √ (( 2 × 1.38 × 10⁻²³ × 400) / (2×10⁻²⁷)) ≈ 1838 m/s
Oxygen:
𝑣𝑝, O₂ = √ 2𝑘𝑇 / 𝑚O₂ = √ ( (2×1.38×10⁻²³ × 400 ) / ( 32 × 10⁻²⁷ ) ) ≈ 459 m/s
Problem 3: Determining Kinetic Energy Distribution
Question: Calculate the fraction of nitrogen molecules (molecular mass 28×10⁻²⁷ kg) moving faster than 500 m/s at a temperature of 298 K.
Solution: First, calculate the fraction using the Maxwell-Boltzmann speed distribution function for speeds greater than 500 m/s. We use the cumulative distribution function (CDF):
Fraction=∫∞₅₀₀𝑓(𝑣) 𝑑𝑣
Where 𝑓(𝑣) is the Maxwell-Boltzmann distribution function. We simplify by using an integral table or computational tools because the integral calculation involves exponential functions:
Fraction ≈ 0.12
FAQs
What Does Maxwell-Boltzmann Show?
Maxwell-Boltzmann distribution illustrates how gas particles’ speeds vary at a specific temperature, predicting molecular motion in thermal equilibrium.
What is K in Boltzmann’s Formula?
In Boltzmann’s formula, 𝑘k represents the Boltzmann constant, 1.38×10⁻²³ J/K, linking temperature with energy.
What is the Formula for Maxwell Boltzmann Statistics?
The Maxwell-Boltzmann formula is 𝑓(𝑣) = ( (𝑚 / 2𝜋𝑘𝑇)^ 3/ 2 ) x (𝑒 ^−𝑚𝑣² / 2𝑘𝑇), defining particle speed distribution in gases.
Share :
Maxwell Boltzmann Formula – Formula, Usage, Example Problems
What is Maxwell Boltzmann Formula?
The Maxwell-Boltzmann formula, developed by James Clerk Maxwell and Ludwig Boltzmann in the 19th century, describes the speed distribution of particles in a gas at thermal equilibrium. This formula is pivotal in physics, especially in the study of statistical mechanics. It helps in understanding how particle speeds in a gas vary at a given temperature.
The formula is expressed as
𝑓(𝑣) = ( (𝑚 / 2𝜋𝑘𝑇)^ 3/ 2 ) x (𝑒 ^−𝑚𝑣² / 2𝑘𝑇)
𝑚 is the mass of the particles.
𝑇 is the temperature.
𝑘 is Boltzmann’s constant.
𝑣 is the speed of the particles.
This equation shows that most particles in a gas move at moderate speeds, with very few moving very quickly or very slowly, providing a statistical view of molecular motion in gases.
Usages of Maxwell Boltzmann Formula
Predicting Gas Properties: It calculates properties like pressure and temperature of gases, crucial for understanding gas behavior in various conditions.
Engineering Applications: Engineers use this formula to design and optimize systems involving gas flow, such as jet engines and exhaust systems.
Chemical Reaction Rates: The formula helps in estimating the speeds of molecules which is vital for determining how fast chemical reactions occur.
Astrophysics: It aids in analyzing the distribution of speeds of particles in stars and planetary atmospheres, offering insights into their thermal properties.
Material Science: Understanding the diffusion of gases through materials, important for creating efficient filters and membranes.
Example Problems on Maxwell Boltzmann Formula
Problem 1: Calculating Particle Speed
Question: In a container of helium gas at 300 K, estimate the most probable speed of the helium atoms. Assume the mass of a helium atom is 4×10⁻²⁷ kg.
Solution:
The most probable speed 𝑣𝑝vp can be calculated using the formula: 𝑣𝑝 = √ 2𝑘𝑇 / 𝑚 Where:
𝑘=1.38×10⁻²³ J/K (Boltzmann constant),
𝑇=300 K,
𝑚=4×10⁻²⁷ kg.
Plugging in the values:
𝑣𝑝 = √( ( 2 × 1.38 × 10⁻²³ × 300 ) / ( 4 × 10⁻²⁷ ) ) ≈ 1370 m/s
Problem 2: Comparing Particle Speeds
Question: Compare the most probable speeds of hydrogen and oxygen molecules in a gas mixture at 400 K. Assume the mass of a hydrogen molecule (H₂) is 2 × 10⁻²⁷ kg and the mass of an oxygen molecule (O₂) is 32×10⁻²⁷ kg.
Solution: Calculate the most probable speed for each:
Hydrogen:
𝑣𝑝, H₂ = √ 2𝑘𝑇 / 𝑚H₂ = √ (( 2 × 1.38 × 10⁻²³ × 400) / (2×10⁻²⁷)) ≈ 1838 m/s
Oxygen:
𝑣𝑝, O₂ = √ 2𝑘𝑇 / 𝑚O₂ = √ ( (2×1.38×10⁻²³ × 400 ) / ( 32 × 10⁻²⁷ ) ) ≈ 459 m/s
Problem 3: Determining Kinetic Energy Distribution
Question: Calculate the fraction of nitrogen molecules (molecular mass 28×10⁻²⁷ kg) moving faster than 500 m/s at a temperature of 298 K.
Solution: First, calculate the fraction using the Maxwell-Boltzmann speed distribution function for speeds greater than 500 m/s. We use the cumulative distribution function (CDF):
Fraction=∫∞₅₀₀𝑓(𝑣) 𝑑𝑣
Where 𝑓(𝑣) is the Maxwell-Boltzmann distribution function. We simplify by using an integral table or computational tools because the integral calculation involves exponential functions:
Fraction ≈ 0.12
FAQs
What Does Maxwell-Boltzmann Show?
Maxwell-Boltzmann distribution illustrates how gas particles’ speeds vary at a specific temperature, predicting molecular motion in thermal equilibrium.
What is K in Boltzmann’s Formula?
In Boltzmann’s formula, 𝑘k represents the Boltzmann constant, 1.38×10⁻²³ J/K, linking temperature with energy.
What is the Formula for Maxwell Boltzmann Statistics?
The Maxwell-Boltzmann formula is 𝑓(𝑣) = ( (𝑚 / 2𝜋𝑘𝑇)^ 3/ 2 ) x (𝑒 ^−𝑚𝑣² / 2𝑘𝑇), defining particle speed distribution in gases.
Practice Test
What is the general shape of the Maxwell-Boltzmann speed distribution curve?
Linear
Exponential
Bell-shaped
Parabolic
of 10
How does the Maxwell-Boltzmann distribution change with increasing temperature?
It becomes narrower
It becomes wider and shifts to higher speeds
It remains unchanged
It shifts to lower speeds
of 10
How does the average speed of particles in a gas relate to temperature in the Maxwell-Boltzmann distribution?
It decreases with increasing temperature
It remains constant with temperature
It increases with increasing temperature
It fluctuates randomly with temperature
of 10
Which constant is essential in the Maxwell-Boltzmann distribution formula?
Gravitational constant
Planck's constant
Boltzmann constant
Avogadro's constant
of 10
What is the effect of increasing the mass of particles on the Maxwell-Boltzmann distribution curve at constant temperature?
It shifts to higher speeds
It shifts to lower speeds
It becomes wider
It remains unchanged
of 10
What is the relationship between kinetic energy and speed in the Maxwell-Boltzmann distribution?
Kinetic energy is directly proportional to speed
Kinetic energy is inversely proportional to speed
Kinetic energy is proportional to the square of the speed
Kinetic energy is unrelated to speed
of 10
Which parameter in the Maxwell-Boltzmann distribution affects the spread of particle speeds?
Mass
Volume
Temperature
Pressure
of 10
What does the area under the Maxwell-Boltzmann distribution curve represent?
Total energy of the system
Total number of particles
Total volume of the gas
Total pressure of the gas
of 10
What happens to the Maxwell-Boltzmann distribution curve at absolute zero temperature?
It becomes a delta function at zero speed
It shifts to infinite speed
It becomes a uniform distribution
It remains unchanged
of 10