If two vectors A and B form a parallelogram and the resultant vector R is along one of the vectors, what can be inferred about the angle between them?
0 degrees
45 degrees
90 degrees
180 degrees

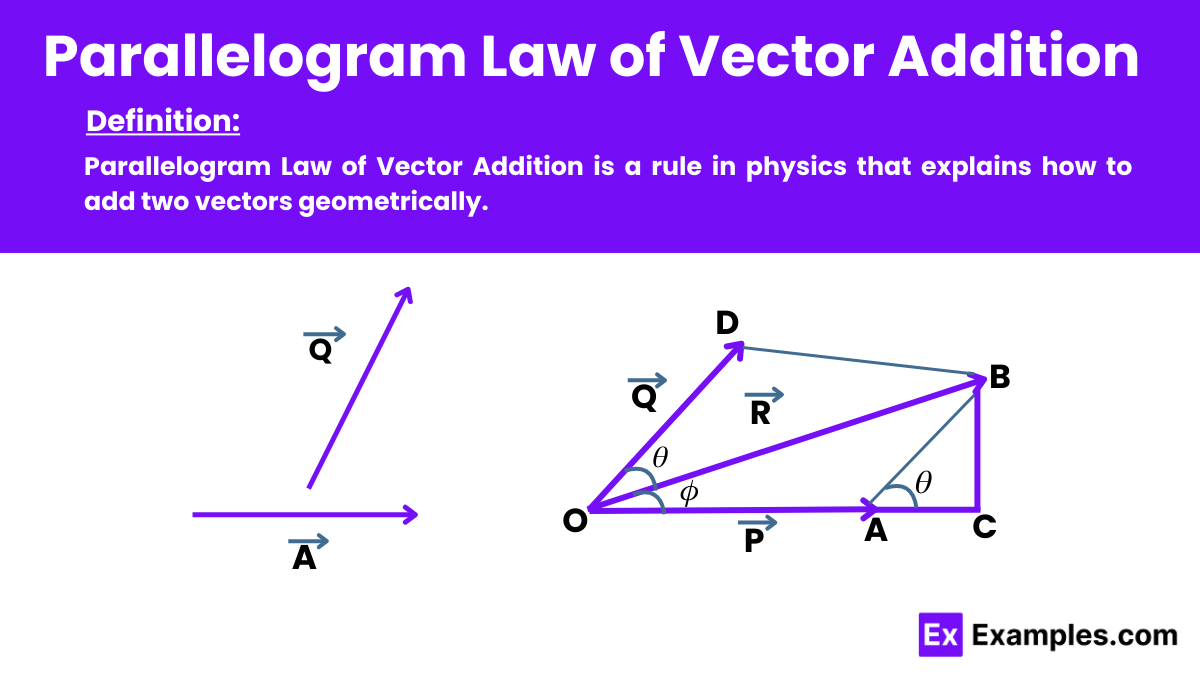
Parallelogram Law of Vector Addition is a rule in physics that explains how to add two vectors geometrically. According to this law of physics, if two vectors are represented as adjacent sides of a parallelogram, the diagonal that starts from the same point as the vectors represents their resultant. The magnitude and direction of this diagonal give the sum of the vectors. This law is fundamental in physics, as it helps in visually determining the resultant vector of two forces acting at an angle to each other.
The Parallelogram Law of Forces helps you find the resultant of two forces acting simultaneously on an object. According to this law, when two forces act on an object from the same point and at a certain angle to each other, you represent them as adjacent sides of a parallelogram. Consequently, the diagonal of the parallelogram, drawn from the same point, provides the magnitude and direction of the resultant force. By applying this law, you can effectively determine the combined impact of two distinct forces acting on an object. Providing a clear visual method for understanding vector addition in physics.
The formula for the Parallelogram Law of Vector Addition involves finding the resultant of two vectors. 𝐴 and 𝐵, which form adjacent sides of a parallelogram. The magnitude of the resultant vector 𝑅 is:
where:
The direction of the resultant vector can also be found using trigonometric relationships based on the magnitudes and the angle between the original vectors.
Let vectors 𝑃 and 𝑄 represent two adjacent sides of a parallelogram. The diagonal 𝑅 represents the resultant vector.
2. Applying the Law: The parallelogram law states that the diagonal 𝑅 equals the sum of vectors 𝑃 and 𝑄: R=P+Q
3. Breaking Down the Geometry: Extend line DA to C, and draw BC perpendicular to OC. From the right-angled triangle △𝑂𝐶: 𝑂𝐵²=𝑂𝐶²+𝐵𝐶² Substitute 𝑂𝐶=𝑂𝐴+𝐴𝐶 to get: 𝑂𝐵²=(𝑂𝐴+𝐴𝐶)²+𝐵𝐶²
4. Analyzing the Angles: In triangle △𝐴𝐵𝐶:
5. Substituting and Simplifying: Substitute the values of 𝐴𝐶 and 𝐵𝐶 into the expression:
𝑅²=√(𝑃+𝑄cos𝜃)²+(𝑄sin𝜃)²
Expanding and simplifying further: 𝑅²=𝑃²+2𝑃𝑄cos𝜃+𝑄²cos²𝜃+𝑄²sin²𝜃
Using the trigonometric identity cos²𝜃+sin²𝜃=1: 𝑅²=𝑃²+2𝑃𝑄cos𝜃+𝑄²
6. Resultant Magnitude: Taking the square root gives the magnitude of the resultant vector: 𝑅=√𝑃²+𝑄²+2𝑃𝑄cos𝜃
7. Direction of Resultant Vector: To find the angle 𝜙 between 𝑅 and 𝑃,
we use: tan𝜙=𝑄sin𝜃/𝑃+𝑄cos𝜃
Thus, the direction of 𝑅 is : 𝜙=tan⁻¹(𝑄sin𝜃/𝑃+𝑄cos𝜃)
| Case | Expression | Magnitude of Resultant Vector | Direction of Resultant Vector |
|---|---|---|---|
| P is parallel to Q in the same direction. | P | | Q | |R| = P + Q | ϕ = 0° |
| P is parallel to Q but in a different direction. | P ↑↓ Q | |R| = P – Q or Q – P | ϕ = 0° or 180° |
| P is perpendicular to Q. | P ⊥ Q | |R| = √(P² + Q²) | ϕ = tan⁻¹(Q/P) |
Here are some important points about the Parallelogram Law of Vector Addition:
This method involves reversing the direction of one vector and then applying the parallelogram law to find the resultant. Effectively subtracting one vector from another.
The two types of vector addition include the head-to-tail method and the parallelogram method, which allow the graphical or analytical combination of two or more vectors.
You can find the resultant force by summing all individual forces as vectors. Considering both magnitude and direction to find their combined effect.

Parallelogram Law of Vector Addition is a rule in physics that explains how to add two vectors geometrically. According to this law of physics, if two vectors are represented as adjacent sides of a parallelogram, the diagonal that starts from the same point as the vectors represents their resultant. The magnitude and direction of this diagonal give the sum of the vectors. This law is fundamental in physics, as it helps in visually determining the resultant vector of two forces acting at an angle to each other.
The Parallelogram Law of Vector Addition is a principle in physics and mathematics that describes how to combine two vectors to find their resultant. According to this law, if two vectors are represented as adjacent sides of a parallelogram, then the diagonal of the parallelogram, which starts from the same point as the vectors, represents their sum or resultant vector. The direction and magnitude of this diagonal indicate the resultant vector’s direction and magnitude.
The Parallelogram Law of Addition enables you to find the resultant of two vectors graphically. When you represent two vectors as adjacent sides of a parallelogram, the diagonal of this parallelogram. Starting from the same point, represents their sum or resultant. This diagonal directly shows you the magnitude and direction of the resultant vector. Effectively combining the two initial vectors into a single vector that represents their combined effect.
The Parallelogram Law of Forces helps you find the resultant of two forces acting simultaneously on an object. According to this law, when two forces act on an object from the same point and at a certain angle to each other, you represent them as adjacent sides of a parallelogram. Consequently, the diagonal of the parallelogram, drawn from the same point, provides the magnitude and direction of the resultant force. By applying this law, you can effectively determine the combined impact of two distinct forces acting on an object. Providing a clear visual method for understanding vector addition in physics.
The formula for the Parallelogram Law of Vector Addition involves finding the resultant of two vectors. 𝐴 and 𝐵, which form adjacent sides of a parallelogram. The magnitude of the resultant vector 𝑅 is:
𝑅=√P²+Q²+2PQcos𝜃
where:
𝑅: Magnitude of the resultant vector
P: Magnitude of vector P
Q: Magnitude of vector Q
𝜃: Angle between vectors 𝐴 and 𝐵
The direction of the resultant vector can also be found using trigonometric relationships based on the magnitudes and the angle between the original vectors.
Let vectors 𝑃 and 𝑄 represent two adjacent sides of a parallelogram. The diagonal 𝑅 represents the resultant vector.
2. Applying the Law: The parallelogram law states that the diagonal 𝑅 equals the sum of vectors 𝑃 and 𝑄: R=P+Q
3. Breaking Down the Geometry: Extend line DA to C, and draw BC perpendicular to OC. From the right-angled triangle △𝑂𝐶: 𝑂𝐵²=𝑂𝐶²+𝐵𝐶² Substitute 𝑂𝐶=𝑂𝐴+𝐴𝐶 to get: 𝑂𝐵²=(𝑂𝐴+𝐴𝐶)²+𝐵𝐶²
4. Analyzing the Angles: In triangle △𝐴𝐵𝐶:
cos𝜃=𝐴𝐶/𝐴𝐵⇒𝐴𝐶=𝐴𝐵cos𝜃=𝑄cos𝜃
sin𝜃=𝐵𝐶/𝐴𝐵⇒𝐵𝐶=𝐴𝐵sin𝜃=𝑄sin𝜃
5. Substituting and Simplifying: Substitute the values of 𝐴𝐶 and 𝐵𝐶 into the expression:
𝑅²=√(𝑃+𝑄cos𝜃)²+(𝑄sin𝜃)²
Expanding and simplifying further: 𝑅²=𝑃²+2𝑃𝑄cos𝜃+𝑄²cos²𝜃+𝑄²sin²𝜃
Using the trigonometric identity cos²𝜃+sin²𝜃=1: 𝑅²=𝑃²+2𝑃𝑄cos𝜃+𝑄²
6. Resultant Magnitude: Taking the square root gives the magnitude of the resultant vector: 𝑅=√𝑃²+𝑄²+2𝑃𝑄cos𝜃
7. Direction of Resultant Vector: To find the angle 𝜙 between 𝑅 and 𝑃,
we use: tan𝜙=𝑄sin𝜃/𝑃+𝑄cos𝜃
Thus, the direction of 𝑅 is : 𝜙=tan⁻¹(𝑄sin𝜃/𝑃+𝑄cos𝜃)
Case | Expression | Magnitude of Resultant Vector | Direction of Resultant Vector |
|---|---|---|---|
P is parallel to Q in the same direction. | P | | Q | |R| = P + Q | ϕ = 0° |
P is parallel to Q but in a different direction. | P ↑↓ Q | |R| = P – Q or Q – P | ϕ = 0° or 180° |
P is perpendicular to Q. | P ⊥ Q | |R| = √(P² + Q²) | ϕ = tan⁻¹(Q/P) |
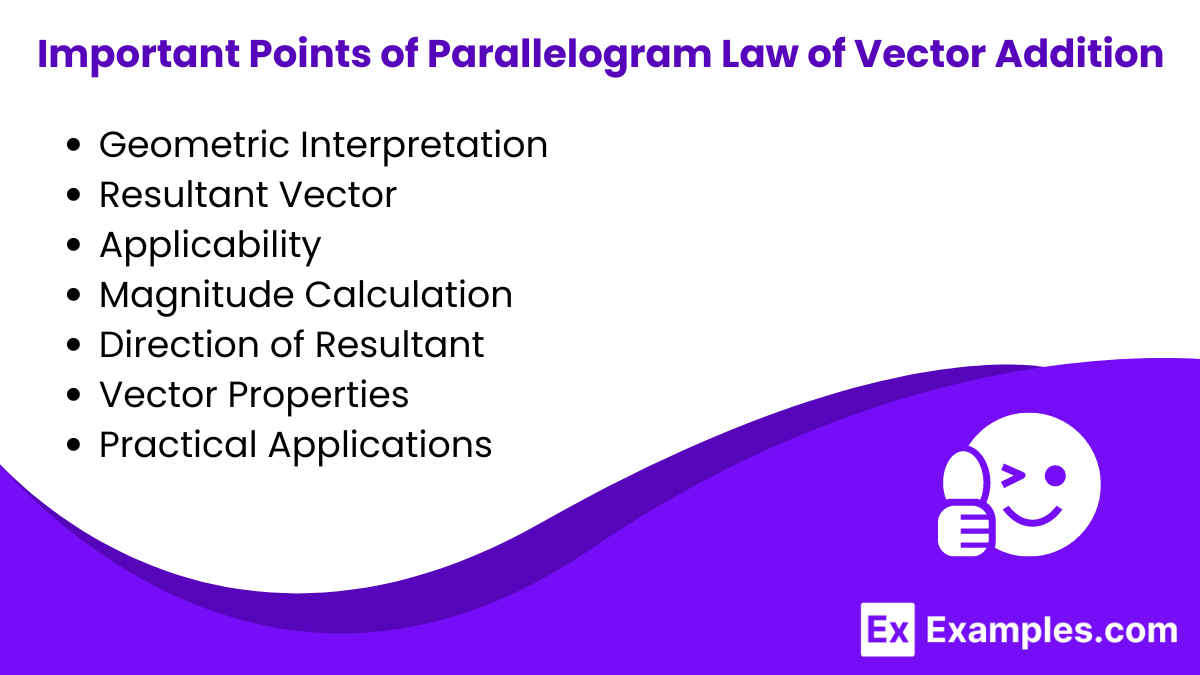
Here are some important points about the Parallelogram Law of Vector Addition:
Geometric Interpretation: The law provides a graphical method to find the resultant of two vectors. If you draw the two vectors as adjacent sides of a parallelogram, the diagonal represents their sum.
Resultant Vector: The resultant vector is the diagonal starting from the same point as the vectors. Its magnitude and direction are determined by the relative magnitudes and directions of the original vectors.
Applicability: The law applies to any two vectors, regardless of their magnitudes and the angle between them, allowing you to compute the resultant vector in many scenarios.
Magnitude Calculation: You can calculate the magnitude of the resultant vector using the formula 𝑅=√𝐴²+𝐵²+2𝐴𝐵cos𝜃, where 𝜃 is the angle between the two vectors.
Direction of Resultant: The direction of the resultant vector can be calculated using trigonometric relationships, providing both the magnitude and direction.
Vector Properties: The law maintains vector properties such as commutativity. Meaning you get the same resultant vector regardless of the order of the two vectors.
Practical Applications: Engineers and physicists use this law frequently to analyze forces, velocities, and other vector quantities in physics and engineering problems.
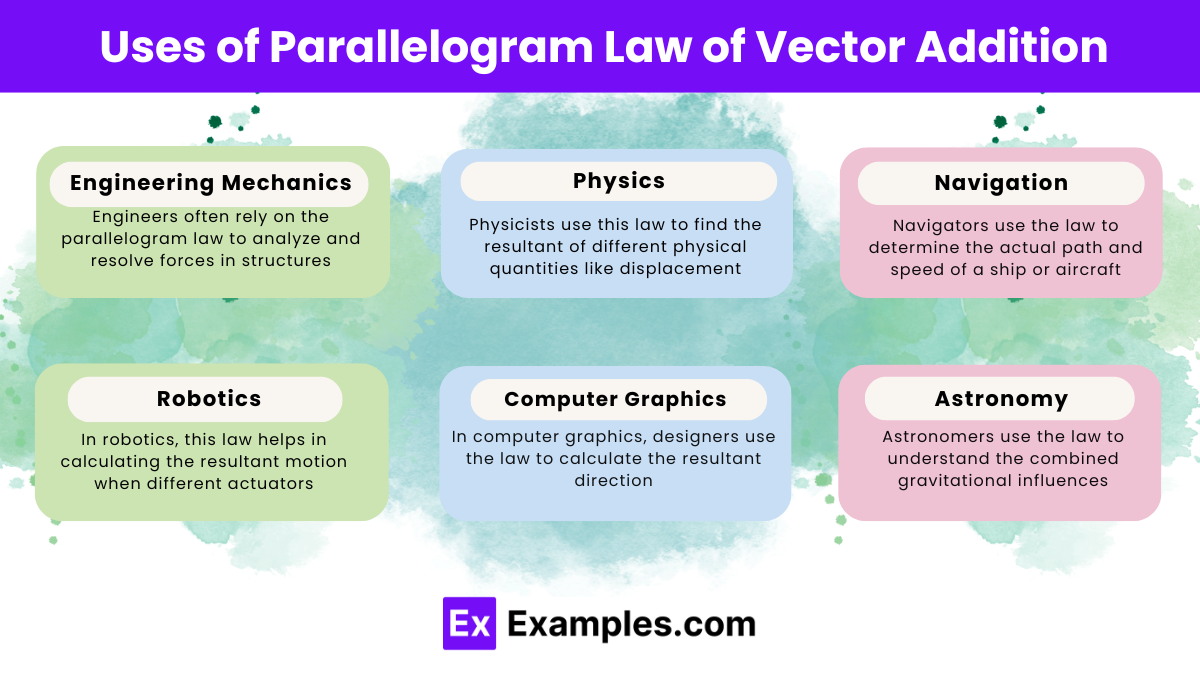
Engineering Mechanics: Engineers often rely on the parallelogram law to analyze and resolve forces in structures. Thereby ensuring stability and safety in bridges, buildings, and machines.
Physics: Physicists use this law to find the resultant of different physical quantities like displacement, velocity, and acceleration, simplifying complex motion problems.
Navigation: Navigators use the law to determine the actual path and speed of a ship or aircraft when subjected to multiple forces such as wind and currents.
Robotics: In robotics, this law helps in calculating the resultant motion when different actuators apply varying forces on a robotic arm, making control and movement more precise.
Computer Graphics: In computer graphics, designers use the law to calculate the resultant direction and magnitude of forces affecting an object. Allowing for more realistic motion simulations.
Astronomy: Astronomers use the law to understand the combined gravitational influences on celestial bodies. Improving predictions of planetary orbits and space travel trajectories.
Bridge Design: Engineers use the parallelogram law to calculate the resultant forces acting on a bridge. This simplifies the design process by ensuring the structure withstands multiple loads effectively.
Navigation: Pilots apply the law to find the actual course and speed of an aircraft. They consider the effects of wind and other factors. Allowing for precise navigation.
Physics Problems: Physicists employ the parallelogram law to resolve two-dimensional force problems. This helps in analyzing the resultant forces acting on an object in motion.
Robot Movement: In robotics, developers rely on this law to determine the direction and speed of a robot. It helps them understand the combined influence of different actuators.
Vehicle Motion: Automotive engineers use this law to calculate the resultant vector of forces acting on a vehicle. It helps optimize performance by analyzing factors like engine power and road friction.
This method involves reversing the direction of one vector and then applying the parallelogram law to find the resultant. Effectively subtracting one vector from another.
The two types of vector addition include the head-to-tail method and the parallelogram method, which allow the graphical or analytical combination of two or more vectors.
You can find the resultant force by summing all individual forces as vectors. Considering both magnitude and direction to find their combined effect.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
If two vectors A and B form a parallelogram and the resultant vector R is along one of the vectors, what can be inferred about the angle between them?
0 degrees
45 degrees
90 degrees
180 degrees
If vectors A and B have magnitudes of 7 units and 24 units respectively, and the angle between them is 90 degrees, what is the magnitude of the resultant vector R?
25 units
31 units
17 units
30 units
What is the direction of the resultant vector R formed by two vectors A and B using the Parallelogram Law?
It is always along vector A
It is always along vector B
It is perpendicular to both vectors
It is along the diagonal of the parallelogram formed by A and B**
If vectors A and B have magnitudes of 3 units and 4 units respectively, and are in the same direction, what is the magnitude of the resultant vector R?
1 unit
5 unit
7 unit
8 unit
What does the Parallelogram Law of Vector Addition state about the resultant vector?
It is always equal to the sum of the vectors.
It lies in the direction of the smaller vector.
It is represented by the diagonal of the parallelogram formed by the two vectors.
It has the same magnitude as the larger vector.
According to the Parallelogram Law of Vector Addition, what happens to the resultant vector when the angle between two vectors is 90 degrees?
It becomes zero.
It becomes the sum of the magnitudes of the vectors.
It becomes the difference of the magnitudes of the vectors.
It forms a right triangle with the two vectors.
In the Parallelogram Law of Vector Addition, if the two vectors have the same magnitude and are in opposite directions, what is the resultant vector?
It is zero.
It is double the magnitude of one of the vectors.
It is half the magnitude of one of the vectors.
It is the same as the magnitude of one of the vectors.
Which of the following is true about the direction of the resultant vector in the Parallelogram Law of Vector Addition?
It always points in the direction of the larger vector.
It is perpendicular to the vectors being added.
It lies along the diagonal of the parallelogram formed by the two vectors.
It always points in the direction of the smaller vector.
In the Parallelogram Law of Vector Addition, what determines the length of the diagonal representing the resultant vector?
The sum of the angles between the vectors.
The magnitudes of the vectors and the angle between them.
The difference in magnitudes of the vectors.
The average of the magnitudes of the vectors.
What shape is formed when two vectors are added using the Parallelogram Law and their magnitudes are equal and the angle between them is 90 degrees?
Square
Rectangle
Triangle
Trapezoid
Before you leave, take our quick quiz to enhance your learning!

