Square & Square Root of 1444
Square & Square Root of 1444
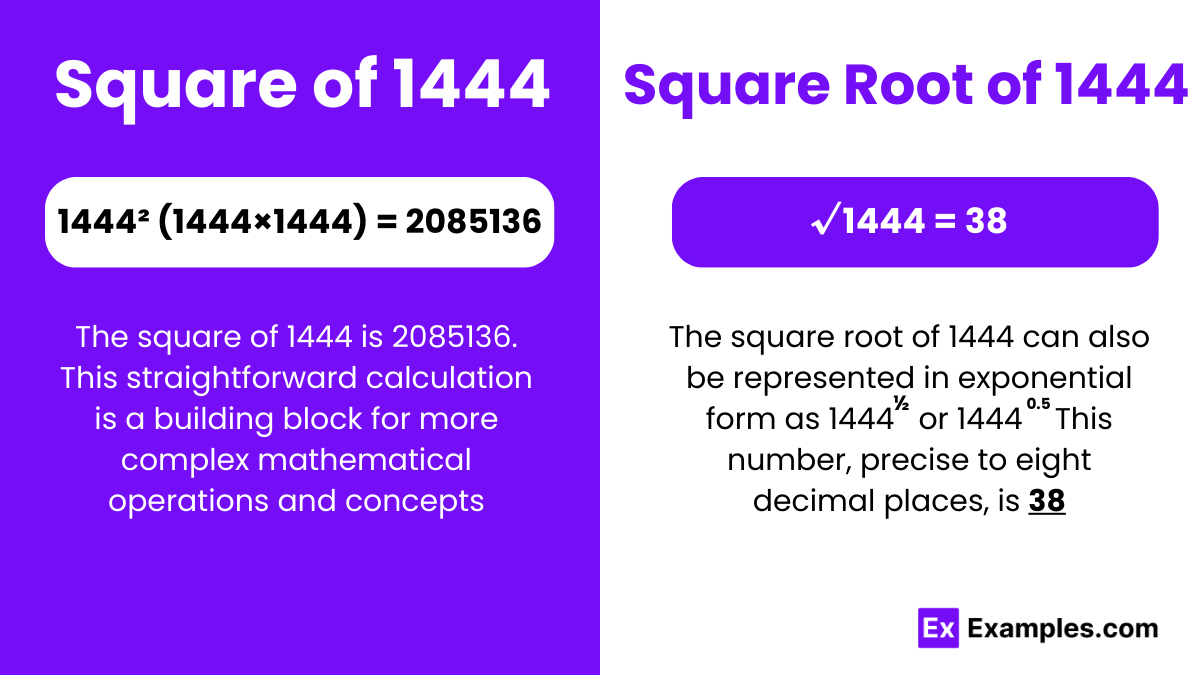
In mathematics, particularly in algebra, the concepts of square and square roots are fundamental. Squaring a number, like 1444, means multiplying it by itself, yielding a result such as 2,085,136. This operation is crucial in exploring properties of both rational numbers (which can be expressed as a fraction of two integers) and Irrational Numbers (which cannot be neatly expressed as a fraction). Understanding these concepts helps deepen knowledge of mathematical relationships and patterns.
Square of 1444
This calculation is what is referred to as “squaring” the number 1444, and the result, 2,085,136, is the “square” of 1444. This process is used in various mathematical applications to represent area or to perform computations in algebra and geometry.
Square Root of 1444
The square root of a number is a value that, when multiplied by itself, gives the original number. In this case, the number is 1444.
Finding the square root of 1444 involves determining what number, when multiplied by itself, equals 1444. Mathematically, this is represented as:
1444 = 38
This means that 38 multiplied by 38 equals 1444:
38×38 = 1444
The square root operation is widely used in mathematics, particularly in algebra and geometry, to solve various equations and problems involving areas and lengths.
Exponential Form: 1444^½ or 1444^0.5
Radical Form: √1444
Is the Square Root of 1444 Rational or Irrational?
The square root of 1444 is rational. A number is considered rational if it can be expressed as the fraction of two integers (where the denominator is not zero). In this case, the square root of 1444 is 38, which can be expressed as a fraction 38/1. Since 38 is an integer, its square root is a rational number.
Methods to Find Value of Root 1444
Finding the square root of 1444 can be approached using several methods, each with its own application and level of precision. Here are some common methods:
Prime Factorization:
Break down 1444 into its prime factors. Since 1444 = 2 × 2 × 19 × 19, its square root is the product of each factor taken once, i.e., √1444 = 2×19 =3 8.
Long Division Method:
This is a manual method that involves a step-by-step division-like process to find the square root. It’s particularly useful for larger numbers or when the prime factors aren’t easily apparent.
Using a Calculator:
The most straightforward method is to use a calculator, either a physical one or a software-based one like those on computers and smartphones.
Newton’s Method (Newton-Raphson):
This is a numerical technique for finding approximate solutions to numerically solving equations like 𝑥²−1444 = 0. It involves iterative guessing and refining the guess based on the derivative of the function.
Babylonian Method (Guess and Check):
This ancient method, also known as the “Heron’s method”, involves making an initial guess at the square root and then improving the guess and Repeat until the value stabilizes.
Square Root of 1444 by Long Division Method
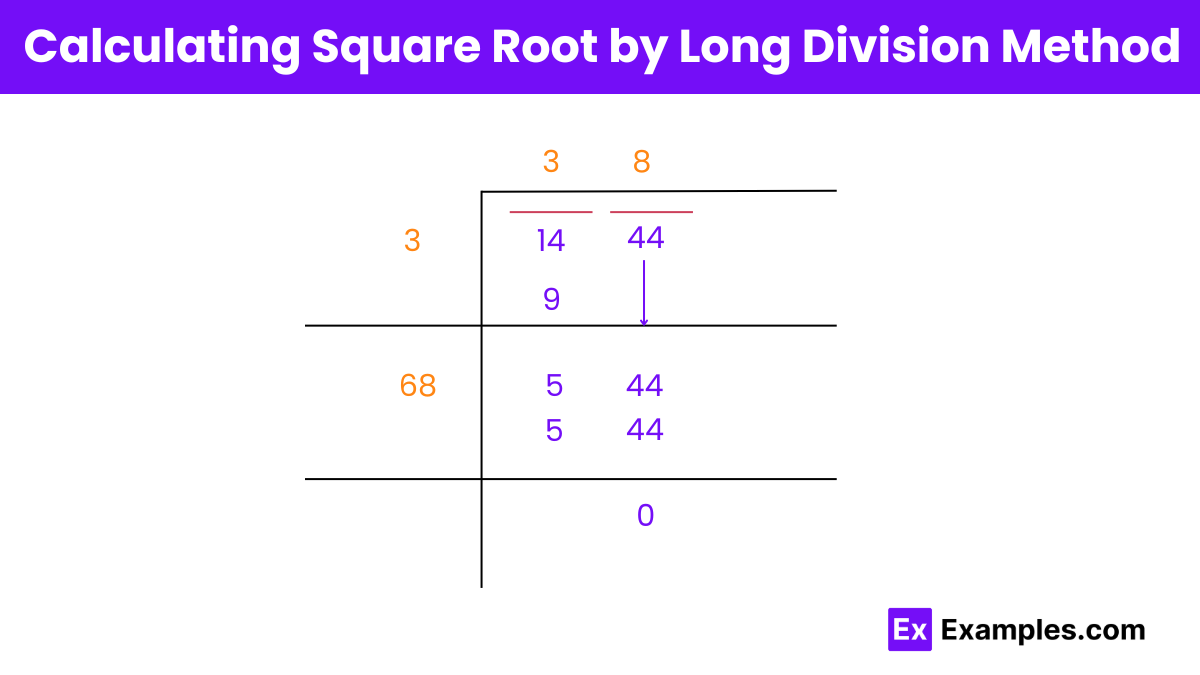
Pairing the Number:
- Divide the number 1444 into pairs from right to left. In this case, you have two pairs: 14 and 44.
Finding the First Digit of the Root:
- Look for a number Y whose square is less than or equal to the first pair (14). The largest such number is 3, because 3² = 9 and 4² = 16 (which is too large).
- Now, divide 14 by 3. The quotient is approximately 3 and the remainder is 14 – 3×3 = 14−9 = 5.
Form the Next Dividend:
- Bring down the next pair (44) next to the remainder 5 to form a new dividend, 544.
Updating the Divisor:
- Add the last digit of the current quotient (3) to the divisor (3), so 3+3 = 6.
- Find the largest digit Z such that when added to 6 as the tens place, and multiplied by itself, it is less than or equal to 544. The correct Z here is 8, because 68×8 = 544.
Perform the Division:
- Divide 544 by 68, resulting in a quotient of 8 and a remainder of 544−68×8 = 544−544 = 0.
Conclusion:
- Since the remainder is 0 and there are no more digits to bring down, the process stops here.
- The quotient, 38, is the square root of 1444.
1444 is Perfect Square root or Not
This calculation shows that 1444 is the result of squaring 38, thereby confirming it as a perfect square. This concept is used in various mathematical contexts, particularly in algebra and geometry, where recognizing perfect squares can help simplify computations and solve equations more effectively. Additionally, perfect squares play a role in number theory and are essential for understanding the properties of integers and their relationships.
FAQs
Why is understanding squares and square roots important in mathematics?
Understanding squares and square roots is crucial in mathematics for solving equations, understanding geometric concepts like area and volume, and analyzing patterns and relationships in numbers.
What are the applications of knowing the square and square root of numbers like 1444 in real life?
The Babylonian method, also known as the method of guess and check, is an ancient technique for finding square roots. It involves making an initial guess at the square root, then refining that guess by averaging the guess and the result of dividing the original number by the guess. For 1444, you would start with an initial guess (such as 37 or 39), compute 1444 divided by the guess, and average this quotient with the guess to get a new guess. Repeat until the guesses converge to 38.
Share :
