Ohms Law Formula – Formulas, Usage, Problems

What is Ohms Law Formula?

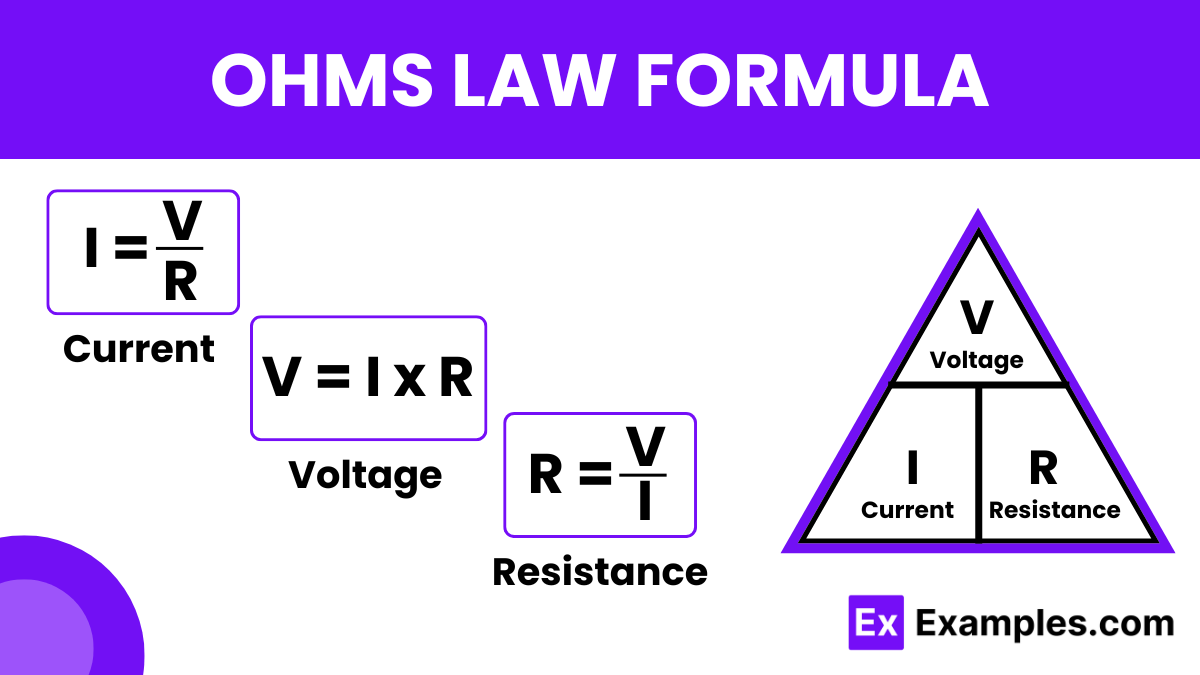
Ohm’s Law, a crucial concept in electrical physics, was discovered by Georg Simon Ohm, a German physicist, in 1827. This law provides a simple formula to understand the relationship between voltage, current, and resistance in an electrical circuit. The formula is expressed as:

• 𝐼 stands for current, measured in amperes (A),
• V represents voltage, measured in volts (V)
• R denotes resistance, measured in ohms (Ω).

The formula indicates that the current (𝐼) flowing through a conductor is directly proportional to the voltage (𝑉) applied across its ends and inversely proportional to the resistance (𝑅) of the conductor. This means that if you increase the voltage, the current will increase if the resistance remains constant. Similarly, if you increase the resistance, the current will decrease for a constant voltage.

To derive Ohm’s Law, consider a circuit where a voltage V is applied across a resistor R. The current 𝐼 that flows due to this voltage can be calculated by rearranging the formula to solve for I, leading to 𝐼 = 𝑉 / 𝑅​. This straightforward formula helps in designing and analyzing circuits by allowing calculations of how changes in voltage and resistance affect the current.

Different Formats of Ohms Law Formula

Calculating Voltage (V)

This formula helps to determine the voltage required across a component to produce a certain current flow, given the component’s resistance. It is particularly useful in power supply design, where maintaining specific voltage levels is crucial for device functionality. For instance, if you wish a current of 3 amperes to flow through a resistor of 4 ohms, the required voltage across the resistor would be 𝑉=3×4=12 volts.

Calculating Resistance (R)

​This formula is used to find the resistance of a component when the voltage across it and the current flowing through it are known. This formula is essential when designing circuits with specific resistance values to control current flow. For example, if a voltage of 20 volts results in a current of 4 amperes, the resistance of the circuit must be 𝑅=20 / 4=5 ohms.

Usage of Ohms Law Formula

1. Calculating Circuit Values: It helps in determining one of the three key electrical quantities—current, voltage, and resistance—when the other two are known. This is crucial for circuit design and troubleshooting.
2. Designing Electrical Devices: Engineers use Ohm’s Law to specify the required values of resistance in electronic components, ensuring devices operate safely under specified voltages.
3. Educational Tool: It is fundamental in educational settings for teaching basic and advanced concepts in electronics and electrical circuits.
4. Power Calculations: By combining Ohm’s Law with the power formula 𝑃=𝑉𝐼 (where P is power), one can calculate the power consumption in a circuit, which is vital for ensuring that electrical systems do not exceed their power handling capabilities.
5. Safety Assessments: It’s used to ensure that electrical installations like wiring in buildings have appropriate resistance values to handle expected voltage levels without overheating or causing electrical fires.
6. Battery-Operated Devices: In designing battery-operated gadgets like smartphones and laptops, Ohm’s Law helps in determining how long a device will operate on a charge by calculating the flow of current through the device’s components.
7. Automotive Systems: This law aids in the design and maintenance of automotive electrical systems, including the charging system, lighting, and audio systems, ensuring they function efficiently within the voltage and resistance parameters of the vehicle.
8. Electronic Circuit Design: Ohm’s Law is crucial in the development of resistive circuits in electronics, helping engineers design circuits with the right current flow for signal processing, power regulation, and other critical functions.
9. Heating Elements: Ohm’s Law is used to design heating elements in appliances like electric heaters and toasters, ensuring that the resistance is suitable for the voltage supply to produce the desired heat output without excessive energy consumption or risk of damage.

Problems on Ohms Laws

Problem 1: Calculating Current

Given: A 12-volt battery is connected across a resistor with a resistance of 6 ohms.

Question: What is the current flowing through the resistor?

Solution: Using Ohm’s Law 𝐼 = 𝑉 / R​,

𝐼=12/6 = 2 amperes

Thus, the current flowing through the resistor is 2 amperes.

Problem 2: Determining Voltage

Given: A current of 5 amperes flows through a circuit that includes a single resistor of 10 ohms.

Question: What is the voltage across the resistor?

Solution: Applying Ohm’s Law 𝑉 = 𝐼 x 𝑅, 𝑉=5×10=50 volts

Therefore, the voltage across the resistor is 50 volts.

Problem 3: Finding Resistance

Given: A circuit component has a current of 3 amperes flowing through it when a voltage of 15 volts is applied across it.

Question: What is the resistance of the component?

Solution: Using the formula 𝑅 = 𝑉 / 𝐼​, 𝑅=153=5 ohms

Hence, the resistance of the component is 5 ohms.

FAQs

Can Ohm’s Law be applied to all types of circuits?

Ohm’s Law applies to ohmic materials with constant resistance, not to non-ohmic materials like diodes.

What are the limitations of Ohm’s Law?

Ohm’s Law only applies to linear, ohmic components and fails with components that change resistance.

What happens if I double the voltage in a circuit?

Doubling the voltage while keeping resistance constant doubles the current, as per Ohm’s Law.