What is the square of 216?
46656
43200
58320
51840


Square root prime factorization is a method used in mathematics to break down a number into its prime factors to easily find its square and square root. This technique is particularly useful in algebra and number theory, where understanding the properties of integers and their relationships is crucial. The process also intersects with concepts of rational and irrational numbers, especially when determining if a square root is a perfect square or not. Additionally, the method ties into statistical analysis and data fitting through the least squares method, which minimizes the discrepancies between observed values and those predicted by a model. This approach is foundational in various branches of mathematics, including algebra, statistics, and arithmetic involving square and square roots.
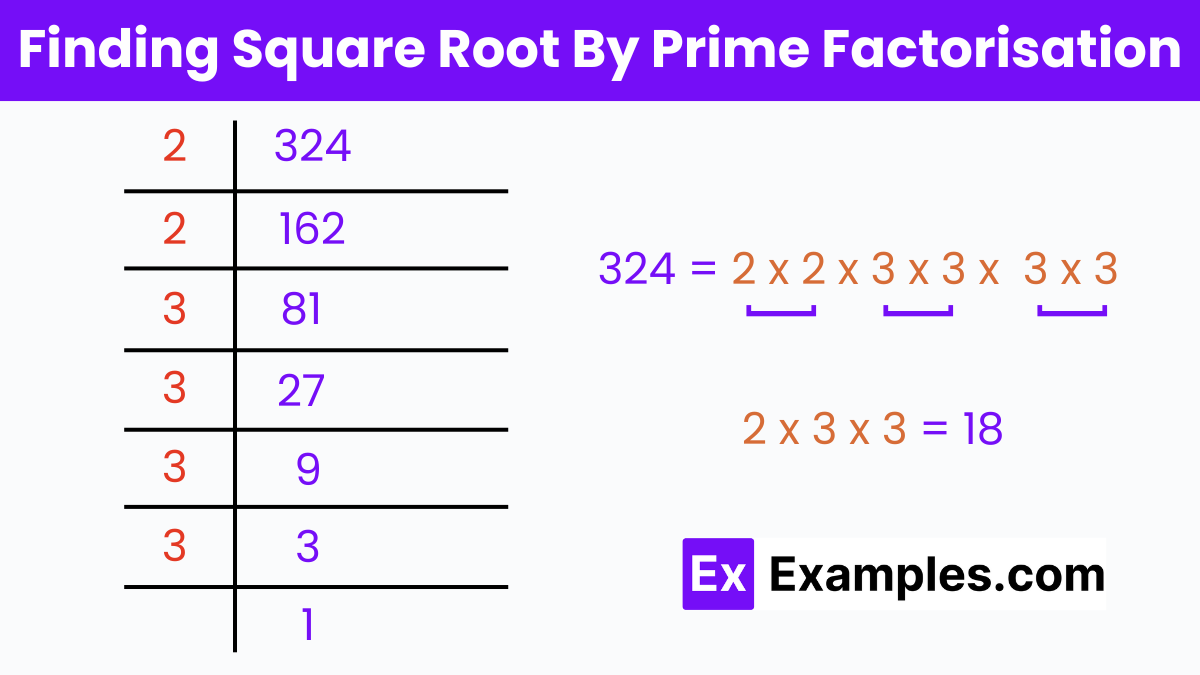
Finding the square root of a number through prime factorization is a systematic method that breaks down a number into its basic prime components. This process is especially useful when dealing with large numbers or when simplifying square roots in algebra. Below are detailed steps on how to find the square root by prime factorization:
Begin by dividing the number by the smallest prime number (usually 2) that can exactly divide it without leaving a remainder. Continue this process with the quotient until you reach a prime number. This will give you the prime factors of the number.
To factorize 72:
Arrange the prime factors in a visible format (like a list) and pair identical factors. If a factor does not have a pair, it remains single.
For each pair of identical primes, select one prime from the pair and multiply them. If a prime remains unpaired, it stays under the square root.
From the prime factors 2³×3²:
Multiply the results of the previous step together, and multiply this product by the square root of any leftover unpaired primes to find the square root of the original number.
First, factorize 144 into its prime components:
Since all factors are paired, the square root is:
Factorize 200:
Prime factors are 2²×5².
The prime factorization method involves breaking down a number into its prime factors, pairing them, and using these pairs to simplify the extraction of the square root. This method is especially useful for large numbers and helps in determining whether the square root is a perfect square or involves irrational numbers.
A number is a perfect square if all its prime factors can be paired without any remainder. During the factorization process, if every prime factor can be grouped into identical pairs, then the number is a perfect square. If any prime factor remains unpaired, the number is not a perfect square.
Yes, the prime factorization method can be used for any positive integer. However, the method is most effective for numbers where prime factors can be easily determined. For very large numbers or numbers involving large prime factors, the process can be time-consuming without the aid of computational tools.
Yes, the prime factorization method can also be applied to algebraic expressions involving square roots. Factors in polynomial expressions that are perfect squares can be treated similarly to numerical prime factors, allowing for simplification of square roots in algebra.
Unpaired prime factors remain under the square root symbol in the final expression. If a prime factor does not have a pair, it indicates that the square root of the number will be an irrational number, and the unpaired prime factor will be part of the radical expression.

Square root prime factorization is a method used in mathematics to break down a number into its prime factors to easily find its square and square root. This technique is particularly useful in algebra and number theory, where understanding the properties of integers and their relationships is crucial. The process also intersects with concepts of rational and irrational numbers, especially when determining if a square root is a perfect square or not. Additionally, the method ties into statistical analysis and data fitting through the least squares method, which minimizes the discrepancies between observed values and those predicted by a model. This approach is foundational in various branches of mathematics, including algebra, statistics, and arithmetic involving square and square roots.
Square Root Prime Factorization is a mathematical process used to determine the square root of a number by first breaking it down into its prime factors. This method simplifies the extraction of roots, particularly when dealing with large numbers, by utilizing the fundamental properties of primes.
Finding the square root of a number through prime factorization is a systematic method that breaks down a number into its basic prime components. This process is especially useful when dealing with large numbers or when simplifying square roots in algebra. Below are detailed steps on how to find the square root by prime factorization:
Begin by dividing the number by the smallest prime number (usually 2) that can exactly divide it without leaving a remainder. Continue this process with the quotient until you reach a prime number. This will give you the prime factors of the number.
To factorize 72:
72÷2 = 36
36÷2 = 18
18÷2 = 9
9÷3 = 3
3÷3 = 1
Prime factors of 72 are: 2³×3²
Arrange the prime factors in a visible format (like a list) and pair identical factors. If a factor does not have a pair, it remains single.
For each pair of identical primes, select one prime from the pair and multiply them. If a prime remains unpaired, it stays under the square root.
From the prime factors 2³×3²:
Pairs are: (2,2),(2),(3,3)
Multiply one element from each pair: 2×3
Multiply the results of the previous step together, and multiply this product by the square root of any leftover unpaired primes to find the square root of the original number.
Paired result is 2×3 = 6
Unpaired prime is 2
The square root of 72 is 6×√2 or 6√2.
First, factorize 144 into its prime components:
Divide by 2: 144÷2 = 72
Divide by 2: 72÷2 = 36
Divide by 2: 36÷2 = 18
Divide by 2: 18÷2 = 9
Divide by 3: 9÷3 = 3
Divide by 3: 3÷3 = 1
Prime factors are 2⁴×3².
Pairs: (2,2),(2,2),(3,3)
Multiply one from each pair: 2×2×3 = 12
Since all factors are paired, the square root is:
√144 = 12
Factorize 200:
Divide by 2: 200÷2 = 100
Divide by 2: 100÷2 = 50
Divide by 2: 50÷2 = 25
Divide by 5: 25÷5 = 5
5 is a prime number.
Prime factors are 2²×5².
Pairs: (2,2),(5,5)
Unpaired: 2
Multiply one from each pair: 2×5 = 10
Since there is an unpaired factor, the square root is:
√200=10√2.
The prime factorization method involves breaking down a number into its prime factors, pairing them, and using these pairs to simplify the extraction of the square root. This method is especially useful for large numbers and helps in determining whether the square root is a perfect square or involves irrational numbers.
A number is a perfect square if all its prime factors can be paired without any remainder. During the factorization process, if every prime factor can be grouped into identical pairs, then the number is a perfect square. If any prime factor remains unpaired, the number is not a perfect square.
Yes, the prime factorization method can be used for any positive integer. However, the method is most effective for numbers where prime factors can be easily determined. For very large numbers or numbers involving large prime factors, the process can be time-consuming without the aid of computational tools.
Yes, the prime factorization method can also be applied to algebraic expressions involving square roots. Factors in polynomial expressions that are perfect squares can be treated similarly to numerical prime factors, allowing for simplification of square roots in algebra.
Unpaired prime factors remain under the square root symbol in the final expression. If a prime factor does not have a pair, it indicates that the square root of the number will be an irrational number, and the unpaired prime factor will be part of the radical expression.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 216?
46656
43200
58320
51840
Which of the following is the square root of 216?
14.7
15
16
14.5
What is the result of squaring the square root of 216?
216
432
108
324
If the square root of a number is 14.7, what is the approximate square of this root?
216
215
220
225
What is the approximate value of the square root of 216?
14.6
14.7
14.8
14.9
What is 216 raised to the power of 2?
46656
43200
58320
51840
What is the value of (√216)²?
216
432
108
324
Which number squared gives 216?
14.7
14
15
16
What is the nearest whole number to the square root of 216?
14
15
16
17
What is 216 divided by its square root?
14.7
15
14
13
Before you leave, take our quick quiz to enhance your learning!

