Arbitrage, Replication, and the Cost of Carry in Pricing Derivatives are foundational concepts that play a crucial role in determining the fair value of derivative instruments. Arbitrage ensures that price discrepancies across markets are quickly corrected, promoting market efficiency. Replication involves constructing a portfolio that mimics the payoff of a derivative, allowing for precise valuation through synthetic positions. The cost of carry, which includes costs and benefits of holding an asset, adjusts derivative prices based on financing rates, storage, dividends, or yields, ensuring consistent pricing across markets.

Free CFA Practice Test

Learning Objectives

In studying “Arbitrage, Replication, and the Cost of Carry in Pricing Derivatives” for the CFA Exam, you should learn to understand the key principles that underpin derivative pricing. Analyze how arbitrage opportunities create no-arbitrage pricing boundaries and explore replication strategies to determine fair values for derivative instruments. Evaluate the impact of cost of carry—incorporating interest rates, storage costs, and income yields—on forward and futures prices. Additionally, apply these concepts to real-world scenarios to assess how derivatives are priced and valued in financial markets, and interpret calculations involving arbitrage-free pricing and cost of carry in CFA practice questions.

1. Arbitrage in Derivative Pricing

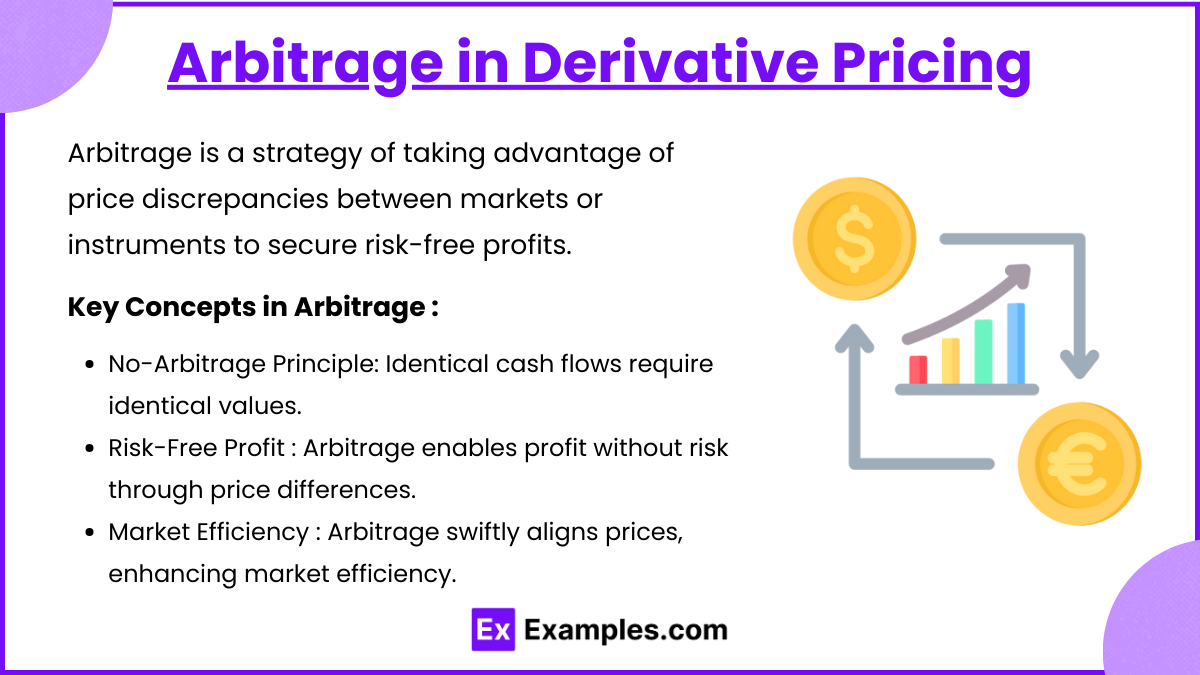
Arbitrage is a strategy of taking advantage of price discrepancies between markets or instruments to secure risk-free profits. Arbitrage plays a critical role in derivative pricing, as the principle of no-arbitrage underpins most pricing models.

Key Concepts in Arbitrage:

No-Arbitrage Principle: This principle asserts that if two portfolios produce identical cash flows, they must have the same value. If this condition is violated, arbitrageurs would exploit the price difference, leading the prices to converge.
Risk-Free Profit: Arbitrage allows for the possibility of generating a profit with no risk by simultaneously buying and selling equivalent assets at different prices.
Market Efficiency: Arbitrage opportunities are quickly exploited by market participants, pushing prices toward fair values and creating efficiency.

Arbitrage Example in Futures Pricing:

For example, if a futures contract on an asset is mispriced relative to the spot price and the cost of carry, an arbitrageur can:

Buy the asset in the spot market.
Simultaneously sell the corresponding futures contract.
Hold the asset until the futures contract’s expiration, earning a risk-free profit as the prices realign.

Arbitrage opportunities like this are typically short-lived in liquid markets due to competitive trading, reinforcing the no-arbitrage condition in derivative pricing.

2. Replication in Derivative Pricing

Replication involves creating a portfolio that mimics the payoff of a derivative by combining other assets. This approach is essential in derivative pricing, as it allows for pricing complex derivatives based on the cost of replicating their payoffs using simpler instruments.

Key Concepts in Replication:

Synthetic Positions: By holding a combination of assets and/or other derivatives, investors can create synthetic positions that replicate the payoffs of the desired derivative.
Pricing by Replication: The price of a derivative should equal the cost of a portfolio that replicates its cash flows. This concept is fundamental in options pricing models, like the Black-Scholes model.
Applications in Options: For example, a call option on a stock can be replicated by purchasing the underlying stock and taking a short position in a bond. This synthetic position mirrors the payoff of the call option, enabling the derivation of its fair price.

Replication Example:

For a call option on a stock, the replication portfolio would consist of:

Buying a fraction of the stock (delta).
Borrowing the remaining amount, creating a portfolio that behaves like the option.

By matching the payoffs, this portfolio allows for the calculation of the option’s price, providing an arbitrage-free valuation based on replication.

3. Cost of Carry in Derivative Pricing

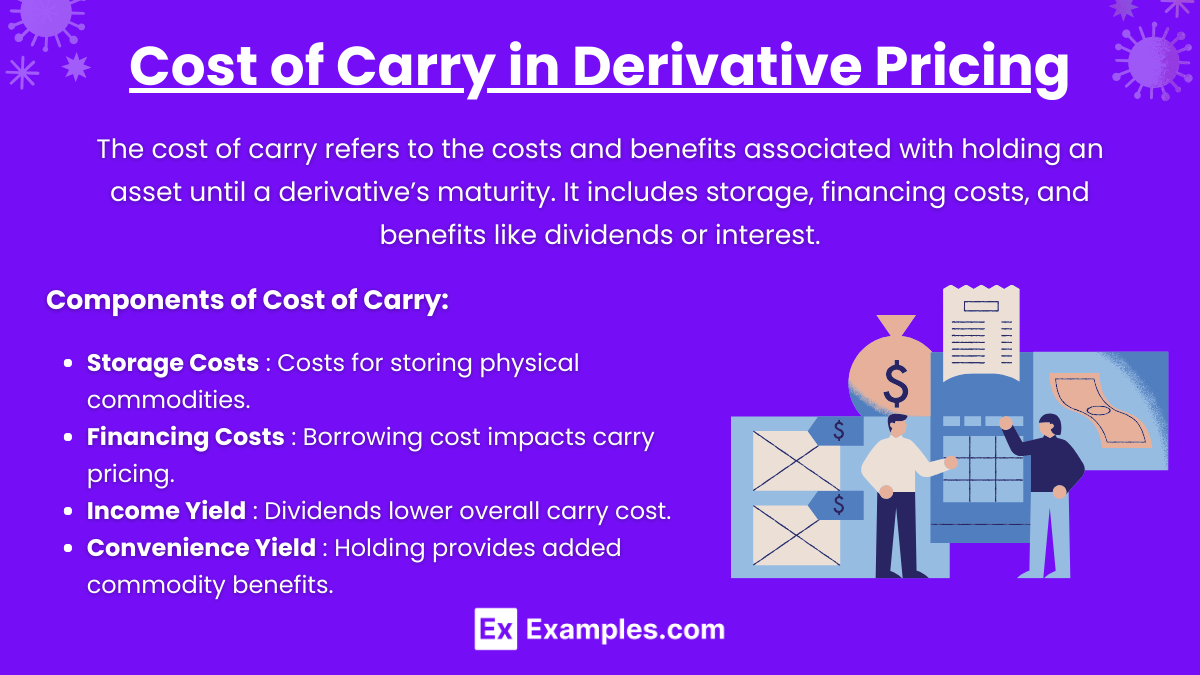
The cost of carry refers to the costs and benefits associated with holding an asset until a derivative’s maturity. It includes storage, financing costs, and benefits like dividends or interest. The cost of carry is crucial in pricing forward and futures contracts, as it affects the relationship between the spot and future prices of an asset.

Components of Cost of Carry:

Storage Costs: Physical commodities, such as oil or gold, may incur storage costs, which are factored into the derivative’s price.
Financing Costs: The cost of borrowing funds to hold an asset (typically represented by the risk-free rate) is a primary component of cost of carry.
Income Yield: Assets that provide dividends or other income streams, like stocks or bonds, reduce the cost of carry because they generate cash flow benefits for the holder.
Convenience Yield: For commodities, there may be an additional benefit (convenience yield) to holding the physical asset, such as immediate availability for production, which can impact pricing.

Cost of Carry Formula for Futures Pricing:

The fair price of a futures contract can be calculated using the cost of carry model:

Futures Price = Spot Price × e ^(r+s−y)T

Where:

r = Risk-free interest rate (financing cost)
s = Storage costs
y = Income yield (e.g., dividends)
T = Time to maturity

The futures price will adjust based on the combined costs and benefits of holding the underlying asset, balancing the spot and futures markets through the cost of carry.

Examples

Example 1: Arbitrage in Futures Pricing

Suppose the current spot price of gold is 1,800 dollars per ounce, while the price of a futures contract expiring in one year is 1,900 dollars. If the risk-free rate is 5% and there are no storage costs, the futures price should theoretically be $1,800 × e^0.05, or approximately 1,890 dollars. The futures price of 1,900 dollars suggests an arbitrage opportunity. An arbitrageur could buy gold at 1,800 dollars, sell the futures at 1,900 dollars, and finance the purchase at 5%, securing a risk-free profit as the futures and spot prices converge at expiration.

Example 2: Cost of Carry in Commodity Futures

For a commodity like oil, storage costs are substantial. If the spot price of oil is $50 per barrel, with a storage cost of 2% annually, and the risk-free rate is 3%, the cost of carry includes both storage and financing costs. The futures price, accounting for these costs, is calculated as 50×e^(0.03+0.02), approximately $52.53. This cost of carry model helps determine the fair price of the oil futures, reflecting the full cost of holding the physical commodity.

Example 3: Replication in Options Pricing

To replicate a call option on a stock, an investor can create a synthetic position by buying a fraction of the stock (delta) and financing the remaining position with a short position in risk-free bonds. For example, if a stock has a current price of 100 dollars and the delta of the call option is 0.6, the investor would buy 0.6 shares and borrow 40 dollars (assuming a 100 dollars call option). This portfolio replicates the call option’s payoff and allows for the calculation of the call’s fair price using the replication approach.

Example 4: Arbitrage in Interest Rate Parity

Consider a scenario in which the interest rates in the U.S. and Europe are 2% and 1%, respectively, and the spot exchange rate for USD/EUR is 1.20. According to interest rate parity, the forward rate should equal 1.20 × e^{(0.02−0.01)}, or approximately 1.212. If the forward rate offered in the market is 1.215, an arbitrage opportunity exists. An arbitrageur could borrow in euros, convert to dollars, invest in the U.S., and enter a forward contract to lock in a profit when rates converge.

Example 5: Cost of Carry with Dividend-Paying Stocks

Suppose the spot price of a stock is $200, with a risk-free rate of 4% and an annual dividend yield of 2%. The cost of carry must factor in the income generated by the dividends, reducing the overall cost of holding the stock. The futures price, incorporating this dividend yield, is 200 × e^{(0.04−0.02)}, or approximately $204. This calculation shows how the cost of carry, adjusted for income from dividends, impacts the fair value of stock futures.

Practice Questions

Question 1

Suppose the current spot price of a commodity is $100, the risk-free interest rate is 5% per annum, and the cost of storage for holding the commodity is 2% per annum. Assuming no income yield, what is the theoretical futures price for a one-year futures contract on this commodity?

A. $105
B. $107
C. $102
D. $107.10

Answer: B. $107

Explanation: The cost of carry model for pricing futures is calculated as:

Futures Price = Spot Price × e^(r+s−y)T

Where:

Spot Price = $100
r = Risk-free interest rate = 5%
s = Storage cost = 2%
y = Income yield = 0% (none given)
T = Time to maturity = 1 year

Plugging in the values:

Futures Price = 100 × e^{(0.05+0.02)×1} = 100 × e^0.07 ≈ 100 × 1.0725 = 107.25

Rounding to two decimal places, the theoretical futures price is approximately $107.

Question 2

An investor replicates a call option by buying 0.6 shares of the underlying stock and borrowing $40. Which of the following is true about this replication strategy?

A. The investor is creating a synthetic call option position.
B. The investor is hedging against a decrease in the stock’s price.
C. The investor is exposed only to downside risk.
D. The investor is creating a protective put strategy.

Answer: A. The investor is creating a synthetic call option position.

Explanation: Replicating a call option involves creating a synthetic position that mimics the payoff of a call option. This replication is achieved by buying a fraction of the stock (equal to the option’s delta, in this case, 0.6) and borrowing the remainder to cover the strike price. This strategy replicates the payoff of a call option, where the investor benefits if the stock price rises but has limited downside risk due to the borrowed funds. Therefore, option A is correct. Option B is incorrect because the investor is exposed to upside potential, not primarily hedging. Option C is also incorrect because a call option replication includes both upside and downside exposure, and D is incorrect since a protective put involves buying a stock and purchasing a put option, not borrowing.

Question 3

The current exchange rate for USD/EUR is 1.15. The interest rate in the U.S. is 2% and in Europe, it is 1%. According to interest rate parity, what should the one-year forward exchange rate for USD/EUR be?

A. 1.1485
B. 1.1615
C. 1.1620
D. 1.1370

Answer: B. 1.1615

Explanation: The interest rate parity formula is used to calculate the forward exchange rate based on differences in interest rates:

Forward Rate = Spot Rate × ^{e(rdomestic−rforeign)T}

Where:

Spot Rate = 1.15
rdomestic = U.S. interest rate = 2%
rforeign = Euro interest rate = 1%
T = Time to maturity = 1 year

Plugging in the values:

Forward Rate = 1.15 × e^{(0.02−0.01)} × 1 = 1.15 × e^0.01 ≈ 1.15 × 1.01005 = 1.1615.

Thus, the correct forward rate is approximately 1.1615, making B the correct answer.