Kinetic Molecular Theory

Last Updated: September 24, 2024

Notes

Learning Objectives

When studying the Kinetic Molecular Theory (KMT) for the AP Chemistry exam, focus on understanding its five key postulates and how they explain the behavior of ideal gases. This includes gas particles in constant motion, negligible volume, no intermolecular forces, kinetic energy proportional to temperature, and pressure resulting from collisions with container walls. Relate these postulates to macroscopic properties such as pressure, temperature, and volume, and explain the gas laws (Boyle’s, Charles’s, and Avogadro’s) using KMT. Understand deviations from ideal behavior at low temperatures and high pressures, and learn to use the Van der Waals equation. Grasp diffusion and effusion concepts, apply Graham’s Law, and analyze the Maxwell-Boltzmann distribution of molecular speeds. Develop problem-solving skills by solving numerical problems with the Ideal Gas Law, interpreting relevant graphs, and proposing experiments to test gas behavior hypotheses. Synthesize KMT knowledge with thermodynamics and kinetics, and explain how experimental evidence supports KMT.

Free AP Chemistry Practice Test

Introduction

The Kinetic Molecular Theory (KMT) is a fundamental model in chemistry that explains the behavior of gases through the motion of their particles. It posits that gas particles are in constant, random motion and collide elastically with each other and the walls of their container. The theory assumes the volume of individual gas particles is negligible compared to the container’s volume and that there are no intermolecular forces acting between them. Additionally, the average kinetic energy of gas particles is directly proportional to the absolute temperature, providing a comprehensive framework for understanding gas laws and behaviors.

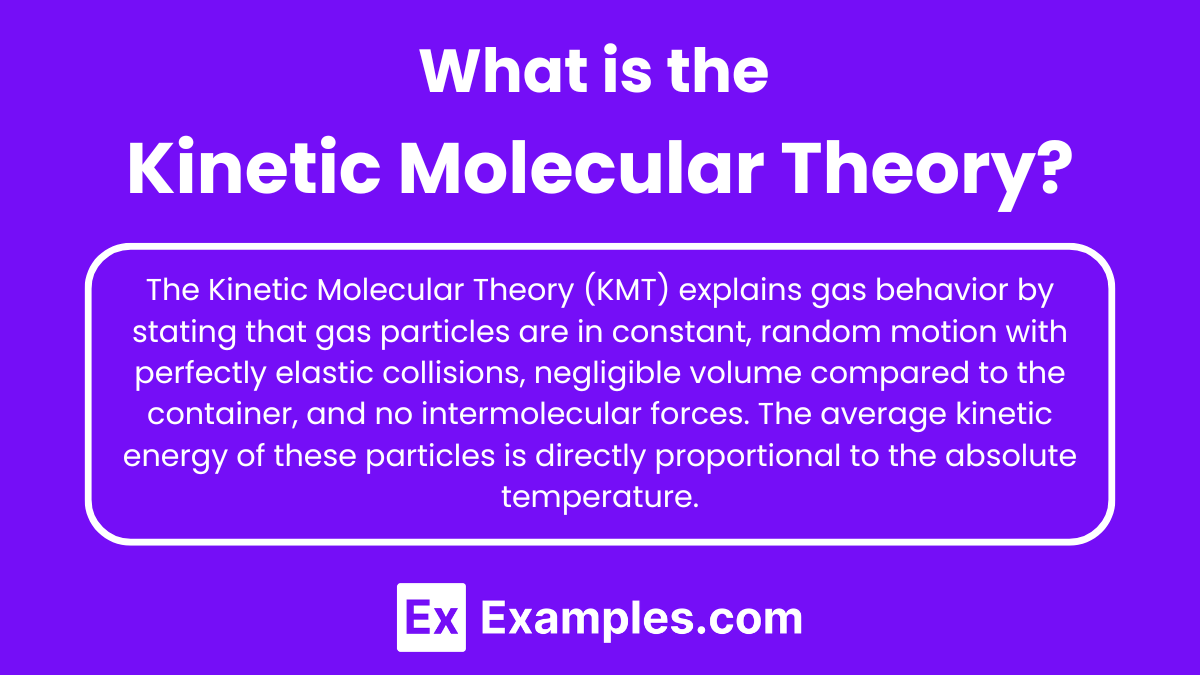
What is the Kinetic Molecular Theory?

The Kinetic Molecular Theory (KMT) is a model that explains the behavior of gases in terms of the motion of their particles. It states that gas particles are in constant, random motion, and their collisions with each other and the walls of their container are perfectly elastic. The theory assumes that the volume of individual gas particles is negligible compared to the volume of the container, and that there are no intermolecular forces acting between them. The average kinetic energy of the gas particles is directly proportional to the absolute temperature of the gas.

Key Postulates of Kinetic Molecular Theory

Gas Particles are in Constant Motion
- Gas particles are always moving in straight lines until they collide with each other or the walls of their container.
- These collisions are elastic, meaning there is no net loss of kinetic energy.
Negligible Particle Volume
- The volume of individual gas particles is negligible compared to the volume of the container.
- This implies that gas particles are very small and the space between them is large relative to their size.
No Intermolecular Forces
- There are no attractive or repulsive forces between gas particles.
- Each particle moves independently of the others.
Kinetic Energy and Temperature
- The average kinetic energy of gas particles is directly proportional to the absolute temperature (measured in Kelvin).
- As temperature increases, the kinetic energy and, thus, the speed of the gas particles increase.
Pressure and Collisions
- The pressure exerted by a gas in a container results from collisions between the gas particles and the container walls.
- The more frequent and forceful these collisions, the higher the pressure.

Gas Laws and Kinetic Molecular Theory

Boyle’s Law (Pressure-Volume Relationship)

Statement: Boyle’s law states that at constant temperature, the volume of a gas is inversely proportional to its pressure.

Mathematical Expression: $\text{P} \times \text{V} = \text{constant}$

KMT Explanation: According to KMT, gas particles are in constant motion and collide with the walls of their container, exerting pressure. When the volume of the container decreases, the gas particles have less space to move around, resulting in more frequent collisions with the walls. This increase in collision frequency leads to an increase in pressure. Thus, as volume decreases, pressure increases, and vice versa, assuming temperature remains constant.

Charles’s Law (Temperature-Volume Relationship)

Statement: At constant pressure, the volume of a gas is directly proportional to its absolute temperature.

Mathematical Expression: $\frac{V}{T} = \text{constant}$

KMT Explanation: The average kinetic energy of gas particles is directly proportional to temperature. As temperature increases, gas particles move faster and with greater energy. To maintain constant pressure, the volume must increase to allow the gas particles more space to move and to keep the frequency of collisions with the container walls constant. Therefore, as temperature increases, volume increases, and vice versa, assuming pressure remains constant.

Avogadro’s Law (Volume-Mole Relationship)

Statement: At constant temperature and pressure, the volume of a gas is directly proportional to the number of moles of gas.

Mathematical Expression: $\frac{V}{n} = \text{constant}$

KMT Explanation: Adding more gas particles (increasing the number of moles) at constant temperature and pressure means more particles will collide with the container walls. To keep the pressure constant, the volume must increase so that the gas particles have more space to move and the frequency of collisions remains the same. Thus, as the number of moles increases, the volume increases.

Ideal Gas Law

Statement: The state of an ideal gas is described by the equation PV=nRT, where R is the gas constant.

KMT Explanation: The Ideal Gas Law combines the relationships described by Boyle’s, Charles’s, and Avogadro’s laws. It provides a comprehensive model for gas behavior, where pressure, volume, temperature, and the number of moles of gas are all interrelated. According to KMT, gas particles are in constant, random motion, and their collisions with the container walls generate pressure. The temperature of the gas affects the kinetic energy of the particles, influencing both pressure and volume.

Real Gases vs. Ideal Gases

Ideal Gases

Definition: Ideal gases are hypothetical gases that perfectly follow the Kinetic Molecular Theory and the Ideal Gas Law under all conditions.

Characteristics:

No Intermolecular Forces: Ideal gases are assumed to have no attractive or repulsive forces between particles.
Negligible Particle Volume: The volume of individual gas particles is negligible compared to the volume of the container.
Elastic Collisions: Collisions between gas particles and with the container walls are perfectly elastic, meaning no kinetic energy is lost in collisions.
Behavior: Ideal gases strictly obey the gas laws (Boyle’s Law, Charles’s Law, Avogadro’s Law, and the Ideal Gas Law) under all conditions of temperature and pressure.

Real Gases

Definition: Real gases are actual gases that do not always perfectly follow the Ideal Gas Law, especially under certain conditions.

Characteristics:

Intermolecular Forces: Real gases have intermolecular attractions (Van der Waals forces) and repulsions between particles, which become significant at low temperatures and high pressures.
Finite Particle Volume: The volume of gas particles is not negligible and becomes significant at high pressures.
Non-Elastic Collisions: Collisions between particles and with container walls may result in some energy loss, deviating from perfect elasticity.

Deviations from Ideal Behavior

Low Temperature:

At low temperatures, gas particles move more slowly, which allows intermolecular forces to have a greater effect. Attractive forces between particles can cause the gas to condense into a liquid, leading to deviations from ideal behavior.

High Pressure:

At high pressures, gas particles are compressed into a smaller volume, making the volume of the particles themselves significant compared to the volume of the container. This reduces the free space available for particle movement and increases the effect of intermolecular forces, causing deviations from ideal gas behavior.

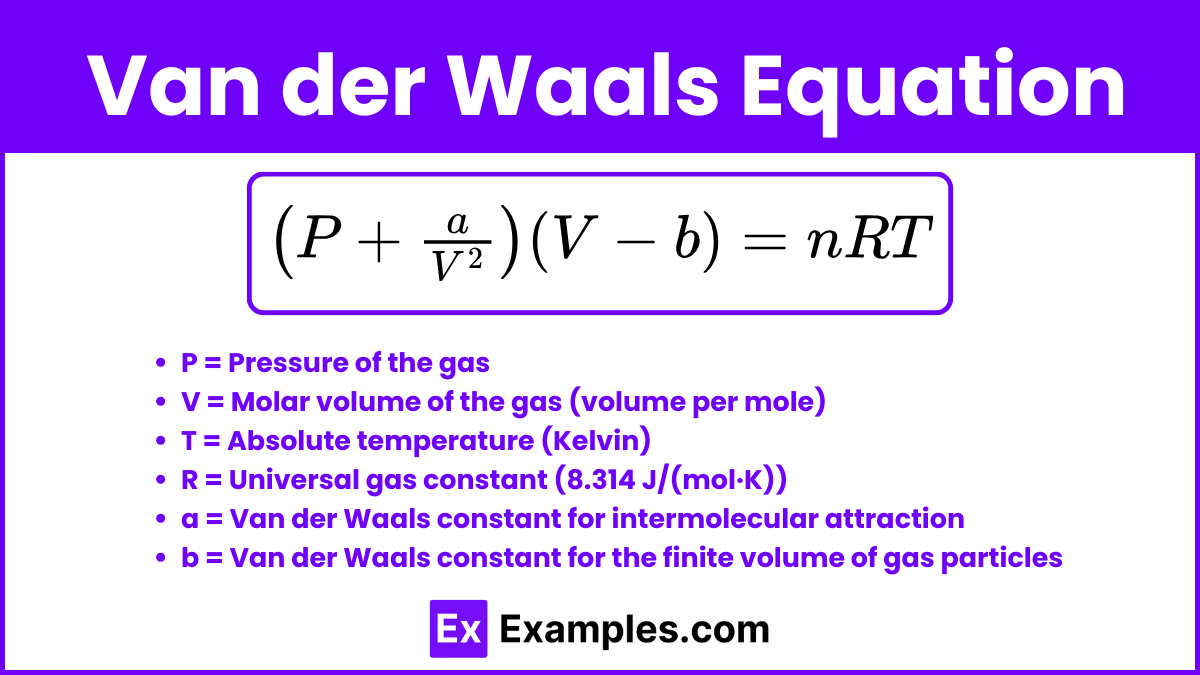
Van der Waals Equation

The Van der Waals equation is a modified version of the Ideal Gas Law that accounts for the behavior of real gases. It introduces correction factors to address the two main assumptions of the Ideal Gas Law that do not hold true for real gases: the lack of intermolecular forces and the negligible volume of gas particles.

Van der Waals Equation

$\left( P + \frac{a}{V^2} \right) (V - b)$ = nRT

Where:

P = Pressure of the gas
V = Molar volume of the gas (volume per mole)
T = Absolute temperature (Kelvin)
R = Universal gas constant (8.314 J/(mol·K))
a = Van der Waals constant for intermolecular attraction
b = Van der Waals constant for the finite volume of gas particles

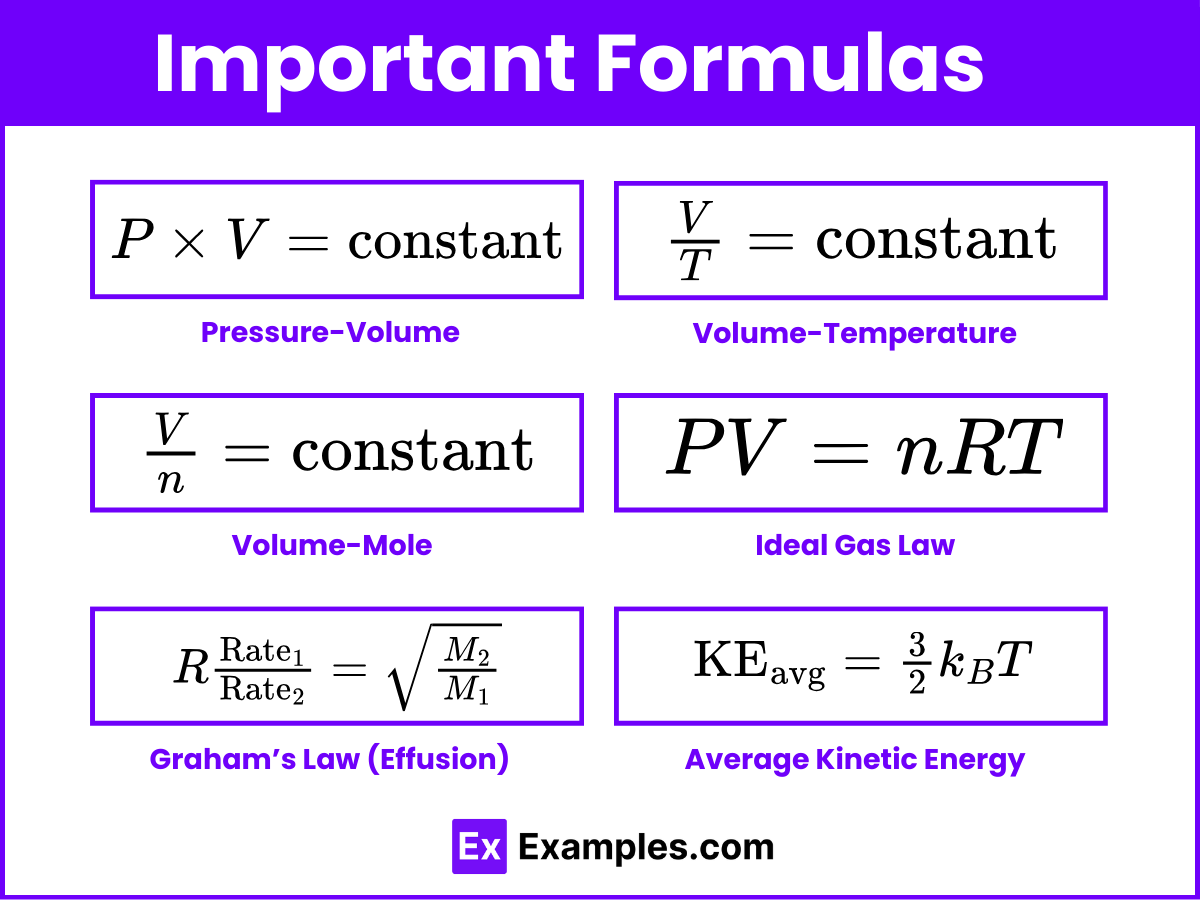
Important Formulas and Constants

Quantity	Formula/Expression
Pressure-Volume	$P \times V = \text{constant}$
Volume-Temperature	$\frac{V}{T} = \text{constant}$
Volume-Mole	$\frac{V}{n} = \text{constant}$
Ideal Gas Law	PV = nRT
Graham’s Law (Effusion)	$R\frac{\text{Rate}_1}{\text{Rate}_2} = \sqrt{\frac{M_2}{M_1}}$
Average Kinetic Energy	$\text{KE}_{\text{avg}} = \frac{3}{2} k_B T$

Gas Constant (R): 0.0821 L·atm/mol·K or 8.314 J/mol·K
Boltzmann Constant (k_B): 1.38 × 10⁻²³ J/K

Applications of Kinetic Molecular Theory

Diffusion and Effusion

Diffusion: The process by which gas particles spread out to evenly fill a container.
- Graham’s Law of Diffusion: The rate of diffusion of a gas is inversely proportional to the square root of its molar mass.
Effusion: The process by which gas particles pass through a tiny opening.
- Graham’s Law of Effusion: The rate of effusion of a gas is inversely proportional to the square root of its molar mass.

Maxwell-Boltzmann Distribution

Describes the distribution of speeds among gas particles at a given temperature.
Key Points:
- Not all gas particles move at the same speed.
- The distribution shifts to higher speeds at higher temperatures.