Find the derivative of f(x) = 3x²+ 4x + 5.
6x+4
6x+5
3x²+4
3x+4

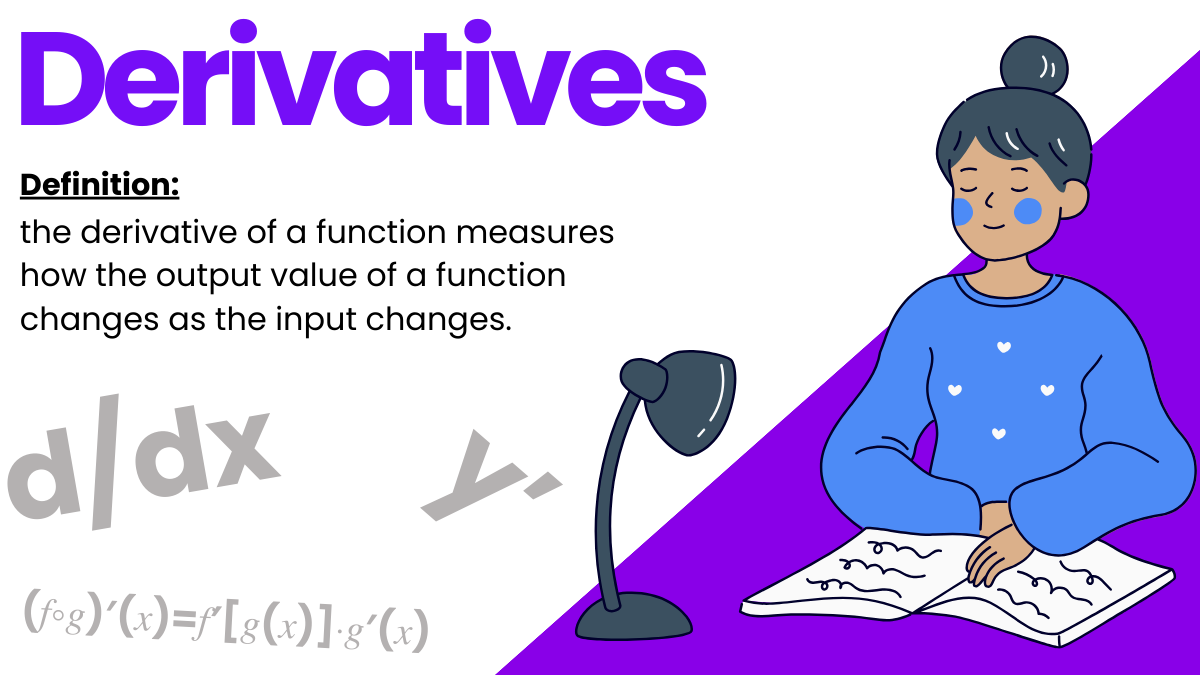
In simple terms, the derivative of a function measures how the output value of a function changes as the input changes. It is often represented as the slope of the tangent line at any point of the function graph. The derivative at a point tells us the rate at which the function’s value is changing at that point.
Derivatives are an essential component of calculus, serving as a powerful tool to measure the sensitivity of one quantity in relation to changes in another. This concept is foundational in analyzing how functions change, which is vital for modeling dynamic systems across various scientific and mathematical disciplines.
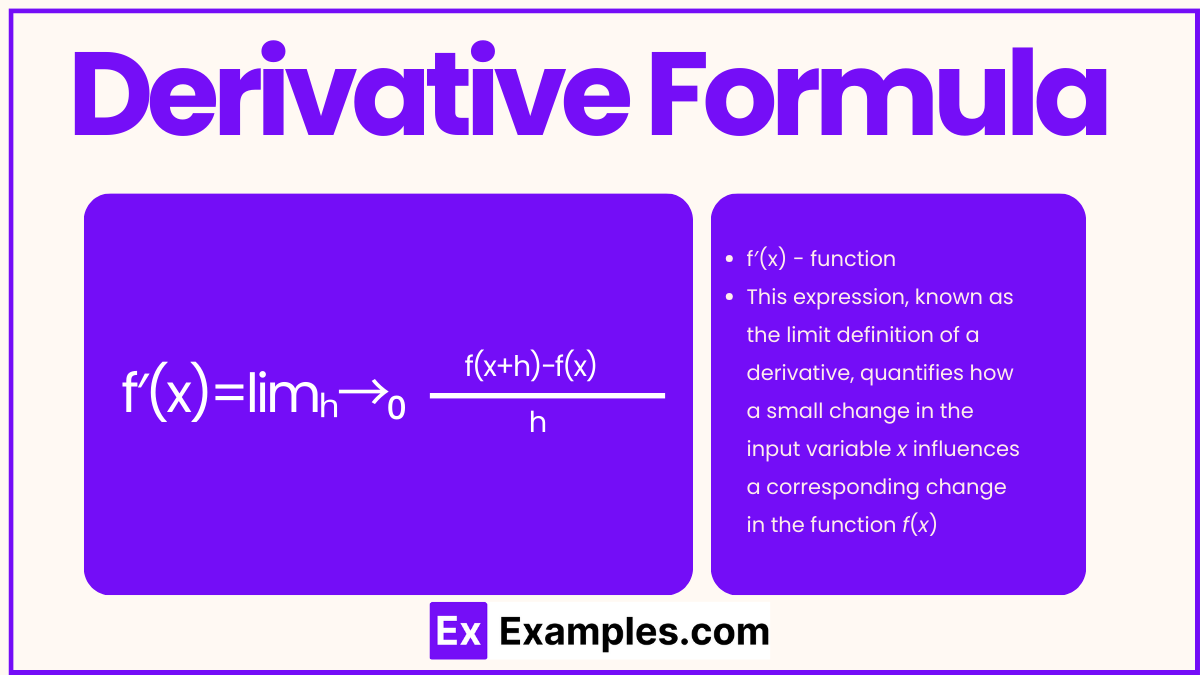
The formula for the derivative of a function with respect to a real variable can be expressed mathematically as:
The three foundational categories of derivatives—algebraic, logarithmic/exponential, and trigonometric—are derived from the first principles of differentiation. These categories, which include derivatives of algebraic expressions, logarithms, exponents, and trigonometric functions, form the core of standard derivative formulas, crucial tools in calculus. Each type serves a specific function and is derived by applying the fundamental concept of differentiation. This comprehensive breakdown highlights the essential nature of these derivatives in various mathematical and real-world applications
Using the example above, the derivative of 𝑥² is 2𝑥. Following this pattern, we can also determine that the derivative of 𝑥³ is 3𝑥², and the derivative of 𝑥⁴ is 4𝑥³ This observation leads us to the Power Rule in differentiation, which generalizes this concept for any power of 𝑥x. The Power Rule is formally stated as:
d/dx(xⁿ)=nxⁿ⁻¹
The derivative of ln x is, d/dx (ln x) = 1/x
The derivative of log x is, d/dx (logₐ x) = 1/(x ln a)
The derivative of e^x is, d/dx (eˣ) = eˣ
The derivative of a^x is, d/dx (aˣ) = aˣ ln a
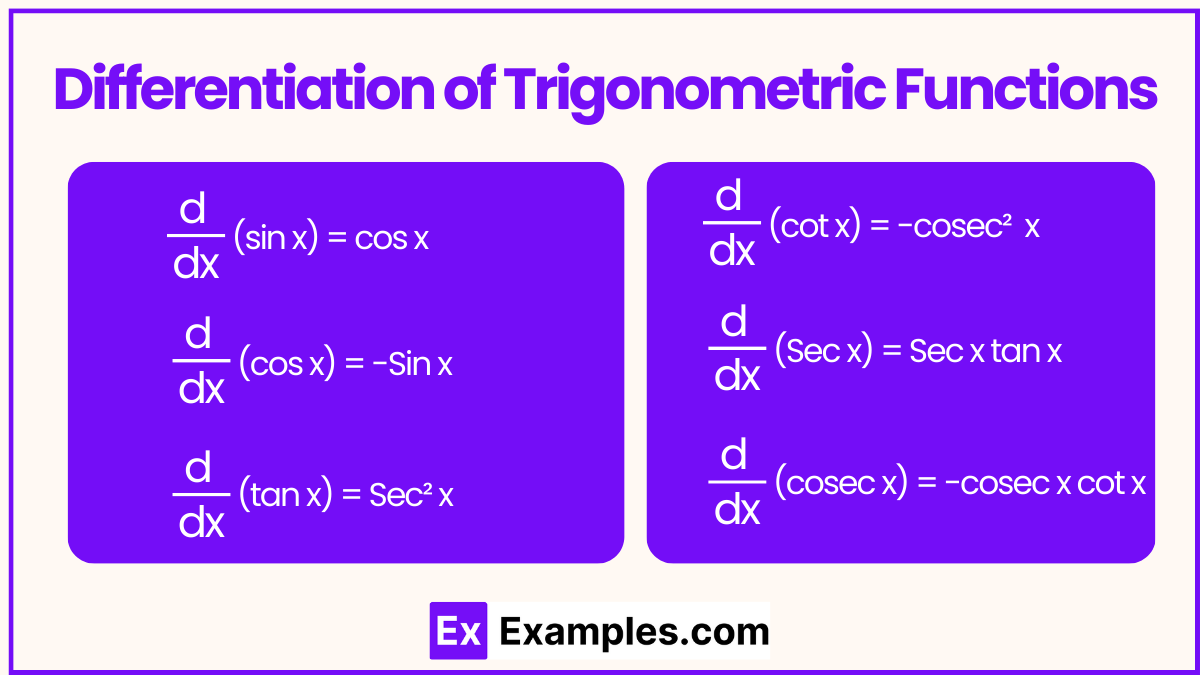
If y = sin x, y’ = cos x
If y = cos x, y’ = -sin x
If y = tan x, y’ = sec² x
If y = cot x, y’ = -cosec² x
If y = sec x, y’ = sec x tan x
If y = cosec x, y’ = -cosec x cot x
Here are the derivatives of trigonometric functions

The derivative of inverse sine is, d/dx (sin⁻¹x) = 1/√(1-x²)
The derivative of inverse cosine is, d/dx (cos⁻¹x) = -1/√(1-x²)
The derivative of inverse tan is, d/dx (tan⁻¹x) = 1/(1 + x²)
The derivative of inverse cot is, d/dx (cot⁻¹x) = -1/(1 + x²)
The derivative of inverse cosec is, d/dx (csc⁻¹x) = -1/ [|x| √(x² – 1) ], x ≠ 1, -1, 0
The derivative of inverse sec is, d/dx (sec⁻¹x) = 1/ [|x| √(x² – 1) ], x ≠ 1, -1, 0
The following are the fundamental rules of derivatives. Let us discuss them in detail.
The Power Rule simplifies the differentiation of power functions. If 𝑦=xⁿ, then the derivative 𝑑𝑦/𝑑𝑥 is given by 𝑛𝑥ⁿ⁻¹. For example, for 𝑓(𝑥)=𝑥⁵, the derivative 𝑓′(𝑥) would be 5𝑥⁴
This rule states that the derivative of the sum or difference of two functions is the sum or difference of their derivatives. Mathematically,
dy/dx [u ± v]= du/dx ± dv/dx.
This rule is particularly useful for handling complex expressions composed of simpler terms.
The product rule of derivatives states that if a function is a product of two functions, then its derivative is the derivative of the second function multiplied by the first function added to the derivative of the first function multiplied by the second function.
dy/dx [u × v] = u · dv/dx + v · du/dx. If y = x⁵eˣ , we have y’ = x5 . eˣ + eˣ. 5x⁴ = eˣ (x⁵ + 5x⁴)
When differentiating a quotient of two functions, the Quotient Rule is applied. It is given by d/dx (u/v) = (v · du/dx – u · dv/dx)/ v² This formula is essential for functions where one function is divided by another.
This rule states that the derivative of a constant multiplied by a function is the constant multiplied by the derivative of the function. Formally, if 𝑦=𝑐⋅𝑓(𝑥), then d/dx [c(f(x)] = c · d/dx f(x).Constant Rule
The Constant Rule states that the derivative of a constant value is zero. If 𝑦=𝑘 where k is a constant, then 𝑑𝑦/𝑑𝑥=0. This result follows logically from the Power Rule as the power of x in a constant is zero.
If f and g are differentiable functions within their respective domains, then the composite function 𝑓(𝑔(𝑥)) is also differentiable. This principle is encapsulated by the chain rule for differentiation, which is essential for handling composite functions. The derivative of the composite function (𝑓∘𝑔)′(𝑥) is given by:
(𝑓∘𝑔)′(𝑥)=𝑓′[𝑔(𝑥)]⋅𝑔′(𝑥)
Alternatively, this can be expressed as: If 𝑦=𝑓(𝑢) and 𝑢=𝑔(𝑥), then 𝑑𝑦/𝑑𝑥=𝑑𝑦/𝑑𝑢⋅𝑑𝑢/𝑑𝑥
For instance, consider the function 𝑦=tan²(𝑥). This function is composite, allowing us to define it in terms of 𝑢, where 𝑢=tan(𝑥)), and thus 𝑦=𝑢². Calculating the derivatives, we have:
Applying the chain rule, the derivative of 𝑦 with respect to 𝑥 is:
𝑑𝑦/𝑑𝑥=𝑑𝑦/𝑑𝑢⋅𝑑𝑢/𝑑𝑥=2𝑢⋅sec²(𝑥)=2tan(𝑥)sec²(𝑥)
In situations where 𝑦 is a function of 𝑥 but cannot be explicitly expressed using the variables 𝑥 and 𝑦, we use a method called implicit differentiation. This technique is especially useful when the relationship between 𝑥 and 𝑦 forms an equation that can’t easily be solved for 𝑦. To apply implicit differentiation, you start by differentiating both sides of the equation with respect to 𝑥.
For example, consider the equation 2𝑥+𝑦=12. To find the derivative of 𝑦 with respect to 𝑥, differentiate each term of the equation with respect to 𝑥:
d/dx(2x+y)=d/dx(12)
The derivative of 2x with respect to 𝑥 is 2, and since y is a function of 𝑥, its derivative becomes 𝑑𝑦/𝑑𝑥. The derivative of a constant, like 12, is 0:
2+dx/dy=0
To isolate 𝑑𝑦/𝑑𝑥, solve for it:
𝑑𝑦/𝑑𝑥=−2
In a function, we may have the dependent variables x and y which are dependent on the third independent variable. If x = f(t) and y = g(t), then derivative is calculated as dy/dx = f'(x)/g'(x).
Higher-order derivatives refer to the derivatives taken multiple times on a function. The first derivative of a function represents the rate at which the function’s output changes with respect to its input. The second derivative, the derivative of the first derivative, provides information about the curvature or the acceleration of the function. Similarly, third-order derivatives and beyond can also be calculated.
The notation for higher-order derivatives can vary. For a function 𝑦=𝑓(𝑥), the first three derivatives are often denoted as:
If u = f(x,y) we can find the partial derivative of with respect to y by keeping x as the constant or we can find the partial derivative with respect to x by keeping y as the constant. Suppose f(x, y) = x³ y² , the partial derivatives of the function are:
∂f/∂x(x³ y²) = 3x²y and
∂f/∂y(x³ y²) = x³ 2y
Further, we can find the second-order partial derivatives also like ∂²f/∂x², ∂²f/∂y², ∂²f/∂x ∂y, and ∂²f/∂y ∂x.
In instances where functions are highly complex or involve one function raised to the power of another, such as 𝑦=𝑓(𝑥)ᵍ⁽ˣ⁾, traditional methods of differentiation may not suffice. To tackle these challenges, we can employ a technique called logarithmic differentiation. This method involves taking the natural logarithm (ln) of both sides of the equation, simplifying using logarithmic properties, and then differentiating both sides with respect to 𝑥. Here’s how logarithmic differentiation is typically applied:
Maxima and minima refer to the highest and lowest values, respectively, that a function achieves within a given range. A maximum point on a function is where the function value is higher than at any other point nearby, while a minimum point is where the function value is lower than at any point in its vicinity. These points can be categorized as either local or global:
Problem 1: Calculate the derivative of the function: 𝑓(𝑥)=7𝑥⁴
Solution: Using the power rule, 𝑓′(𝑥)=4⋅7𝑥⁴⁻¹=28𝑥³.
Problem 2: Find the derivative of the function: 𝑔(𝑥)=𝑥²⋅ln(𝑥)
Solution:
Using the product rule, 𝑔′(𝑥)=(𝑥²)′⋅ln(𝑥)+𝑥²⋅(ln(𝑥))′
𝑔′(𝑥)=2𝑥⋅ln(𝑥)+𝑥²⋅1/𝑥=2𝑥ln(𝑥)+𝑥
Derivatives are financial instruments whose value is derived from the performance of underlying assets such as stocks, bonds, commodities, or market indexes. They are used primarily for hedging risk or speculating on price movements of the underlying assets.
The four main types of derivatives are:
The basic concept of derivatives is to provide a way to manage financial risk by allowing investors to hedge against potential losses or speculate on changes in the underlying asset’s price. Derivatives set terms for buying or selling assets at future dates, based on predictions of asset price movements.
In real life, derivatives are used in various ways:
Making money from derivatives involves strategies like hedging, which protects against losses in other investments, and speculation, where traders predict market movements to profit from price discrepancies. Success in derivatives trading requires understanding market conditions and the behavior of the underlying assets.

In simple terms, the derivative of a function measures how the output value of a function changes as the input changes. It is often represented as the slope of the tangent line at any point of the function graph. The derivative at a point tells us the rate at which the function’s value is changing at that point.
Derivatives are an essential component of calculus, serving as a powerful tool to measure the sensitivity of one quantity in relation to changes in another. This concept is foundational in analyzing how functions change, which is vital for modeling dynamic systems across various scientific and mathematical disciplines.
The formula for the derivative of a function with respect to a real variable can be expressed mathematically as:

The three foundational categories of derivatives—algebraic, logarithmic/exponential, and trigonometric—are derived from the first principles of differentiation. These categories, which include derivatives of algebraic expressions, logarithms, exponents, and trigonometric functions, form the core of standard derivative formulas, crucial tools in calculus. Each type serves a specific function and is derived by applying the fundamental concept of differentiation. This comprehensive breakdown highlights the essential nature of these derivatives in various mathematical and real-world applications
Algebraic Functions: These involve polynomials or rational functions where the derivative is obtained using basic rules such as the power rule.
Logarithmic and Exponential Functions: The derivatives of functions like 𝑒𝑥 and ln(𝑥) are foundational in studying natural growth processes and decay.
Trigonometric Functions: Derivatives of sine, cosine, and other trigonometric functions are pivotal in physics and engineering for modeling periodic behavior.
Using the example above, the derivative of 𝑥² is 2𝑥. Following this pattern, we can also determine that the derivative of 𝑥³ is 3𝑥², and the derivative of 𝑥⁴ is 4𝑥³ This observation leads us to the Power Rule in differentiation, which generalizes this concept for any power of 𝑥x. The Power Rule is formally stated as:
d/dx(xⁿ)=nxⁿ⁻¹
The derivative of ln x is, d/dx (ln x) = 1/x
The derivative of log x is, d/dx (logₐ x) = 1/(x ln a)
The derivative of e^x is, d/dx (eˣ) = eˣ
The derivative of a^x is, d/dx (aˣ) = aˣ ln a

If y = sin x, y’ = cos x
If y = cos x, y’ = -sin x
If y = tan x, y’ = sec² x
If y = cot x, y’ = -cosec² x
If y = sec x, y’ = sec x tan x
If y = cosec x, y’ = -cosec x cot x
Here are the derivatives of trigonometric functions

The derivative of inverse sine is, d/dx (sin⁻¹x) = 1/√(1-x²)
The derivative of inverse cosine is, d/dx (cos⁻¹x) = -1/√(1-x²)
The derivative of inverse tan is, d/dx (tan⁻¹x) = 1/(1 + x²)
The derivative of inverse cot is, d/dx (cot⁻¹x) = -1/(1 + x²)
The derivative of inverse cosec is, d/dx (csc⁻¹x) = -1/ [|x| √(x² – 1) ], x ≠ 1, -1, 0
The derivative of inverse sec is, d/dx (sec⁻¹x) = 1/ [|x| √(x² – 1) ], x ≠ 1, -1, 0
The following are the fundamental rules of derivatives. Let us discuss them in detail.
The Power Rule simplifies the differentiation of power functions. If 𝑦=xⁿ, then the derivative 𝑑𝑦/𝑑𝑥 is given by 𝑛𝑥ⁿ⁻¹. For example, for 𝑓(𝑥)=𝑥⁵, the derivative 𝑓′(𝑥) would be 5𝑥⁴
This rule states that the derivative of the sum or difference of two functions is the sum or difference of their derivatives. Mathematically,
dy/dx [u ± v]= du/dx ± dv/dx.
This rule is particularly useful for handling complex expressions composed of simpler terms.
The product rule of derivatives states that if a function is a product of two functions, then its derivative is the derivative of the second function multiplied by the first function added to the derivative of the first function multiplied by the second function.
dy/dx [u × v] = u · dv/dx + v · du/dx. If y = x⁵eˣ , we have y’ = x5 . eˣ + eˣ. 5x⁴ = eˣ (x⁵ + 5x⁴)
When differentiating a quotient of two functions, the Quotient Rule is applied. It is given by d/dx (u/v) = (v · du/dx – u · dv/dx)/ v² This formula is essential for functions where one function is divided by another.
This rule states that the derivative of a constant multiplied by a function is the constant multiplied by the derivative of the function. Formally, if 𝑦=𝑐⋅𝑓(𝑥), then d/dx [c(f(x)] = c · d/dx f(x).Constant Rule
The Constant Rule states that the derivative of a constant value is zero. If 𝑦=𝑘 where k is a constant, then 𝑑𝑦/𝑑𝑥=0. This result follows logically from the Power Rule as the power of x in a constant is zero.
If f and g are differentiable functions within their respective domains, then the composite function 𝑓(𝑔(𝑥)) is also differentiable. This principle is encapsulated by the chain rule for differentiation, which is essential for handling composite functions. The derivative of the composite function (𝑓∘𝑔)′(𝑥) is given by:
(𝑓∘𝑔)′(𝑥)=𝑓′[𝑔(𝑥)]⋅𝑔′(𝑥)
Alternatively, this can be expressed as: If 𝑦=𝑓(𝑢) and 𝑢=𝑔(𝑥), then 𝑑𝑦/𝑑𝑥=𝑑𝑦/𝑑𝑢⋅𝑑𝑢/𝑑𝑥
For instance, consider the function 𝑦=tan²(𝑥). This function is composite, allowing us to define it in terms of 𝑢, where 𝑢=tan(𝑥)), and thus 𝑦=𝑢². Calculating the derivatives, we have:
𝑑𝑦/𝑑𝑢=2𝑢 since the derivative of 𝑢2 with respect to 𝑢 is 2𝑢.
𝑑𝑢/𝑑𝑥=sec²(𝑥) because the derivative of tan(𝑥) with respect to 𝑥 is sec²(𝑥).
Applying the chain rule, the derivative of 𝑦 with respect to 𝑥 is:
𝑑𝑦/𝑑𝑥=𝑑𝑦/𝑑𝑢⋅𝑑𝑢/𝑑𝑥=2𝑢⋅sec²(𝑥)=2tan(𝑥)sec²(𝑥)
In situations where 𝑦 is a function of 𝑥 but cannot be explicitly expressed using the variables 𝑥 and 𝑦, we use a method called implicit differentiation. This technique is especially useful when the relationship between 𝑥 and 𝑦 forms an equation that can’t easily be solved for 𝑦. To apply implicit differentiation, you start by differentiating both sides of the equation with respect to 𝑥.
For example, consider the equation 2𝑥+𝑦=12. To find the derivative of 𝑦 with respect to 𝑥, differentiate each term of the equation with respect to 𝑥:
d/dx(2x+y)=d/dx(12)
The derivative of 2x with respect to 𝑥 is 2, and since y is a function of 𝑥, its derivative becomes 𝑑𝑦/𝑑𝑥. The derivative of a constant, like 12, is 0:
2+dx/dy=0
To isolate 𝑑𝑦/𝑑𝑥, solve for it:
𝑑𝑦/𝑑𝑥=−2
In a function, we may have the dependent variables x and y which are dependent on the third independent variable. If x = f(t) and y = g(t), then derivative is calculated as dy/dx = f'(x)/g'(x).
Higher-order derivatives refer to the derivatives taken multiple times on a function. The first derivative of a function represents the rate at which the function’s output changes with respect to its input. The second derivative, the derivative of the first derivative, provides information about the curvature or the acceleration of the function. Similarly, third-order derivatives and beyond can also be calculated.
The notation for higher-order derivatives can vary. For a function 𝑦=𝑓(𝑥), the first three derivatives are often denoted as:
First derivative: 𝑓′(𝑥) or 𝑑𝑦/𝑑𝑥
Second derivative: 𝑓′′(𝑥) or 𝑑²𝑦/𝑑𝑥
Third derivative: 𝑓′′′(𝑥) or 𝑑³𝑦/𝑑𝑥
If u = f(x,y) we can find the partial derivative of with respect to y by keeping x as the constant or we can find the partial derivative with respect to x by keeping y as the constant. Suppose f(x, y) = x³ y² , the partial derivatives of the function are:
∂f/∂x(x³ y²) = 3x²y and
∂f/∂y(x³ y²) = x³ 2y
Further, we can find the second-order partial derivatives also like ∂²f/∂x², ∂²f/∂y², ∂²f/∂x ∂y, and ∂²f/∂y ∂x.
In instances where functions are highly complex or involve one function raised to the power of another, such as 𝑦=𝑓(𝑥)ᵍ⁽ˣ⁾, traditional methods of differentiation may not suffice. To tackle these challenges, we can employ a technique called logarithmic differentiation. This method involves taking the natural logarithm (ln) of both sides of the equation, simplifying using logarithmic properties, and then differentiating both sides with respect to 𝑥. Here’s how logarithmic differentiation is typically applied:
Start by taking the natural logarithm of both sides: ln(𝑦)=ln(𝑓(𝑥)𝑔(𝑥)).
Simplify using the logarithm power rule: ln(𝑦)=𝑔(𝑥)⋅ln(𝑓(𝑥))
Differentiate both sides with respect to 𝑥, applying the chain rule on the left side and the product rule on the right side.
Solve for 𝑑𝑦/𝑑𝑥 to find the derivative of the original function
Maxima and minima refer to the highest and lowest values, respectively, that a function achieves within a given range. A maximum point on a function is where the function value is higher than at any other point nearby, while a minimum point is where the function value is lower than at any point in its vicinity. These points can be categorized as either local or global:
Local maximum (or minimum): The function value is the highest (or lowest) in a small surrounding neighborhood.
Global maximum (or minimum): The function value is the highest (or lowest) across the entire domain of the function.
Find the derivative: Calculate the first derivative 𝑓′(𝑥) of the function 𝑓(𝑥). This derivative represents the rate of change of the function.
Set the derivative to zero: Solve the equation 𝑓′(𝑥)=0 to find the critical points. These are potential candidates for maxima and minima since the slope of the tangent at these points is zero.
Determine the nature of critical points: To classify each critical point as a maximum, minimum, or neither, use the second derivative test:
Compute the second derivative 𝑓′′(𝑥).
Substitute the critical points into 𝑓′′(𝑥):
If 𝑓′′(𝑥)>0, the function has a local minimum at that point.
If 𝑓′′(𝑥)<0, the function has a local maximum at that point.
If 𝑓′′(𝑥)=0, the test is inconclusive, and further analysis is required (possibly using higher derivatives or other methods).
Problem 1: Calculate the derivative of the function: 𝑓(𝑥)=7𝑥⁴
Solution: Using the power rule, 𝑓′(𝑥)=4⋅7𝑥⁴⁻¹=28𝑥³.
Problem 2: Find the derivative of the function: 𝑔(𝑥)=𝑥²⋅ln(𝑥)
Solution:
Using the product rule, 𝑔′(𝑥)=(𝑥²)′⋅ln(𝑥)+𝑥²⋅(ln(𝑥))′
𝑔′(𝑥)=2𝑥⋅ln(𝑥)+𝑥²⋅1/𝑥=2𝑥ln(𝑥)+𝑥
Derivatives are financial instruments whose value is derived from the performance of underlying assets such as stocks, bonds, commodities, or market indexes. They are used primarily for hedging risk or speculating on price movements of the underlying assets.
The four main types of derivatives are:
Futures: Contracts to buy or sell an asset at a predetermined future date and price.
Options: Contracts that give the right, but not the obligation, to buy or sell an asset at a set price before a certain date.
Swaps: Agreements to exchange one set of cash flows for another.
Forwards: Customized contracts to buy or sell an asset at a set price and date, typically used by institutions.
The basic concept of derivatives is to provide a way to manage financial risk by allowing investors to hedge against potential losses or speculate on changes in the underlying asset’s price. Derivatives set terms for buying or selling assets at future dates, based on predictions of asset price movements.
In real life, derivatives are used in various ways:
Businesses use them to hedge against changes in commodity prices or currency exchange rates.
Investors use them to speculate on price movements without actually owning the underlying asset.
Insurance companies use them to manage risk exposure.
Making money from derivatives involves strategies like hedging, which protects against losses in other investments, and speculation, where traders predict market movements to profit from price discrepancies. Success in derivatives trading requires understanding market conditions and the behavior of the underlying assets.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Find the derivative of f(x) = 3x²+ 4x + 5.
6x+4
6x+5
3x²+4
3x+4
Find the derivative of g(x) = eˣ.
eˣ
eˣ + 1
xeˣ
eˣ - 1
Calculate the derivative of h(x) = ln(x).
xln(x)
ln(x)
1/X
1/X + 1
Find the derivative of f(x) = sin(x).
cos(x)
−sin(x)
sin(x)
−cos(x)
Determine the derivative of f(x) = cos(x).
cos(x)
−sin(x)
sin(x)
−cos(x)
Find the derivative of f(x) = x³.
2x²
3x²
x³
3x
Find the derivative of f(x) = x² sin(x) using the product rule.
x²cos(x) − 2xsin(x)
2xcos(x) − x²sin(x)
xcos(x) + 2x²sin(x)
2xsin(x) + x²cos(x)
Determine the derivative of f(x) = ln(3x).
1/X
3/X
1/3X
1/X
Find the derivative of f(x) = 5x³ − 2x² + 4x − 7.
15x² − 4x + 4
15x² − 4x + 4 − 7
15x² − 4x + 7
15x² − 2x + 4
Determine the derivative of f(x)=e²ˣ.
e²ˣ
2eˣ
2e²ˣ
eˣ
Before you leave, take our quick quiz to enhance your learning!

