What is the decimal representation of 3/4?
0.75
0.70
0.80
0.85

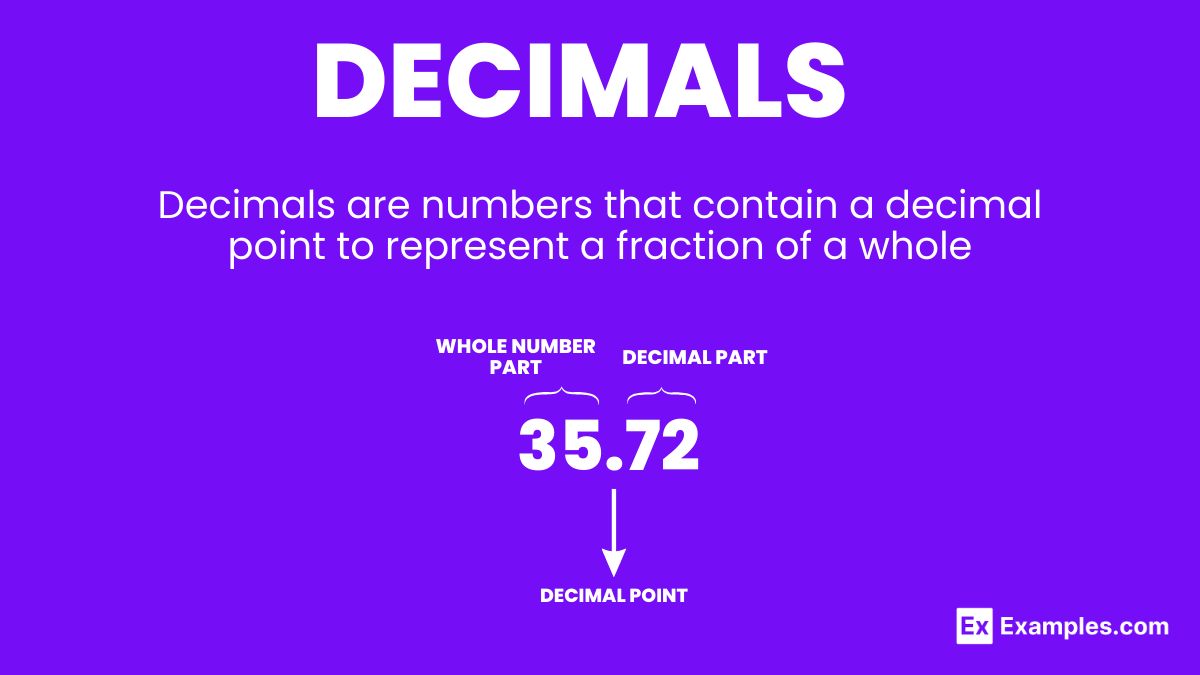
Decimals are a fundamental concept in mathematics, representing numbers between whole numbers. Understanding decimals is crucial for students to excel in math, especially in topics like measurement, money, and fractions. This guide breaks down decimals into simple, easy-to-grasp concepts with practical examples, helping teachers convey these ideas effectively to students. Whether it’s adding, subtracting, multiplying, or dividing, decimals play a key role in everyday math applications, making this guide essential for classroom success.
Decimals are numbers that contain a decimal point to represent a fraction of a whole. Unlike whole numbers, decimals allow for greater precision by dividing a number into parts smaller than one. Think of them as an extension of the place value system, where each position to the right of the decimal point represents tenths, hundredths, thousandths, and so on. For example, the decimal 0.5 signifies half of a whole, and 1.75 represents one whole plus three-quarters of another. Understanding decimals is vital for accurate measurement, financial calculations, and scientific data representation.
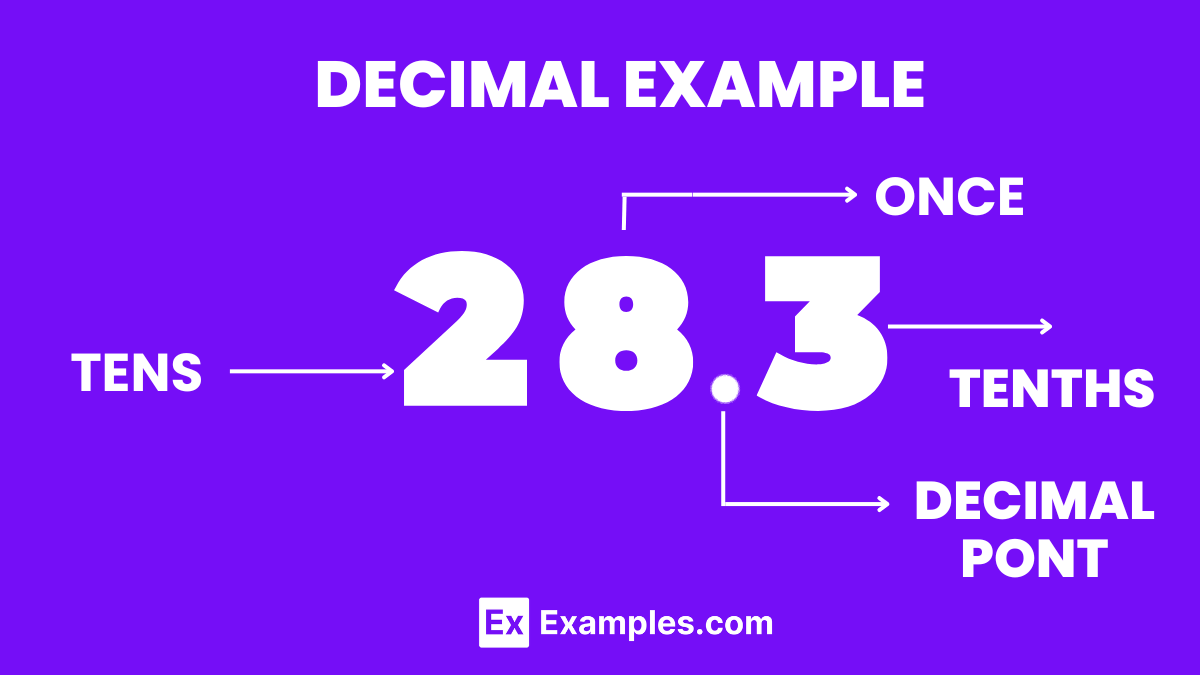
Decimal numbers are a crucial part of mathematics, representing values between whole numbers. They consist of a whole number part, a decimal point, and a fractional part.
Each type plays a specific role in mathematics and real-world applications, helping students understand the nuances of numerical values.
The place value system in decimals extends the concept of whole numbers to include positions to the right of the decimal point. Each place represents a fraction of ten. For instance, in the decimal number 3.142, the 1 signifies one-tenth (0.1), the 4 represents four one-hundredths (0.04), and the 2 stands for two one-thousandths (0.002). Understanding place value is essential for performing arithmetic operations with decimals and helps students grasp the concept of magnitude and precision in numerical data.
Decimals exhibit unique properties that make them fundamental in mathematics. Key properties include:
These properties ensure consistency and predictability in calculations, aiding in the teaching and understanding of mathematical concepts involving decimals.
Performing arithmetic operations on decimals is fundamental in mathematics, enabling precise calculations. These operations include addition, subtraction, multiplication, and division, each requiring attention to decimal place alignment for accurate results.
Converting decimals to fractions involves understanding the place value of the decimal and simplifying the resulting fraction to its lowest terms.
These examples illustrate the direct relationship between decimals and fractions, emphasizing the importance of place value and simplification in conversions.
The Decimal Place Value Chart is a tool used to understand and visualize the position and value of digits in a decimal number. It helps in breaking down the number into its constituent parts, such as tenths, hundredths, thousandths, etc., enhancing clarity and comprehension.
The Decimal Place Value Chart is instrumental in teaching the concept of decimals, offering a visual representation of how each digit’s position affects its value. It underscores the relationship between places to the left and right of the decimal point, facilitating a deeper understanding of decimals.
This comprehensive guide outlines the significance and utility of the Decimal Place Value Chart in teaching and understanding decimals, providing a solid foundation for arithmetic operations and conceptual clarity.
Decimal problems involve scenarios where operations on decimal numbers are required to find solutions. These can range from basic arithmetic to more complex applications in real-world contexts, helping students to understand decimals’ practicality and develop their numerical skills.
Decimals play a vital role in mathematics and everyday life, offering precision in measurements, calculations, and financial transactions. Understanding their properties, operations, and applications enhances numerical literacy and problem-solving skills. This guide has explored various aspects of decimals, from basic concepts to real-world applications, aiming to provide a comprehensive understanding that empowers both teachers and students to navigate the world of decimals with confidence and clarity.

Decimals are a fundamental concept in mathematics, representing numbers between whole numbers. Understanding decimals is crucial for students to excel in math, especially in topics like measurement, money, and fractions. This guide breaks down decimals into simple, easy-to-grasp concepts with practical examples, helping teachers convey these ideas effectively to students. Whether it’s adding, subtracting, multiplying, or dividing, decimals play a key role in everyday math applications, making this guide essential for classroom success.
Decimals are numbers that contain a decimal point to represent a fraction of a whole. Unlike whole numbers, decimals allow for greater precision by dividing a number into parts smaller than one. Think of them as an extension of the place value system, where each position to the right of the decimal point represents tenths, hundredths, thousandths, and so on. For example, the decimal 0.5 signifies half of a whole, and 1.75 represents one whole plus three-quarters of another. Understanding decimals is vital for accurate measurement, financial calculations, and scientific data representation.

Decimal numbers are a crucial part of mathematics, representing values between whole numbers. They consist of a whole number part, a decimal point, and a fractional part.
Terminating Decimals: These decimals have a finite number of digits after the decimal point. For example, 0.75 has two digits after the decimal point and does not continue indefinitely.
Repeating Decimals: These decimals have one or more repeating digits after the decimal point. An example is 0.333…, where the 3 repeats indefinitely.
Non-repeating, Non-terminating Decimals: These decimals do not have a repeating pattern and continue indefinitely without terminating. An example is the decimal representation of π (pi), which is approximately 3.14159…
Each type plays a specific role in mathematics and real-world applications, helping students understand the nuances of numerical values.
The place value system in decimals extends the concept of whole numbers to include positions to the right of the decimal point. Each place represents a fraction of ten. For instance, in the decimal number 3.142, the 1 signifies one-tenth (0.1), the 4 represents four one-hundredths (0.04), and the 2 stands for two one-thousandths (0.002). Understanding place value is essential for performing arithmetic operations with decimals and helps students grasp the concept of magnitude and precision in numerical data.
Decimals exhibit unique properties that make them fundamental in mathematics. Key properties include:
Commutative Property of Addition and Multiplication: The order of adding or multiplying decimals does not change the result.
For example, 0.5 + 0.3 = 0.3 + 0.5, and 0.2 * 0.4 = 0.4 * 0.2.
Associative Property of Addition and Multiplication: When adding or multiplying decimals, the grouping does not affect the outcome.
For example, (0.5 + 0.2) + 0.3 = 0.5 + (0.2 + 0.3).
Distributive Property: Multiplication over addition or subtraction holds true for decimals. For instance, 0.3 * (0.5 + 0.2) = (0.3 * 0.5) + (0.3 * 0.2).
These properties ensure consistency and predictability in calculations, aiding in the teaching and understanding of mathematical concepts involving decimals.
Performing arithmetic operations on decimals is fundamental in mathematics, enabling precise calculations. These operations include addition, subtraction, multiplication, and division, each requiring attention to decimal place alignment for accurate results.
Addition: 0.75 + 0.25 = 1.00
Adding two decimals, align the decimal points to ensure each place value is correctly summed, resulting in a whole number.
Subtraction: 2.5 – 0.5 = 2.00
Subtracting decimals involves aligning the decimal points and subtracting each digit in its respective place value.
Multiplication: 0.5 * 2 = 1.0
When multiplying, the total number of decimal places in the product equals the sum of the decimal places in the factors.
Division: 1.5 ÷ 0.5 = 3
Dividing decimals requires converting the divisor to a whole number by adjusting the dividend accordingly, facilitating straightforward division.
Mixed Operations: (0.75 + 1.25) * 2 = 4.00
Combining operations, follow the order of operations (PEMDAS/BODMAS) to achieve accurate results.
Subtracting Larger from Smaller: 0.5 – 1.0 = -0.5
When the minuend is smaller, the result is a negative decimal, indicating the difference.
Multiplying by Powers of 10: 0.25 * 100 = 25
Multiplying decimals by powers of 10 shifts the decimal point to the right, increasing the value.
Converting decimals to fractions involves understanding the place value of the decimal and simplifying the resulting fraction to its lowest terms.
Terminating Decimal to Fraction: 0.5 = 1/2
Recognizing 0.5 represents half, it directly converts to the fraction 1/2.
Repeating Decimal to Fraction: 0.\overline{3} = 1/3
A repeating decimal like 0.\overline{3} signifies an infinite series of 3s, equivalent to the fraction 1/3.
Non-Repeating Decimal: 0.25 = 1/4
The decimal 0.25, representing twenty-five hundredths, simplifies to the fraction 1/4.
Complex Repeating Decimal: 0.\overline{66} = 2/3
A repeating decimal such as 0.\overline{66} indicates a pattern that simplifies to 2/3 as a fraction.
Small Decimal Value: 0.01 = 1/100
The decimal 0.01 signifies one hundredth, directly converting to the fraction 1/100.
Large Decimal to Fraction: 2.75 = 11/4
A decimal with a whole number part, like 2.75, converts to a mixed number, then to an improper fraction, 11/4.
Decimal with Multiple Digits: 0.125 = 1/8
Understanding 0.125 represents one hundred twenty-five thousandths simplifies to the fraction 1/8.
These examples illustrate the direct relationship between decimals and fractions, emphasizing the importance of place value and simplification in conversions.
The Decimal Place Value Chart is a tool used to understand and visualize the position and value of digits in a decimal number. It helps in breaking down the number into its constituent parts, such as tenths, hundredths, thousandths, etc., enhancing clarity and comprehension.
0.789 on the Chart
Tenths: 7
Hundredths: 8
Thousandths: 9
The digit 7 is in the tenths place, 8 in the hundredths, and 9 in the thousandths, illustrating the value of each decimal place.
3.04 on the Chart
Ones: 3
Tenths: 0
Hundredths: 4
The number 3 is in the ones place, showing the whole number part, while 4 is in the hundredths place, indicating a fractional part.
5.600 on the Chart
Ones: 5
Tenths: 6
Hundredths: 0
Thousandths: 0
This demonstrates how zeros in the hundredths and thousandths places indicate precision but do not add to the decimal’s value.
0.003 on the Chart
Thousandths: 3
This example highlights the importance of zeros preceding the 3 in indicating its place value as thousandths.
12.345 on the Chart
Tens: 1
Ones: 2
Tenths: 3
Hundredths: 4
Thousandths: 5
Each digit’s place value is clearly defined, showing both the whole number and the decimal parts.
100.001 on the Chart
Hundreds: 1
Ones: 0
Thousandths: 1
This shows a large whole number with a very small decimal part, emphasizing the chart’s range.
0.9999 on the Chart
Tenths: 9
Hundredths: 9
Thousandths: 9
Ten-thousandths: 9
Demonstrating the decimal places extending beyond thousandths, useful for high precision numbers.
The Decimal Place Value Chart is instrumental in teaching the concept of decimals, offering a visual representation of how each digit’s position affects its value. It underscores the relationship between places to the left and right of the decimal point, facilitating a deeper understanding of decimals.
Identifying Place Value:
Recognizing that each step to the left or right changes the digit’s value tenfold is fundamental. For example, moving from the tenths to the hundredths place divides the value by 10.
Comparing Decimals:
Using the chart to compare two decimals, such as 0.5 and 0.05, illustrates the importance of place value in determining which is larger.
Adding and Subtracting Decimals:
Aligning decimals on the chart ensures that digits are correctly lined up by their place value for accurate addition or subtraction.
Multiplying Decimals:
Understanding how multiplication affects the place value and the overall number of digits in the product.
Dividing Decimals:
Visualizing the shift in place value as decimals are divided, highlighting how division impacts the decimal’s position and value.
Rounding Decimals:
The chart helps in determining which digit to look at when rounding to a certain place value, making it easier to understand the rounding process.
Converting Decimals to Fractions:
By identifying the place value of the last digit in a decimal, one can convert it to an equivalent fraction accurately.
This comprehensive guide outlines the significance and utility of the Decimal Place Value Chart in teaching and understanding decimals, providing a solid foundation for arithmetic operations and conceptual clarity.
Decimal problems involve scenarios where operations on decimal numbers are required to find solutions. These can range from basic arithmetic to more complex applications in real-world contexts, helping students to understand decimals’ practicality and develop their numerical skills.
Calculating Money:
Problem: If you have $5.75 and you spend $2.45, how much money do you have left?
Explanation: This problem teaches subtraction with decimals, reflecting a common real-life scenario.
Measuring Lengths:
Problem: A rope is 3.5 meters long. If you cut off 1.25 meters, how long is the remaining piece?
Explanation: This requires subtraction and introduces decimals in the context of measurement.
Multiplying Quantities:
Problem: If one liter of paint covers 2.5 square meters, how much area would 3 liters cover?
Explanation: This illustrates multiplication with decimals, applying it to calculate area coverage.
Dividing Portions:
Problem: A pie is cut into 8 equal slices. If each slice is 0.125 of the whole pie, how many slices do you get from half a pie?
Explanation: It involves division and multiplication, teaching fractions to decimals conversion.
Temperature Changes:
Problem: If the temperature rises from 17.5°C to 20.1°C, what is the increase?
Explanation: This scenario requires subtraction and introduces decimals in a scientific context.
Speed Calculation:
Problem: If a car travels 150.5 kilometers in 3 hours, what is its average speed in kilometers per hour?
Explanation: This problem teaches division with decimals, calculating rate of speed.
Mixing Solutions:
Problem: If you mix 250.75 ml of water with 100.25 ml of solution, what is the total volume of the mixture?
Explanation: It involves addition with decimals, applying it to volume measurements.
How do you read decimals?
Explanation: Decimals are read by stating the whole number part followed by “point” for the decimal point, and then reading each digit individually in the fractional part.
How do you convert fractions to decimals?
Explanation: To convert a fraction to a decimal, divide the numerator by the denominator using long division or a calculator.
What is the difference between a terminating and a non-terminating decimal?
Explanation: A terminating decimal has a finite number of digits after the decimal point, while a non-terminating decimal has an infinite number of digits without repeating patterns.
How do you add and subtract decimals?
Explanation: To add or subtract decimals, align the decimal points and add or subtract the numbers as you would with whole numbers, ensuring digits are correctly lined up.
How do you multiply decimals?
Explanation: Multiply the numbers ignoring the decimal points, then count the total number of digits to the right of the decimal points in the original numbers and place the decimal point in the product so that it has the same number of digits to the right.
How do you divide decimals?
Explanation: To divide by a decimal, you can multiply both the dividend and the divisor by the same power of 10 to make the divisor a whole number, then proceed with the division as usual, placing the decimal point in the quotient accordingly.
Decimals play a vital role in mathematics and everyday life, offering precision in measurements, calculations, and financial transactions. Understanding their properties, operations, and applications enhances numerical literacy and problem-solving skills. This guide has explored various aspects of decimals, from basic concepts to real-world applications, aiming to provide a comprehensive understanding that empowers both teachers and students to navigate the world of decimals with confidence and clarity.
Text prompt
Add Tone
Decimal Problems
Arithmetic Operations on Decimals
What is the decimal representation of 3/4?
0.75
0.70
0.80
0.85
Which decimal is equivalent to 0.6?
3/5
1/2
2/3
1/4
What is 7.8 rounded to the nearest whole number?
7
8
9
10
How do you convert 0.125 to a fraction?
1/8
1/4
1/10
1/2
Which of the following is the decimal form of 7/20?
0.35
0.30
0.40
0.45
What is the result of adding 4.25 and 3.75?
7.00
8.00
7.50
8.50
If you subtract 0.4 from 2.3, what is the result?
1.8
1.9
1.7
1.6
What is 0.875 expressed as a fraction in simplest form?
7/8
3/4
5/6
9/10
What is the product of 0.6 and 0.4?
0.20
0.24
0.26
0.28
Which decimal is greater: 0.75 or 0.7?
0.75
0.7
They are equal
Cannot be determined
Before you leave, take our quick quiz to enhance your learning!

